Can you find the side lengths of the triangle? | (No Calculators!) |#math #maths #geometry

PreMath
11 Mar 202411:54
EducationalLearning
32 Likes 10 Comments

TLDRIn this pre-calculus video, the presenter tackles the challenge of finding the side lengths of a right triangle without using a calculator. Given a 15° angle and an area of 1 cm², the video demonstrates the application of the Triangle Sum Theorem, the area formula for triangles, and the properties of a 30-60-90 special triangle. Through a series of logical steps and mathematical manipulations, the presenter successfully calculates the lengths of the triangle's sides, engaging viewers with a clear and methodical approach to problem-solving.

Takeaways
  • 📐 The video is a pre-calculus lesson focused on solving a right triangle problem without a calculator.
  • 📊 Given a right triangle with an area of 1 cm² and a 15° angle, the task is to find the side lengths AB, BC, and AC.
  • 🔢 The Triangle Sum Theorem is used to find that angle ACB is 75° (180° - 90° - 15°).
  • 🥂 The area formula for a triangle (area = 1/2 * base * height) is applied with AB as the base and BC as the height.
  • 📐 Assumptions are made: base AB is X and height BC is Y, leading to the equation X*Y = 2.
  • 🏹 By creating a new right triangle CBD and assuming an angle of 60°, it's deduced that triangle ADC is isosceles with CD = AD.
  • 📐 The special 30-60-90 triangle properties are used to determine the side ratios: shortest leg (opposite 30°) is half, longest leg (opposite 90°) is the shortest leg times √3.
  • 🔢 The side lengths are scaled by Y: BC becomes Y, CD and BD become Y*√3.
  • 📊 By equating the lengths and using the previously found X*Y = 2, the value of Y is solved to be √3 - 1.
  • 📐 Substituting Y back into the equation, X (AB) is found to be 2(√3 - 1) + (√3)².
  • 📐 Using the Pythagorean theorem, the third side AC is calculated to be 2*√2.
  • 👍 The video concludes with the solution and a reminder to subscribe for more educational content.
Q & A

  • What is the given angle in the right triangle ABC?

    -The given angle in the right triangle ABC is 15°.

  • What is the area of the right triangle ABC?

    -The area of the right triangle ABC is 1 square centimeter.

  • What is the Triangle Sum Theorem mentioned in the script?

    -The Triangle Sum Theorem states that the sum of the three interior angles of a triangle is always 180°.

  • How is the area of a triangle calculated?

    -The area of a triangle is calculated using the formula: area = 1/2 * base * height.

  • What are the assumptions made for the base and height of the right triangle?

    -The base of the right triangle is assumed to be X and the height is assumed to be Y.

  • What is the significance of the right triangle CBD?

    -The right triangle CBD is significant because it is a special 30-60-90 triangle, which has specific side length ratios.

  • What is the relationship between the smallest and largest leg in a 30-60-90 triangle?

    -In a 30-60-90 triangle, the longest leg is twice the length of the smallest leg.

  • How is the length of side CD determined in the right triangle ADC?

    -The length of side CD in the right triangle ADC is determined to be equal to the length of side AD, as triangle ADC is an isosceles triangle with angle CAD being 15°.

  • What is the final calculated length of side AB?

    -The final calculated length of side AB is √3 + 1.

  • What is the final calculated length of side AC?

    -The final calculated length of side AC is 2√2.

  • How is the Pythagorean theorem applied to find the length of side AC?

    -The Pythagorean theorem is applied by setting up the equation: a² + b² = c², where a and b are the lengths of the legs and c is the length of the hypotenuse. By substituting the values and simplifying, the length of side AC (c) is found to be 2√2.

Outlines
00:00
📚 Introduction to Premath - Solving a Right Triangle Problem

This paragraph introduces the video's focus on a pre-calculus problem involving a right triangle. The problem presents a triangle with a 15° angle and an area of 1 cm², challenging the viewer to calculate the side lengths without using a calculator. The video begins by recalling the Triangle Sum Theorem and proceeds to set up an equation based on the area of a triangle. The base and height of the triangle are denoted as X and Y, respectively, and the area equation is solved to find the relationship between X and Y.

05:02
🔍 Analyzing the Triangle and Establishing Relationships

The second paragraph delves into the analysis of the right triangle, creating a new triangle by connecting point C to point D. The speaker assumes an angle of 60° and uses the sum of angles in a triangle to deduce other angles. The triangle ADC is identified as isosceles, leading to the conclusion that side lengths CD and AD are equal. Focus then shifts to triangle BCD, recognized as a special 30-60-90 triangle. The properties of this triangle are used to establish the relationships between the sides, which are then substituted into the original area equation to solve for Y, the height of the original triangle.

10:05
📐 Applying the Pythagorean Theorem to Find the Final Side Length

The final paragraph concludes the problem-solving process by applying the Pythagorean theorem to find the length of the last remaining side, AC. The speaker designates the longest leg of the 30-60-90 triangle as C and the shorter leg as a, using these to calculate the hypotenuse (AC). Through a series of algebraic manipulations involving identities and simplifications, the speaker solves for the value of C and subsequently determines the length of AC. The video wraps up with the side lengths of the triangle AB (X), BC (y), and AC (C), and a reminder for viewers to subscribe for more content.

Mindmap
Keywords
💡Right Triangle
A right triangle is a type of triangle that has one angle measuring exactly 90 degrees. In the video, the right triangle ABC is used as the basis for the mathematical problem, with angle BAC being the right angle at 90 degrees.
💡Area
The area of a shape represents the amount of space enclosed by the shape. For triangles, the area is calculated by the formula: area = 1/2 * base * height. In the video, the area of the right triangle is given as 1 square centimeter, which is used to set up an equation to solve for the side lengths.
💡Triangle Sum Theorem
The Triangle Sum Theorem states that the sum of the interior angles of a triangle is always 180 degrees. This fundamental property of triangles is used in the video to find the measure of the third angle in the right triangle ABC when one angle is known.
💡Base and Height
In the context of triangles, the base and height refer to specific sides that are perpendicular to each other. The base is the side on which the height is measured from, and the height is the perpendicular distance from the base to the opposite vertex. These terms are crucial in calculating the area of a triangle.
💡Special 30-60-90 Triangle
A special 30-60-90 triangle is a right triangle where the angles are 30 degrees, 60 degrees, and 90 degrees. The side lengths of such a triangle have a specific ratio: the side opposite the 30-degree angle is half the length of the hypotenuse, and the side opposite the 60-degree angle is the length of the shortest side times the square root of 3.
💡Isosceles Triangle
An isosceles triangle is a triangle with at least two sides of equal length. In the video, the triangle ADC is identified as isosceles because it has two equal angles of 15 degrees, implying that the sides opposite these angles are also equal.
💡Pythagorean Theorem
The Pythagorean Theorem is a fundamental principle in geometry that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It is used to find the length of one side when the lengths of the other two sides are known.
💡Square Root
A square root is a mathematical operation that finds the value which, when multiplied by itself, gives the original number (the radicand). The square root is denoted by the symbol √ and is used in various mathematical calculations, including solving for side lengths in right triangles.
💡Algebraic Manipulation
Algebraic manipulation refers to the process of transforming and combining mathematical expressions using algebraic rules to solve equations. This includes operations such as factoring, expanding, and simplifying expressions.
💡Trigonometric Identities
Trigonometric identities are equations that involve trigonometric functions (such as sine, cosine, and tangent) and are always true. They are used to simplify and solve trigonometric equations. The script uses identities like a² - b² = (a + b)(a - b) and a + b = a² + b² / 2ab to manipulate and solve for the values of y and x.
💡Substitution
Substitution is a mathematical technique used to solve equations by replacing one variable with the expression or value of another. It is a fundamental algebraic method that helps in simplifying complex equations and finding the unknown values.
Highlights

The video begins with an introduction to a pre-calculus problem involving a right triangle ABC with a given area and a known angle of 15°.

The Triangle Sum Theorem is recalled to determine the remaining angle in the right triangle ABC, which is found to be 75°.

The area of the triangle is given as 1 cm², and the formula for the area of a triangle is used to establish a relationship between the base (AB) and height (BC) of the right triangle.

By setting up the equation AB * BC = 2, the product of the base and height of the triangle is determined without using a calculator.

A new right triangle CBD is introduced with an assumed angle of 60°, leading to the discovery that angle BCD is 30°.

Triangle ACD is identified as an isosceles triangle due to the two 15° angles, which allows for the conclusion that CD = AD.

Triangle BCD is recognized as a special 30-60-90 triangle, with the longest leg being twice the smallest leg.

The sides of the 30-60-90 triangle are scaled by a factor of y, leading to the equation 2y + y*√3 for the length of AB.

Equation x = 2y + √3*y is derived by substituting the expression for AB into the equation AB * BC = 2.

The value of y is solved by rationalizing the denominator and applying algebraic identities, resulting in y = √3 - 1.

The length of BC is calculated to be √3 - 1 by substituting the value of y into the equation for BC.

The length of AB is found to be √3 + 1 by substituting the value of y into the equation for AB.

The Pythagorean theorem is applied to find the length of AC, with a and b representing the legs of the triangle and c the hypotenuse.

After applying algebraic identities and simplifying, the length of AC is determined to be 2*√2.

The video concludes with the lengths of the triangle's sides AB, BC, and AC being √3 + 1, √3 - 1, and 2*√2 respectively.

The presenter encourages viewers to subscribe for more educational content, highlighting the practical application of the mathematical concepts discussed.

Transcripts

Browse More Related Video

Special Right Triangles - 30 60 90 - Geometry & Trigonometry | SAT Math

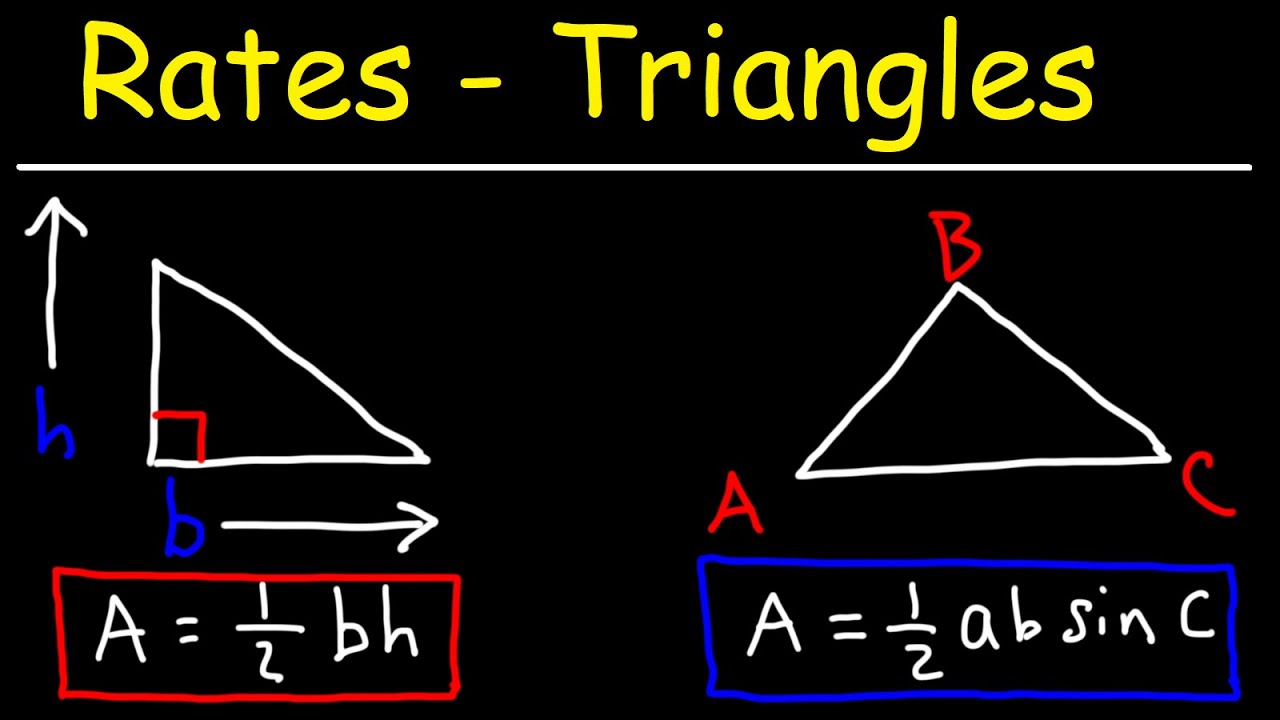
Related Rates - Area of a Triangle

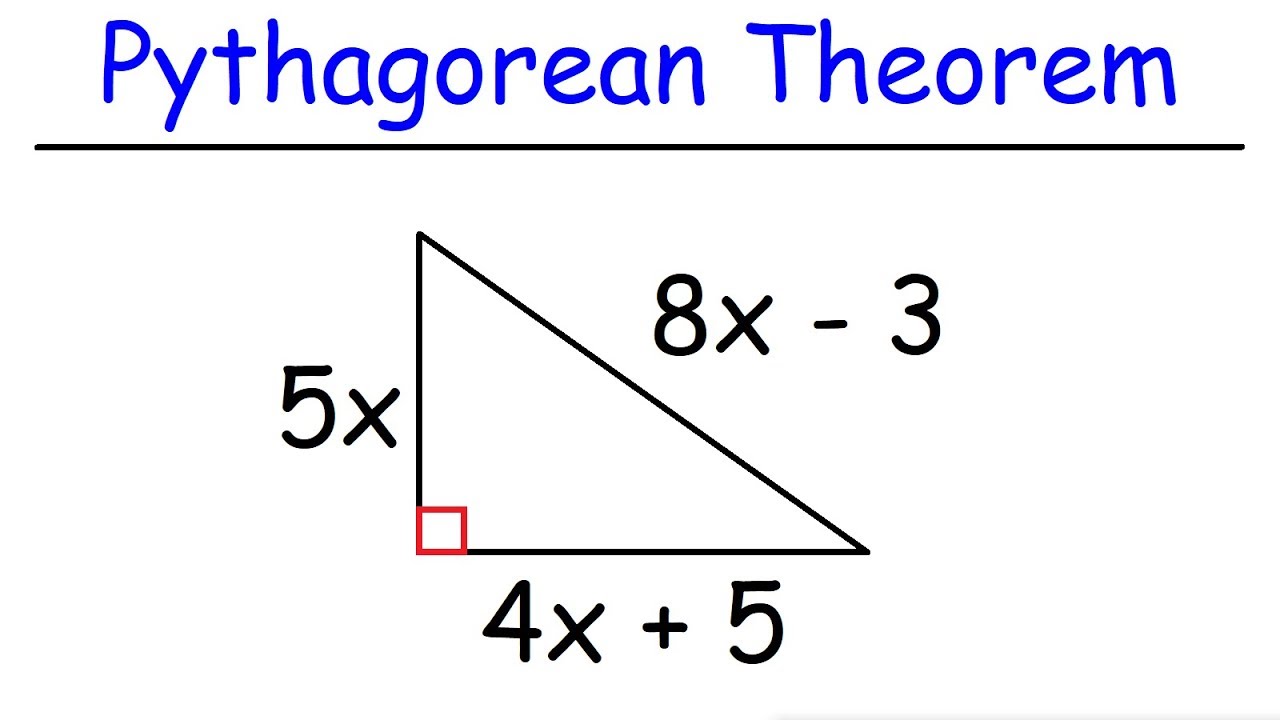
Pythagorean Theorem - Basic Introduction

30-60-90 Triangles - Special Right Triangle Trigonometry

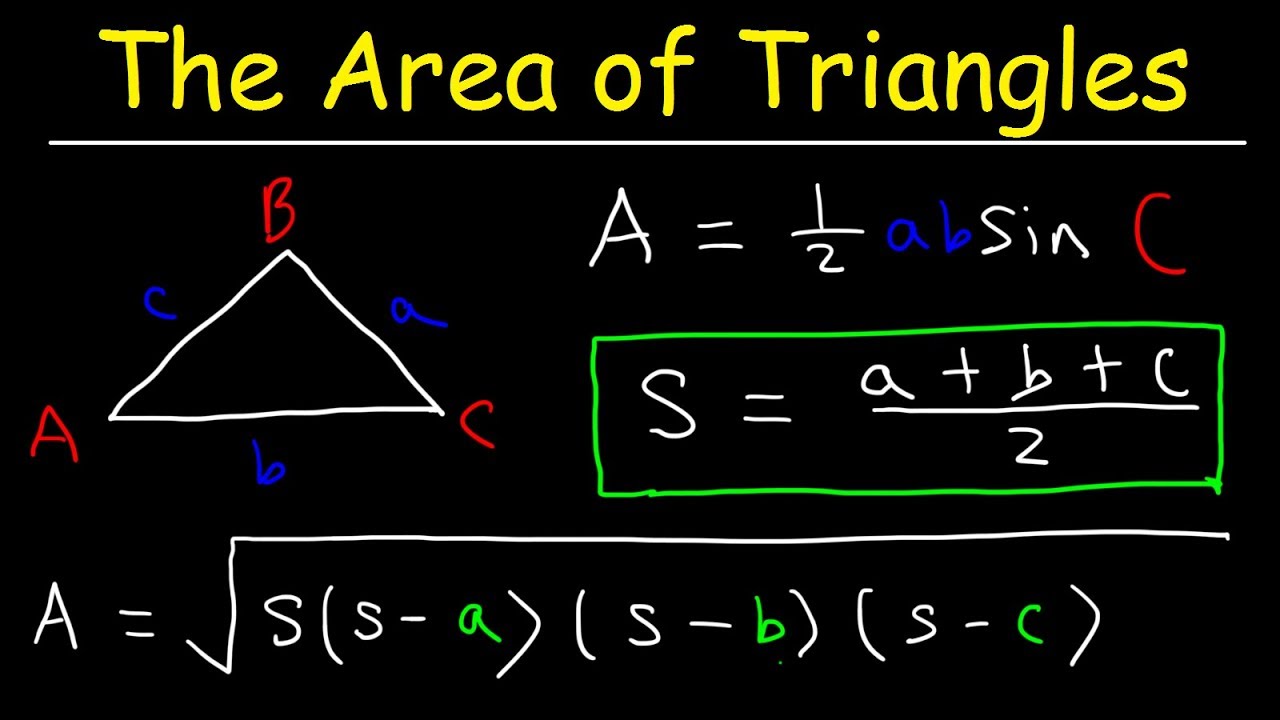
Area of an Oblique Triangle - SAS & SSS - Heron's Formula, Trigonometry

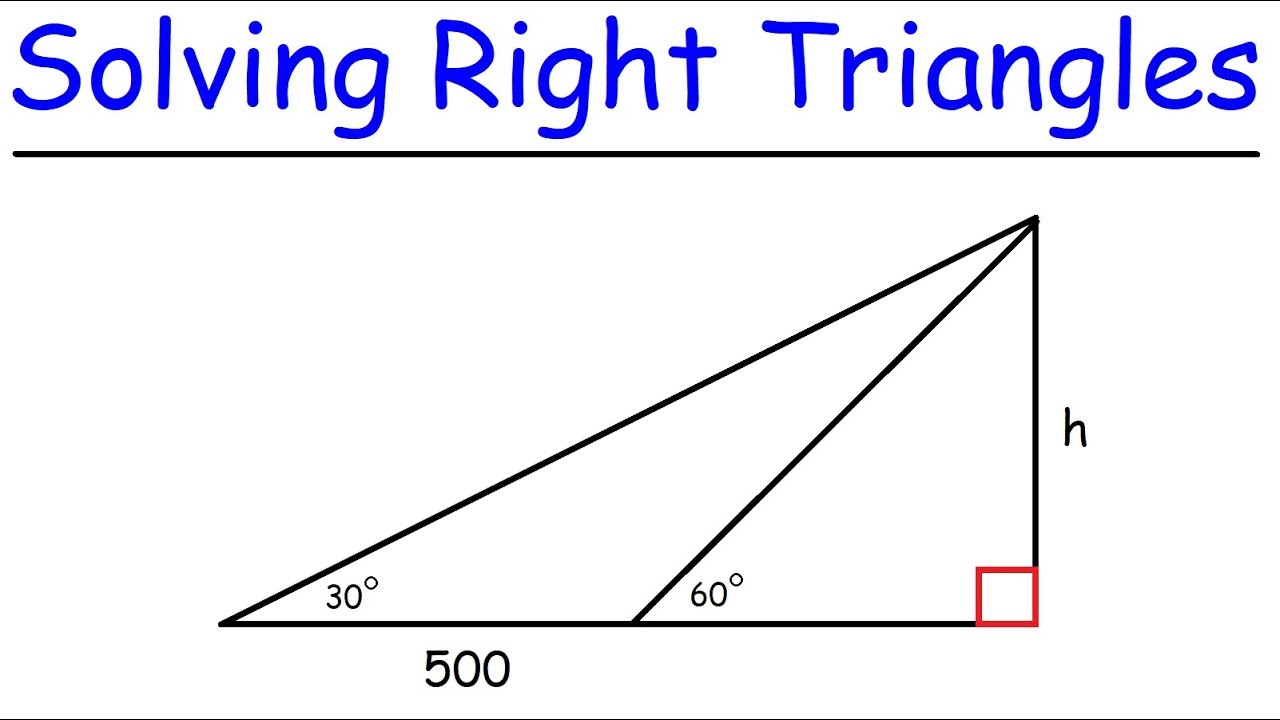
Trigonometry - How To Solve Right Triangles

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
GeometryRight TriangleArea CalculationNo CalculatorTriangle Sum Theorem30-60-90 TriangleIsoceles TrianglePythagorean TheoremMathematics EducationProblem Solving