AP Physics B Kinematics Presentation #47
TLDRThis script demonstrates the process of calculating the magnitude of the resultant vector when adding two vectors, A and B. Vector A is 24 units east, and vector B is 15 units south. By using the tail-to-tip method and applying the Pythagorean theorem, the script illustrates how to find the magnitude of vector C, which is the resultant. The calculation involves squaring the components, summing them, and taking the square root, yielding a magnitude of approximately 28.3 units for the resultant vector.
Takeaways
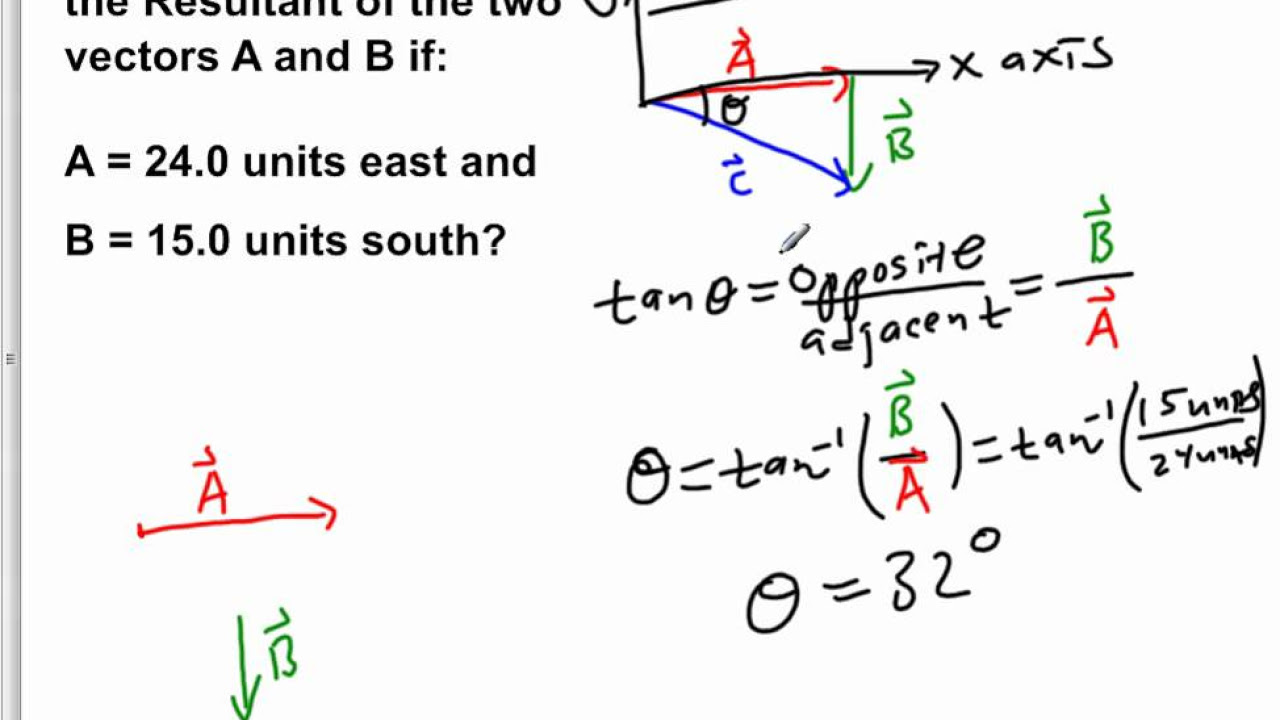
- 📏 The problem involves finding the magnitude of the resultant vector from two given vectors, A and B.
- 🧭 Vector A is described as 24 units in the East direction, and Vector B as 15 units in the South direction.
- 📐 The process begins with a visual representation of the vectors using the tail-to-tip method.
- 🔍 The resultant vector C is found by adding Vector A and Vector B, which are at right angles to each other.
- 📉 The Pythagorean theorem is applied to find the magnitude of the resultant vector C, which is perpendicular to the hypotenuse of the right-angled triangle formed by A and B.
- 🔢 The calculation involves squaring the magnitudes of A and B and then taking the square root of their sum to find C.
- 📘 The values for A and B are squared, resulting in 576 units squared for A and 225 units squared for B.
- 📙 The sum of the squared values gives 801 units squared, which is used to find the magnitude of C.
- 📚 The magnitude of the resultant vector C is calculated to be approximately 28.3 units using the square root of 801.
- 📈 The process demonstrates the application of vector addition and the Pythagorean theorem in physics and mathematics.
- 📝 The final result is a clear and concise summary of the magnitude of the resultant vector, which is the main objective of the script.
Q & A
What is the direction and magnitude of vector A?
-Vector A is directed to the east with a magnitude of 24 units.
What is the direction and magnitude of vector B?
-Vector B is directed to the south with a magnitude of 15 units.
What method is used to add the two vectors?
-The tail-to-tip method is used to add the two vectors.
What shape is formed when vector A and vector B are added together using the tail-to-tip method?
-A right triangle is formed when vector A and vector B are added together using the tail-to-tip method.
What theorem is used to calculate the magnitude of the resultant vector?
-The Pythagorean theorem is used to calculate the magnitude of the resultant vector.
How is the Pythagorean theorem applied to find the magnitude of vector C?
-The Pythagorean theorem is applied by using the formula C = √(A² + B²).
What are the squared values of vector A and vector B?
-The squared value of vector A is 576 units², and the squared value of vector B is 225 units².
What is the sum of the squared values of vectors A and B?
-The sum of the squared values of vectors A and B is 801 units².
What is the magnitude of the resultant vector C?
-The magnitude of the resultant vector C is approximately 28.3 units.
In what direction does the resultant vector C point?
-The resultant vector C points diagonally southeast, as it is the hypotenuse of the right triangle formed by vectors A and B.
Outlines
📏 Vector Addition and Resultant Magnitude Calculation
This paragraph details the process of finding the magnitude of the resultant vector when two vectors, A and B, are combined. Vector A is described as 24 units east, and vector B as 15 units south. The method involves visualizing these vectors on a diagram, using the tail-to-tip method to add them, resulting in vector C. The Pythagorean theorem is then applied to calculate the magnitude of C, which is the square root of the sum of the squares of A and B's magnitudes. By substituting the given values, the calculation leads to the magnitude of the resultant vector C being approximately 28.3 units.
Mindmap
Keywords
💡Magnitude
💡Resultant
💡Vector A
💡Vector B
💡Tail-to-Tip Method
💡Pythagorean Theorem
💡Hypotenuse
💡Square Root
💡Units Squared
💡East
💡South
Highlights
The magnitude of the resultant of two vectors is calculated using the Pythagorean theorem.
Vector A is 24 units East.
Vector B is 15 units South.
The tail-to-tip method is used to add the two vectors.
The resultant vector C is found by adding A and B.
C squared is equal to A squared plus B squared.
C is the square root of (A squared + B squared).
The Pythagorean theorem is applied to find the magnitude of the resultant vector.
A squared is 576 units squared, B squared is 225 units squared.
The sum of A squared and B squared is 801 units squared.
The magnitude of the resultant vector C is the square root of 801.
The calculated magnitude of C is approximately 28.3 units.
The resultant vector is shown in blue.
The process involves drawing the vectors and applying the Pythagorean theorem.
The method demonstrates a practical application of vector addition.
The explanation provides a clear step-by-step guide for finding the magnitude of a resultant vector.
The use of the tail-to-tip method simplifies the vector addition process.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: