AP Physics B Kinematics Presentation #40

The New Jersey Center for Teaching and Learning
26 Jun 201203:11
EducationalLearning
32 Likes 10 Comments

TLDRThis educational script explains the difference between scalar and vector quantities. It clarifies that scalars, such as speed and distance, do not depend on direction, while vectors, like acceleration, are direction-dependent. The script uses examples to illustrate that speed is a scalar because it's calculated by distance over time, both of which are scalars. It also points out that area is a scalar, unaffected by the direction of movement. The key takeaway is that acceleration is a vector because it involves a change in velocity, which is inherently directional.

Takeaways
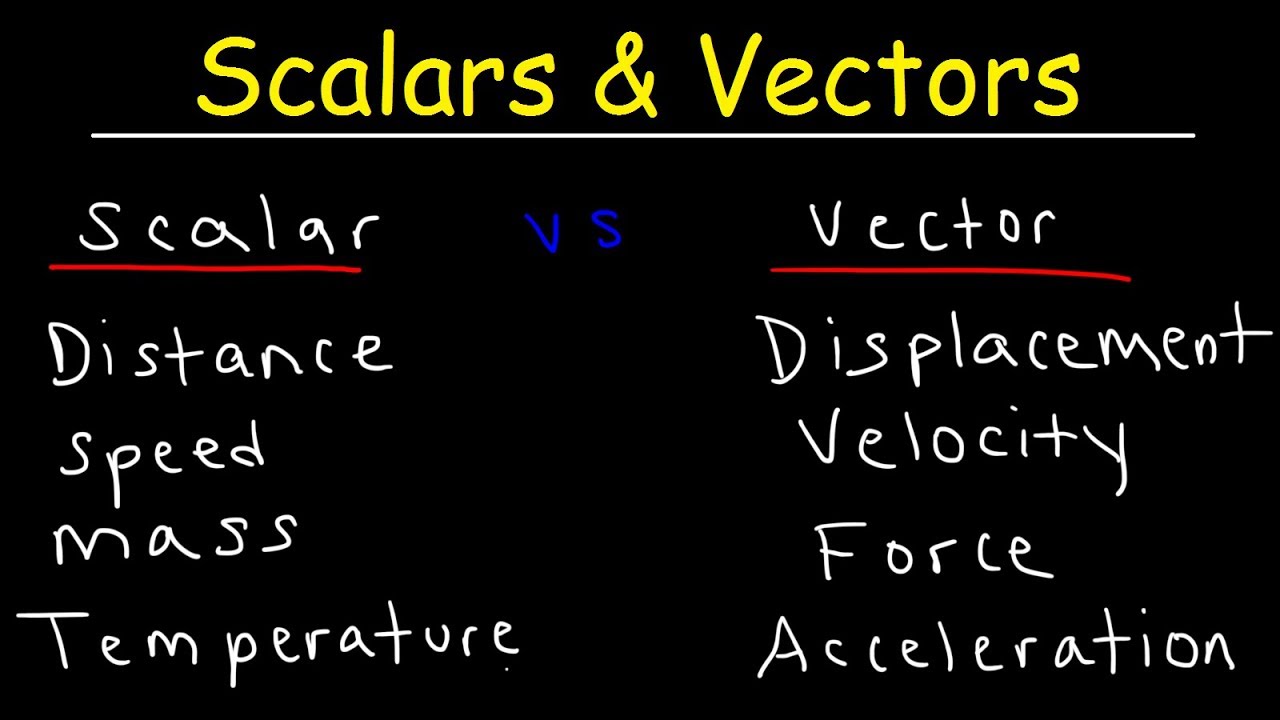
  • 📚 A vector is a quantity that is dependent on direction, unlike a scalar which is not.
  • 🔢 The equation for speed is distance over time, which makes speed a scalar as it does not depend on direction.
  • 🔄 Even if you travel in a circle or any odd path, the distance covered remains a scalar because it's the same regardless of direction.
  • ⏳ Time is a scalar as it is not influenced by the direction of movement.
  • 📏 The concept of area is a scalar because it remains constant regardless of the direction or orientation of an object.
  • 🚀 Acceleration is defined as the change in velocity over time, and since velocity is a vector, acceleration is also a vector.
  • 🧭 Velocity is a vector because it includes both magnitude and direction, making it dependent on the direction of movement.
  • 🔄 Directional change in movement results in a change in velocity, which in turn affects acceleration, confirming acceleration as a vector.
  • 📐 The script emphasizes the importance of understanding the difference between scalar and vector quantities in physics.
  • 📚 The explanation uses the example of traveling in a circle to illustrate the concept of scalar quantities like distance and time.
  • 📝 The final answer to the problem presented in the script is that acceleration is the vector quantity, as it is dependent on the direction of movement.
Q & A

  • What is the main difference between a scalar and a vector?

    -A scalar is a quantity that has only magnitude, while a vector has both magnitude and direction.

  • What is speed and why is it considered a scalar?

    -Speed is the distance traveled per unit of time, and it is a scalar because it does not depend on the direction of motion.

  • How does the script describe the concept of distance in relation to scalars?

    -The script explains that distance is a scalar because it is the total path length traveled, irrespective of the direction taken.

  • Why is time considered a scalar in the context of this script?

    -Time is considered a scalar because it is a measure of the duration of an event and does not vary with direction.

  • What is the equation for speed and how does it relate to scalars?

    -The equation for speed is distance over time. Both distance and time are scalars, making speed a scalar as well.

  • Can you provide an example from the script that illustrates the concept of a scalar?

    -An example from the script is the concept of area, which remains constant regardless of the direction or orientation of the object.

  • What is velocity and how does it differ from speed?

    -Velocity is the rate of change of an object's position, which includes both speed and direction. Unlike speed, velocity is a vector.

  • How is acceleration related to velocity?

    -Acceleration is the rate of change of velocity over time. Since velocity is a vector, acceleration is also a vector, as it includes changes in speed and/or direction.

  • What is the correct answer to the problem presented in the script?

    -The correct answer is acceleration (e), as it is the only option that is dependent on direction, making it a vector.

  • Why is the script's explanation of acceleration important for understanding vectors?

    -The script's explanation of acceleration is important because it clarifies how changes in direction affect the vector nature of acceleration, reinforcing the concept that vectors have directionality.

  • Can you summarize the script's main teaching point about vectors and scalars?

    -The script's main teaching point is to distinguish between scalars, which have only magnitude, and vectors, which have both magnitude and direction, using examples like speed, distance, area, and acceleration.

Outlines
00:00
🚀 Understanding Vectors and Scalars

This paragraph explains the fundamental difference between vectors and scalars. It clarifies that a scalar quantity, such as speed or area, does not depend on direction, whereas a vector quantity, such as acceleration, does. The explanation uses the example of traveling a certain distance in any direction to illustrate that scalars like distance and time remain the same regardless of the path taken. The paragraph concludes by identifying acceleration as a vector because it involves a change in velocity, which is inherently directional.

Mindmap
Keywords
💡Vector
A vector is a mathematical object that has both magnitude and direction. In physics, vectors are used to represent quantities such as force, velocity, and acceleration, which have a specific direction in space. In the script, the concept of vectors is central to distinguishing between quantities that depend on direction and those that do not. For example, acceleration is identified as a vector because it depends on the direction of velocity change.
💡Scalar
A scalar is a quantity that can be described with a single number, representing only magnitude without any direction. In the video script, scalars are contrasted with vectors to illustrate the importance of direction in certain physical quantities. Speed is given as an example of a scalar because it is calculated as distance over time, regardless of the direction of travel.
💡Direction
Direction refers to the course along which something moves or is oriented in space. It is a fundamental aspect of vectors, as the script explains that vectors are 'dependent upon direction.' The concept is used to differentiate vectors from scalars, where direction does not affect the value of the quantity, such as in the case of speed.
💡Speed
Speed is the scalar quantity that measures how fast an object is moving, calculated as the distance traveled divided by the time taken. The script uses speed to illustrate the concept of scalars, emphasizing that speed does not depend on the direction of motion, making it a scalar rather than a vector.
💡Distance
Distance is a scalar measure of the interval between two points, typically the length of the shortest route between them. In the script, distance is mentioned as a scalar because it is a measure that does not consider direction, only the magnitude of how far something has traveled.
💡Time
Time is a scalar quantity that refers to the duration or interval between events. It is a fundamental scalar in the script, used in the calculation of speed and emphasized as not being dependent on direction, which is a key characteristic of scalars.
💡Area
Area is a scalar quantity that represents the size of a two-dimensional surface or the extent of a planar lamina. The script mentions area to demonstrate that regardless of the direction in which a box is moved, the area it occupies remains unchanged, thus confirming it as a scalar.
💡Velocity
Velocity is a vector quantity that describes the rate of change of an object's position with respect to time, including both speed and direction. The script uses velocity to illustrate the concept of vectors, as it is dependent on the direction of motion, making it distinct from scalar quantities like speed.
💡Acceleration
Acceleration is the rate of change of velocity with respect to time and is a vector quantity because it includes both a magnitude and a direction. In the script, acceleration is identified as a vector because it depends on the direction of velocity, which changes as the object's motion direction changes.
💡Change
Change refers to the act or instance of making or becoming different. In the context of the script, change is particularly relevant when discussing acceleration, which is defined as the change in velocity over time. The concept of change is crucial for understanding how vectors, unlike scalars, are influenced by the direction of motion.
💡Magnitude
Magnitude is the measure of the size or quantity of something and is a key component of scalars. While vectors have both magnitude and direction, scalars are defined solely by their magnitude. The script contrasts magnitude with direction to explain the difference between scalars and vectors.
Highlights

A scalar is a quantity that does not depend on direction, while a vector is dependent upon direction.

Speed is calculated as distance over time and is a scalar because it does not depend on direction.

Distance traveled is a scalar as it is the total path covered regardless of direction.

Time is a scalar quantity as it is independent of direction.

The concept of speed being a scalar is reiterated, emphasizing its direction independence.

Area is a scalar because it remains constant regardless of the direction of movement.

The area of a box does not change with direction, confirming it as a scalar quantity.

Velocity is a vector because it is dependent on both magnitude and direction.

Acceleration is defined as the change in velocity over time.

Since velocity is a vector, acceleration is also a vector due to its directional dependency.

A change in the direction of movement results in a change in velocity and consequently in acceleration.

The final answer to the problem is acceleration, which is identified as a vector due to its directional nature.

The importance of understanding the difference between scalars and vectors in physics is highlighted.

The concept of directionality is crucial in distinguishing between scalar and vector quantities.

The transcript provides a clear explanation of the properties of scalar and vector quantities.

The problem presented helps to illustrate the directional dependency of vectors in contrast to scalars.

The solution to the problem emphasizes the directional aspect of acceleration as a vector.

Transcripts

Browse More Related Video

AP Physics B Kinematics Presentation #39

Scalars and Vectors

Intro to vectors & scalars | One-dimensional motion | Physics | Khan Academy

GCSE Physics - Scalar and Vector Quantities #41

Displacement and Velocity - How is it different from Distance and Speed? | Physics

What is Velocity? Physics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsVectorsScalarsSpeedDistanceTimeAccelerationEducationDirectionVelocityConcept