AP Physics B Kinematics Presentation #39
TLDRThis script explains the distinction between scalar and vector quantities through examples. It clarifies that scalars, such as distance and mass, do not depend on direction, whereas vectors, like velocity, are direction-dependent. The script uses the example of a ball's velocity in different directions to illustrate the concept of vectors, emphasizing that velocity's magnitude and direction are crucial, making it a vector quantity.
Takeaways
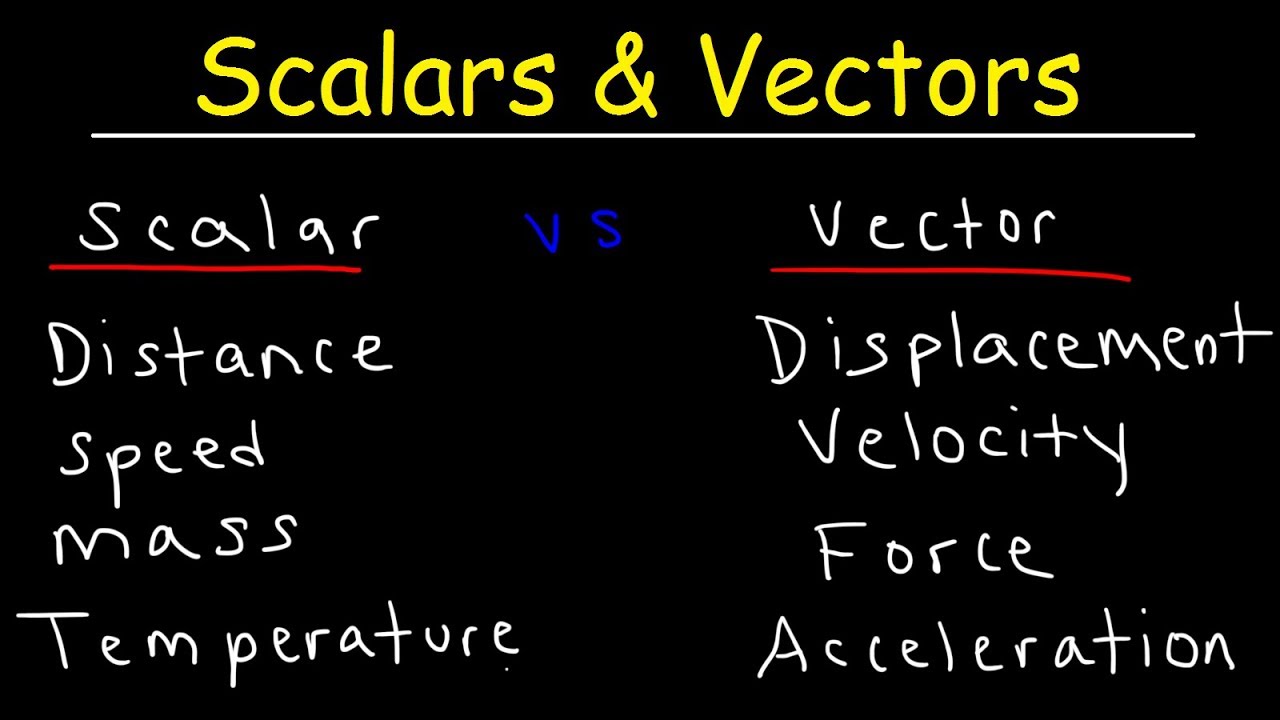
- 📚 The fundamental difference between a scalar and a vector is that a scalar has no direction, while a vector is direction-dependent.
- 🔍 Distance is an example of a scalar quantity because it remains constant regardless of the path taken or the direction of travel.
- 🚀 Velocity is identified as a vector because it has both magnitude and direction, and changing the direction results in a change of velocity sign.
- 🪨 Mass is a scalar quantity; it remains constant and is not affected by the position or orientation of an object in space.
- 📏 The area of a shape, such as a rectangle, is a scalar because it does not change with the shape's orientation or position.
- 🧭 Scalar quantities are independent of direction, which is a key characteristic used to distinguish them from vectors.
- 🛣️ The example of traveling in a circle and returning to the starting point illustrates that distance is a scalar because it is the same no matter the path.
- 🔄 The concept of velocity in different directions demonstrates the directional dependence of vectors, as the sign of velocity changes with direction.
- 📐 The properties of mass and area are used to emphasize that these quantities do not vary with spatial orientation, classifying them as scalars.
- 🔄 The script uses the analogy of flipping a box to explain that the area remains a scalar because it is not influenced by changes in the box's direction.
- 📝 The transcript provides clear examples to differentiate between scalars and vectors, highlighting the importance of direction in vector quantities.
Q & A
What is the primary difference between a scalar and a vector?
-A scalar is a quantity that does not depend on direction, while a vector is a quantity that has both magnitude and direction.
Why is distance considered a scalar quantity?
-Distance is a scalar because it represents the magnitude of how far an object has traveled, without regard to the direction of the path taken.
How does the script illustrate the concept of a scalar with the example of distance?
-The script explains that regardless of the direction taken when moving around a circle or in any other path, the distance covered remains the same, indicating no dependency on direction.
What is velocity and why is it classified as a vector?
-Velocity is the speed of an object in a particular direction. It is a vector because it has both magnitude (speed) and direction.
How does the script demonstrate the directional dependence of velocity?
-The script uses the example of a ball moving in two opposite directions, showing that the velocity can have positive or negative values depending on the direction, which indicates its vector nature.
What is mass and why is it not considered a vector?
-Mass is the amount of matter in an object and remains constant regardless of its position or orientation. It does not change with direction, making it a scalar quantity.
How does the script explain the concept of mass as a scalar?
-The script states that moving an object from one position to another does not change its mass, showing that mass is independent of direction.
What is the script's explanation for area being a scalar?
-The script explains that the area, calculated as length times width, remains constant regardless of the orientation of an object, indicating that area does not depend on direction.
How does the script contrast the properties of area with those of velocity?
-The script points out that while the area of an object remains the same regardless of its orientation, the velocity changes if the direction of motion is reversed, emphasizing the directional dependence of vectors.
What is the significance of the script's discussion on the difference between scalars and vectors?
-The script's discussion is significant as it helps to clarify the fundamental distinction between scalar and vector quantities, which is crucial for understanding various physical concepts and calculations.
Can you provide an example from the script that shows the magnitude of a vector?
-The script uses velocity as an example, where the magnitude of the velocity is represented by the speed of the ball, and the direction is indicated by the positive or negative sign.
Outlines
📏 Understanding Scalars and Vectors
This paragraph explains the fundamental difference between scalar and vector quantities. It emphasizes that scalars, such as distance, do not depend on the direction of the path taken, whereas vectors, like velocity, are inherently direction-dependent. The paragraph uses the example of a ball moving in different directions to illustrate how velocity, as a vector, changes with direction, while distance remains constant regardless of the path. It concludes by identifying velocity as a vector due to its directional dependency.
Mindmap
Keywords
💡Scalar
💡Vector
💡Direction
💡Distance
💡Velocity
💡Mass
💡Area
💡Circumference
💡Magnitude
💡Positive and Negative
💡Independence
Highlights
The difference between a scalar and a vector is explained, with a scalar not being dependent on direction.
A vector is characterized by its dependence on direction.
Distance is demonstrated as a scalar quantity, as it does not change regardless of the path taken.
Velocity is identified as a vector due to its directional dependency, with examples of positive and negative velocities.
Mass is confirmed as a scalar quantity, as it remains constant regardless of an object's position.
Area is shown to be a scalar, as it is not affected by the orientation of an object.
The concept of velocity's directional dependency is further explained with the example of flipping direction resulting in a change of velocity sign.
The importance of understanding the distinction between scalar and vector quantities in physics is emphasized.
A practical example of how distance measurement remains the same regardless of the path illustrates the scalar property.
The transcript provides a clear definition of a scalar, emphasizing its lack of directionality.
The transcript offers a clear definition of a vector, highlighting its directional nature.
The transcript uses the example of moving in a circle to explain the scalar property of distance.
The transcript explains how velocity can have both magnitude and direction, fulfilling the criteria of a vector.
The transcript clarifies that mass does not change with position, reinforcing its classification as a scalar.
The transcript uses the example of a box to demonstrate that area remains constant regardless of orientation, indicating it is a scalar.
The transcript concludes by reiterating that velocity is a vector due to its directional properties.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: