GCSE Physics - Scalar and Vector Quantities #41
TLDRThis educational video simplifies the distinction between scalar and vector quantities, essential concepts in physics. Scalars are defined as physical quantities possessing magnitude (size) but lacking direction, illustrated with examples like speed, distance, mass, temperature, and time. Conversely, vectors are quantities that have both magnitude and direction, such as velocity, displacement, acceleration, force, and momentum. The video further elucidates the difference through practical examples, emphasizing that vectors are represented by arrows indicating both magnitude and direction. This foundational knowledge sets the stage for deeper exploration in subsequent videos, aiming to enhance viewers' understanding of these fundamental physics concepts.
Takeaways
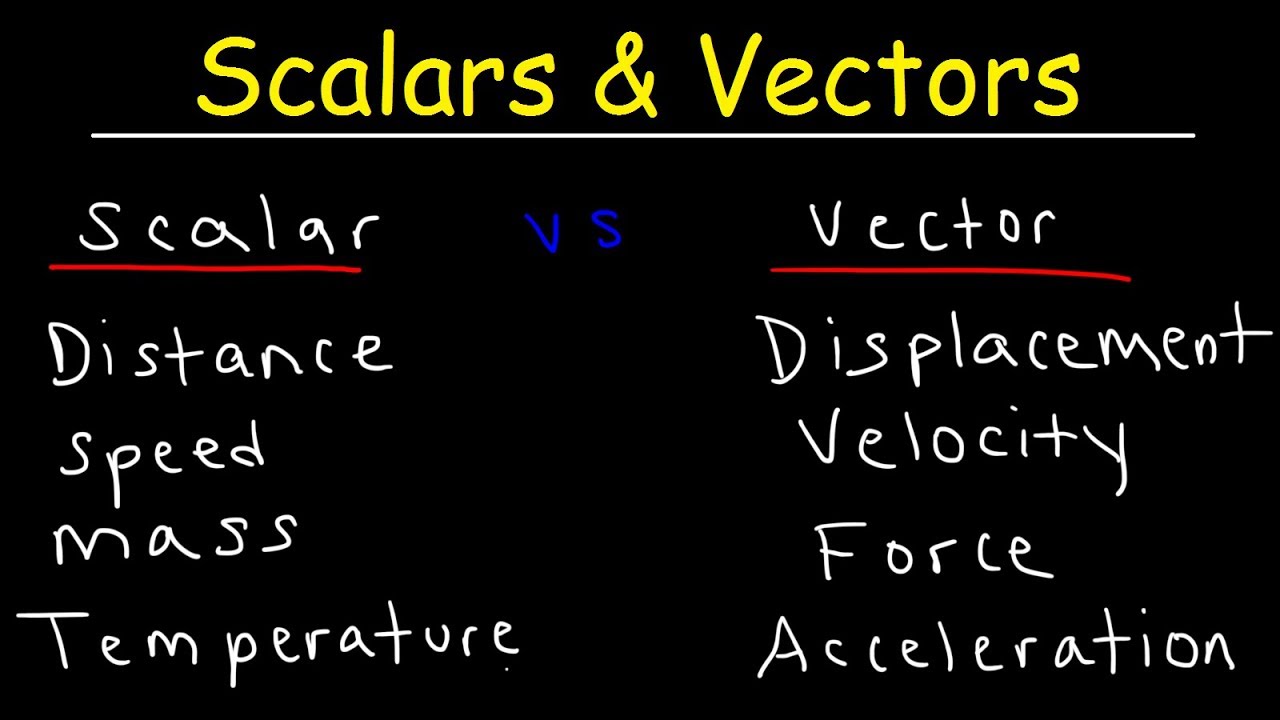
- 📏 Scalars are physical quantities with only magnitude, no direction, such as distance, mass, temperature, and time.
- 🚗 Speed is a scalar quantity because it doesn't include directional information.
- 🔢 The magnitude of a quantity is essentially its size, which can be expressed numerically.
- 📍 Vectors have both magnitude and direction, like velocity, displacement, acceleration, force, and momentum.
- 🔄 The difference between scalars and vectors can be illustrated by walking a certain distance in different directions.
- 🏃♂️ Walking 3 km without a specified direction ends you somewhere on a circle's circumference, demonstrating a scalar quantity.
- 🧭 Specifying a direction (e.g., 3 km east) gives an exact location, making displacement a vector quantity.
- 🖌️ Vectors are represented by arrows, where the arrow's length indicates magnitude and its direction shows the vector's orientation.
- 🌏 Four kilometers north and two kilometers west are represented by arrows pointing in their respective directions with different lengths.
- 🔽 Negative vectors can be represented by reversing the direction, such as labeling a 2 km west vector as minus two kilometers east.
- 📚 Understanding the distinction between scalars and vectors is fundamental for grasping various physical concepts.
Q & A
What defines a scalar quantity?
-A scalar quantity is defined by having only magnitude (size) but no direction. It can be measured with a numerical value.
Can you give an example of a scalar quantity and explain it?
-Speed is an example of a scalar quantity. For instance, a car traveling at 22 meters per second has a speed where 22 represents the magnitude of the speed, but since speed does not indicate direction, it is considered a scalar.
What are some other examples of scalar quantities?
-Other examples of scalar quantities include distance, mass, temperature, and time, among others.
How are vector quantities different from scalar quantities?
-Vector quantities differ from scalar quantities in that vectors have both magnitude and direction, such as velocity, displacement, acceleration, force, and momentum.
How can you visually represent a vector quantity?
-Vectors are represented using arrows, where the length of the arrow indicates the magnitude of the vector and the direction in which the arrow points indicates the direction of the vector.
Provide an example to illustrate how distance and displacement differ as scalar and vector quantities.
-If you start at point A and walk a distance of three kilometers, you could end up anywhere on the circumference of a circle centered at A, showing distance as a scalar. However, if you walked three kilometers east from A, displacement, being a vector, would specify both the magnitude (three kilometers) and the direction (east), pinpointing your exact ending location.
How can the direction of a vector be negative?
-The direction of a vector can be considered negative if it points in the opposite direction to a predefined positive direction. For example, two kilometers west can be labeled as minus two kilometers east, indicating a reversal in the eastward direction.
What is meant by the magnitude of a vector, and how is it shown?
-The magnitude of a vector refers to its size or amount, which is visually represented by the length of the arrow in a vector diagram. The longer the arrow, the greater the magnitude.
Why is it important to understand the difference between scalars and vectors?
-Understanding the difference between scalars and vectors is crucial for accurately describing physical quantities in physics, as it helps distinguish between quantities that require direction for a complete description and those that do not.
What implications does the concept of vectors have on the study of physics?
-The concept of vectors is fundamental in physics for analyzing forces, motion, and other phenomena that involve direction and magnitude, facilitating a deeper understanding of how objects interact and move in space.
Outlines
📏 Introduction to Scalars and Vectors
This paragraph introduces the fundamental difference between scalar and vector quantities. Scalars, such as distance, mass, temperature, and time, are physical quantities with only magnitude and no direction. The example of a car's speed clarifies that scalars can be represented by numerical values without directional components. Vectors, in contrast, possess both magnitude and direction, with examples including velocity, displacement, acceleration, force, and momentum. The distinction is further illustrated by contrasting the concept of walking a distance with walking a specific displacement, highlighting the directional aspect of vectors. The use of arrows to represent vectors is explained, with the length indicating magnitude and the arrow's orientation indicating direction. Negative vectors are briefly touched upon, using the example of a two-kilometer westward movement being equivalent to minus two kilometers eastward. The paragraph concludes with a summary and a call to action for viewers to like and subscribe for more content.
Mindmap
Keywords
💡Scalar
💡Vector
💡Magnitude
💡Direction
💡Velocity
💡Displacement
💡Acceleration
💡Force
💡Momentum
💡Arrows
💡Negative Vectors
Highlights
Introduction to the difference between scalar and vector quantities.
Scalars have magnitude but no direction, with examples like speed, distance, mass, temperature, and time.
Vectors have both magnitude and direction, with examples like velocity, displacement, acceleration, force, and momentum.
Explanation of magnitude as the size or numerical value of a quantity.
Speed as an example of a scalar quantity because it lacks direction.
The distinction between distance (a scalar) and displacement (a vector).
Use of arrows to represent vectors, with length for magnitude and direction indicated by the arrow's pointing.
An example of walking 3 kilometers without a specified direction illustrates the concept of scalar quantities.
Walking 3 kilometers east as an example of a vector quantity, showcasing both magnitude and direction.
Visual representation of vectors using arrows for direction and magnitude.
Comparison of a 4 kilometers north vector to a 2 kilometers west vector to explain magnitude and direction.
Introduction of negative vectors, with a 2 kilometers west vector being equivalent to -2 kilometers east.
Closing summary and encouragement to like and subscribe for more educational content.
Promise of deeper exploration into vector and scalar quantities in future videos.
Invitation for viewer engagement through likes and subscriptions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: