Calculus AB/BC – 1.15 Limits at Infinity and Horizontal Asymptotes
TLDRIn this educational video, Mr. Bean explains the concept of horizontal asymptotes in calculus, focusing on their relation to limits. He clarifies the difference between horizontal and vertical asymptotes and provides basic rules for determining horizontal asymptotes by comparing the growth rates of numerators and denominators. The lesson includes examples and emphasizes the importance of understanding the end behavior of functions as x approaches infinity or negative infinity, illustrating the process with various functions and their asymptotic behavior.
Takeaways
- 📚 Horizontal asymptotes refer to the end behavior of a graph, showing how it approaches a certain y-value as x approaches infinity or negative infinity.
- 🚫 Unlike vertical asymptotes, graphs can cross horizontal asymptotes, which represent a y-value that the graph approaches but may not necessarily limit to.
- 🔢 The basic rules for determining horizontal asymptotes involve comparing the growth rates of the numerator and denominator of a rational function: if the denominator grows faster, the asymptote is y=0; if they grow at the same rate, the asymptote is the ratio of leading coefficients; if the numerator grows faster, there is no horizontal asymptote.
- 📈 Exponential functions grow the fastest, followed by polynomial functions, and logarithmic functions grow the slowest, which is crucial for understanding the behavior of functions near horizontal asymptotes.
- 🔍 When finding horizontal asymptotes, focus on the terms with the highest degrees in the numerator and denominator, as they dictate the end behavior of the function.
- ✂️ For rational functions, if the numerator grows faster than the denominator, the function approaches infinity and does not have a horizontal asymptote.
- 🔄 If the numerator and denominator grow at the same rate, the horizontal asymptote is determined by the ratio of the leading coefficients.
- 📉 When the denominator grows faster than the numerator, the horizontal asymptote is y=0.
- 🔃 For functions involving square roots or other radicals, simplify the expression before determining the horizontal asymptote, especially when considering x approaching both positive and negative infinity.
- 📌 The horizontal asymptote can differ when x approaches positive infinity versus negative infinity, as seen in examples where the sign of the leading term changes.
- 🚫 The limit (and thus the horizontal asymptote) does not exist for functions that oscillate between positive and negative values, such as sine or cosine functions, as they do not approach a specific y-value.
Q & A
What is a horizontal asymptote and how is it related to the end behavior of a graph?
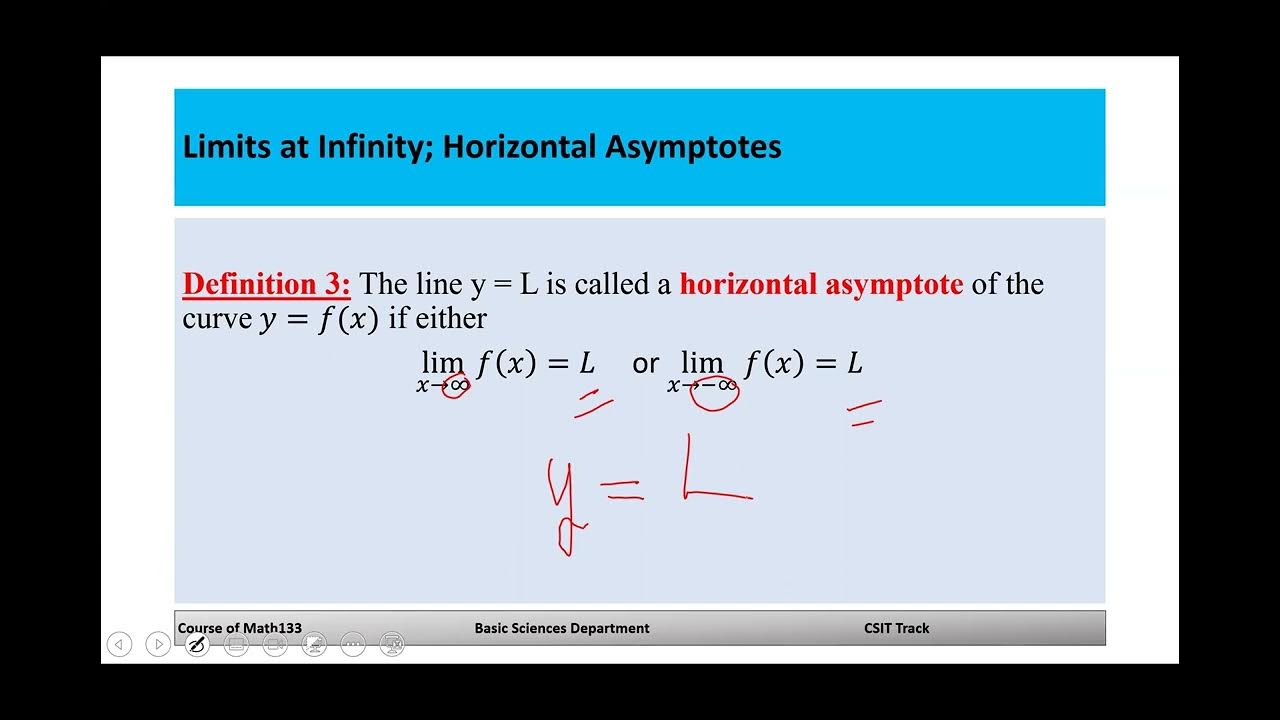
-A horizontal asymptote refers to the end behavior of a graph where the graph approaches a certain horizontal line as it extends towards infinity. Unlike vertical asymptotes, a graph can cross a horizontal asymptote. The y-value of the horizontal asymptote is the value that the graph gets closer to as x approaches either positive or negative infinity.
Can you explain the difference between horizontal and vertical asymptotes?
-Horizontal asymptotes are horizontal lines that a graph approaches as it extends towards positive or negative infinity, and it's possible for the graph to cross these lines. Vertical asymptotes, on the other hand, are vertical lines that the graph cannot cross. As the graph approaches a vertical asymptote, it is pushed upwards or downwards without crossing the line.
What happens when the denominator of a rational function grows faster than the numerator?
-When the denominator of a rational function grows faster than the numerator, the overall value of the function approaches zero. This is because the large values in the denominator dominate the division, resulting in a very small quotient.
How do you determine if a rational function has a horizontal asymptote of y = 1?
-A rational function has a horizontal asymptote of y = 1 if the numerator and denominator grow at the exact same rate. In this case, the coefficients of the highest degree terms in the numerator and denominator are equal, and the horizontal asymptote is the ratio of these coefficients.
What is the horizontal asymptote of a function where the numerator grows faster than the denominator?
-If the numerator of a function grows faster than the denominator, the function does not have a horizontal asymptote. Instead, the function approaches infinity as x goes to either positive or negative infinity.
How do you find the horizontal asymptote when the degree of the numerator is less than the degree of the denominator?
-When the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is y = 0. This is because the terms with the highest degree in the denominator will dominate the behavior of the function as x approaches infinity.
What is the significance of exponential functions in determining the horizontal asymptote of a rational function?
-Exponential functions grow the fastest compared to polynomial and logarithmic functions. When determining horizontal asymptotes, if the exponential function is in the numerator and grows faster than the denominator, the function approaches infinity and does not have a horizontal asymptote.
Why do logarithmic functions not have a horizontal asymptote?
-Logarithmic functions do not have a horizontal asymptote because they grow very slowly and flatten out as x approaches infinity. They do not approach a specific y-value as the graph extends towards infinity.
How does the horizontal asymptote differ when x approaches positive infinity versus negative infinity for certain functions?
-For some functions, the horizontal asymptote can differ when x approaches positive versus negative infinity. This happens when the function contains an odd root or involves an odd power of x, which can result in different signs for positive and negative values of x.
What is the end behavior of a function with a horizontal asymptote of y = 2/3 when x approaches negative infinity?
-If a function has a horizontal asymptote of y = 2/3 as x approaches positive infinity, and if the function involves an odd root or an odd power of x, the horizontal asymptote as x approaches negative infinity will be y = -2/3 due to the sign change of the terms involved.
Why might the limit of a trigonometric function as x approaches infinity not exist?
-The limit of a trigonometric function like sine or cosine as x approaches infinity does not exist because these functions are periodic and oscillate between -1 and 1. They do not approach a specific y-value; instead, they continue to oscillate without converging to a single point.
Outlines
📚 Introduction to Horizontal Asymptotes and Limits
Mr. Bean introduces the concept of horizontal asymptotes as the end behavior of a graph, explaining that they represent the y-values a graph approaches as x tends towards infinity or negative infinity. He clarifies that graphs may cross horizontal asymptotes unlike vertical asymptotes. The importance of understanding the growth rate of numerators and denominators in functions is emphasized, with rules for determining horizontal asymptotes based on their relative growth: if the denominator grows faster, the limit approaches zero; if they grow at the same rate, the horizontal asymptote is the ratio of the coefficients; and if the numerator grows faster, the function approaches infinity with no horizontal asymptote.
🔍 Analyzing Growth Rates to Determine Horizontal Asymptotes
The lesson continues with an in-depth analysis of how to determine horizontal asymptotes by comparing the growth rates of the numerator and the denominator in a function. Mr. Bean provides a chart with various functions and demonstrates how to identify the terms with the highest growth rate. He explains that exponential functions grow the fastest, followed by polynomials, and then logarithms. The process involves simplifying the function to its most significant terms and applying the growth rate rules to find the horizontal asymptote or to determine that the function approaches infinity or negative infinity without a specific asymptote.
📉 Special Cases of Horizontal Asymptotes at Positive and Negative Infinity
This section discusses special cases where the horizontal asymptote differs when x approaches positive or negative infinity. Mr. Bean illustrates this with examples, showing how to handle square roots and other algebraic manipulations in the context of limits. He emphasizes the importance of considering the original form of the function when dealing with negative infinity to avoid incorrect simplifications. The examples provided demonstrate how to find the horizontal asymptote for functions with different behaviors at positive and negative infinity, including cases where the limit does not exist due to oscillating functions like sine and cosine as x approaches infinity.
📝 Conclusion on Horizontal Asymptotes and Limit Behavior
The final paragraph wraps up the lesson on horizontal asymptotes, reiterating the importance of understanding the end behavior of functions and their limits as x approaches infinity or negative infinity. Mr. Bean highlights common mistakes students make, such as confusing limits as x approaches zero with those approaching infinity. He provides examples of limits involving exponential functions and trigonometric functions, emphasizing the difference in behavior and the resulting horizontal asymptotes or lack thereof. The lesson concludes with a reminder to practice the mastery check problems to solidify understanding of horizontal asymptotes.
Mindmap
Keywords
💡Horizontal Asymptote
💡Limit
💡Numerator
💡Denominator
💡Exponential Functions
💡Polynomial Functions
💡Logarithms
💡End Behavior
💡Trigonometric Functions
💡Oscillation
Highlights
Introduction to horizontal asymptotes and their relation to limits.
Explanation of how horizontal asymptotes describe the end behavior of a graph.
Difference between horizontal and vertical asymptotes.
Horizontal asymptotes can be crossed by the graph, unlike vertical asymptotes.
Discussion on limits as x approaches infinity and negative infinity.
Basic rules for determining horizontal asymptotes based on the growth rates of the numerator and denominator.
If the denominator grows faster, the limit approaches zero.
If the numerator and denominator grow at the same rate, the limit is the ratio of their leading coefficients.
If the numerator grows faster, the limit approaches infinity.
Ranking of different functions based on their growth rates: exponential functions grow the fastest.
Application of growth rate rules to determine horizontal asymptotes for specific functions.
Example calculations showing how to determine the horizontal asymptote by comparing the degrees of the numerator and denominator.
Explanation of the horizontal asymptote when the numerator and denominator grow at the same rate.
Case where the denominator grows faster, resulting in a horizontal asymptote of y = 0.
Special cases where the limit depends on whether x approaches positive or negative infinity.
Clarification on the proper approach to plugging values into the original function versus the simplified version.
Examples illustrating the different horizontal asymptotes when approaching positive versus negative infinity.
The concept of end behavior and its equivalence to horizontal asymptotes and limits.
Discussion on limits involving exponential functions and their end behavior.
Explanation of why some limits do not exist due to the oscillating nature of sine and cosine functions.
Importance of understanding the difference between limits as x approaches zero and as x approaches infinity.
Examples of limits involving trigonometric functions and their behavior as x approaches infinity.
Final summary emphasizing the importance of mastering these concepts for test preparation.
Transcripts
Browse More Related Video

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes
Math 133 Lecture 2 6

AP Calculus AB - 1.14 Connecting Infinite Limits and Vertical Asymptotes

Horizontal Asymptotes!

Finding an Oblique Asymptote of a Rational Function (Precalculus - College Algebra 41)

Calculus 1 Lecture 3.5: Limits of Functions at Infinity
5.0 / 5 (0 votes)
Thanks for rating: