Horizontal Asymptotes!
TLDRThe video script delves into the concept of horizontal asymptotes and their mathematical definition using calculus. It explains how to evaluate the limits of functions as they approach positive or negative infinity, highlighting the conditions under which horizontal asymptotes exist. The script also introduces algebraic shortcuts for rational functions, discussing the implications of the degrees of the numerator and denominator on the presence and nature of horizontal asymptotes. The content is engaging, informative, and provides a solid foundation for understanding the behavior of functions at infinity.
Takeaways
- 📈 The concept of horizontal asymptotes is important for understanding the end behavior of functions, which can be relevant in real-world contexts like carbon emissions and average costs.
- 🌟 The limit of a function as X approaches infinity or negative infinity helps define the horizontal asymptote, which is the value that the function's output approaches but never actually reaches.
- 🔍 Limits do not always exist; they are only defined if the function settles on a single, finite Y value as X tends towards positive or negative infinity.
- 📊 To evaluate limits, you can use intuitive graphical analysis or algebraic methods, including factoring and applying the limit rules for rational functions.
- ∞ When plugging in infinity into a limit, you may encounter an indeterminate form like infinity over infinity, which requires further algebraic manipulation.
- 🍕 A useful mnemonic for understanding limits involving infinity is to think of dividing a finite number by infinity, which results in zero.
- 📚 For rational functions (polynomials divided by polynomials), the horizontal asymptote can be determined by comparing the degrees of the numerator and denominator.
- 🔢 If the degree of the numerator is greater than the denominator, there is no horizontal asymptote.
- 🔭 If the degree of the denominator is greater, the horizontal asymptote is y equals zero.
- 📐 When the degrees are equal, the horizontal asymptote is determined by the ratio of the leading coefficients of the polynomials.
- ✅ Shortcuts for rational functions can simplify the process of finding horizontal asymptotes, but it's important to understand the underlying algebra to justify these shortcuts.
Q & A
What is a horizontal asymptote?
-A horizontal asymptote is a horizontal line that a function approaches as the input (x) either approaches infinity or negative infinity. It represents the end behavior of a function.
How can you determine if a function has a horizontal asymptote?
-You can determine if a function has a horizontal asymptote by evaluating the limits of the function as x approaches infinity and negative infinity. If these limits settle on a single finite y-value, a horizontal asymptote exists at that y-value.
What is the significance of horizontal asymptotes in real-world contexts?
-Horizontal asymptotes can be significant in real-world contexts as they help in understanding the long-term behavior of functions, such as carbon emissions, average costs, and production limits as they extend to infinity.
What happens when you divide a finite number by infinity?
-When you divide a finite number by infinity, the result is zero. This concept is used to evaluate limits as x approaches infinity in calculus.
What is an indeterminate form and why does it occur?
-An indeterminate form, such as infinity over infinity, occurs when direct substitution of infinity into an expression does not yield a determinate result. It indicates that more algebraic manipulation is required to evaluate the limit.
How do you evaluate the limit of a rational function as x approaches infinity?
-To evaluate the limit of a rational function as x approaches infinity, you can use shortcuts based on the degrees of the polynomials in the numerator and denominator. If the degree of the numerator is greater than the denominator, there is no horizontal asymptote. If the degree of the denominator is greater, the horizontal asymptote is y equals zero. If the degrees are equal, the horizontal asymptote is the ratio of the leading coefficients.
What is the horizontal asymptote of a function if the degree of the polynomial in the numerator is greater than the degree of the polynomial in the denominator?
-If the degree of the polynomial in the numerator is greater than the degree of the polynomial in the denominator, the function does not have a horizontal asymptote.
What is the horizontal asymptote of a function if the degree of the polynomial in the denominator is greater than the degree of the polynomial in the numerator?
-If the degree of the polynomial in the denominator is greater than the degree of the polynomial in the numerator, the horizontal asymptote is y equals zero.
What is the horizontal asymptote of a function if the degrees of the polynomials in the numerator and denominator are equal?
-If the degrees of the polynomials in the numerator and denominator are equal, the horizontal asymptote is the ratio of the leading coefficients of the polynomials.
Why is it important to factor out the highest power of x in a rational function when evaluating limits?
-Factoring out the highest power of x in a rational function allows you to simplify the expression and cancel out the common factors, making it easier to evaluate the limit as x approaches infinity or negative infinity.
What is the next topic that will be covered after discussing horizontal asymptotes?
-After discussing horizontal asymptotes, the next topic to be covered is derivatives, specifically focusing on exponential and logarithmic functions.
Outlines
🌟 Introduction to Horizontal Asymptotes
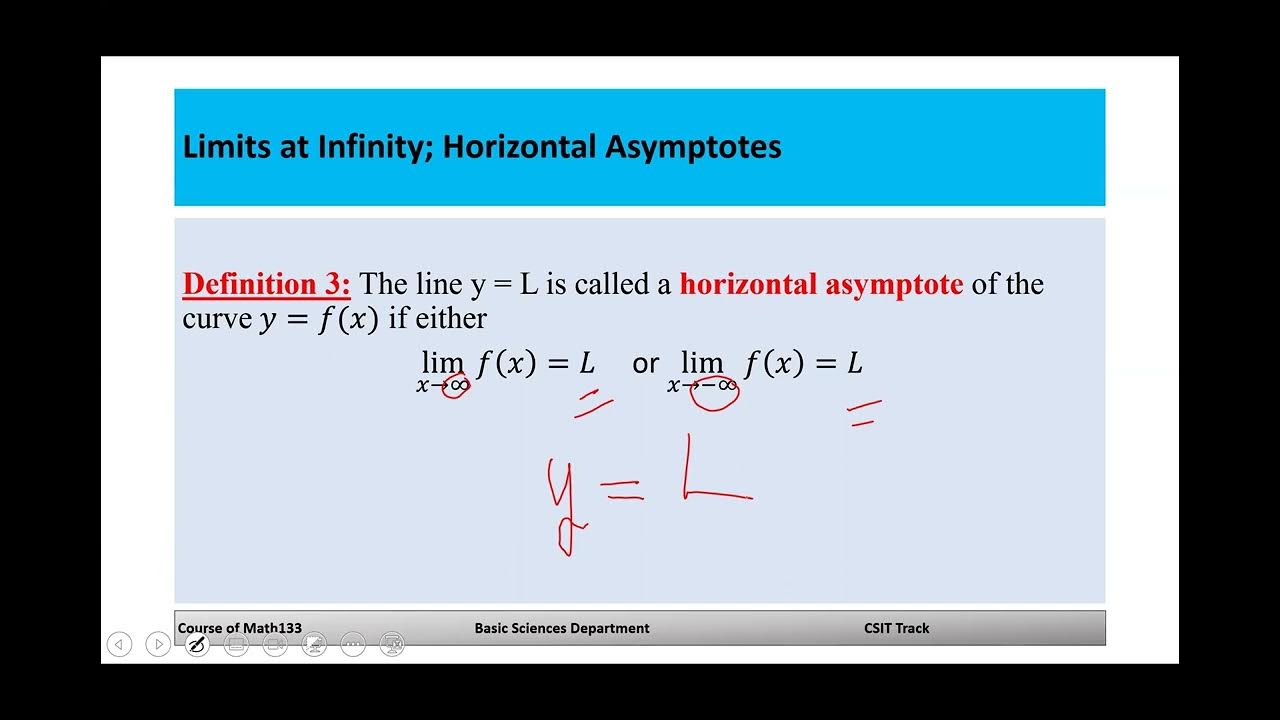
The first paragraph introduces the concept of horizontal asymptotes and their significance in understanding the behavior of functions as they approach infinity. The speaker discusses how these mathematical concepts can be applied to real-world scenarios, such as carbon emissions and average costs in production. The paragraph establishes the notation for limits as X approaches positive and negative infinity and explains that these limits represent the behavior of the Y-value as X becomes infinitely large. The speaker also clarifies that these limits may not always exist and provides an example to illustrate how to evaluate them, including the case where the limit does not exist due to the function not settling on a single Y-value.
🧮 Algebraic Approach to Limits at Infinity
The second paragraph delves into an algebraic method for evaluating limits as X approaches infinity. It emphasizes the concept that a finite number divided by infinity equals zero, using the analogy of dividing a single pizza among an infinite family. The speaker provides a step-by-step approach to handling limits, particularly when encountering the indeterminate form of infinity over infinity. Several examples are given to demonstrate how to apply algebraic techniques, such as factoring out the highest power of X, to find the limit of different functions as X approaches infinity. The paragraph also introduces shortcuts for rational functions and explains how to justify these shortcuts with algebra.
📚 Shortcuts for Rational Functions
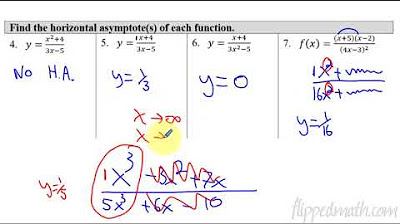
The third paragraph focuses on shortcuts for evaluating limits of rational functions based on the degrees of the polynomials in the numerator and denominator. The speaker outlines three scenarios: when the degree of the numerator is greater than the denominator, resulting in no horizontal asymptote; when the degree of the denominator is greater, resulting in a horizontal asymptote at y equals zero; and when the degrees are equal, leading to a horizontal asymptote given by the ratio of the leading coefficients. Each scenario is illustrated with examples, and the algebraic process to justify the shortcuts is explained.
🔍 Recap and Transition to Derivatives
The fourth and final paragraph recaps the key points discussed in the video about horizontal asymptotes and limits as X approaches plus or minus infinity. The speaker summarizes the algebraic techniques for evaluating these limits and the shortcuts for rational functions based on the degrees of the polynomials. The paragraph concludes with a teaser for the next chapter, which will cover derivatives, specifically exponentials and logarithms, indicating a progression in the mathematical concepts being explored.
Mindmap
Keywords
💡Horizontal Asymptotes
💡Limits
💡Calculus
💡Rational Functions
💡Indeterminate Forms
💡Algebraic Shortcuts
💡Leading Coefficients
💡Infinity
💡Polynomials
💡Degree of a Polynomial
Highlights
The concept of horizontal asymptotes is introduced as a key aspect of evaluating the end behavior of functions, particularly relevant in contexts like carbon emissions and average cost analysis.
The notation and definition of limits as X approaches infinity and negative infinity are discussed to understand the behavior of Y values.
It's highlighted that limits do not always exist and are only defined if they settle on a single, finite value.
An example function is used to illustrate how to evaluate the limit as X approaches infinity and negative infinity, resulting in the identification of horizontal asymptotes.
A sine-like function is presented to demonstrate a case where the limit as X approaches infinity does not exist, emphasizing the need for a consistent Y value for the limit to exist.
The relationship between limits as X approaches infinity and horizontal asymptotes is established, with conditions for the existence of zero, one, or two horizontal asymptotes.
An algebraic approach to evaluate limits as X approaches infinity is introduced, with the concept that a finite number divided by infinity tends towards zero.
Shortcuts for rational functions are explained based on the degrees of the polynomials in the numerator and denominator, providing a method to determine horizontal asymptotes without direct calculation.
The case where the degree of the numerator is greater than the denominator results in no horizontal asymptote, as demonstrated algebraically.
When the degree of the denominator is greater, a horizontal asymptote of y equals zero exists, as justified both algebraically and through shortcuts.
If the degrees of the polynomials are equal, the horizontal asymptote is determined by the ratio of the leading coefficients, a shortcut that simplifies the process.
The algebraic method involves factoring out the highest power of X and canceling out terms to simplify the limit as X approaches infinity.
The transcript provides a comprehensive understanding of how to handle indeterminate forms such as infinity over infinity through algebraic manipulation.
The importance of justifying shortcuts with algebra is emphasized to ensure a solid mathematical foundation for understanding limits and horizontal asymptotes.
A recap of the chapter is given, summarizing the definition of horizontal asymptotes, the conditions for their existence, and the algebraic techniques used to evaluate them.
The next chapter is teased, promising a continuation of the discussion on derivatives, this time focusing on exponential and logarithmic functions.
Transcripts
Browse More Related Video

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes

Finding a Horizontal Asymptote of a Rational Function (Precalculus - College Algebra 40)
Math 133 Lecture 2 6

Calculus AB Homework 1.4 Limits Involving Infinity

Finding an Oblique Asymptote of a Rational Function (Precalculus - College Algebra 41)
Calculus AB/BC – 1.15 Limits at Infinity and Horizontal Asymptotes
5.0 / 5 (0 votes)
Thanks for rating: