AP Calculus AB - 1.14 Connecting Infinite Limits and Vertical Asymptotes
TLDRIn this AP Calculus AB video, Mr. Bortnick explores the concept of infinite limits and vertical asymptotes, focusing on how functions approach infinity or negative infinity at specific values. He illustrates this with examples, including identifying vertical asymptotes by setting the denominator of a rational function to zero and checking for discontinuities. Mr. Bortnick demonstrates how to evaluate limits approaching these asymptotes by plugging in values close to the discontinuity points, emphasizing the importance of understanding the behavior of the function as it approaches vertical asymptotes.
Takeaways
- 📚 The lecture is about 'Infinite Limits and Vertical Asymptotes' in the context of AP Calculus AB, focusing on understanding limits that approach infinity or negative infinity.
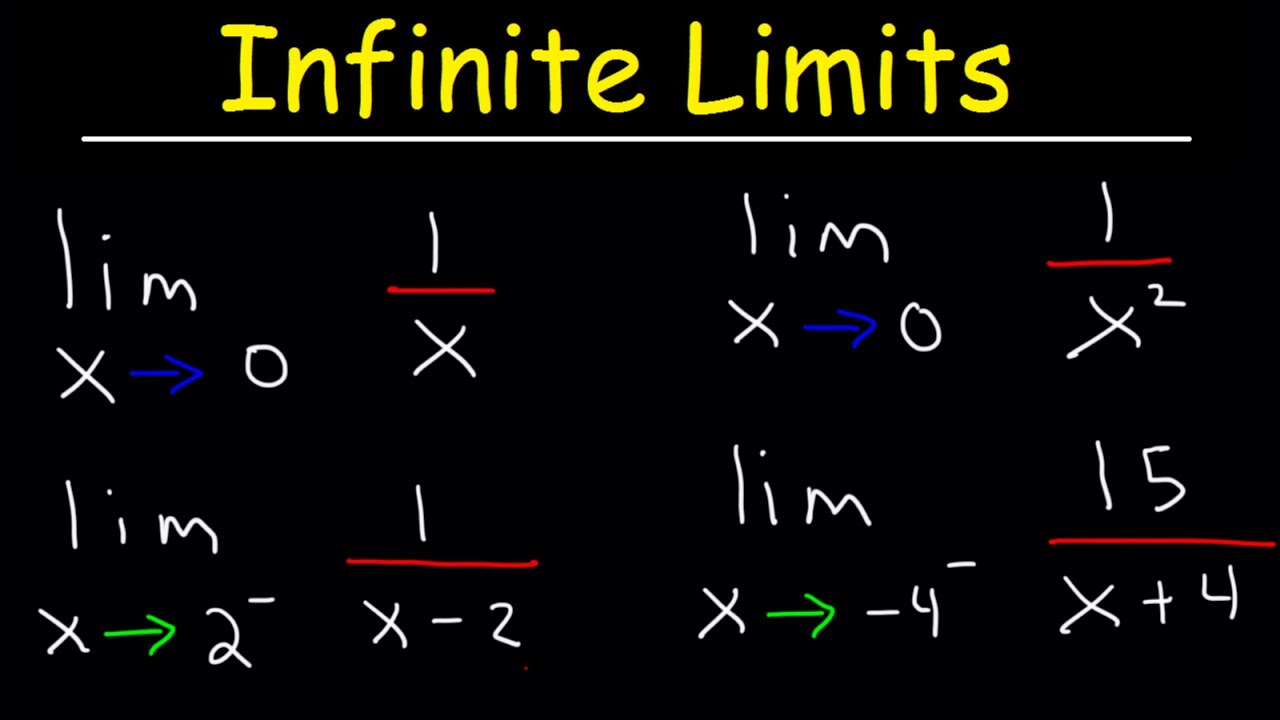
- 🌟 Infinite limits are special cases where the limit does not exist but indicates a function's behavior of going to positive or negative infinity.
- 📉 Vertical asymptotes occur where a function cannot cross a certain value, and the function approaches infinity or negative infinity as it gets closer to that value.
- 🔍 To identify vertical asymptotes, one must find where the denominator of a rational function equals zero, without any corresponding factor in the numerator to cancel it out.
- 📐 The function \( f(x) = \frac{x^2 + 2x - 8}{x^2 + x - 12} \) is used as an example to demonstrate how to identify vertical asymptotes by factoring and analyzing the behavior as \( x \) approaches the values that make the denominator zero.
- 🤔 To determine the direction of the limit (towards positive or negative infinity) at a vertical asymptote, one can plug in values close to the asymptote and observe the sign of the result.
- 📝 The process involves checking the sign of the function as \( x \) approaches the asymptote from both the left and the right to determine if the limit approaches positive or negative infinity.
- ❗ The overall limit at a vertical asymptote does not exist if the left and right limits do not agree, as they must be equal for the limit to exist.
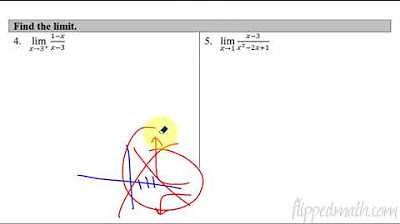
- 📈 For the function \( \frac{1 - x}{x - 3} \), the limit as \( x \) approaches 3 from the right is negative infinity, indicating the function's behavior as it approaches the vertical asymptote at \( x = 3 \).
- 📊 The function \( \frac{x - 3}{x^2 - 2x + 1} \) has a vertical asymptote at \( x = 1 \), and the limit from both the left and right sides as \( x \) approaches 1 is negative infinity.
- 📚 The importance of understanding the relationship between infinite limits and vertical asymptotes is emphasized for solving calculus problems, especially in no-calculator sections of exams.
Q & A
What is the main topic discussed in the script?
-The main topic discussed in the script is connecting infinite limits and vertical asymptotes in the context of calculus.
What are the two possible values that limits can approach when a function has a vertical asymptote?
-When a function has a vertical asymptote, the limits can approach either positive infinity or negative infinity.
What is a vertical asymptote and how is it related to limits?
-A vertical asymptote is a vertical line that the graph of a function approaches but never crosses. It is related to limits because the function's value can approach infinity or negative infinity as it gets closer to the asymptote.
How can you determine if a discontinuity is a vertical asymptote?
-You can determine if a discontinuity is a vertical asymptote by factoring the function and checking if there are factors in the denominator that do not cancel out with the numerator.
What is the significance of the function f(x) = (x^2 + 2x - 8) / (x^2 + x - 12) in the script?
-The function f(x) = (x^2 + 2x - 8) / (x^2 + x - 12) is used as an example to illustrate how to identify vertical asymptotes and evaluate limits as x approaches certain values related to those asymptotes.
How does the script suggest evaluating limits when a function approaches a vertical asymptote?
-The script suggests evaluating limits by plugging in values very close to the point of discontinuity (from both the left and right sides) and determining if the function's value is approaching positive or negative infinity.
What is the conclusion for the limit as x approaches 3 for the function f(x) = (x^2 + 2x - 8) / (x^2 + x - 12)?
-The conclusion for the limit as x approaches 3 from the left is negative infinity, and from the right is positive infinity. Since these two values are not equal, the overall limit does not exist.
What technique is used to determine the sign of the function's value as x approaches a certain value?
-The technique used is to plug in values slightly less than or greater than the point of interest and analyze the signs of the resulting expression to determine if the function's value is positive or negative.
How does the script handle the case where the limit is being evaluated from both the left and right sides?
-The script evaluates the limit separately from the left and right sides by plugging in values slightly less than (for the left) or greater than (for the right) the point of interest and then compares the results.
What is the significance of the numerator and denominator signs in determining the limit's value as x approaches a vertical asymptote?
-The signs of the numerator and denominator are crucial because they determine whether the function's value will be positive or negative as it approaches the vertical asymptote, which in turn indicates whether the limit is positive or negative infinity.
What is the script's approach to factoring and evaluating the function g(x) = (1 - x) / (x - 3)?
-The script does not factor g(x) = (1 - x) / (x - 3) because it cannot be factored in a way that would cancel out the x terms. Instead, it directly evaluates the limit by plugging in a value close to the point of interest (x = 3.1) and analyzing the resulting expression.
How does the script handle the limit as x approaches a value where the function is not factorable?
-For non-factorable functions, the script directly evaluates the limit by plugging in values close to the point of interest and analyzing the resulting expression to determine the limit's value.
Outlines
📚 Introduction to Infinite Limits and Vertical Asymptotes
In this first paragraph, Mr. Bortnick introduces the topic of infinite limits and vertical asymptotes in the context of AP Calculus AB. He explains that limits can approach infinity or negative infinity, which are special cases where the function's value does not exist but indicates a trend of unbounded growth or decrease. The concept is connected to vertical asymptotes, which occur when a function cannot cross a certain line, causing the function to either shoot towards positive infinity or plummet towards negative infinity. The instructor provides a function as an example and demonstrates how to identify vertical asymptotes by factoring the numerator and denominator and looking for factors in the denominator that do not cancel out with the numerator.
🔍 Analyzing Limits Approaching Vertical Asymptotes
The second paragraph delves into the process of determining the behavior of limits as they approach vertical asymptotes. Mr. Bortnick suggests using values very close to the point of discontinuity to infer whether the function approaches positive or negative infinity. He illustrates this with a specific function, factoring it and then plugging in values just less than and greater than the point of the vertical asymptote to observe the sign of the result. This method helps in understanding the direction of the function's trend towards the asymptote without needing to graph the function. The paragraph also touches on the concept of removable discontinuities, explaining how they differ from vertical asymptotes.
📉 Evaluating Limits at Vertical Asymptotes
In the third paragraph, the focus is on evaluating the limits at vertical asymptotes for given functions. The instructor provides a step-by-step approach to determine the limits as x approaches specific values from both the left and right sides. He uses the concept of plugging in numbers close to the point of discontinuity to deduce whether the limit tends towards positive or negative infinity. The paragraph also covers how to handle cases where the function has a factorable denominator, indicating a vertical asymptote, and how to assess the limit by considering the signs of the numerator and denominator. The summary concludes with an example of a function with a vertical asymptote at x=1, demonstrating how to find the limit from both sides and confirming that the limit exists and is equal to negative infinity.
Mindmap
Keywords
💡Infinite Limits
💡Vertical Asymptotes
💡Continuity
💡Discontinuities
💡Factoring
💡Numerator
💡Denominator
💡Limit
💡Asymptote Behavior
💡Removable Discontinuity
Highlights
Introduction to the topic of infinite limits and vertical asymptotes in calculus.
Explaining that limits can approach infinity or negative infinity, indicating direction without existing.
Connection between vertical asymptotes and functions that do not cross but approach infinity or negative infinity.
Example of a function with a vertical asymptote at x=4, illustrating the concept.
Method to identify vertical asymptotes by setting the denominator of a rational function to zero.
Factoring the denominator to determine the type of discontinuity and potential vertical asymptotes.
Explanation of how factors in the numerator and denominator relate to removable discontinuities versus vertical asymptotes.
Demonstration of finding vertical asymptotes using the function f(x) = (x^2 + 2x - 8) / (x^2 + x - 12).
Technique of plugging in values close to the point of discontinuity to determine the direction of the limit.
Graphical interpretation of limits approaching vertical asymptotes and the concept of infinity.
Calculating the limit as x approaches 3 from the left and right for the given function.
Determination of the overall limit at x=3 and the conclusion that it does not exist due to differing left and right limits.
Application of the technique to find the limit as x approaches 3 from the right side of the simplified function.
Exploration of the limit as x approaches 1 for the function (1 - x) / (x - 3), considering both left and right approaches.
Factoring the function to identify a vertical asymptote at x=1 and the subsequent limits from both sides.
Final conclusion that the limit as x approaches 1 is negative infinity, based on consistent left and right limits.
Encouragement for students to practice with the provided problems and to seek help for any questions.
Transcripts
Browse More Related Video

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes
Calculus AB/BC – 1.14 Infinite Limits and Vertical Asymptotes
AP Calculus AB - Chapter 1 Review - Part 2
Infinite Limits and Vertical Asymptotes
Math 133 Lecture 2 6
Calculus AB/BC – 1.15 Limits at Infinity and Horizontal Asymptotes
5.0 / 5 (0 votes)
Thanks for rating: