AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes

Daniel Bortnick

12 Oct 202028:46

EducationalLearning

32 Likes 10 Comments

TLDRThe video script by Mr. Bortnick for AP Calculus AB covers Unit 1 on limits and continuity, focusing on the relationship between limits at infinity and horizontal asymptotes. It explains the concept of horizontal asymptotes as lines that a graph approaches but never touches as x values go to infinity. The script provides rules for determining horizontal asymptotes by comparing the growth rates of the numerator and denominator of a rational function. It also discusses the behavior of exponential, polynomial, and logarithmic functions as x approaches infinity, using the 'Fepel' rule to compare growth rates. Several practice problems illustrate how to find horizontal asymptotes and limits at infinity for various functions, including those with trigonometric components.

Takeaways

📚 The lesson is about connecting limits at infinity and horizontal asymptotes in calculus.
📉 Horizontal asymptotes represent the behavior of a graph as it approaches infinity, either to the left or right, and can be approached but not necessarily crossed by the function.
🔍 Limits at infinity are used to determine the y-value a function approaches as x approaches positive or negative infinity, which could be a specific number, infinity, or negative infinity.
🔢 Basic rules for horizontal asymptotes involve comparing the degrees of the numerator and denominator of a rational function to determine the limit as x approaches infinity.
📈 Functions can grow without bound, approach a specific horizontal asymptote, or decay without bound towards negative infinity.
🌟 Exponential functions with larger bases grow faster than those with smaller bases, and are faster than polynomial functions, which in turn grow faster than logarithmic functions.
📊 To determine the horizontal asymptote, consider the behavior of the largest exponent terms in the numerator and denominator of a rational function.
🤔 The function's growth rate helps in identifying whether the limit approaches zero, a specific value, or infinity.
📐 Practice problems illustrate how to find horizontal asymptotes by focusing on the highest degree terms and understanding their behavior as x approaches infinity or negative infinity.
🚫 Special trigonometric rules, such as sine of x over x approaching 1 as x approaches 0, do not apply when considering limits at infinity.
🌠 The behavior of trigonometric functions like sine and cosine when combined with infinity results in oscillating functions without a defined horizontal asymptote.

Q & A

What is the main topic of the video script?
-The main topic of the video script is 'Limits at Infinity and Horizontal Asymptotes' in the context of AP Calculus AB.
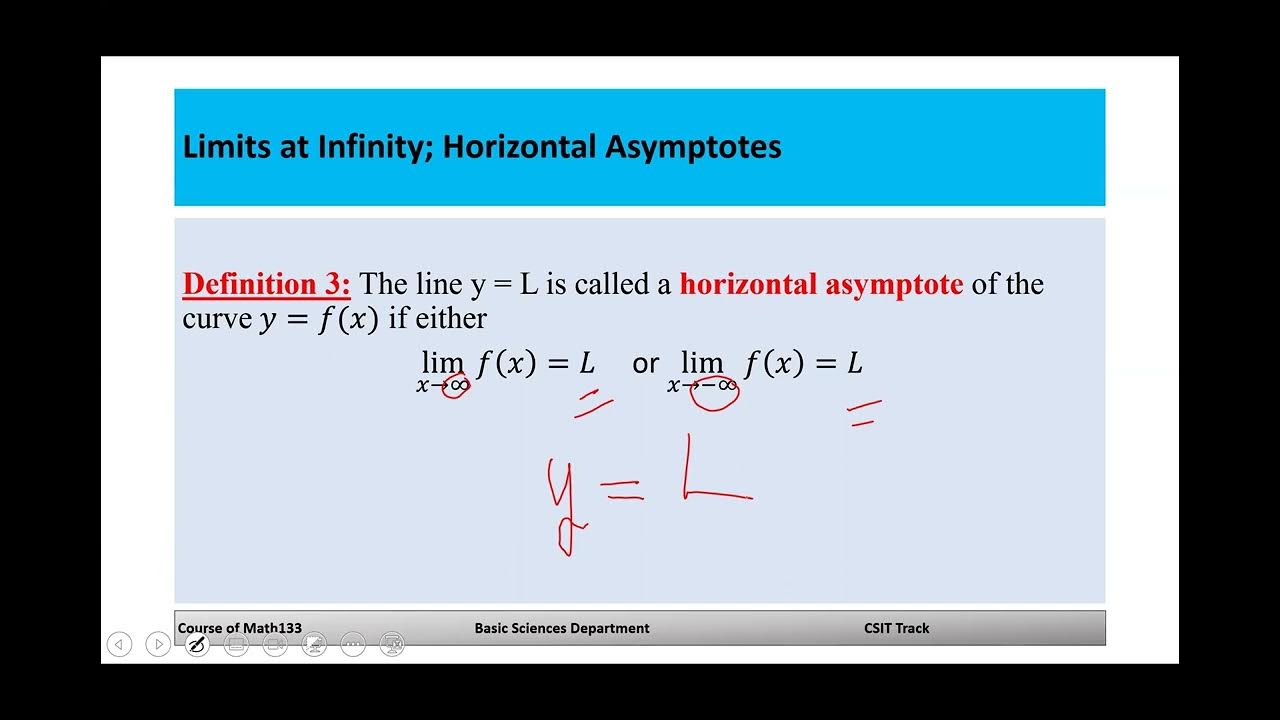
What is a horizontal asymptote and how does it differ from a vertical asymptote?
-A horizontal asymptote is a line that a graph of a function approaches as the value of x gets very large or very small, without actually touching it. Unlike a vertical asymptote, where the function is not defined at a certain x value, a horizontal asymptote may be crossed by the function multiple times.
How can a function cross its horizontal asymptote?
-A function can cross its horizontal asymptote if it oscillates, meaning it bounces back and forth along the asymptote, getting closer to it as it approaches infinity.
What are the basic rules for determining horizontal asymptotes in the context of rational functions?
-The basic rules for determining horizontal asymptotes involve comparing the degrees of the numerator and denominator of a rational function. If the degree of the denominator is greater, the limit approaches zero. If they are equal, the horizontal asymptote is the ratio of the leading coefficients. If the degree of the numerator is greater, the function grows without bound.
What does the script suggest about the growth of exponential functions compared to polynomial functions?
-The script suggests that exponential functions will always grow faster than polynomial functions as x approaches infinity.
What is the FEP rule mentioned in the script, and how does it help in understanding the growth of functions?
-The FEP rule is a mnemonic for remembering the order of growth of different types of functions: Factorials, Exponential, Polynomial, and Logarithmic functions. It helps in understanding that exponential functions grow faster than polynomial functions, which in turn grow faster than logarithmic functions.
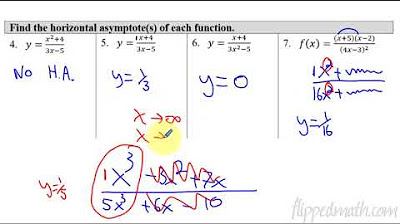
How can you determine the horizontal asymptote of a function like y = x^2 + 4 / (3x - 5)?
-To determine the horizontal asymptote, you only consider the terms with the largest exponents. In this case, x^2 in the numerator and 3x in the denominator. Since the exponent of x in the numerator is higher, the function grows without bound and has no horizontal asymptote.
What is the horizontal asymptote of y = x + 4 / (3x - 5) as x approaches infinity?
-The horizontal asymptote is y = 1/3, as the exponents of the largest terms in the numerator and denominator are the same, resulting in the ratio of the coefficients (1/3).
How does the script explain the behavior of the function y = (x^2 + 3x - 10) / (4x^2 - 3) as x approaches infinity?
-The script explains that the function does not have a horizontal asymptote because the degree of the polynomial in the denominator is higher than that in the numerator, causing the function to grow without bound.
What is the significance of understanding limits at infinity and horizontal asymptotes in calculus?
-Understanding limits at infinity and horizontal asymptotes is significant as it helps in determining the long-term behavior of functions, which is crucial for analyzing the behavior of mathematical models in various real-world applications.

Outlines

00:00

📚 Introduction to Limits and Horizontal Asymptotes

Mr. Bortnick introduces the topic of limits at infinity and horizontal asymptotes in calculus, explaining the concept of horizontal asymptotes as lines that a graph approaches but never touches as it extends towards positive or negative infinity. He contrasts this with vertical asymptotes, where the function is undefined at a specific point. The instructor also discusses the behavior of functions as they approach infinity, including growing without bound or decaying without bound, and introduces basic rules for determining horizontal asymptotes by comparing the growth rates of the numerator and denominator of a rational function.

05:00

📈 Understanding Growth Rates and Horizontal Asymptotes

The script delves into how to determine horizontal asymptotes by examining the growth rates of functions in the numerator and denominator. It explains that if the denominator grows faster, the limit approaches zero, while if the numerator and denominator grow at the same rate, the horizontal asymptote is the ratio of the leading coefficients. If the numerator grows faster, the function grows without bound. The instructor provides examples of functions and their growth rates, emphasizing the importance of recognizing which functions grow faster as x values increase, using the FEP (Factorials, Exponential, Polynomial, Logarithmic) rule to compare growth rates.

10:02

🔍 Analyzing Practice Problems on Horizontal Asymptotes

Practice problems are presented to find horizontal asymptotes, which is equivalent to finding the limit as x approaches infinity. The instructor focuses on the terms with the largest exponents, as they dominate the behavior of the function at infinity. He explains that if the exponents are the same, the horizontal asymptote is the ratio of the coefficients, and if not, the function may grow without bound or approach zero. The script also discusses how to handle factored expressions and the importance of comparing the largest terms' exponents to determine the horizontal asymptote.

15:05

📉 Horizontal Asymptotes at Positive and Negative Infinity

The script addresses how functions can have different horizontal asymptotes as x approaches positive and negative infinity. It provides examples of functions with a single horizontal asymptote in both directions and those with different asymptotes for positive and negative infinity. The instructor explains how to determine the asymptotic behavior in both directions by considering the impact of squaring and taking square roots of terms involving infinity.

20:06

🌐 Exponential Functions and Trigonometric Limits

The instructor discusses the behavior of exponential functions like e^x and their horizontal asymptotes, emphasizing that e^x has a horizontal asymptote at y=0. He also touches on the limits involving exponential functions as x approaches infinity and negative infinity, explaining that these limits approach zero or grow without bound, depending on the exponent's sign. Additionally, the script introduces the concept of trigonometric functions and their limits as x approaches infinity, noting that sine and cosine functions oscillate and do not have a horizontal asymptote.

25:07

📚 Conclusion and Further Practice

The script concludes with a summary of the different approaches to finding horizontal asymptotes and limits at infinity, including the use of trigonometric identities and the behavior of exponential functions. The instructor encourages students to practice these concepts and check their answers, offering help during class or office hours for any questions. He emphasizes the importance of understanding the behavior of functions at infinity for a deeper comprehension of calculus.

Mindmap

Keywords

💡Horizontal Asymptote

A horizontal asymptote is a horizontal line that a function approaches as its argument increases without bound or decreases without bound. In the context of the video, horizontal asymptotes are used to determine the behavior of a graph as it extends towards positive or negative infinity. The script explains that unlike vertical asymptotes, functions can cross horizontal asymptotes and that they can provide insights into the long-term behavior of a function, such as whether it grows without bound or levels off at a certain value.

💡Limit at Infinity

The limit at infinity refers to the value that a function approaches as its input (x-value) approaches either positive or negative infinity. The script discusses how to find horizontal asymptotes by evaluating these limits, which is crucial for understanding the end behavior of functions. For instance, if a function's limit as x approaches infinity is a specific number, the graph of the function will approach a horizontal line at that y-value as x increases without bound.

💡Vertical Asymptote

A vertical asymptote is a value of x for which the function is not defined and around which the graph of the function approaches infinity or negative infinity. The video script contrasts vertical asymptotes with horizontal asymptotes, noting that while a function never touches a vertical asymptote, it can cross a horizontal asymptote. The concept is foundational for understanding the behavior of functions at their undefined points.

💡Growth Without Bound

Growth without bound describes a situation where a function's value increases indefinitely as its input (x-value) approaches a certain value, typically infinity. In the script, this concept is used to describe functions like e^x, which continue to rise without ever reaching a maximum value. This is an important aspect of understanding the behavior of exponential functions.

💡Numerator and Denominator

In the context of the video, the numerator and denominator refer to the top and bottom parts, respectively, of a fraction, which is often a rational function in calculus. The script explains how to compare the growth rates of the numerator and denominator to determine the horizontal asymptote of a rational function. If the denominator grows faster, the function approaches zero; if they grow at the same rate, the horizontal asymptote is the ratio of the leading coefficients.

💡Leading Coefficients

Leading coefficients are the numerical factors in front of the terms with the highest power in the numerator and denominator of a rational function. According to the script, when the exponents of the leading terms in the numerator and denominator are the same, the horizontal asymptote is determined by the ratio of these leading coefficients. This concept is key to finding horizontal asymptotes for certain types of functions.

💡Exponential Functions

Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant. The video script mentions that exponential functions grow faster than polynomial functions as x approaches infinity. Examples given in the script include 2^x, e^x, and 4^x, which are used to illustrate the concept of growth rates and how they relate to horizontal asymptotes.

💡Polynomial Functions

Polynomial functions are expressions involving a sum of powers in the variable raised to non-negative integer exponents. The script discusses how the growth rate of polynomial functions is determined by the highest exponent, with higher exponents leading to faster growth. Polynomial functions are a common type of function for which students learn to determine horizontal asymptotes.

💡Logarithmic Functions

Logarithmic functions are the inverse of exponential functions, often written as log_b(x), where 'b' is the base and 'x' is the argument. The script places logarithmic functions at the slowest end of the growth rate spectrum, meaning they grow more slowly than both polynomial and exponential functions as x approaches infinity.

💡Trigonometric Functions

Trigonometric functions, such as sine and cosine, are periodic functions that relate the angles of a triangle to the lengths of its sides. In the script, the behavior of these functions as x approaches infinity or negative infinity is discussed, particularly in the context of their limits. For example, the script mentions that the sine function oscillates between -1 and 1, and thus does not have a horizontal asymptote.

Highlights

Introduction to the concept of horizontal asymptotes and their difference from vertical asymptotes.

Explanation of how a graph approaches a horizontal asymptote as it goes to infinity without necessarily touching it.

Discussion on the possibility of functions crossing horizontal asymptotes multiple times and the concept of oscillation.

Importance of horizontal asymptotes in determining the behavior of a graph as it approaches infinity.

Illustration of how to find limits at infinity and their relation to horizontal asymptotes.

Differentiation between functions that grow without bound versus those approaching a specific horizontal asymptote.

Basic rules for determining horizontal asymptotes by comparing the growth rates of the numerator and denominator of a fraction.

Use of the FEPOL theorem to rank functions by their growth rate from fastest to slowest as x approaches infinity.

Analysis of exponential functions and their growth compared to polynomial and logarithmic functions.

Practice problems demonstrating how to find horizontal asymptotes by examining the largest exponent terms in a function.

Explanation of how to handle factored expressions when determining horizontal asymptotes and the irrelevance of removable discontinuities in this context.

Examples of functions with different horizontal asymptotes for positive and negative infinity, showcasing varying behavior.

Background knowledge on the e^x function, its characteristics, and its horizontal asymptote at y=0.

Approach to solving limits involving exponential functions and their behavior as x approaches infinity.

Introduction to trigonometric functions and their limits, specifically sine and cosine, in the context of horizontal asymptotes.

Special trigonometric limit rules and their applicability only when x approaches zero, not infinity.

Conclusion emphasizing the importance of understanding function growth rates for determining horizontal asymptotes and limits at infinity.

Transcripts

Browse More Related Video

Calculus AB/BC – 1.15 Limits at Infinity and Horizontal Asymptotes

Math 133 Lecture 2 6

AP Calculus AB - 1.14 Connecting Infinite Limits and Vertical Asymptotes

Limits Involving Infinity

Math 1325 Lecture 9 2 - Continuous Functions & Limits at Infinity

Finding a Horizontal Asymptote of a Rational Function (Precalculus - College Algebra 40)

Related Tags

Calculus Limits Continuity Asymptotes Infinity Trigonometry Exponential Growth Educational Content Mathematics AP Study Horizontal Asymptote