How To Find The LCM and HCF Quickly!

The Organic Chemistry Tutor
20 Apr 201907:24
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a clear guide on calculating the Least Common Multiple (LCM) and Highest Common Factor (HCF), also known as the Greatest Common Factor (GCF), for pairs and trios of numbers. It demonstrates the process of listing multiples and factors to identify the LCM as the smallest number divisible by all given numbers and the HCF as the largest factor common to all. Examples with numbers like 2, 3, 4, 12, 16, 24, 30, 40, 60, and 75 are used to illustrate the method, making it accessible for viewers to grasp these fundamental mathematical concepts.

Takeaways
  • 🔢 The script introduces the concepts of LCM (Least Common Multiple) and HCF (Highest Common Factor), also known as GCF (Greatest Common Factor).
  • 📝 To find the LCM of two numbers, list the multiples of each number and identify the smallest number that appears in both lists.
  • 📝 For the HCF, list the factors of each number and find the highest number that is common to both lists.
  • 📐 The script provides a step-by-step example to find the LCM of 2 and 3, which is 6.
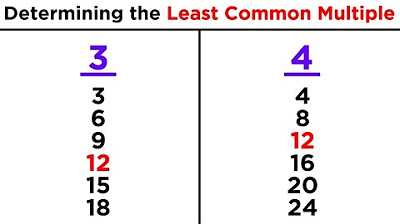
  • 📐 Another example demonstrates finding the LCM of 3 and 4, resulting in 12.
  • 📐 The script also shows how to find the LCM of larger numbers, such as 12 and 16, which is 48.
  • 📐 An example with three numbers (2, 3, and 4) is given to find the LCM, which is 12.
  • 🔢 The HCF of 12 and 16 is found to be 4, by comparing common factors.
  • 🔢 The script explains how to find the HCF of 24 and 30, which is 6.
  • 🔢 The HCF for three numbers (40, 60, and 75) is determined to be 5.
  • 👍 The video concludes by summarizing the process of finding LCM and HCF for two or three numbers and encourages viewers to subscribe.
Q & A

  • What does LCM stand for?

    -LCM stands for Least Common Multiple, which is the smallest number that is a multiple of two or more numbers.

  • How do you find the LCM of two numbers, for example, 2 and 3?

    -To find the LCM of 2 and 3, you list the multiples of each number and identify the smallest number that appears in both lists. In this case, the multiples of 2 are 2, 4, 6, 8, 10, 12, etc., and for 3, they are 3, 6, 9, 12, 15, etc. The smallest common multiple is 6, so the LCM of 2 and 3 is 6.

  • What is the LCM of the numbers 3 and 4?

    -The LCM of 3 and 4 is found by listing the multiples of each number. For 3, the multiples are 3, 6, 9, 12, 15, 18, etc., and for 4, they are 4, 8, 12, 16, etc. The smallest common multiple is 12, so the LCM of 3 and 4 is 12.

  • How can you find the LCM of larger numbers, such as 12 and 16?

    -To find the LCM of 12 and 16, list the multiples of each number. For 12, they are 12, 24, 36, 48, 60, 72, etc., and for 16, they are 16, 32, 48, 64, 80, etc. The smallest number that appears in both lists is 48, so the LCM of 12 and 16 is 48.

  • What is the LCM of three numbers, 2, 3, and 4?

    -To find the LCM of 2, 3, and 4, list the multiples of each: for 2, they are 2, 4, 6, 8, 10, 12, etc.; for 3, they are 3, 6, 9, 12, 15, etc.; and for 4, they are 4, 8, 12, 16, 20, etc. The smallest common multiple in all three lists is 12, so the LCM of 2, 3, and 4 is 12.

  • What does HCF stand for and what is its equivalent term?

    -HCF stands for Highest Common Factor, which is the same as the GCF, or Greatest Common Factor, representing the largest factor that two or more numbers have in common.

  • How do you find the HCF of 12 and 16?

    -To find the HCF of 12 and 16, list the factors of each number. For 12, the factors are 1, 2, 3, 4, 6, and 12. For 16, the factors are 1, 2, 4, 8, and 16. The highest number that appears in both lists is 4, so the HCF of 12 and 16 is 4.

  • What is the HCF of the numbers 24 and 30?

    -The HCF of 24 and 30 is found by listing the factors of each number. For 24, the factors are 1, 2, 3, 4, 6, 8, 12, and 24. For 30, the factors are 1, 2, 3, 5, 6, 10, 15, and 30. The highest common factor in both lists is 6, so the HCF of 24 and 30 is 6.

  • How can you find the HCF of three numbers, 40, 60, and 75?

    -To find the HCF of 40, 60, and 75, list the factors of each: for 40, they are 1, 2, 4, 5, 8, 10, 20, and 40; for 60, they are 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, and 60; and for 75, they are 1, 3, 5, 15, 25, and 75. The highest common factor in all three lists is 5, so the HCF of 40, 60, and 75 is 5.

  • Why is the HCF useful in mathematics and problem-solving?

    -The HCF is useful in mathematics and problem-solving because it helps to simplify fractions, find common factors, and determine the greatest number of times a particular factor divides into multiple numbers, which is essential in various calculations and mathematical operations.

Outlines
00:00
🔢 Understanding LCM and HCF Basics

This paragraph introduces the concepts of Least Common Multiple (LCM) and Highest Common Factor (HCF), also known as Greatest Common Factor (GCF). The video explains how to find the LCM of two numbers, using 2 and 3 as examples, by listing their multiples and identifying the smallest common one, which is 6. It then challenges the viewer to find the LCM of 3 and 4, and provides the solution, which is 12. The paragraph also covers finding the LCM of larger numbers, such as 12 and 16, and concludes with 48 as the LCM. Additionally, it touches on finding the LCM of three numbers, 2, 3, and 4, and identifies 12 as the LCM. The paragraph sets the stage for further exploration of these mathematical concepts.

05:01
📚 Advanced Techniques for Finding LCM and HCF

In this paragraph, the script delves deeper into finding the LCM and HCF with examples involving larger and multiple numbers. It explains the process of finding the HCF of 12 and 16 by listing their factors and identifying the highest common one, which is 4. The viewer is then encouraged to find the HCF of 24 and 30, and the solution reveals 6 as the HCF. The paragraph extends the concept to three numbers, 40, 60, and 75, and demonstrates how to find their HCF, concluding with 5 as the common factor. The summary emphasizes the step-by-step method of listing multiples for LCM and factors for HCF, providing a clear guide for viewers to understand and apply these mathematical techniques.

Mindmap
Keywords
💡LCM
LCM stands for Least Common Multiple, which is the smallest non-zero number that is a multiple of two or more numbers. In the video, the LCM is used to find the smallest number that can be divided evenly by the given numbers. For example, the LCM of 2 and 3 is 6, as it is the smallest number that both 2 and 3 divide into without leaving a remainder.
💡HCF
HCF, also known as the Highest Common Factor or GCF (Greatest Common Factor), is the largest positive integer that divides two or more numbers without leaving a remainder. The video explains how to find the HCF by listing the factors of each number and identifying the largest factor they have in common. For instance, the HCF of 12 and 16 is 4, as it is the highest number that divides both 12 and 16 evenly.
💡Multiples
Multiples are the product of a number and any integer. In the context of finding the LCM, the video script involves listing the multiples of given numbers to identify the smallest common multiple. For example, multiples of 2 are 2, 4, 6, 8, 10, and so on, and the multiples of 3 are 3, 6, 9, 12, 15, etc., which helps in determining the LCM of 2 and 3 as 6.
💡Factors
Factors are the numbers that divide into another number without leaving a remainder. The video script uses the concept of factors to determine the HCF of two numbers by listing the factors of each and finding the highest one they share. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12, and the HCF of 12 and 16 is found to be 4 by comparing their factors.
💡Divisibility
Divisibility refers to the property of a number being able to be divided by another number without leaving a remainder. The video explains that to find the LCM, one must find the lowest number that is divisible by all the given numbers. For example, 48 is divisible by both 12 and 16, making it the LCM of these two numbers.
💡Greatest
In the context of the video, 'greatest' is used to describe the largest common factor shared between two or more numbers, which is the HCF or GCF. The script illustrates this by finding the greatest factor that 24 and 30 have in common, which is 6, thus making it their HCF.
💡Least
'Least' is used to describe the smallest common multiple shared between two or more numbers, which is the LCM. The video demonstrates this by finding the least number that is a multiple of both 2 and 3, which is 6, thus establishing it as their LCM.
💡Integer
An integer is a whole number that can be positive, negative, or zero. In the video, when discussing divisibility and factors, integers are used to determine if a number can be divided without leaving a remainder. For example, the script mentions that 4 does not divide into 30, indicating that 30 is not an integer multiple of 4.
💡Divide
To divide in the context of the video means to split a number into equal parts according to another number. The script uses division to find common multiples and factors. For example, when finding the LCM of 12 and 16, the script divides both numbers by 48 to show that 48 is divisible by both, hence the LCM.
💡Remainder
A remainder is what is left over after division of one number by another that does not result in an exact quotient. The video script mentions remainders in the context of finding factors and multiples, where no remainder indicates a number is a multiple or a factor of another. For example, since 48 divided by 12 has no remainder, it is a multiple of 12.
Highlights

Introduction to the concept of LCM (Least Common Multiple) and its significance.

Explanation of how to find the LCM of two numbers using the example of 2 and 3.

Identification of 6 as the LCM of 2 and 3, demonstrating the method.

Encouragement for viewers to pause and try finding the LCM of 3 and 4.

Demonstration of finding the LCM of 3 and 4, with 12 identified as the result.

Introduction of a larger number example, LCM of 12 and 16, with the result being 48.

Explanation of the division method to confirm the LCM, using 48 as an example.

Introduction to finding the LCM of three numbers, using 2, 3, and 4 as an example.

Identification of 12 as the LCM for the numbers 2, 3, and 4.

Transition to the concept of HCF (Highest Common Factor), also known as GCF (Greatest Common Factor).

Explanation of how to find the HCF of 12 and 16, with the result being 4.

Encouragement for viewers to work out the HCF of 24 and 30 on their own.

Demonstration of finding the HCF of 24 and 30, with the result being 6.

Introduction to finding the HCF for three numbers, using 40, 60, and 75 as an example.

Identification of 5 as the HCF for the numbers 40, 60, and 75.

Conclusion of the video with a summary of how to find LCM and HCF for two or three numbers.

Call to action for viewers to subscribe to the channel for more educational content.

Transcripts

Browse More Related Video

Greatest Common Factor (GCF)

How To Find The LCM of 3 Numbers - Plenty of Examples!

How To Find The Greatest Common Factor Quickly!

GED MATH 2024 Preparation Course - from the Absolute Beginning to Advanced Level

Least Common Multiple (LCM)

5 Essential GCSE Maths Calculator Skills You Need To Know! | Casio Calculator

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
LCMHCFGreatest Common FactorLeast Common MultipleMathematicsEducationalNumber TheoryMultiplesFactorsTutorial