A Level Physics Revision: All of Work, Energy and Power (in 18 minutes)
TLDRIn this educational video, the host delves into the fundamental concepts of work, energy, and power in physics, tailored to the OCR physics A-level specification but universally applicable. They explain the formula for work done, emphasizing the role of force, distance, and angle, and highlight when work is maximized. The principle of conservation of energy is discussed, illustrating the transformation between kinetic and potential energy. The video also derives the equations for kinetic and potential energy from first principles and explores real-world scenarios involving resistive forces. Finally, it touches on power as the rate of work done and introduces the concept of mechanical efficiency, providing a comprehensive review of these key physics topics.
Takeaways
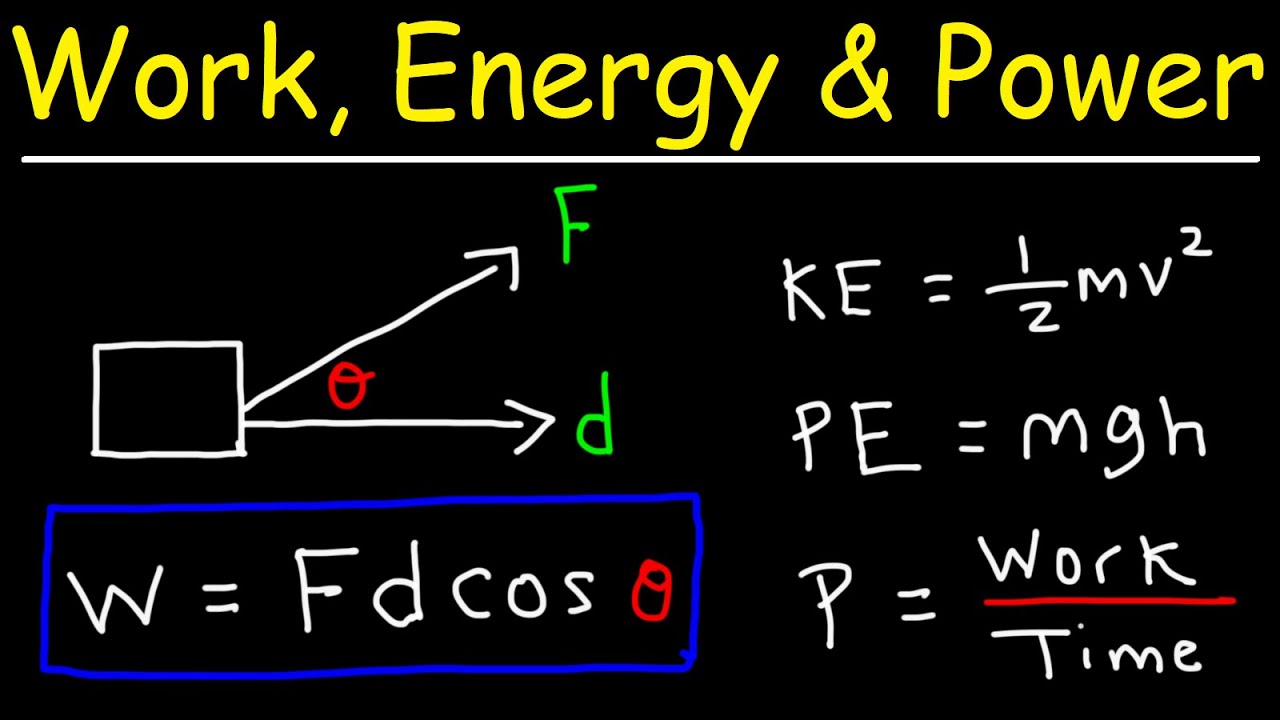
- 🔧 Work done is defined as the product of force and the distance moved in the direction of the force.
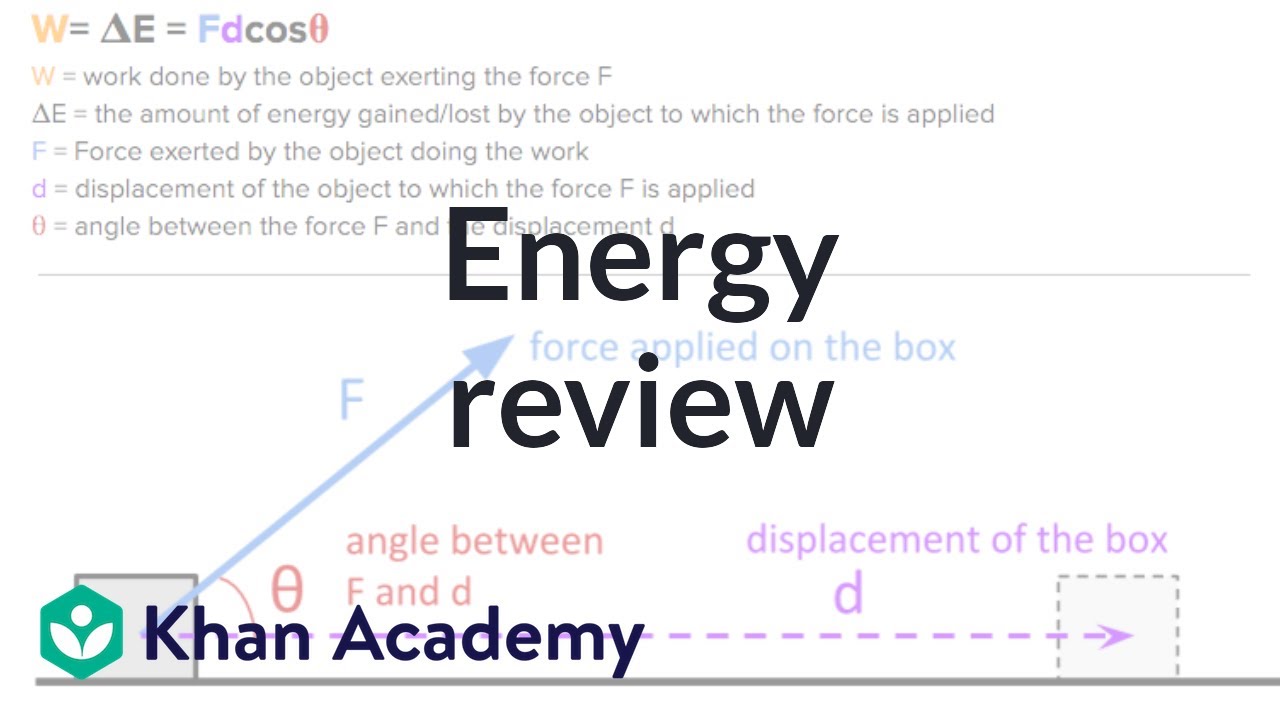
- 📐 The formula for work done is W = F * cos(θ), where θ is the angle between the force and the displacement.
- 🔍 Maximum work is done when the force is parallel to the displacement (θ = 0 degrees), and no work is done when the force is perpendicular (θ = 90 degrees).
- ⚖️ The unit for work done is the joule, which is the same as the unit for energy.
- 🔄 The principle of conservation of energy states that energy cannot be created or destroyed, only transferred between forms.
- 🌐 The change in energy of a system is equal to the work done on that system, expressed as ΔE = E_final - E_initial = Work done.
- 📚 Potential energy (PE) is given by the formula PE = m * g * h, where m is mass, g is gravity, and h is height.
- 🚀 Kinetic energy (KE) is derived from the work-energy principle and is given by KE = 1/2 * m * v^2.
- ↕️ The exchange between gravitational potential energy and kinetic energy can be calculated using conservation of energy principles.
- 🛤️ An example calculation showed how to determine the final speed of a ball rolling down a hill using potential and kinetic energy.
- ⏳ Power is the rate of work done or energy transfer, mathematically expressed as P = W/t or P = F * v, where v is velocity.
- 🛠️ Mechanical efficiency of a system is calculated by comparing the useful output energy to the total input energy, expressed as a percentage.
Q & A
What is the definition of work done in physics?
-Work done is defined as the product of the force applied and the distance moved in the direction of the force.
What is the formula for work done in A-Level Physics?
-The formula for work done is W = F × cos(θ), where F is the force, θ is the angle between the force and the displacement, and W is the work done.
When is work done at its maximum?
-Work done is at its maximum when the angle θ is zero degrees, meaning the force is parallel to the displacement.
What is the unit of work done and how is it related to the unit of energy?
-The unit of work done is the joule, which is the same as the unit for energy.
How is the base unit of the joule derived from the formula for work done?
-The base unit of the joule is derived from the formula W = F × x × cos(θ), where force (F) is in newtons (kg·m/s²), distance (x) is in meters, and cosine has no units, resulting in the joule (kg·m²/s²).
What is the principle of conservation of energy?
-The principle of conservation of energy states that energy cannot be created or destroyed, only transferred from one form to another.
How is the change in energy in a system related to the work done on that system?
-The change in energy in a system is equal to the work done on that system, represented by ΔE = E_final - E_initial = W.
What are the equations for potential and kinetic energy?
-Potential energy is given by PE = m × g × h, and kinetic energy is given by KE = 0.5 × m × v².
How can the equation for kinetic energy be derived from the principle of conservation of energy?
-The equation for kinetic energy can be derived by considering the work done on an object and applying Newton's second law, leading to the equation ΔE = m × a × s, and using the SUVAT equation v² = u² + 2as, which can be rearranged to find a × s = v² / 2.
What is power and how is it mathematically defined?
-Power is the rate of work done or the rate of energy transfer, mathematically defined as P = W / t, where W is work and t is time.
How can power be expressed in terms of force and velocity?
-Power can also be expressed as P = F × v, where F is the force applied and v is the velocity of the object.
What is the mechanical efficiency of a system and how is it calculated?
-Mechanical efficiency of a system is the ratio of useful output energy to the total input energy, calculated as a percentage using the formula: Efficiency (%) = (Useful Output Energy / Total Input Energy) × 100.
Outlines
🔧 Work, Energy, and Power Basics
This paragraph introduces the topic of work, energy, and power within the context of the OCR physics specification. The presenter explains the concept of work done as the product of force and the distance moved in the direction of the force. The formula for work is presented as W = F * cos(theta), where theta is the angle between the force and the displacement. It's emphasized that maximum work is done when the force is parallel to the displacement (theta = 0 degrees), and no work is done when the force is perpendicular (theta = 90 degrees). The unit of work, the joule, is also discussed, and its relation to mass, acceleration, and distance is explored through the derived formula for work.
🌐 Principles of Energy Conservation and Energy Types
The paragraph delves into the principle of conservation of energy, stating that energy can neither be created nor destroyed but only transformed from one form to another. Potential and kinetic energy are introduced as two forms of energy, with potential energy defined by the formula mgh, where m is mass, g is the acceleration due to gravity, and h is height. The presenter explains how work done is related to the change in energy of an object, and how this principle can be used to derive the equations for potential and kinetic energy. The concept of energy transformation is illustrated through the example of raising and dropping an object, and the connection between work done and energy change is highlighted.
🚀 Derivation of Kinetic Energy and Energy Exchange
Building on the previous discussion, this paragraph focuses on the derivation of the kinetic energy formula from first principles. The kinetic energy equation, KE = 1/2 mv^2, is derived by considering the work done on an object and applying Newton's second law (F = ma) along with the SUVAT equations. The example of a ball rolling down a hill is used to demonstrate the exchange between gravitational potential energy and kinetic energy, showing how the initial potential energy is converted to kinetic energy at the bottom of the hill. The final speed of the ball is calculated using the conservation of energy principle, and a realistic scenario involving resistive forces is introduced to calculate the resistive force acting on the ball.
⚙️ Power and Mechanical Efficiency
The final paragraph shifts the focus to power, defined as the rate of work done or energy transfer. The formula for power, P = W/t, is introduced, and alternative expressions for power are explored, including P = F * v, where v is velocity. The concept of mechanical efficiency is also discussed, explaining how it can be calculated using the ratio of useful output energy to total input energy. The efficiency is expressed as a percentage, providing a measure of how effectively a system converts input energy into useful work. The summary concludes with a recap of the topics covered in the video, emphasizing the importance of understanding work, energy, and power in physics.
Mindmap
Keywords
💡Work Done
💡Joule
💡Conservation of Energy
💡Kinetic Energy
💡Potential Energy
💡Power
💡Mechanical Efficiency
💡Force
💡Displacement
💡Resistive Forces
Highlights
Work done is defined as the product of force and the distance moved in the direction of the force.
The formula for work done is W = F x cos(θ), where F is force, θ is the angle between force and displacement.
Work is maximized when the force is parallel to the displacement, i.e., when θ equals zero degrees.
Work done is zero when the force is perpendicular to the displacement, i.e., when θ equals 90 degrees.
The unit for work done is the joule, which is also the unit for energy.
The base unit of the joule is derived from the units of mass, distance, and acceleration.
The principle of conservation of energy states that energy cannot be created or destroyed, only transferred.
The change in energy of a system is equal to the work done on that system.
Potential energy is given by mgh, where m is mass, g is acceleration due to gravity, and h is height.
Potential energy can be derived from the work done against gravity to raise an object.
Kinetic energy can be derived from the work-energy principle and Newton's second law.
The formula for kinetic energy is KE = 1/2 mv^2, derived from the work done on an object.
The exchange between gravitational potential and kinetic energy can be analyzed using conservation of energy.
The final speed of an object rolling down a hill can be calculated using the conservation of energy principle.
Resistive forces can be calculated using the change in energy and the work done against these forces.
Power is defined as the rate of work done or energy transfer, given by W/t or F×v.
Mechanical efficiency of a system can be calculated using the ratio of useful output energy to total input energy.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: