Work and Energy
TLDRThis lecture delves into the physics of work and energy, defining work as the product of force and displacement in a specific direction. It distinguishes work in physics from everyday understanding and explores the relationship between work, kinetic energy, and potential energy. The script covers the angle dependence of work, the calculation of work done by forces such as gravity, and introduces the concepts of conservative and non-conservative forces. It also explains the work-energy theorem, the conservation of mechanical energy, and concludes with the principle of energy conservation in various forms, emphasizing that energy can only be transformed, not created or destroyed.
Takeaways
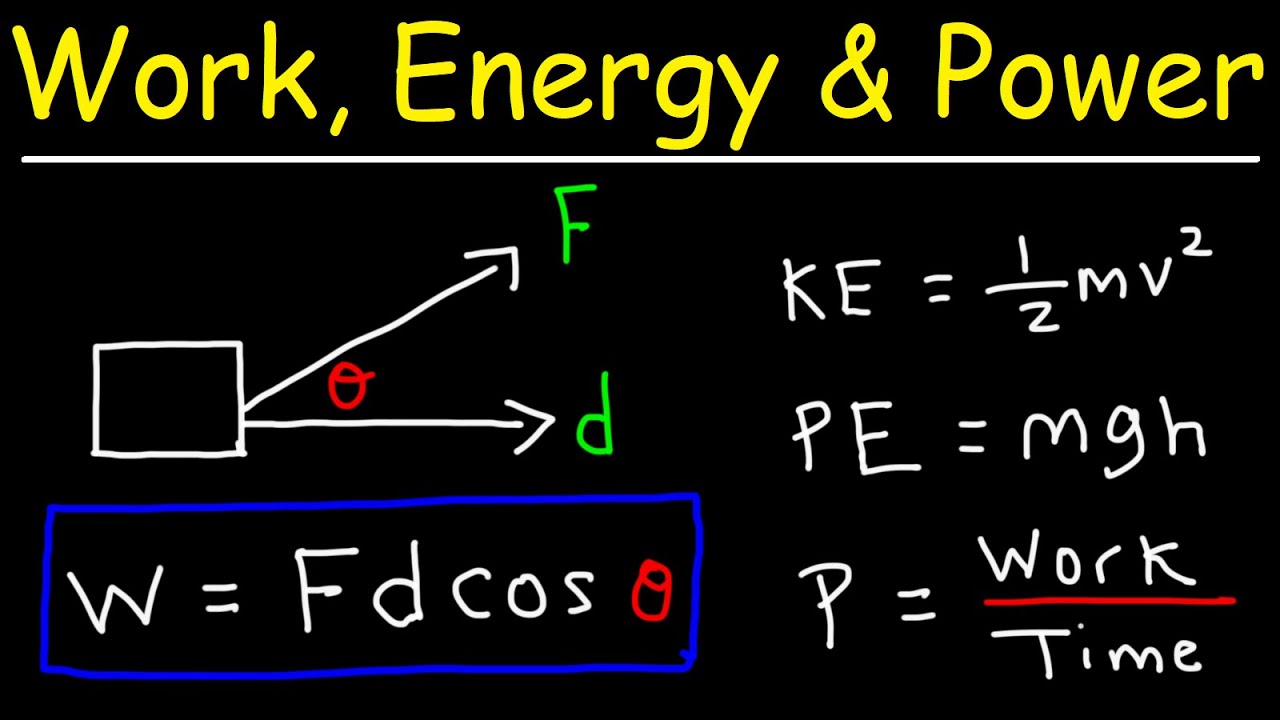
- 🔧 The definition of work in physics is the product of the force applied on an object and the distance of displacement in the direction of the force, differing from everyday understanding.
- 📏 Work is calculated as the dot product of force and displacement vectors, which accounts for direction and requires the angle between them.
- 📐 The unit of work is the joule, derived from the product of the units of force (newtons) and distance (meters), and is used to avoid confusion with other units like torque.
- ⚡ The work-energy theorem links the work done by a net external force to the change in an object's kinetic energy, expressed as the final kinetic energy minus the initial kinetic energy.
- 🎯 The angle between force and displacement affects the work done, with maximum work occurring when they are parallel and minimum (or zero) when they are perpendicular.
- 🌐 Gravitational potential energy is the energy an object possesses due to its position in a gravitational field, calculated as the product of mass, gravitational acceleration, and height above a reference level.
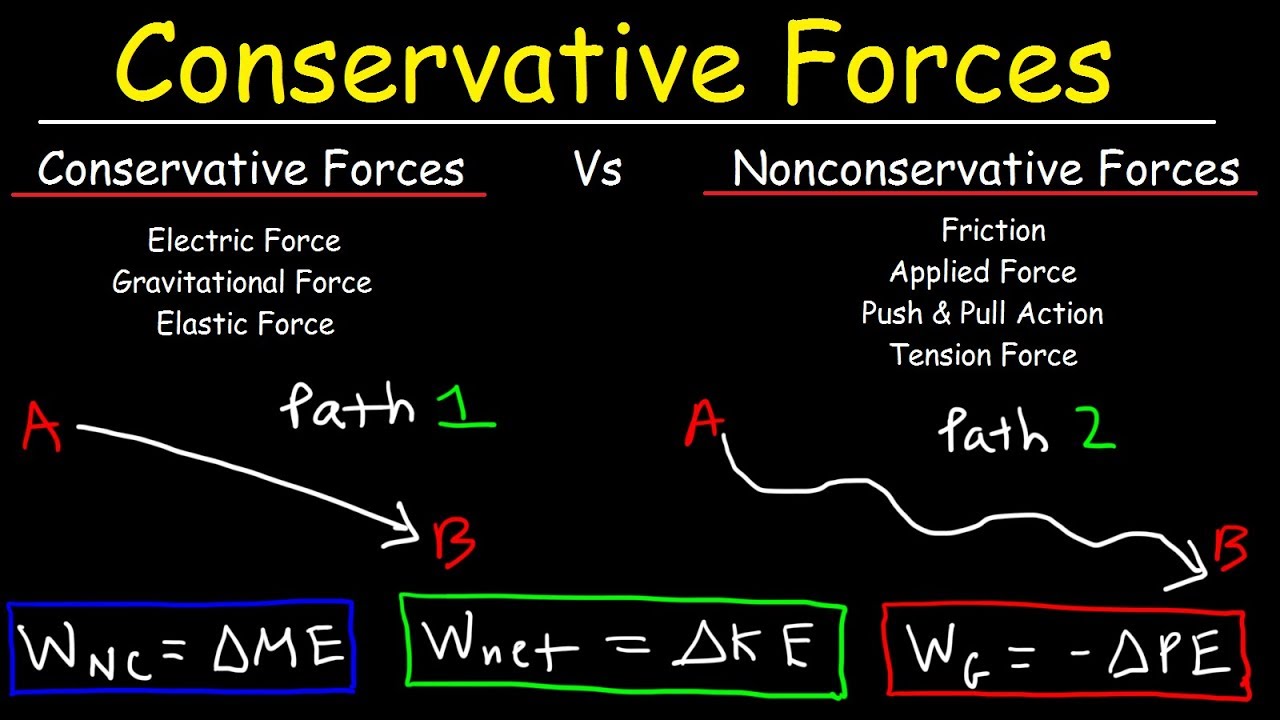
- 🔄 Conservative forces, like gravity and elastic spring force, do work that is independent of the path taken and can be fully recovered or converted into other forms of energy.
- 🚫 Non-conservative forces, such as friction, dissipate energy as heat and do not satisfy the conditions of conservative forces, leading to a loss of mechanical energy.
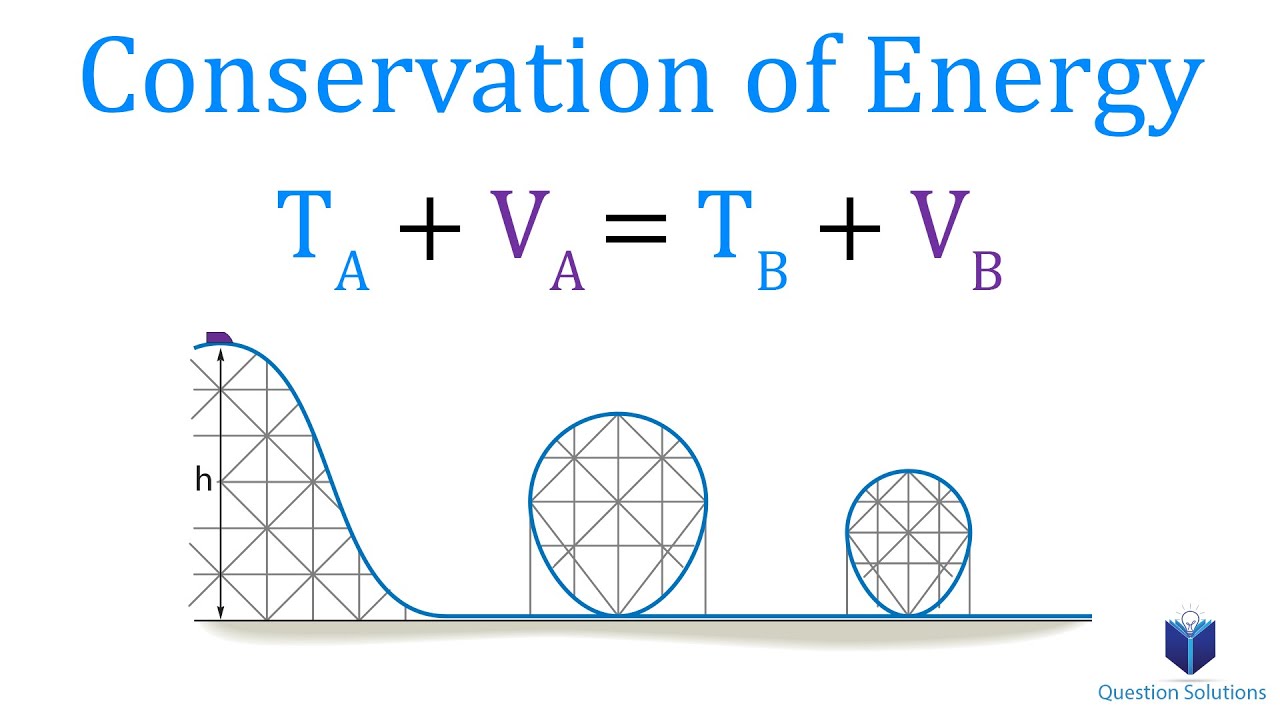
- ⚖️ The principle of conservation of mechanical energy states that the total mechanical energy of an isolated system remains constant if no non-conservative forces do work on the system.
- ⏱️ Power is the rate at which work is done, measured in watts, and reflects the efficiency of the work process by considering the time taken to perform the work.
- 🌟 The broader principle of conservation of energy in the universe asserts that energy cannot be created or destroyed, only transformed from one form to another, maintaining a universal balance.
Q & A
What is the definition of work in physics?
-In physics, work is defined as the product of the force applied on an object and the distance of displacement the object experiences as a result of that force in the direction of the force.
What is the relationship between work and energy?
-Work is related to energy in that the work done by a force on an object results in a change in the object's energy. This relationship is particularly evident in the work-energy theorem, which states that the net work done on an object is equal to the change in its kinetic energy.
What are the units used for measuring work?
-The units for work are joules (J), which are derived from the product of the units of force (newtons, N) and distance (meters, m), resulting in newton meters.
How does the angle between the force vector and the displacement vector affect the work done?
-The angle between the force vector and the displacement vector affects the work done through the component of the force that is in the direction of the displacement. Work is calculated as the product of the force component in the direction of displacement (F*cos(theta)) and the displacement (s).
Why is the work done by a force perpendicular to the direction of motion considered zero?
-The work done by a force perpendicular to the direction of motion is considered zero because the cosine of a 90-degree angle (theta = 90°) is zero, and since work is calculated as F*cos(theta)*s, it results in no work being done when the force is applied at a right angle to the displacement.
What is the work-energy theorem?
-The work-energy theorem states that the net work done on an object is equal to the change in its kinetic energy, which can be mathematically expressed as W = ΔKE = (1/2)mvf^2 - (1/2)mvi^2, where W is work, KE is kinetic energy, m is mass, vf is final velocity, and vi is initial velocity.
How is gravitational potential energy related to work done by gravity?
-Gravitational potential energy (PE) is the energy an object possesses due to its position relative to a reference level, typically the Earth's surface. The work done by gravity on an object is equal to the change in gravitational potential energy, expressed as W = ΔPE = mghf - mghi, where m is mass, g is the acceleration due to gravity, and hf and hi are the final and initial heights, respectively.
What is the principle of conservation of mechanical energy?
-The principle of conservation of mechanical energy states that in the absence of non-conservative forces (like friction), the total mechanical energy of a system (the sum of kinetic and potential energy) remains constant throughout the motion of the object.
How is power defined and what unit is used to measure it?
-Power is defined as the rate at which work is done, calculated by dividing the work by the time it takes to perform that work. Power is measured in watts (W), where 1 watt is equivalent to 1 joule per second.
What is the difference between conservative and non-conservative forces?
-Conservative forces, such as gravity and elastic spring force, do work that depends only on the initial and final positions of an object, not the path taken. Non-conservative forces, like friction and air resistance, dissipate energy as heat and do not satisfy this property, meaning they can cause a loss of mechanical energy in a system.
Outlines
🔧 Physics of Work and Energy
The lecture introduces the concept of work in physics, distinguishing it from everyday understanding. It defines work as the product of the force applied to an object and the distance that object moves in the direction of the force, expressed as 'force times displacement'. The script uses the example of pushing a car to illustrate work and introduces the unit of measurement for work as joules, relating it to the concept of energy and the role of force in rotational motion.
📏 Directional Dependence in Work Calculation
This paragraph delves into the directional component of work, explaining how the angle between the force vector and the displacement vector affects the work done. It uses the example of a person pulling luggage at an angle to demonstrate how only the horizontal component of the force contributes to work. The summary also covers the implications of different angles, such as 0, 90, and 180 degrees, on the work done, and provides a numerical example with a force of 45 newtons, an angle of 50 degrees, and a displacement of 75 meters to calculate work.
🏋️♂️ Work and Kinetic Energy in Motion
The script discusses the relationship between work and kinetic energy, particularly in the context of motion. It explains how the work done by a constant net external force on an object is equivalent to the change in the object's kinetic energy. The paragraph provides a step-by-step derivation of the work-energy theorem, which states that work done on an object is equal to the difference between its final and initial kinetic energies, and illustrates this with an example involving an airplane and a force applied to it.
🚀 Gravitational Potential Energy and Work
This section introduces gravitational potential energy, explaining how it is the energy an object possesses due to its position relative to Earth, measured by height. The script discusses how work done by gravity is equal to the change in gravitational potential energy and uses the example of a basketball in free fall to illustrate this concept. It also explores the work done by gravity when an object is thrown upwards and how it relates to the object's kinetic energy.
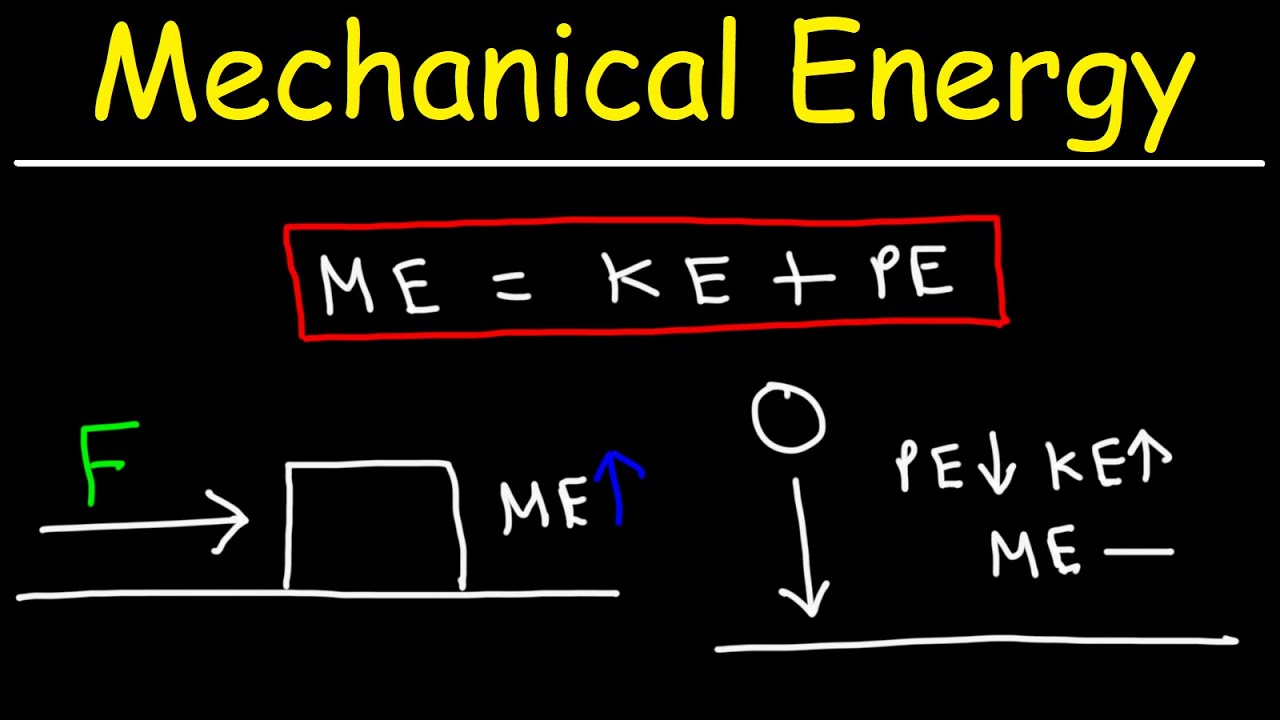
🤸♀️ Conservation of Mechanical Energy
The lecture explores the principle of conservation of mechanical energy, which states that the total mechanical energy of an object remains constant if no non-conservative forces, such as friction, are acting on it. It explains how mechanical energy is the sum of kinetic and potential energies and uses the example of a gymnast jumping on a trampoline to demonstrate how initial kinetic energy is converted into gravitational potential energy and back to kinetic energy.
🔄 Conservative and Non-Conservative Forces
This paragraph distinguishes between conservative and non-conservative forces. Conservative forces, such as gravity and elastic spring force, do work that depends only on the initial and final positions of an object, not the path taken. Non-conservative forces, like friction, result in energy loss, primarily as heat, and do not conserve work done on the object. The script provides examples of both types of forces and explains their implications on the work-energy principle.
⚡ Power and Efficiency in Work
The script concludes with a discussion on power, defined as the rate at which work is done, measured in watts. It emphasizes the importance of power in determining the efficiency of work processes, as it considers both the amount of work done and the time taken to do it. The paragraph provides formulas for calculating power based on work and velocity and includes examples of power expenditure in various human activities, such as running, skiing, biking, walking, and sleeping.
🌐 Universal Energy Conservation
The final paragraph summarizes the broader principle of energy conservation, applicable to the universe, stating that energy cannot be created or destroyed, only converted from one form to another. It provides examples of energy conversion, such as potential to kinetic energy in free fall, kinetic to thermal energy due to friction, and solar to electrical energy. The paragraph reinforces the idea that energy is always in balance, highlighting the fundamental nature of energy conservation.
Mindmap
Keywords
💡Work
💡Energy
💡Force
💡Displacement
💡Joules
💡Angle Dependence
💡Kinetic Energy
💡Gravitational Potential Energy
💡Conservative Forces
💡Non-Conservative Forces
💡Power
Highlights
Work in physics is defined as the product of the applied force and the displacement of an object in the direction of the force.
The concept of work in physics differs from everyday understanding, focusing on force and displacement calculations.
Work's unit is the newton meter, but joules are commonly used to align with energy units and avoid confusion with torque.
The angle between force and displacement affects the work done, with only the horizontal component of force contributing to work in non-parallel cases.
Work done is maximized when force and displacement vectors are parallel and minimized when perpendicular, with no work done in the latter case.
An example calculation demonstrates work done on a car being pushed, illustrating the formula's application.
Different measurement systems use different units for force, distance, and work, such as ergs in the CGS system and foot-pounds in the imperial system.
The work-energy theorem links the work done by a net external force to the change in an object's kinetic energy.
Kinetic energy depends on the mass of an object and the square of its velocity, indicating the importance of speed in energy calculations.
Gravitational potential energy is defined as the energy an object possesses due to its position relative to Earth, measured by height.
Conservative forces, such as gravity and elastic spring force, do work independent of the path taken between two points.
Non-conservative forces, like friction, result in energy loss and do not satisfy the definitions of conservative forces.
The work-energy theorem can be expanded to include both conservative and non-conservative forces, affecting an object's mechanical energy.
The principle of conservation of mechanical energy states that the total mechanical energy remains constant if no non-conservative forces do work.
Power is defined as the rate of work done, measured in watts, and indicates the efficiency of the work process over time.
The principle of conservation of energy applies to all forms of energy in the universe, stating that energy cannot be created or destroyed, only converted.
Examples of energy conversion include gravitational potential energy to kinetic energy in free fall and kinetic energy to thermal energy due to friction.
The lecture concludes with an overview of energy conservation and its universal application across different forms of energy.
Transcripts
Browse More Related Video

AP Physics C: Work, Energy, and Power Review (Mechanics)

8.01x - Lect 11 - Work, Kinetic & Potential Energy, Gravitation, Conservative Forces
Work, Energy, and Power - Basic Introduction
Conservative & Nonconservative Forces, Kinetic & Potential Energy, Mechanical Energy Conservation
Conservation of Energy (Learn to solve any problem)
Mechanical Energy - Basic Overview
5.0 / 5 (0 votes)
Thanks for rating: