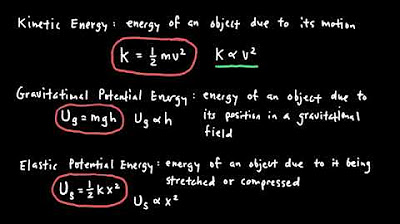College Physics 1: Lecture 28 - Work and Energy
TLDRIn this 28th lecture of College Physics 1, the focus is on work and energy. The lecturer introduces the concept of total energy in a system, explaining kinetic, gravitational potential, elastic potential, thermal, and chemical energy. The work-energy equation is discussed, emphasizing that work is the mechanical transfer of energy, quantified as force times displacement times the cosine of the angle between them, with units in joules. Examples illustrate work done in various scenarios, including the transformation of energy types. The lecture prepares students for understanding the conservation of energy in upcoming lectures.
Takeaways
- 📚 The lecture introduces the concepts of work and energy, setting the foundation for understanding energy conservation.
- 🔋 Energy is the capacity to do work and exists in various forms, including kinetic, gravitational potential, elastic potential, thermal, and chemical energy.
- 🏃 Kinetic energy is the energy of motion and is present in all moving objects, a common type of energy in physics problems.
- 🌐 Gravitational potential energy is the stored energy associated with an object's height above a surface, which can be transformed into kinetic energy.
- 🔄 Elastic potential energy is stored when a spring or elastic object is stretched or compressed, and it can be felt directly when manipulating such objects.
- 🔥 Thermal energy is the sum of all the kinetic and potential energies of molecules within an object, related to temperature and molecular motion.
- 🔁 Energy transformations are common and occur when one form of energy is turned into another, such as friction generating thermal energy during motion.
- ⚡ Work is the mechanical transfer of energy, involving a force causing a displacement, and is distinct from heat transfer, which is non-mechanical.
- 🔧 The work-energy equation (W = ΔE) states that the work done on a system results in a change in energy, with work being zero in an isolated system where no energy transfer occurs.
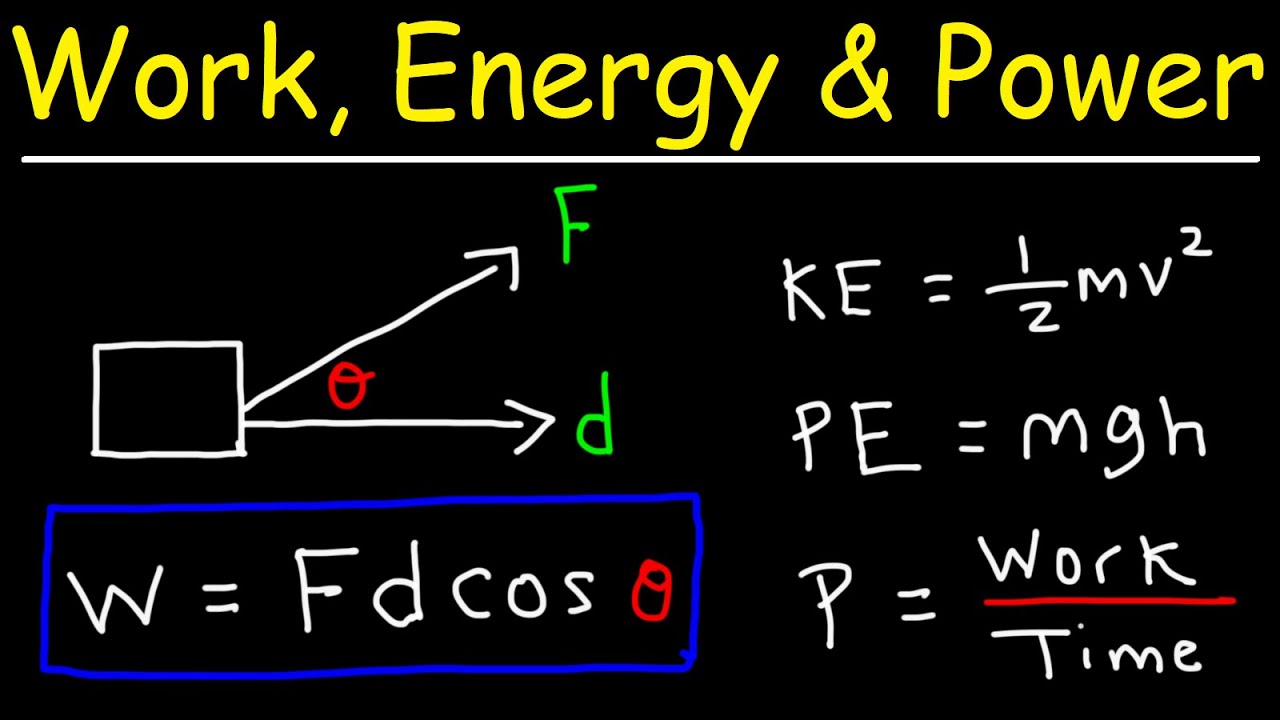
- ⚖️ Work is calculated using the equation W = Fd cos(Θ), where F is the force applied, d is the displacement, and Θ is the angle between force and displacement.
- ♻️ Negative work can occur when a force acts against the motion of an object, indicating that the object is slowing down or being decelerated.
Q & A
What are the two main topics introduced in Lecture 28 of College Physics 1?
-The two main topics introduced in Lecture 28 are work and energy.
What is the primary goal of the lecture on work and energy?
-The primary goal of the lecture is to set the foundation and get students familiarized with the basics of work and energy before discussing the conservation of energy in Lecture 29.
What does the term 'total energy' represent in the context of a system of interacting objects?
-In the context of a system of interacting objects, 'total energy' represents the sum of all different types of energy present in the system, denoted by the capital letter E.
What is kinetic energy and why is it relevant in physics?
-Kinetic energy, denoted by the capital letter K, is the energy of motion. It is relevant in physics because all moving objects possess this type of energy, and it is a common factor in many physics problems.
Can you explain gravitational potential energy and its relation to an object's height above a surface?
-Gravitational potential energy, denoted by U with the subscript G, is the stored energy associated with an object's height above a surface. It represents the energy that is stored due to an object being lifted off the ground or moving up a hill and can be transformed into kinetic energy when the object is released.
What is elastic potential energy and how is it related to springs or elastic objects?
-Elastic potential energy, denoted by U with the subscript S, is the energy stored when a spring or elastic object is stretched or compressed. It is directly related to the spring nature of the object and is waiting to be used, as can be felt when stretching or compressing a spring.
What is thermal energy and how does it relate to the temperature of an object?
-Thermal energy, denoted by E with the subscript TH, is the sum of all the kinetic and potential energies of molecules within an object. It is related to the temperature of an object in that the hotter an object is, the faster its molecules or atoms are moving, resulting in more kinetic energy and thus more thermal energy.
What is the difference between energy transformation and energy transfer?
-Energy transformation is a change of energy within a system, turning one type of energy into another. Energy transfer, on the other hand, is the exchange of energy between a system and its environment, typically occurring via work or heat.
How is work defined in the context of physics and what is its unit?
-In physics, work is defined as the mechanical transfer of energy due to a force. It is directly proportional to the force applied, the displacement of the object, and the cosine of the angle between the force and displacement. The unit of work is a Newton meter, which is also known as a joule (J).
What is the significance of the angle in the work equation (W = F * D * cos(Theta))?
-The angle in the work equation (Theta) is significant because it determines the component of the force that contributes to the displacement of the object. If the force is in the same direction as the displacement (0 degrees), the work done is at its maximum. If the force is perpendicular to the displacement (90 degrees), no work is done. If the force is in the opposite direction of the displacement (180 degrees), negative work is done, indicating work against the motion.
Can you provide an example of how work is calculated in a practical scenario?
-An example provided in the script is calculating the work done to pull a suitcase through an airport on an inclined surface. Given a displacement of 120 meters, a tension force of 20 newtons, and an angle of 45 degrees, the work done (W) is calculated as W = T * D * cos(Theta), which equals approximately 1700 joules.
What is the relationship between the work done and the change in energy of a system?
-The relationship between the work done and the change in energy of a system is given by the work-energy equation, which states that the work done (W) on a system is equal to the change in energy (ΔE) of that system. This means that when work is done on a system, it results in a change in the system's energy.
How does the mass of an object affect the work done on it when pushed with the same force over the same distance?
-The mass of an object does not directly affect the amount of work done on it when pushed with the same force over the same distance, as work is calculated by the formula W = F * D * cos(Theta). However, the mass of the object does affect its acceleration and final kinetic energy after the force is applied, as per Newton's second law of motion.
What is the difference between positive and negative work in the context of physics?
-Positive work is done when the force applied to an object is in the same direction as its displacement, contributing to the object's motion. Negative work occurs when the force is applied in the opposite direction of the object's motion, acting against the motion and effectively slowing the object down or reducing its energy.
Outlines
📚 Introduction to Work and Energy Concepts
The lecture begins by introducing the topic of work and energy, emphasizing the importance of understanding these concepts as fundamental to physics. The instructor outlines the goal of the lecture, which is to familiarize students with the basics of work and energy, setting the stage for the discussion on the conservation of energy in the subsequent lecture. The concept of total energy in a system of interacting objects is introduced, along with the idea that energy exists in various forms, such as kinetic, gravitational potential, elastic potential, and thermal energy. The lecture aims to clarify what energy is and how it operates within different systems.
🔋 Exploring Different Types of Energy
This paragraph delves deeper into the different types of energy, starting with kinetic energy, which is associated with the motion of objects. Gravitational potential energy is explained as the stored energy related to an object's height above a surface. Elastic potential energy, often linked with springs, is the energy stored when an elastic object is stretched or compressed. Thermal energy is introduced as the sum of all kinetic and potential energies of molecules within an object, which is related to temperature. Lastly, chemical energy, although not a focus of the course, is mentioned as the stored energy in molecular bonds released during chemical reactions.
⚙️ Energy Transformations and Real-World Examples
The lecture continues by discussing energy transformations, which are the processes where one form of energy is converted into another within a system. Examples provided include a baseball player sliding to a base, where kinetic energy is transformed into thermal energy due to friction, a chemical reaction in wood combustion releasing thermal energy, and a diver on a springboard, illustrating the conversion of elastic potential energy to kinetic energy and then to gravitational potential energy. These examples serve to highlight the omnipresence of energy transformations in everyday life.
🔄 Understanding Energy Transfers and Work
The distinction between energy transformations and energy transfers is clarified, with the latter involving the exchange of energy between a system and its environment. Two primary mechanisms for energy transfer are identified: work and heat. Work is defined as the mechanical transfer of energy, where an external force causes an object to move. The lecture provides examples of work being done, such as throwing a shot put, striking a match, and using a slingshot, emphasizing the physical aspect of work.
📐 The Work-Energy Equation and Its Implications
The work-energy equation is introduced, stating that the work done on a system results in a change in energy. The equation W = Δe is established, where W represents work and Δe represents the change in energy. The concept of an isolated system, where no work is done and energy is conserved, is explained. The importance of understanding work mathematically is highlighted, leading to a discussion on how work can be quantified through an equation involving force, displacement, and the angle between them.
📉 Negative Work and Its Conceptual Significance
This section addresses the possibility of negative work, which occurs when the force applied is in the opposite direction of the object's displacement. Examples are given to illustrate how work can be positive, negative, or zero, depending on the angle between the force and displacement. The importance of understanding the directionality of force and displacement when calculating work is emphasized, with the clarification that negative work does not mean no work is being done, but rather work is being done against the motion of the object.
🧳 Applying the Work Formula in a Practical Scenario
A practical example is worked through to demonstrate the application of the work formula in calculating the amount of work done in pulling a suitcase on an inclined surface. The example uses the formula W = T * D * cos(Theta), where T is the tension force, D is the displacement, and Theta is the angle between the force and displacement. The calculation shows that approximately 1700 joules of work are done in the scenario, with a brief discussion on where this energy goes, mainly into thermal energy due to friction.
🔄 Work Done by Different Forces and Energy Transformations
The lecture concludes with a series of questions that reinforce the concepts of work and energy transformations. Questions cover scenarios such as a child on a swing, where gravitational potential energy is converted to kinetic energy, and a crane lowering a girder, where the work done by gravity and tension is analyzed. The discussion clarifies that work can be positive or negative depending on the direction of the force relative to the displacement, and that energy transformations are inherent in these processes.
🚀 Conclusion and Preview of Conservation of Energy
The lecture wraps up with a brief overview of what will be covered in the next lecture, which is the conservation of energy. The instructor reminds students that they will reintroduce the concepts of kinetic, gravitational potential, elastic potential, and thermal energy, defining each with an equation. The goal is to integrate all the information discussed to understand and apply the principle of energy conservation in various physical systems.
Mindmap
Keywords
💡Work
💡Energy
💡Kinetic Energy
💡Gravitational Potential Energy
💡Elastic Potential Energy
💡Thermal Energy
💡Chemical Energy
💡Energy Transformation
💡Energy Transfer
💡Conservation of Energy
Highlights
Introduction to the concepts of work and energy in physics.
The goal of the lecture is to familiarize students with the basics of work and energy before discussing energy conservation.
Total energy of a system is the sum of all different energies present, represented by E.
Kinetic energy (K) is the energy of motion and is inherent in all moving objects.
Gravitational potential energy (Ug) is the stored energy associated with an object's height above a surface.
Elastic potential energy (Us) is stored when a spring or elastic object is stretched or compressed.
Thermal energy (Eth) is the sum of all kinetic and potential energies of molecules within an object.
Chemical energy (Echem) is the stored energy in molecular bonds, released during chemical reactions.
Energy transformations involve changes of energy within a system, such as friction generating thermal energy.
Energy transfers occur between a system and its environment, either through work or heat.
Work is the mechanical transfer of energy, defined as force times displacement times cosine of the angle between them.
Heat is the non-mechanical transfer of energy due to temperature differences.
The work-energy equation states that work done (W) equals the change in energy (Δe).
In an isolated system where no work is done, energy is conserved.
Work can be mathematically defined and its unit is the joule (J), derived from Newton meters.
Work can be negative, indicating that it is acting against the motion of an object.
Example calculation of work done in pulling a suitcase on an inclined surface.
Discussion on energy transformations, such as a child on a swing converting gravitational potential energy to kinetic energy.
Analysis of work done by different forces, such as gravity and tension, on a lowering girder.
Comparison of kinetic energy for a light plastic cart versus a heavy steel cart after being pushed with the same force.
Conclusion summarizing the lecture's coverage of work, energy, and their mathematical definitions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: