AP Physics C: Work, Energy, and Power Review (Mechanics)
TLDRThis video script offers a comprehensive review of work, energy, and power within the AP Physics C Mechanics curriculum. It explains the equation for work done by a constant force, using dot product or force times displacement times cosine of the angle. The example provided illustrates the calculation of work with given force and displacement vectors. The script delves into concepts like Hooke's Law for spring force, elastic potential energy, and the work-energy theorem. It also covers kinetic and gravitational potential energy, the principle of energy conservation, and power as the rate of work done. The importance of identifying initial and final points, and the distinction between conservative and non-conservative forces like friction, are highlighted. The script concludes with a discussion on equilibrium states and their stability.
Takeaways
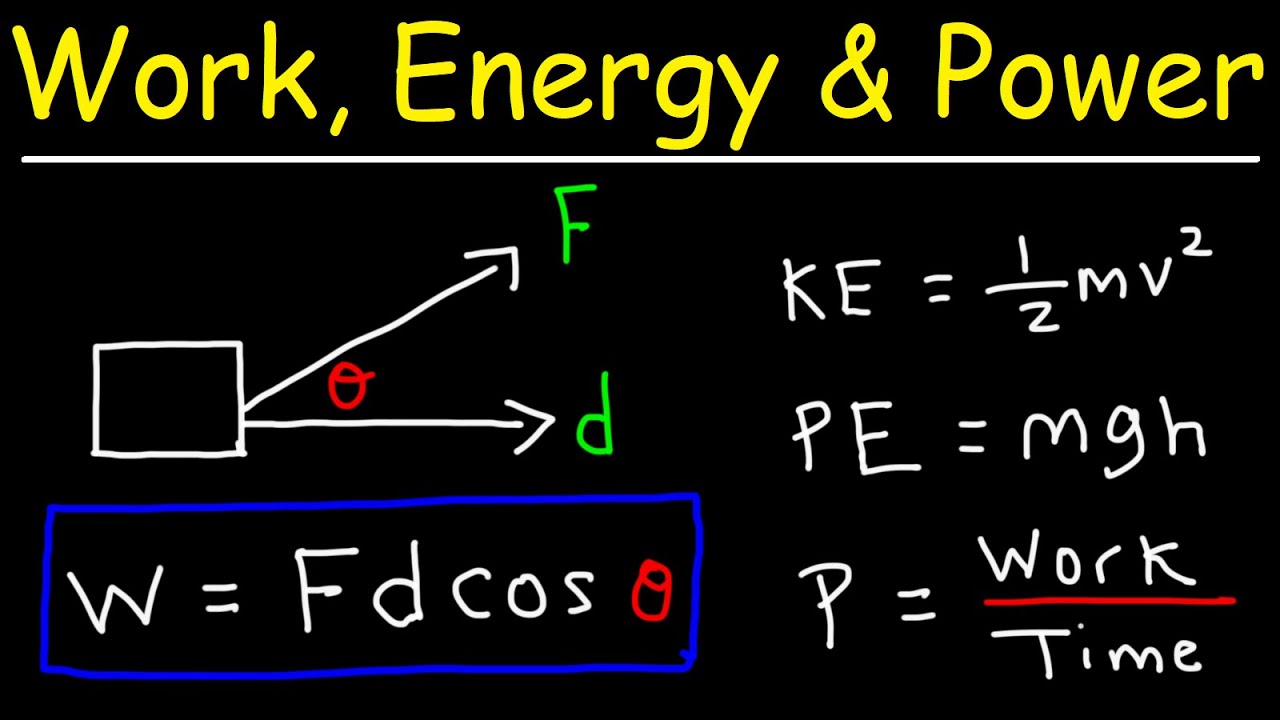
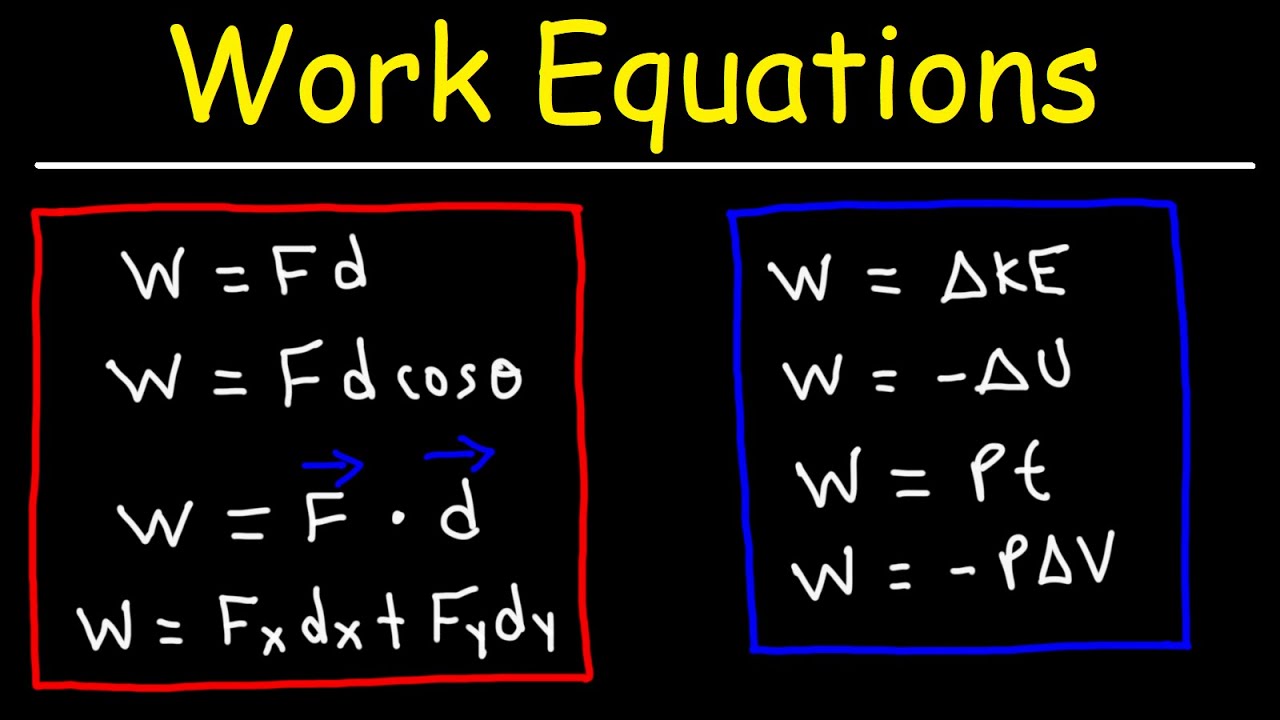
- 🔧 The equation for work done by a constant force is the dot product of the force and displacement vectors, or force times displacement times the cosine of the angle between them.
- 📏 Work is a scalar quantity, and the units for work are joules (J), which is equivalent to newton-meters (N·m).
- 📚 An example calculation shows that the work done is 12.42 joules when a force of 2.7i - 3.1j N acts on an object with a displacement of 4.6i m.
- ∫ For non-constant forces, work is calculated using a definite integral of the force with respect to position from the initial to the final position.
- 💡 The integral represents the area under the curve, distinguishing between positive and negative areas relative to the horizontal axis.
- 🔗 Hooke's Law states that the force exerted by a spring is proportional to its displacement from the equilibrium position, with the equation F = -kx.
- 🔄 The work done by a spring is the integral of the spring force with respect to position, resulting in the change in elastic potential energy.
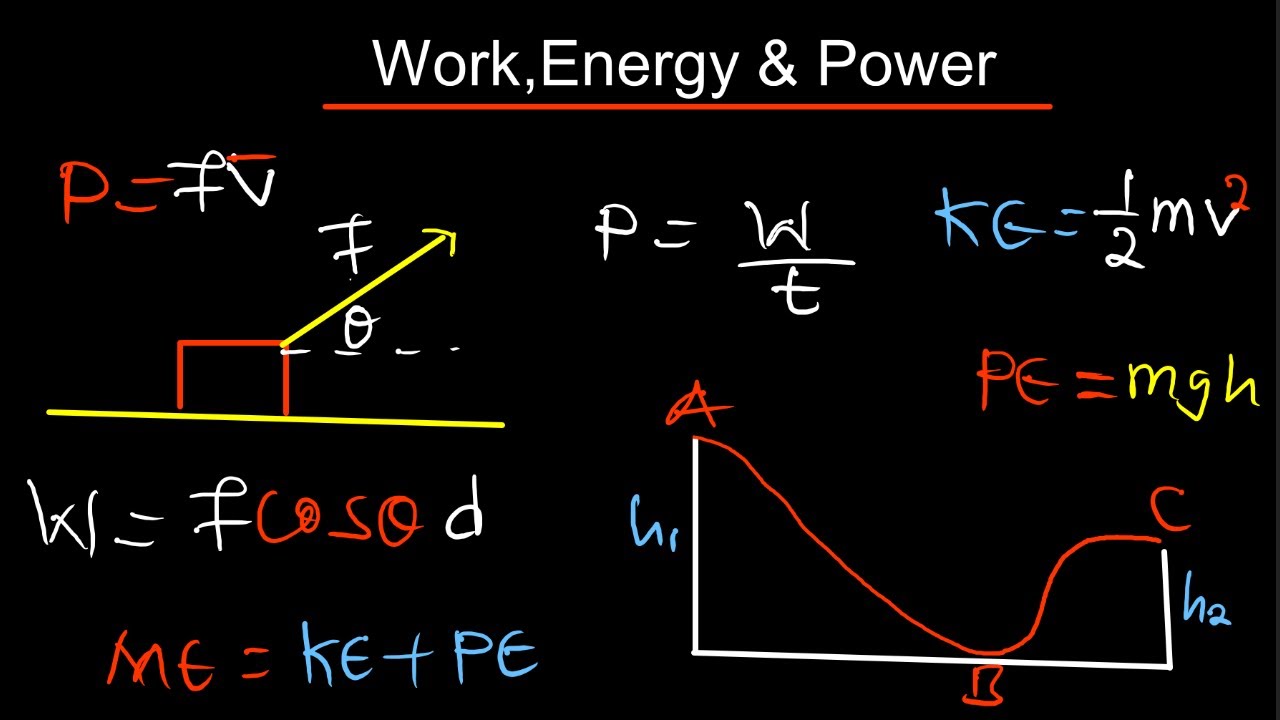
- 🚀 Kinetic energy (KE) is defined as \( \frac{1}{2} \) times the mass times the velocity squared, and gravitational potential energy (U) is mass times gravity times height above the reference level.
- ⚖️ The principle of conservation of energy states that in an isolated system, the total energy remains constant, with energy transformations but not creations or destructions.
- 🔄 The work-energy principle links the work done by non-conservative forces, like friction, to the change in mechanical energy of a system.
- ⏱️ Power is the rate at which work is done, with average power calculated as work over time and instantaneous power as the derivative of work with respect to time.
- 🔄 The relationship between work and power can be expressed through integration and differentiation, where the area under a power-time graph equals the change in work.
Q & A
What is the equation for work done by a constant force?
-The work done by a constant force is given by the dot product of the force doing the work and the displacement of the object, which can also be expressed as force times displacement times the cosine of the angle between the force and the displacement vectors.
Can you provide an example calculation of work done by a constant force?
-An example given in the script is where a force of 2.7i - 3.1j newtons acts on an object that has a displacement of 4.6i meters. The work done is calculated as the dot product of these two vectors, resulting in 2.7 * 4.6 + (-3.1 * 0) = 12.42 joules.
What is a scalar in physics?
-A scalar is a quantity that has magnitude but no direction, unlike a vector which has both magnitude and direction. Work is an example of a scalar quantity.
How is the work done by a force that is not constant calculated?
-For a force that is not constant, the work done is calculated using a definite integral from the initial to the final position of the force with respect to position.
What is Hooke's Law and how is it represented mathematically?
-Hooke's Law states that the force exerted by a spring is directly proportional to the displacement from its equilibrium position and is represented as F = -kx, where k is the spring constant and x is the displacement.
What are the units for the spring constant and how do they relate to the units of work?
-The units for the spring constant are newtons per meter (N/m). Since work is measured in joules (J), and a joule is equivalent to a newton meter (N*m), the spring constant's units are consistent with the units of work.
How does the work done by a spring relate to elastic potential energy?
-The work done by a spring is equal to the negative change in elastic potential energy. This means that when a spring does work, the elastic potential energy of the spring decreases.
What is the net work kinetic energy theorem and where can it be found?
-The net work kinetic energy theorem is a principle that relates the net work done on an object to its change in kinetic energy. The script suggests reviewing the derivation of this theorem through a provided link.
What are the units for power and how are they related to other units?
-The SI unit for power is the watt (W), which is equivalent to joules per second (J/s). An English unit for power is the horsepower (hp), with 746 watts equaling one horsepower.
How is power related to work and time?
-Power is the rate at which work is done, so average power is calculated as the work done divided by the change in time. Instantaneous power is the derivative of work with respect to time.
What is the relationship between conservative forces and potential energy?
-A conservative force is equal to the negative derivative of the potential energy associated with that force. This relationship is key to understanding how conservative forces like gravity and springs work.
Can you explain the concept of equilibrium and its types using the script's examples?
-Equilibrium can be neutral, stable, or unstable. A ball in neutral equilibrium has constant gravitational potential energy regardless of position. A water bottle in stable equilibrium has increasing gravitational potential energy as it moves away from equilibrium, tending to return to the equilibrium position. A dry erase marker in unstable equilibrium has decreasing gravitational potential energy as it moves away from equilibrium, tending to move further away.
Outlines
📚 Work, Energy, and Power in AP Physics C Mechanics
This paragraph introduces the topic of work, energy, and power from the AP Physics C Mechanics curriculum. It explains the equation for work done by a constant force, which is the dot product of the force and displacement vectors or force times displacement times the cosine of the angle between them. An example is provided to calculate the work done with given force and displacement vectors, resulting in 12.42 joules. The concept of a joule as a unit of energy is also discussed, defined as a newton-meter. The paragraph further explains that work done by a non-constant force is calculated using a definite integral from the initial to the final position. Hooke's Law for the force exerted by a spring is introduced, along with the spring constant (k) and displacement (x), and the work done by a spring is related to the change in elastic potential energy.
🔄 Understanding Energy Transfers and Conservation in Physics
The second paragraph delves into the concept of energy transfers and conservation. It discusses the net work kinetic energy theorem and introduces the equations for kinetic energy and gravitational potential energy. The importance of identifying a horizontal zero line when calculating gravitational potential energy is emphasized. The paragraph explains that in a non-isolated system, the change in energy equals the sum of energy transferred into or out of the system, while in an isolated system, the change in energy must be zero. The work done by non-conservative forces, such as friction, is equated to the change in mechanical energy of the system. The distinction between net work and work due to non-conservative forces is highlighted, with the latter being applicable only in isolated systems without energy transfer.
⚡ Power and Its Relation to Work and Energy
This paragraph focuses on power, defined as the rate at which work is done. It differentiates between average power, calculated as work over time, and instantaneous power, which is the derivative of work with respect to time. The relationship between power and energy is explored, with power being the derivative of energy with respect to time. The paragraph also discusses the SI unit for power, which is the watt, and clarifies that while the derivative of work with respect to time can be represented as the dot product of force and velocity for a constant force, the integral form shows that the area under a power-time graph equals the change in work. Additionally, a lesser-known equation relating conservative forces to the negative derivative of potential energy is introduced, with examples provided for spring force and gravity.
🔍 Equilibrium Types and Their Relation to Potential Energy
The final paragraph discusses the concepts of neutral, stable, and unstable equilibrium in relation to gravitational potential energy. It uses examples of a ball, a water bottle, and a dry erase marker to illustrate how the potential energy changes in each case. A ball in neutral equilibrium has constant potential energy regardless of position, a water bottle in stable equilibrium has increasing potential energy as it moves away from equilibrium, indicating a natural return to the equilibrium position, and a dry erase marker in unstable equilibrium has decreasing potential energy as it moves away, indicating a natural movement away from equilibrium. The paragraph concludes the review of work, energy, and power, and invites viewers to explore further topics in physics.
Mindmap
Keywords
💡Work
💡Dot Product
💡Joule
💡Hooke's Law
💡Elastic Potential Energy
💡Conservative Force
💡Nonconservative Force
💡Power
💡Watt
💡Integral
💡Equilibrium
Highlights
Work done by a constant force is calculated using the dot product of the force and displacement vectors.
Work is a scalar quantity, and its unit is the joule, which is equivalent to a newton-meter.
The dot product method for calculating work emphasizes the component of force in the direction of displacement.
For a non-constant force, work is calculated using a definite integral from the initial to final position.
An integral represents the area under the curve, distinguishing between positive and negative areas.
Hooke's Law states that the force of a spring is proportional to its displacement from the equilibrium position, with the spring constant (k) and displacement (x) involved.
The work done by a spring is given by the integral of the spring force with respect to position.
The work-energy theorem links the net work done on an object to its change in kinetic energy.
Kinetic energy is defined as half the mass times the velocity squared.
Gravitational potential energy is calculated as the mass times gravitational acceleration times height above the reference level.
Conservation of energy principle states that energy cannot be created or destroyed, only transferred.
In an isolated system, the total mechanical energy remains constant, assuming no non-conservative forces like friction.
Power is the rate at which work is done, with average power calculated as work done over time and instantaneous power as the derivative of work with respect to time.
The SI unit for power is the watt, with 746 watts equivalent to one horsepower.
The relationship between derivatives and integrals is highlighted, where every derivative can be rearranged as an integral and vice versa.
A conservative force is defined as the negative derivative of the potential energy associated with that force.
Equilibrium states—neutral, stable, and unstable—are explained in terms of changes in gravitational potential energy.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: