Calculating average velocity or speed | One-dimensional motion | Physics | Khan Academy

Khan Academy
11 Jun 201111:45
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concepts of vectors and scalars, using a real-world example of Shantanu's car journey to explain average velocity and speed. It highlights the difference between vector quantities, which include direction (like velocity), and scalar quantities, which do not (like distance). The script then calculates Shantanu's average velocity in kilometers per hour and further converts it to meters per second, demonstrating unit conversion and dimensional analysis. The example provided is a simple application of these concepts in physics and everyday life.

Takeaways
  • 📐 Vectors and scalars are fundamental concepts in physics, with vectors including both magnitude and direction, while scalars only include magnitude.
  • 🚗 Shantanu's example of traveling 5 kilometers north in 1 hour is used to illustrate the calculation of average velocity, which is a vector quantity.
  • 🔄 The average velocity is calculated by dividing the displacement (change in position including direction) by the change in time.
  • 🎯 The velocity in Shantanu's case is 5 kilometers per hour to the north, indicating both speed and direction.
  • 📌 The distinction between velocity (vector) and speed (scalar) is crucial; velocity requires direction for its definition, while speed does not.
  • 🌐 To convert units from kilometers per hour to meters per second, one must perform a unit conversion that takes into account the number of meters in a kilometer and the number of seconds in an hour.
  • 🔢 The conversion process involves multiplying the distance by the number of meters per kilometer and then dividing by the number of seconds per hour.
  • 🧮 Dimensional analysis is a technique used to convert units, which can be particularly useful for more complex unit conversions.
  • 🤔 An intuitive understanding of the conversion process can be aided by recognizing that a smaller unit of time (seconds) will result in a smaller unit of distance when converting from a larger unit of distance traveled per larger unit of time (hours).
  • 📊 After performing the conversion, Shantanu's velocity is found to be approximately 1.39 meters per second, indicating a slow travel speed.
  • 📖 Understanding the difference between vector and scalar quantities, as well as how to calculate and interpret average velocity, is essential for solving common physics problems and everyday scenarios.
Q & A

  • What is the main difference between a vector and a scalar quantity?

    -A vector quantity has both magnitude and direction, and is typically represented with an arrow to indicate its direction. A scalar quantity, on the other hand, only has magnitude and no direction.

  • What is the definition of velocity?

    -Velocity is a vector quantity that represents the rate of change of an object's position, including both the distance and the direction of the movement.

  • How do you calculate average velocity?

    -Average velocity is calculated by dividing the total displacement (change in position) by the total time taken.

  • What is the difference between displacement and distance?

    -Displacement is a vector quantity that includes both the magnitude and direction of an object's movement, while distance is a scalar quantity that only measures the magnitude of how far an object has traveled, without considering direction.

  • What is the definition of speed?

    -Speed is a scalar quantity that refers to the rate at which an object covers distance, without regard to its direction of travel.

  • How did Shantanu travel according to the script?

    -Shantanu traveled 5 kilometers north in 1 hour.

  • What was Shantanu's average velocity during his travel?

    -Shantanu's average velocity was 5 kilometers per hour to the north.

  • What was Shantanu's average speed?

    -Shantanu's average speed was 5 kilometers per hour.

  • How can you convert kilometers per hour to meters per second?

    -To convert kilometers per hour to meters per second, first convert kilometers to meters by multiplying by 1000, then convert hours to seconds by dividing by 3600.

  • What was Shantanu's speed in meters per second?

    -Shantanu's speed was approximately 1.39 meters per second.

  • Why is it important to specify direction when discussing velocity?

    -Specifying direction is important when discussing velocity because it distinguishes it from speed, which only considers magnitude. Velocity, being a vector quantity, takes into account both the magnitude and direction of movement.

  • How does the concept of 'average' come into play when discussing velocity?

    -The concept of 'average' is used when discussing velocity to account for the possibility that an object's velocity might change over time. The average velocity provides a single value that represents the overall velocity over a period of time, assuming a constant velocity.

Outlines
00:00
📚 Introduction to Vectors and Scalars

This paragraph introduces the concepts of vectors and scalars, using Shantanu's travel as an example to explain the difference between the two. It discusses how to calculate average velocity, which is a vector quantity that includes both magnitude and direction, and contrasts it with speed, which is a scalar quantity focusing only on the magnitude of movement. The explanation also touches on the importance of including direction when dealing with vectors and how to represent them with arrows.

05:01
📈 Calculating Average Velocity

In this paragraph, the process of calculating Shantanu's average velocity is detailed. It explains how to convert the given distance and time into velocity by dividing the distance (5 kilometers) by the time (1 hour), resulting in a velocity of 5 kilometers per hour to the north. The paragraph emphasizes the need to specify direction for vector quantities and how omitting it results in a scalar quantity, in this case, speed. It also highlights the difference between kilometers per hour and meters per second, setting the stage for unit conversion in the next paragraph.

10:06
🔄 Unit Conversion: Kilometers per Hour to Meters per Second

This paragraph delves into the unit conversion from kilometers per hour to meters per second. It explains the step-by-step process of converting Shantanu's speed by first changing kilometers to meters and then hours to seconds. The explanation uses the concept of dimensional analysis to illustrate how the units cancel out and how the conversion factors are applied. The paragraph concludes with the calculation that Shantanu's speed is approximately 1.39 meters per second, providing insight into the practical application of unit conversion in physics problems.

Mindmap
Keywords
💡Vectors
Vectors are quantities that have both magnitude and direction. In the context of the video, Shantanu's displacement of 5 kilometers north is an example of a vector because it specifies the distance traveled and the direction of travel. The video emphasizes the importance of the direction in vector quantities, which is indicated by the use of arrows in their representation.
💡Scalars
Scalars are quantities that have magnitude but no direction. The video contrasts scalars with vectors, using distance as an example of a scalar quantity. Distance only concerns how far an object has traveled, without considering the direction of travel. This is illustrated in the video by discussing Shantanu's travel, where the distance of 5 kilometers is a scalar because it does not include directional information.
💡Average Velocity
Average velocity is defined as the displacement of an object divided by the time interval during which the displacement occurred. It is a vector quantity because it includes both the magnitude of the displacement and its direction. In the video, Shantanu's average velocity is calculated by dividing the 5 kilometers he traveled north by the 1 hour it took, resulting in a velocity of 5 kilometers per hour to the north.
💡Displacement
Displacement is the change in position of an object, which includes both the distance traveled and the direction of that travel. It is a vector quantity because it conveys information about the starting and ending points of the motion. In the video, Shantanu's displacement is described as 5 kilometers to the north, which is used to calculate his average velocity.
💡Speed
Speed is the rate at which an object covers distance, without considering the direction of travel. It is a scalar quantity because it only concerns the magnitude of how far an object has moved. In the video, the concept of speed is discussed in relation to Shantanu's travel, where his average speed is the same as his average velocity since it is assumed that he traveled at a constant rate and in a straight line.
💡Rate
Rate, often used interchangeably with speed, refers to the magnitude of how fast something is happening per unit of time. It is a scalar quantity that does not account for direction. In the context of the video, if one were only interested in the magnitude of Shantanu's travel without the direction, they would consider his rate, which is 5 kilometers per hour.
💡Unit Conversion
Unit conversion is the process of changing the units of a physical quantity from one system to another while maintaining the value of that quantity. In the video, unit conversion is demonstrated by changing Shantanu's velocity from kilometers per hour to meters per second, which requires multiplying by the conversion factor of meters per kilometer and dividing by the number of seconds in an hour.
💡Dimensional Analysis
Dimensional analysis is a method used to convert units and solve problems in physics and engineering by canceling out unwanted units. It involves multiplying or dividing quantities with different units to achieve the desired unit. In the video, dimensional analysis is used to convert Shantanu's velocity from kilometers per hour to meters per second, by setting up the conversion so that the unwanted units (kilometers and hours) cancel out, leaving the desired units (meters and seconds).
💡Physics
Physics is the natural science that studies matter, its motion, and the forces that act upon it. The video discusses concepts from physics, such as vectors, scalars, velocity, and displacement, which are fundamental to understanding the behavior of objects in motion. These concepts are applied to a real-world scenario involving Shantanu's travel to illustrate how physics principles can be used to analyze everyday situations.
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. The video briefly mentions calculus when discussing the use of the letter 'S' for displacement instead of 'D', which is commonly used for the derivative operator in calculus to avoid confusion. Although calculus is not the main focus of the video, its mention indicates the broader mathematical context in which physics concepts are often applied.
Highlights

The discussion begins with an application of vectors and scalars in common physics and everyday problems.

Shantanu's travel example is used to illustrate the concept of average velocity.

A review of vectors and scalars is provided, differentiating them by their representation and the inclusion of direction in vectors.

Displacement is introduced as a vector quantity, combining both magnitude and direction.

Velocity is defined as the change in position, including direction, making it a vector quantity.

The distinction between velocity (vector) and speed (scalar) is clarified, emphasizing the importance of direction in velocity.

The calculation of Shantanu's average velocity is detailed, using the given displacement and time.

The concept of average velocity is explored, noting that it assumes a constant velocity over time.

A method for converting units from kilometers per hour to meters per second is explained through dimensional analysis.

The conversion process involves multiplying the distance by the number of meters in a kilometer and dividing by the number of seconds in an hour.

The final calculation shows that Shantanu's car was traveling at a speed of 1.39 meters per second.

The transcript highlights the practical application of physics concepts in understanding and calculating real-world motion.

The importance of including direction when dealing with vector quantities is reiterated.

The transcript provides an intuitive understanding of how unit conversion works in physics problems.

The use of the delta symbol (∆) is explained as a way to denote 'change in' within mathematical expressions.

The transcript emphasizes the importance of unit consistency when performing calculations.

The concept of rate is introduced as another term for speed, focusing on scalar quantities.

The transcript explains why 'S' is used for displacement instead of 'D', which is reserved for the derivative operator in calculus.

An intuitive gut check is suggested as a way to estimate the outcome of unit conversions.

Transcripts

Browse More Related Video

Speed and Velocity (Tagalog)

Solving for time | One-dimensional motion | Physics | Khan Academy

Velocity and speed: Motion in One Dimension

GCSE Physics - The difference between Speed and Velocity & Distance and Displacement #51

AP Physics B Kinematics Presentation #40

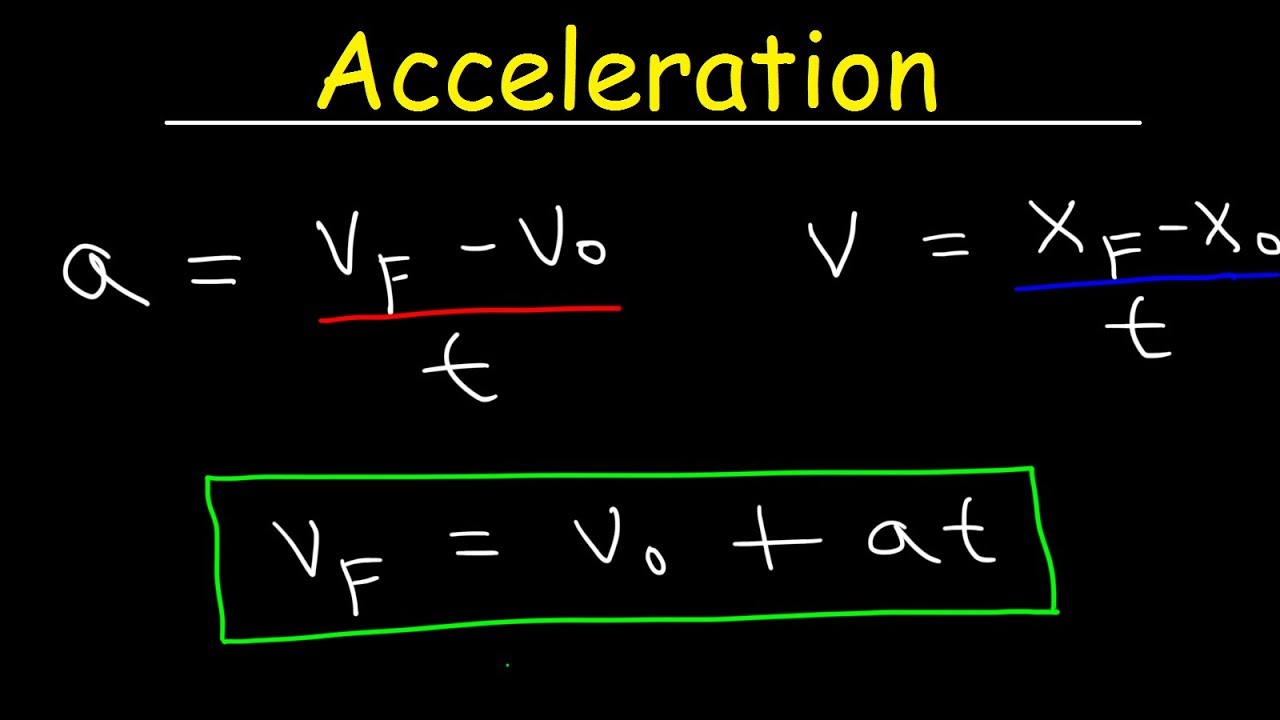
Physics - Acceleration & Velocity - One Dimensional Motion

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics ConceptsVectors vs ScalarsAverage VelocitySpeed CalculationDirectional AnalysisPhysics Problems Everyday ApplicationsUnit ConversionMeters per SecondKilometers per Hour