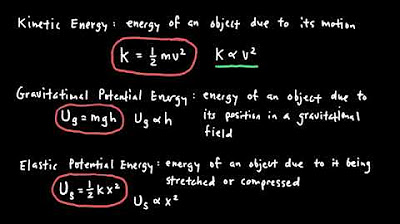AP Physics 1: Work, Energy and Power Review

Flipping Physics
13 Mar 201508:56
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging lesson, the key concepts of work, energy, power, and Hooke's law are reviewed for the AP Physics 1 exam. The discussion begins with the fundamental principle that work done on a system results in an energy change, delving into the work equation and the significance of the force's angle with displacement. The session covers the three types of mechanical energy: kinetic, elastic potential, and gravitational potential energy, emphasizing their equations and the conditions for their conservation. The lesson also explains the concept of power as the rate of doing work and introduces Hooke's law, highlighting the linear relationship between a spring's force and displacement from its equilibrium position. The summary concludes with a reminder of the importance of identifying initial and final points, the horizontal zero line, and the forces involved in work calculations.

Takeaways
  • 📚 The relationship between work, energy, and the system is fundamental in physics, with work leading to a change in a system's energy.
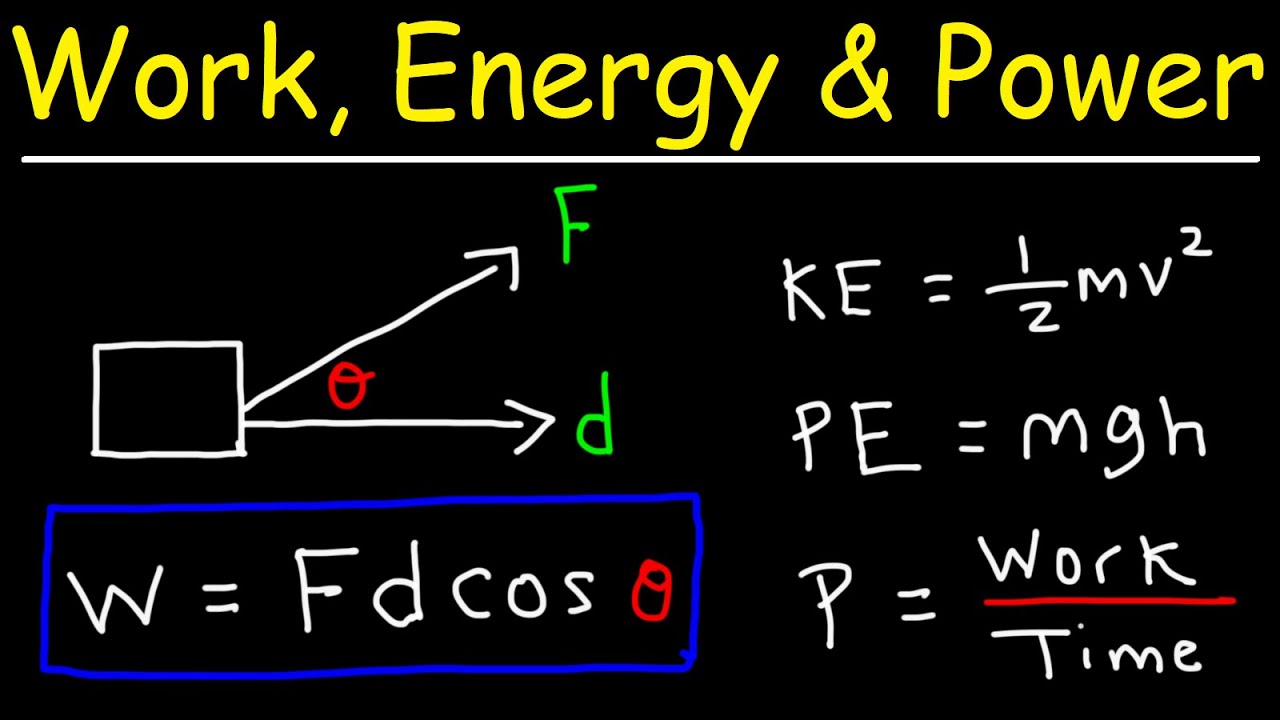
  • 🔧 The work done on a system is equal to the force applied times the displacement of the object times the cosine of the angle (theta) between the force and displacement directions.
  • ⚖️ Work is a scalar quantity with units of joules (N·m), and the joule is defined as a Newton meter (N·m).
  • 🏃 Kinetic energy (KE) is the energy of motion, calculated as (1/2) mass times the velocity squared, and it cannot be negative.
  • 🌟 Elastic potential energy is stored in springs or stretchy materials and is given by (1/2) times the spring constant times the displacement squared from the equilibrium position.
  • 🌐 Gravitational potential energy is associated with an object's position in a gravitational field and is calculated as mass times gravitational acceleration times height above a reference level.
  • 🔄 Mechanical energy is conserved in the absence of non-conservative forces, such as friction, which can convert energy into heat, light, or sound.
  • 🚀 To apply the conservation of mechanical energy, identify initial and final points, the horizontal zero line, and the types of mechanical energy present.
  • 💡 Power is the rate at which work is done, measured in watts (joules per second), and can be related to velocity through the work equation.
  • 📈 Hooke's Law states that the force exerted by a spring is proportional to its displacement from the equilibrium position, with the spring constant being the proportionality factor.
  • 🔍 For problems involving friction, use the work equation to account for the work done against friction, which is the force of friction times displacement times the cosine of 180 degrees.
Q & A

  • What is the fundamental principle that connects work and energy in physics?

    -The fundamental principle is that the change in energy of a system is equal to the work done on the system. This means that when work is performed on an object or a group of objects (the system), it results in a change in the system's energy.

  • How is work defined mathematically in the context of force and displacement?

    -Work is defined mathematically as the force doing work on an object times the displacement of the object times the cosine of the angle (theta) between the direction of the force and the direction of displacement. The formula is given by W = F * d * cos(theta), where W is work, F is the force, d is the displacement, and theta is the angle.

  • What are the dimensions of work and how are they related to the unit of energy, the joule?

    -The dimensions of work are the same as those of energy, which are mass times distance squared divided by time squared (kg * m^2/s^2). This corresponds to the unit of energy, the joule (J), which is also equivalent to a Newton meter (N*m). A joule is a unit of energy that measures the work done when a force of one Newton moves an object through a distance of one meter in the direction of the force.

  • What is kinetic energy and how is it calculated?

    -Kinetic energy is the energy of motion. It is calculated using the formula KE = 0.5 * m * v^2, where KE is the kinetic energy, m is the mass of the object, and v is its velocity. Since mass and velocity are squared in the equation, kinetic energy can never be negative.

  • What is elastic potential energy and how does it relate to a spring's displacement from equilibrium?

    -Elastic potential energy is the energy stored in a spring or an elastic material when it is stretched or compressed. It is calculated using the formula PE_e = 0.5 * k * x^2, where PE_e is the elastic potential energy, k is the spring constant, and x is the displacement from the equilibrium position. Like kinetic energy, elastic potential energy is also never negative because the spring constant and the square of the displacement are always positive.

  • How is gravitational potential energy defined and under what conditions can it be negative?

    -Gravitational potential energy is the energy associated with an object's position in a gravitational field. It is defined as PE_g = m * g * h, where PE_g is the gravitational potential energy, m is the mass of the object, g is the acceleration due to gravity, and h is the vertical height above a chosen horizontal reference line (zero line). Gravitational potential energy can be negative if the object is located below the zero line, making the vertical height negative.

  • What is the conservation of mechanical energy principle and when can it be applied?

    -The conservation of mechanical energy principle states that the total mechanical energy (the sum of kinetic and potential energies) of an isolated system remains constant if only conservative forces, such as gravity and elastic forces, are acting on it. This principle can be applied when there are no non-conservative forces like friction present, which means no energy is being converted into heat, light, or sound.

  • How is power defined and related to work and time?

    -Power is defined as the rate at which work is done or the rate at which energy is transferred. It is calculated using the formula P = dE/dt, where P is power, dE is the change in energy, and dt is the change in time. Power can also be expressed in terms of force, displacement, and velocity as P = F * v * cos(theta), where F is the force, v is the velocity, and theta is the angle between the force and velocity vectors.

  • What are the dimensions of power and how are they related to the unit of power, the watt?

    -The dimensions of power are energy per unit time, which is equivalent to joules per second (J/s). The unit of power is the watt (W), which is equal to one joule per second. A common reference is that 746 watts is equivalent to one horsepower.

  • What is Hooke's Law and how does it relate to the force exerted by a spring?

    -Hooke's Law states that the force exerted by a spring is linearly proportional to the displacement from its equilibrium position. The force is given by F = -k * x, where F is the force, k is the spring constant, and x is the displacement from the equilibrium position. The negative sign indicates that the force exerted by the spring is always in the opposite direction of the displacement, attempting to restore the spring to its equilibrium position.

  • How can the spring constant be determined from a graph of force versus displacement?

    -The spring constant can be determined from a graph of force versus displacement by calculating the slope of the best-fit line through the data points. The spring constant (k) is the ratio of the force (F) to the displacement (x), so the slope of the line on the graph represents the spring constant (k = -F/x).

Outlines
00:00
📚 Introduction to Work, Energy, Power, and Hooke's Law

This paragraph introduces the key concepts of work, energy, power, and Hooke's law, which are essential topics for the AP Physics 1 exam. The discussion begins with the relationship between the change in energy of a system and the work done on it, emphasizing that work results in an energy change. The work equation is explored, highlighting the importance of considering the force's direction relative to the object's displacement. The conversation then shifts to the three types of mechanical energy: kinetic, elastic potential, and gravitational potential energy, each defined by its respective equation and characteristics. The paragraph concludes with a discussion on the dimensions of work and energy, establishing that they are measured in joules.

05:02
🌐 Conservation of Mechanical Energy and Power

This paragraph delves into the principle of conservation of mechanical energy, explaining when and how it can be applied, specifically in the absence of non-conservative forces like friction. The process of identifying initial and final points, as well as the horizontal zero line, is crucial for using the conservation equation. The paragraph also addresses scenarios where energy is converted to heat, light, or sound, and how to account for this using the work equation. The concept of power, defined as the rate of doing work, is introduced, with an emphasis on the relationship between power, force, and velocity. The dimensions of power are discussed, and Hooke's law is explained, detailing the linear relationship between a spring's force and its displacement from the equilibrium position.

Mindmap
Keywords
💡Work
Work in physics is defined as the product of the force applied to an object and the displacement of the object in the direction of the force. It is measured in joules (J) and is central to the concept of energy transfer. In the context of the video, work is the process that leads to a change in a system's energy, such as when a force pushes or pulls an object causing it to move. The work equation is given by W = F * d * cos(theta), where W is work, F is the force, d is the displacement, and theta is the angle between the force and the displacement vector.
💡Energy
Energy is a fundamental concept in physics that describes the ability to do work. It exists in various forms, such as kinetic, potential, and thermal energy. In the video, mechanical energy is the focus, which includes kinetic energy (energy of motion), elastic potential energy (energy stored in a deformed material like a spring), and gravitational potential energy (energy due to an object's position in a gravitational field). The conservation of mechanical energy is a principle stating that the total mechanical energy in an isolated system remains constant in the absence of non-conservative forces like friction.
💡Power
Power is the rate at which work is done or energy is transferred over time. It is a measure of how quickly work is performed. In the video, power is related to the time taken to do work on a system and is calculated using the work equation with a focus on the time component. Power is measured in watts (W), where 1 watt equals 1 joule per second (1 W = 1 J/s).
💡Hooke's Law
Hooke's Law describes the linear relationship between the force exerted by a spring and the displacement from its equilibrium position. The law states that the force (F) exerted by a spring is proportional to the displacement (x) and is represented by the equation F = -k * x, where k is the spring constant and the negative sign indicates that the force exerted by the spring is always opposite in direction to the displacement. This law is fundamental to understanding the behavior of springs and other elastic materials.
💡Joule
A joule (J) is the unit of measurement for work, energy, and heat in the International System of Units (SI). It is defined as the work done when a force of one newton displaces an object by one meter in the direction of the force. In the context of the video, joules are used to quantify both work and energy, with the relationship between them being central to the discussion of mechanical energy conservation.
💡Kinetic Energy
Kinetic energy is the energy that an object possesses due to its motion. It depends on the object's mass and the square of its velocity. The formula for kinetic energy is KE = 1/2 * m * v^2, where m is the mass of the object and v is its velocity. Kinetic energy is always a positive quantity because mass and the square of velocity are always positive.
💡Elastic Potential Energy
Elastic potential energy is the energy stored in an elastic object, such as a spring or a rubber band, when it is stretched or compressed. It is related to the deformation of the material from its equilibrium position and is given by the formula PE_e = 1/2 * k * x^2, where k is the spring constant and x is the displacement from the equilibrium position. Like kinetic energy, elastic potential energy is always positive because the spring constant and the square of displacement are always positive.
💡Gravitational Potential Energy
Gravitational potential energy is the energy an object possesses due to its position in a gravitational field, typically related to its height above a reference point. It is calculated using the formula PE_g = m * g * h, where m is the mass of the object, g is the acceleration due to gravity, and h is the height above the reference point. Unlike kinetic and elastic potential energy, gravitational potential energy can be negative if the object is below the reference point, indicating its position relative to the chosen zero level.
💡Cosine
The cosine is a trigonometric function that relates the angle of a right triangle to the ratio of the adjacent side length to the hypotenuse. In the context of physics, particularly in the calculation of work and power, the cosine is used to determine the component of force that is in the direction of displacement or velocity. The value of cosine ranges from -1 to 1, where 1 corresponds to a force or displacement direction perfectly aligned with the positive axis, -1 to perfectly opposite, and 0 to a direction perpendicular to the axis.
💡Spring Constant
The spring constant, denoted by k, is a measure of the stiffness of a spring. It is defined as the force required to cause a unit displacement of the spring from its equilibrium position. The unit of spring constant is newtons per meter (N/m). A higher spring constant indicates a stiffer spring, meaning it takes more force to cause the same displacement.
💡Conservation of Mechanical Energy
The principle of conservation of mechanical energy states that in a closed system with no external forces or non-conservative forces like friction, the total amount of mechanical energy (sum of kinetic and potential energies) remains constant. This principle is fundamental in solving problems where energy transformations occur without energy loss.
Highlights

Review of key topics for AP Physics 1 exam: work, energy, power, and Hooke's law.

The change in energy of a system equals the work done on the system.

Work is defined as the force doing work times the displacement of the object times the cosine of the angle theta.

Work causes a change in energy of a system, with the equation relating work to force, displacement, and angle.

The force parallel to displacement is calculated as the force times the cosine of theta.

Identify the force used in the work equation and remember to use the magnitude for both force and displacement.

Dimensions for work are in joules, and a joule is defined as a Newton times a meter.

Kinetic energy is the energy of motion and is calculated as (1/2) times mass times the velocity squared.

Elastic potential energy is stored in a spring or elastic material and is given by (1/2) times the spring constant times the displacement squared.

Gravitational potential energy is associated with a gravitational field and is calculated as mass times gravity times height.

Mechanical energy is conserved in the absence of non-conservative forces, such as friction.

To use conservation of mechanical energy, identify initial and final points and the types of mechanical energy present.

When energy is converted to heat, light, or sound via friction, use the work equation to account for the change in mechanical energy.

Power is the rate at which work is done and is calculated based on the time taken to change the system's energy.

The equation for power in terms of velocity is derived from the work equation, considering the force and angle.

Hooke's Law states that the force of a spring is linearly proportional to the displacement from the equilibrium position.

The spring constant is measured in force per unit length, or Newtons per meter.

The review lesson provides a comprehensive overview of work, energy, and power for AP Physics 1 exam preparation.

Transcripts

Browse More Related Video

AP Physics C: Work, Energy, and Power Review (Mechanics)

Work, Energy, and Power - Basic Introduction

College Physics 1: Lecture 28 - Work and Energy

Work and Energy

A Level Physics Revision: All of Work, Energy and Power (in 18 minutes)

AP Physics 1 Energy Review

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationAPReviewMechanicalEnergyHookesLawWorkAndEnergyPowerCalculationElasticPotentialEnergyGravitationalPotentialEnergyFrictionalWorkConservationOfEnergy