Average Acceleration and Instantaneous Acceleration
TLDRThis educational video script delves into the concepts of average acceleration and instantaneous acceleration, fundamental aspects of physics concerning the rate of velocity change. It elucidates how acceleration is determined by the change in velocity over time, highlighting scenarios of positive, zero, and negative acceleration, along with centripetal acceleration during direction changes at constant speed. The script methodically explains the formulas for calculating average and instantaneous acceleration, demonstrates their application through practical examples, and illustrates how to interpret these accelerations graphically using velocity-time graphs. Additionally, it integrates practice problems to reinforce understanding, including calculus applications for precise instantaneous acceleration calculations.
Takeaways
- 📈 Acceleration is the rate of change of velocity, and it can be positive, negative, or zero, reflecting increasing, decreasing, or constant velocity respectively.
- 🔄 When an object changes direction at a constant speed, it experiences centripetal acceleration, which is directed towards the center of its circular path.
- 🚀 Average acceleration is calculated as the change in velocity divided by the change in time (Δv/Δt).
- ⌛ Instantaneous acceleration is the acceleration at a specific instant, found using limits as Δt approaches zero in the Δv/Δt formula.
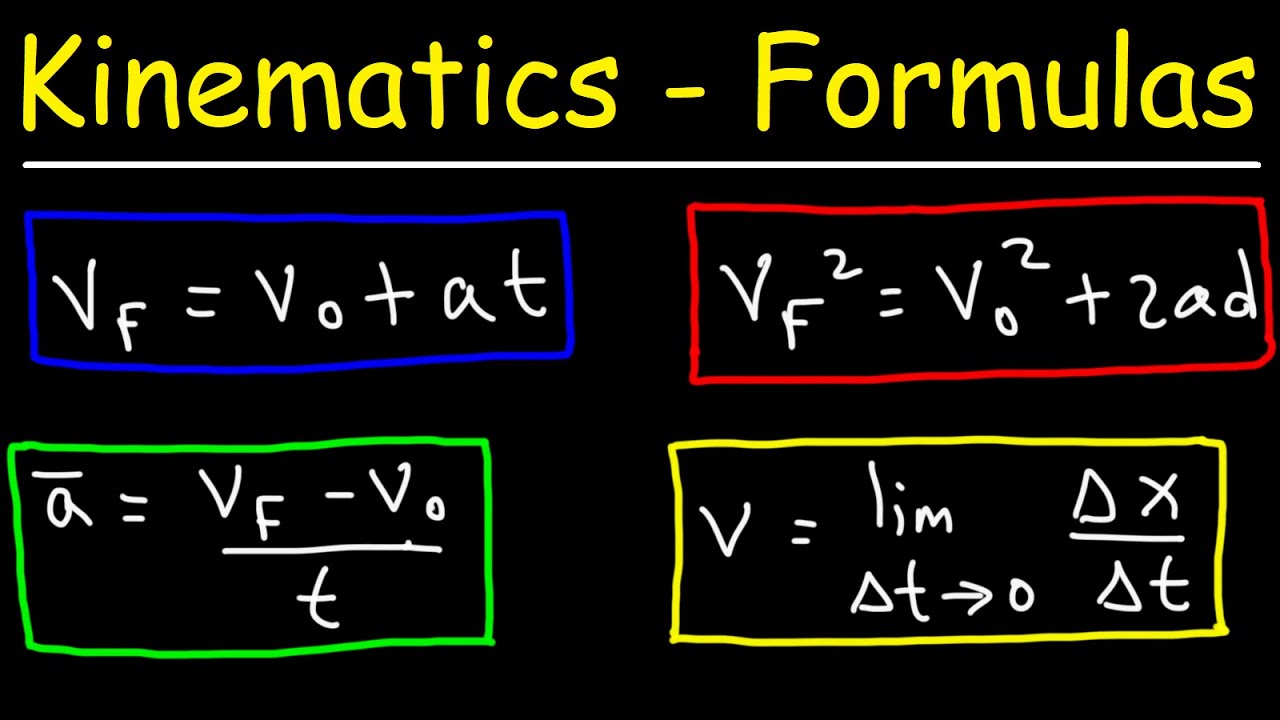
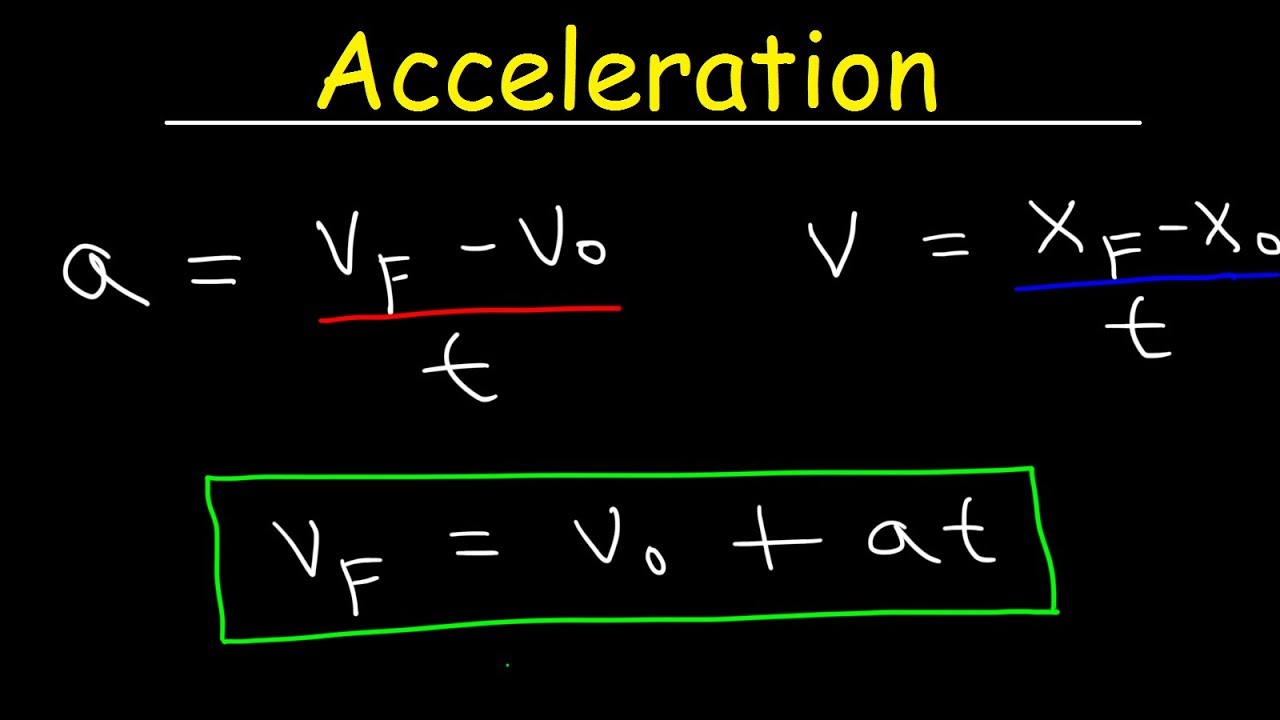
- 📚 Two key formulas for average acceleration in kinematics are: 'v_final = v_initial + at' and 'v_final^2 = v_initial^2 + 2ad'.
- 📉 The acceleration can be visualized on a velocity-time graph, where the slope of a secant line between two points gives the average acceleration, and the slope of a tangent line at a point gives the instantaneous acceleration.
- 🧪 To calculate average acceleration from experimental data, one can use the change in velocity over the change in time based on specific instances or measurements.
- 🎢 For objects undergoing uniform circular motion, the change in direction implies a change in velocity and thus an acceleration, even if the speed is constant.
- 🔢 Example problems illustrate how to apply these concepts to calculate the average acceleration from given velocities and times, or distances, and the instantaneous acceleration from a velocity function.
- 🔍 The instantaneous acceleration can also be estimated from average accelerations over very small time intervals, but the exact value requires calculus, specifically taking the derivative of the velocity function.
Q & A
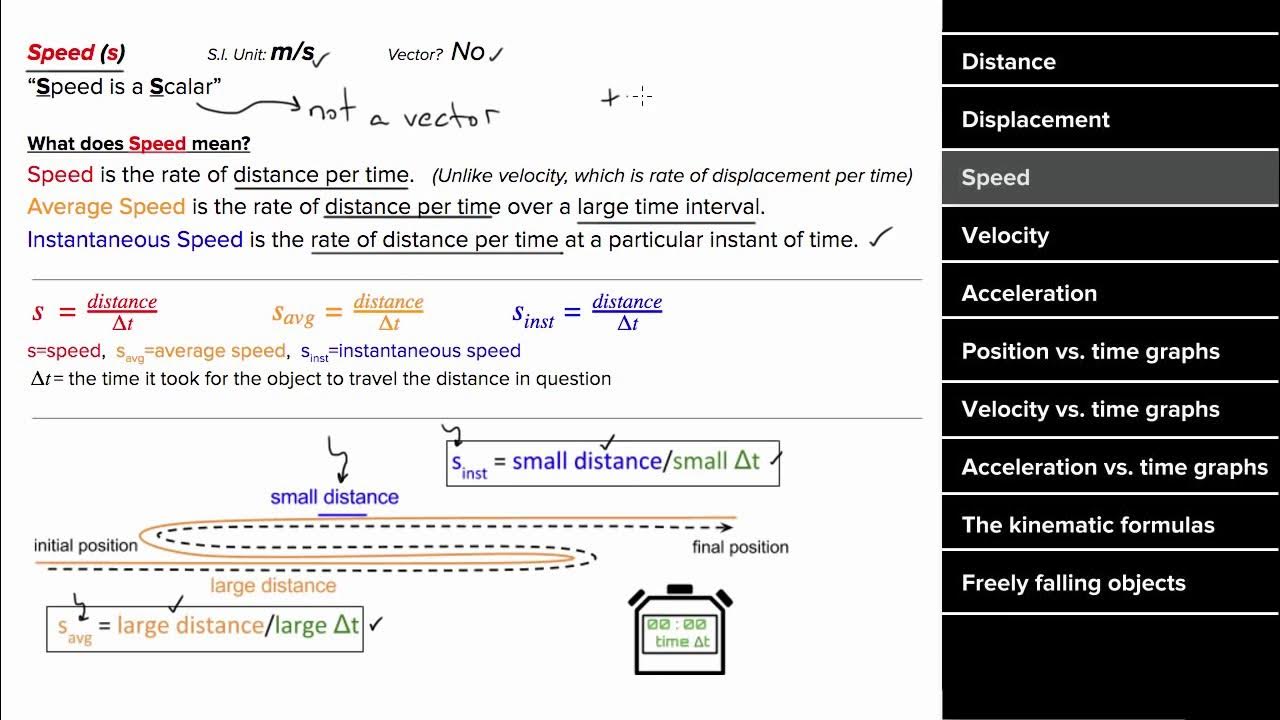
What is acceleration?
-Acceleration is the rate at which velocity is changing.
What does it mean if acceleration is positive?
-If acceleration is positive, it means that the velocity is increasing.
What is the acceleration when the change in velocity is zero?
-When the change in velocity is zero, the velocity is constant and the acceleration will be zero.
What happens to acceleration when velocity decreases?
-When velocity decreases, the change in velocity is negative, which means acceleration is negative.
How is acceleration affected when an object changes direction at a constant speed?
-When an object changes direction at constant speed, there is an acceleration known as centripetal acceleration, which points towards the center of the circle.
What is the formula for average acceleration?
-The formula for average acceleration is the change in velocity divided by the change in time, specifically equal to the final velocity minus the initial velocity, divided by delta t.
How can instantaneous acceleration be determined?
-Instantaneous acceleration can be found using limits, specifically as the limit of the change in velocity over the change in time as delta t approaches zero.
How is acceleration represented in a velocity-time graph?
-In a velocity-time graph, acceleration is represented by the slope. The slope of a secant line gives the average acceleration, while the slope of a tangent line gives the instantaneous acceleration.
What is the relationship between the slope of a tangent line and instantaneous acceleration in a velocity-time graph?
-The slope of the tangent line to the curve at a point represents the instantaneous acceleration at that point.
Can average acceleration formulas be used to approximate instantaneous acceleration?
-Yes, sometimes the formula for average acceleration can be used to approximate the instantaneous acceleration, especially when the time interval delta t becomes very small.
Outlines
🔍 Introduction to Acceleration
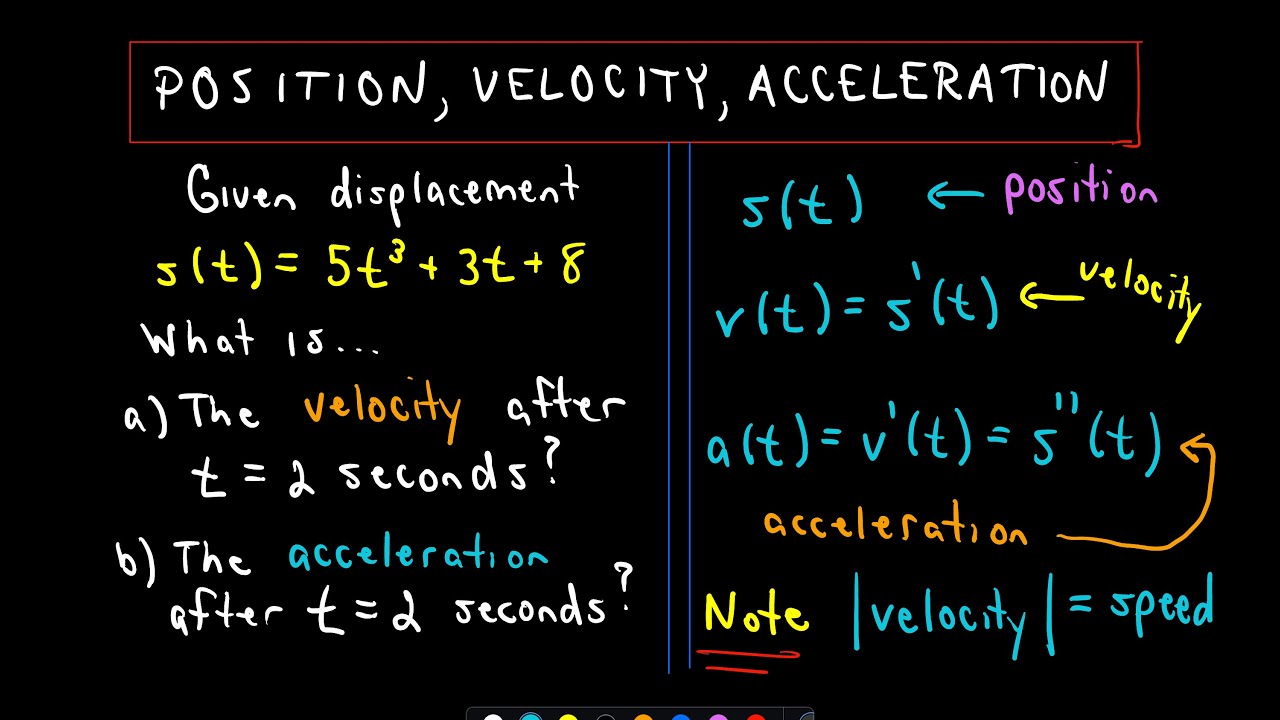
This segment introduces the concept of acceleration, explaining it as the rate of change in velocity. It covers the basics of positive, negative, and zero acceleration, and introduces the concept of centripetal acceleration which occurs when an object changes direction at a constant speed. The paragraph also differentiates between average acceleration, calculated as the change in velocity over the change in time, and instantaneous acceleration, which is determined at a specific instant and can be approached using limits.
📊 Understanding Velocity-Time Graphs
This paragraph delves into how to use velocity-time graphs to calculate average and instantaneous acceleration, explaining the concepts of secant and tangent lines. It also includes practice problems calculating average acceleration in different scenarios, such as a car and a bus accelerating over given distances or times, and introduces relevant formulas for these calculations.
🔢 Average and Instantaneous Acceleration Calculations
Focusing on a particle's motion, this segment provides a detailed walkthrough for calculating average acceleration between two time points using a given velocity function. It then explores estimating instantaneous acceleration through narrowing intervals around a specific time point, demonstrating the concept with calculations at t=3 seconds using closer intervals to approximate the instantaneous acceleration.
✅ Calculus Application in Acceleration
The final paragraph introduces the calculus method for finding instantaneous acceleration by differentiating the velocity function. It explains the differentiation process using the power rule and calculates the instantaneous acceleration of a particle at a specific time. This part emphasizes the importance of calculus in accurately determining instantaneous acceleration and corrects a unit error from earlier in the script.
Mindmap
Keywords
💡Acceleration
💡Velocity
💡Average Acceleration
💡Instantaneous Acceleration
💡Centripetal Acceleration
💡Kinematics Formulas
💡Velocity-Time Graph
💡Secant Line
💡Tangent Line
💡Derivative
Highlights
Acceleration is the rate at which velocity changes.
Positive acceleration indicates increasing velocity.
Zero acceleration means velocity is constant.
Negative acceleration shows decreasing velocity.
Changing direction at constant speed entails acceleration, known as centripetal acceleration.
Velocity includes both speed and direction; any change in these results in acceleration.
Average acceleration is calculated as the change in velocity over the change in time.
Instantaneous acceleration can be found using limits, specifically as delta t approaches zero.
Kinematic equations relate velocity, acceleration, time, and displacement.
Acceleration is the slope on a velocity-time graph.
The slope of a secant line on a velocity-time graph gives average acceleration.
The slope of a tangent line on a velocity-time graph indicates instantaneous acceleration.
Instantaneous acceleration equals the derivative of the velocity function.
Practice problems illustrate how to calculate average and instantaneous acceleration.
Calculus, specifically differentiation, is used to determine exact values of instantaneous acceleration.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: