Candidates Test with Definite Integrals
TLDRThe video script provides an insightful discussion on finding absolute maximums and minimums of a function on a closed interval using the candidate's test, which becomes particularly useful when dealing with definite integrals. The extreme value theorem is introduced, stating that every continuous function on a closed interval has an absolute maximum and minimum. The candidate's test is explained, highlighting that these extreme values occur at either endpoints or critical points. The script continues with examples and a step-by-step problem-solving approach, demonstrating how to find the absolute minimum of a function given its derivative and an initial condition. The process involves identifying critical points, setting up a table with endpoints and critical points as candidates, and calculating function values at these points. The video concludes with another example, emphasizing the importance of using a calculator for definite integrals and rounding to three decimal places for accuracy. The script is a valuable resource for those studying calculus, particularly in preparation for exams like the AP Calculus.
Takeaways
- 📚 The Extreme Value Theorem states that every continuous function on a closed interval has an absolute maximum and minimum value somewhere on the interval.
- 🔍 The Candidates Test is used to find the maximum or minimum values, which must occur at either an endpoint or a critical point of the function.
- 📈 The usefulness of the Candidates Test increases when combined with the ability to do anti-derivatives and definite integrals.
- 📊 To find the absolute minimum or maximum, one must evaluate the function at endpoints and critical points, then compare the results.
- 🤔 The process involves understanding the graph of the first derivative (f') to identify critical points where f'(x) = 0.
- 🧮 When calculating the definite integral for a function, switch the bounds and change the sign if the lower bound is less than the upper bound.
- ⚖️ For a function defined by an integral, the value of the function at a point is calculated by adding or subtracting the integral from the initial condition.
- 📐 The area under the curve of f'(x) can be used to determine the sign of the integral in the accumulation function.
- 📋 It's important to create a table listing all the potential candidates for the absolute maximum or minimum from the endpoints and critical points.
- 🔢 Using a calculator can assist in finding the exact values of the definite integrals for the given interval, which are then compared to find the absolute maximum or minimum.
- 📝 When presenting the answer, specify the value of the absolute maximum or minimum and the point at which it occurs, rounding to an appropriate number of decimal places for precision.
- 🎯 Practice is key for such problems, as they are common in calculus exams, such as the AP Calculus free response questions.
Q & A
What is the Extreme Value Theorem?
-The Extreme Value Theorem states that every continuous function on a closed interval has both an absolute maximum and an absolute minimum value somewhere on the interval.
What does the Candidates Test involve?
-The Candidates Test involves finding the maximum or minimum values of a function, which must occur at either an endpoint or at a critical point of the function.
Why is the Candidates Test more useful when you can do anti-derivatives and definite integrals?
-The Candidates Test becomes more useful with anti-derivatives and definite integrals because it allows for a more comprehensive analysis of the function's behavior, especially when dealing with accumulation functions and areas under curves.
What is meant by an accumulation function in the context of the video?
-In the context of the video, an accumulation function refers to the function f(x) expressed as a sum of an initial value (f(0) = 2 in the example) and the definite integral from 0 to x of the derivative of the function, f'(t) dt.
How do you find the critical points of a function from its derivative?
-To find the critical points of a function from its derivative, you set the derivative equal to zero and solve for x. These x-values are the critical points where the function could have a maximum or minimum.
What is the significance of the sign when switching bounds in a definite integral?
-When switching bounds in a definite integral from the usual order (lower bound to upper bound), the sign of the integral changes. If you're going to the left of the initial condition, you subtract the integral; if you're going to the right, you add it.
Why is it important to always have the lower bound less than the upper bound when calculating a definite integral?
-Having the lower bound less than the upper bound is important because it ensures the correct evaluation of the integral, which represents the signed area under the curve of the function between the two bounds.
How does the process of finding the absolute minimum or maximum of a function on a closed interval typically proceed?
-The process typically involves identifying the endpoints and critical points of the interval, setting up a table with these values, calculating the function values at each candidate point, and then determining the absolute minimum or maximum from the calculated values.
What is the role of a calculator in finding the absolute maximum or minimum of a function on a given interval?
-A calculator is used to compute the definite integrals and evaluate the function at the critical points and endpoints, which are necessary for determining the absolute maximum or minimum of the function on the interval.
Why is it essential to round the final answer to a consistent number of decimal places?
-Rounding to a consistent number of decimal places is essential for maintaining precision and avoiding loss of points in an academic or examination setting, ensuring that the answer is both accurate and uniformly presented.
What is the general approach to solving problems involving the absolute maximum or minimum of a function on a closed interval, as demonstrated in the video?
-The general approach involves understanding the Extreme Value Theorem, applying the Candidates Test to find potential maximum or minimum points, calculating the function values at these points, and then determining the absolute maximum or minimum from the calculated values, often with the aid of a calculator.
Outlines
📚 Finding Absolute Extrema Using the Candidates Test
This paragraph introduces the concept of finding absolute maximums and minimums on a closed interval using the Candidates Test. The Extreme Value Theorem is mentioned, which states that every continuous function on a closed interval has an absolute maximum and minimum. The Candidates Test is explained as a method to find these values, which can occur at either endpoints or critical points of the function. The paragraph also discusses the usefulness of the test when combined with anti-derivatives and definite integrals. Three examples are provided to illustrate the theorem, showing how the maximum and minimum can occur at different points depending on the function's behavior. A problem is then set up to apply the test, involving a function given by an integral and its derivative, with critical points identified from the derivative's graph.
🧮 Calculating Definitive Integrals for Absolute Minimum
The second paragraph delves into the process of calculating the absolute minimum of a function using definite integrals. It provides a step-by-step guide on how to set up and solve the problem, including switching bounds and changing signs when necessary. The process involves creating a table with endpoints and critical points as candidates for the absolute maximum or minimum. The integral is evaluated at each candidate point, and the results are used to determine the absolute minimum. The paragraph concludes with the solution to the problem, identifying the absolute minimum value and the point at which it occurs. It also touches on the importance of using a calculator for more complex integrals and emphasizes the need for precision and rounding to avoid losing points in an exam setting.
Mindmap
Keywords
💡Absolute Maximum and Minimum
💡Closed Interval
💡Continuous Function
💡Candidates' Test
💡Critical Points
💡Definite Integrals
💡Derivatives
💡Accumulation Function
💡Endpoint
💡Extreme Value Theorem
💡Relative Minimum
Highlights
The video discusses finding absolute maximums and minimums on a closed interval using the candidates test.
The candidates test becomes particularly useful when combined with knowledge of definite integrals.
The extreme value theorem states that every continuous function on a closed interval has an absolute maximum and minimum.
The maximum or minimum values of a function must occur at an endpoint or a critical point.
The function needs to be continuous on a closed interval, but it does not have to be differentiable.
Examples are provided to illustrate scenarios where the absolute maximum and minimum occur at endpoints or critical points.
The process for finding the absolute minimum of a function on a given interval is demonstrated using a specific problem.
The accumulation function is introduced as a method to find the absolute minimum or maximum.
Critical points are determined by setting the derivative of the function equal to zero.
A table is constructed with endpoints and critical points to evaluate the function's values at these points.
The concept of switching bounds and changing the sign of the integral when evaluating function values at points to the left of the initial condition is explained.
The importance of ensuring the lower bound is less than the upper bound when calculating definite integrals is emphasized.
The absolute minimum value and the corresponding x-value are identified from the table of function values.
Another problem is solved to find the absolute maximum of a function on an interval using the candidates test.
The use of a calculator for finding critical points and evaluating definite integrals is assumed to be known by the viewer.
The table for the second problem includes endpoints and critical points labeled as A and B to find the absolute maximum.
Definite integrals are calculated using a calculator, with the upper bound of integration being varied to find function values.
The absolute maximum value and the corresponding x-value are identified, and the answer is rounded to three decimal places.
The importance of rounding to maintain precision and avoid losing points in calculations is highlighted.
The video concludes by wishing viewers good luck, implying the usefulness of the method for exams like the AP Calculus.
Transcripts
Browse More Related Video

Calculus 1: Relative Extrema Examples

Calculus Review Maximum and Minimum Values of Functions

Finding Absolute Extrema (Max/Min) on a Closed Interval [a, b]

3.4 - Using Derivatives to Find Absolute Max and Min Values
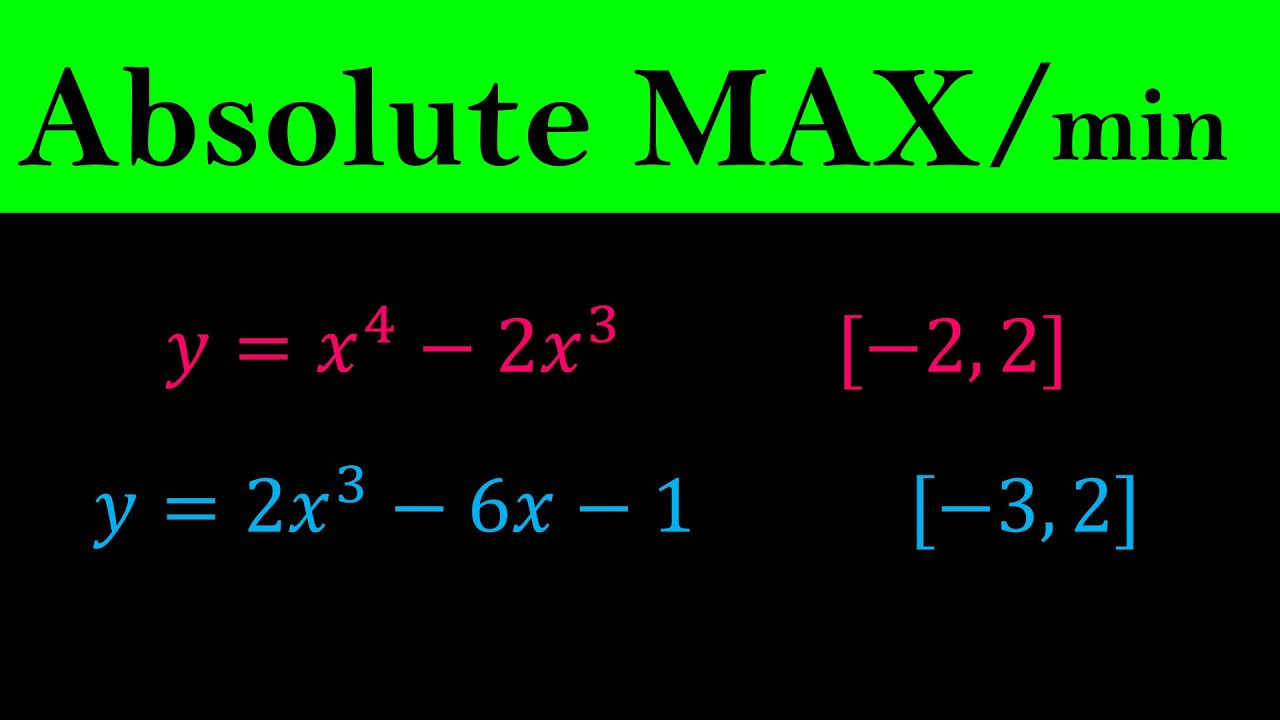
Absolute Maximum and Minimum Values - Finding absolute MAX & MIN of Functions - Calculus

Absolute Extrema
5.0 / 5 (0 votes)
Thanks for rating: