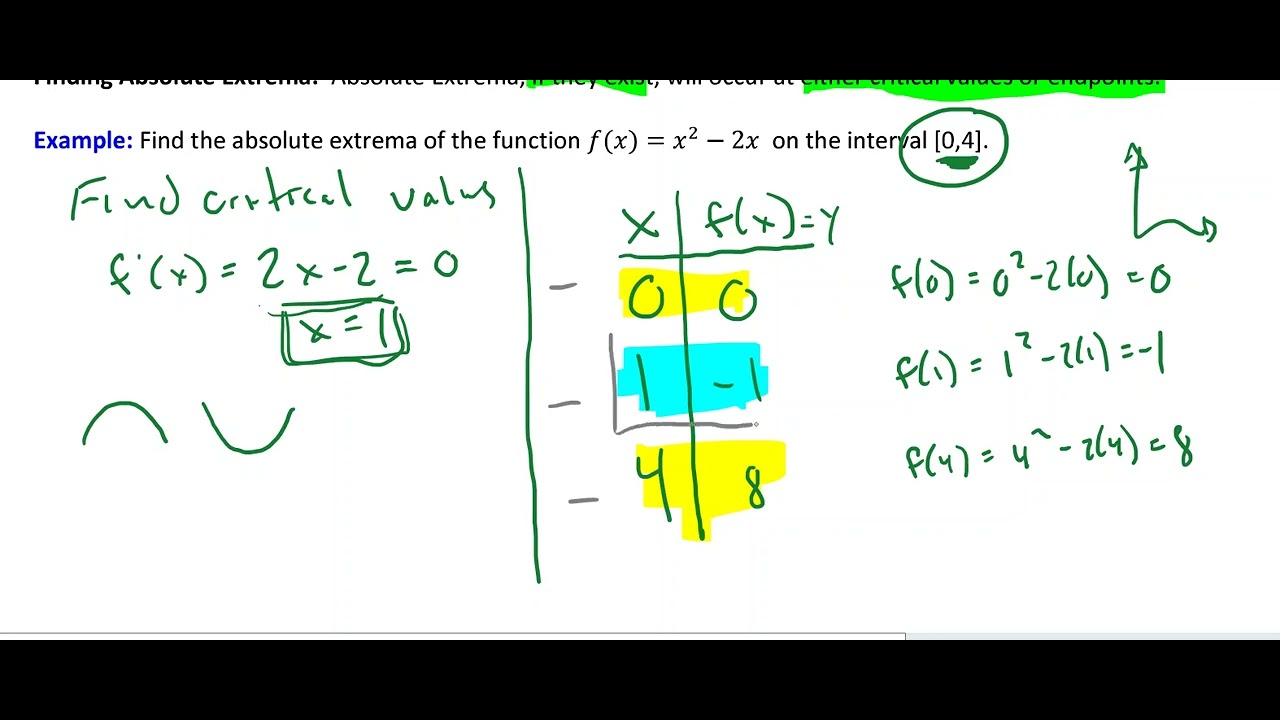
Finding Absolute Extrema (Max/Min) on a Closed Interval [a, b]

Professor Monte
3 Apr 202104:06
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Professor Monty discusses the process of finding absolute extrema, which are the maximum and minimum values, of a function on a closed interval. He illustrates this with a specific function and interval, showing how to find critical values by setting the derivative equal to zero and solving. The video introduces the illegal move method for factoring trinomials, which is useful when the trial and error approach is challenging. After identifying critical values, Monty emphasizes the importance of ensuring they fall within the given interval. He then demonstrates how to evaluate the function at these critical points and the interval's endpoints to determine the absolute maximum and minimum values. The video concludes with a reminder that absolute extrema must occur at critical values or endpoints, and encourages viewers to practice and subscribe for more informative content.

Takeaways
  • 📚 To find absolute extrema (max/min values) on a closed interval, first find critical values by setting the derivative equal to zero.
  • 🔍 For a function like '3x^2 - 2x - 1', factoring can be done using the illegal move method, which is helpful for solving trinomials.
  • 📐 After finding critical values, ensure they are within the given interval; if not, they are irrelevant to the extrema on that interval.
  • 📈 Plug the critical values and endpoints into the original function to find the y-values.
  • 🏁 The largest y-value corresponds to the absolute maximum, and the smallest y-value corresponds to the absolute minimum on the interval.
  • 🔑 The absolute extrema will occur at either a critical value or an endpoint of the interval.
  • 📋 When reporting the absolute extrema, state the value of the function (f(x)) at the point where the extremum occurs.
  • ✅ The process does not require a chart of signs, as the focus is on evaluating the function at specific points.
  • 🤓 Practice is key to becoming proficient in finding absolute extrema of functions.
  • 📹 Watching videos and subscribing to educational channels can be a helpful way to learn and practice finding absolute extrema.
  • 💡 Remember to discard critical values that do not fall within the interval of interest.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is about finding the absolute extrema, which are the maximum and minimum values, of a function on a closed interval.

  • What is the function given in the video?

    -The function given in the video is not explicitly stated, but it is implied to be a quadratic function as the derivative mentioned is '3x^2 - 2x - 1'.

  • What is the interval on which the absolute extrema is being found?

    -The interval on which the absolute extrema is being found is from negative 1 to 2.

  • What is the first step in finding the absolute extrema?

    -The first step in finding the absolute extrema is to take the derivative of the function and set it equal to zero to find the critical values.

  • How does one factor the derivative mentioned in the video?

    -The derivative '3x^2 - 2x - 1' can be factored using the trial and error method or the illegal move method, which is a technique for factoring trinomials.

  • What are the two critical values found from the derivative?

    -The two critical values found from the derivative are x = -1/3 and x = 1.

  • Why are critical values checked against the interval?

    -Critical values are checked against the interval to ensure they are within the range being considered for the absolute extrema, as only values within the interval are relevant.

  • What values are plugged into the function to determine the extrema?

    -The critical values (-1/3 and 1) and the endpoints of the interval (-1 and 2) are plugged into the original function to determine the extrema.

  • What is the absolute maximum value found in the video?

    -The absolute maximum value found in the video is 4, which occurs at x = 2.

  • What is the absolute minimum value found in the video?

    -The absolute minimum value found in the video is 1, which occurs at both x = 1 and x = -1.

  • Why does the video mention that the extrema must occur at a critical value or an endpoint?

    -The extrema must occur at a critical value or an endpoint because, by definition, these are the only places where the function can achieve a maximum or minimum on a closed interval.

  • What does the video suggest for those who need more practice with factoring?

    -The video suggests that those who need more practice with factoring should check out another video that discusses the illegal move method for factoring trinomials.

Outlines
00:00
📚 Finding Absolute Extrema

Professor Monty introduces the topic of finding absolute extrema, which are the maximum and minimum values of a function on a closed interval. He demonstrates the process with a specific function and interval, explaining how to find critical values by taking the derivative and setting it to zero. The derivative of the function is factored using the illegal move method, which is further explained in another video. The critical values are then checked to ensure they fall within the interval of interest. A chart is created with the critical values and endpoints, and the original function is evaluated at these points to determine the absolute maximum and minimum values.

Mindmap
Keywords
💡Absolute extrema
Absolute extrema refers to the largest (maximum) and smallest (minimum) values that a function can attain over a given interval. In the video, the concept is central to understanding how to find the highest and lowest values of a function on a closed interval from 'a' to 'b'. The script discusses finding these values by analyzing critical points and endpoints of the interval.
💡Closed interval
A closed interval includes all the points between its endpoints, which are specified by the script as 'a' and 'b'. In the context of the video, the interval from negative 1 to 2 is a closed interval, and the goal is to find the function's absolute extrema within this range.
💡Derivative
The derivative of a function is a measure of the rate at which the function's value changes with respect to changes in its variable. In the script, taking the derivative of the given function is the first step towards finding critical values, which are potential locations for absolute extrema.
💡Critical values
Critical values are the points at which the derivative of a function is zero or undefined. They are crucial in the process of finding absolute extrema because they represent potential maximum or minimum points of the function. The script demonstrates how to find these by setting the derivative equal to zero and solving for 'x'.
💡Factoring
Factoring is a mathematical method of breaking down a polynomial into its constituent parts, or factors. In the video, the process of factoring is mentioned as a way to simplify the derivative and solve for critical values. The script specifically mentions using the 'ac method' for factoring trinomials.
💡Interval
In the context of the video, an interval refers to a specific range of values on the x-axis within which the function's behavior is being analyzed. The interval is important for determining which critical values are relevant to the search for absolute extrema.
💡Endpoints
Endpoints are the starting and ending points of an interval. In the script, the endpoints of the interval from negative 1 to 2 are negative 1 and 2. These points are included in the analysis because the absolute extrema could occur at these locations.
💡Original function
The original function is the mathematical expression that is being analyzed for its extrema. In the video, the original function is represented by 'f(x)', and its values at critical points and endpoints are calculated to determine the absolute maximum and minimum.
💡Maximum and minimum values
Maximum and minimum values are the highest and lowest points that a function reaches. The script explains how to identify these values by evaluating the function at critical points and endpoints, with the largest value indicating the absolute maximum and the smallest indicating the absolute minimum.
💡Chart of signs
A chart of signs is a technique used in calculus to determine the sign of a function's derivative over intervals. Although the script mentions that a chart of signs is not necessary for this particular problem, it is a method sometimes used in conjunction with finding critical values.
💡Trial and error method
The trial and error method is an informal approach to solving mathematical problems by testing different possibilities until the correct solution is found. In the context of the video, it is mentioned as an alternative way to find the factors of the derivative, although the script prefers the 'ac method'.
Highlights

Professor Monty discusses finding absolute extrema, which are the maximum and minimum values of a function on a closed interval.

The example function given is f(x) = 3x^2 - 2x - 1, and the interval considered is from -1 to 2.

To find critical values, the derivative of the function is taken and set to zero.

The derivative f'(x) = 3x^2 - 2x - 1 is factored using the illegal move method for easier solving.

The factored derivative results in critical values x = -1/3 and x = 1.

Critical values must be within the interval of interest; otherwise, they are disregarded.

A table is created with x-values and corresponding f(x) values for endpoints and critical points.

The largest f(x) value from the table represents the absolute maximum of the function on the interval.

The smallest f(x) value indicates the absolute minimum of the function on the interval.

By plugging in the values into the original function, we find that the absolute maximum is f(2) = 4.

The absolute minimum occurs twice at f(1) = 1 and f(-1) = 1, indicating the minimum value is 1.

Absolute extrema can only occur at critical points or endpoints, not elsewhere.

No need for a chart of signs; instead, compare the function values to find the absolute extrema.

The video encourages viewers to like and subscribe for more educational content.

Practice is emphasized as key to understanding and applying the process of finding absolute extrema.

The method simplifies the process of finding absolute extrema without needing complex sign charts.

The illegal move method for factoring trinomials is mentioned as a helpful technique for solving derivatives.

The video provides a step-by-step guide on finding absolute extrema, making it accessible for learners.

Transcripts

Browse More Related Video

Calculus 1: Relative Extrema Examples

3.4 - Using Derivatives to Find Absolute Max and Min Values

Absolute Maximum and Minimum Values - Finding absolute MAX & MIN of Functions - Calculus

Candidates Test with Definite Integrals

Finding Absolute Extrema (Max/Min) on an Open Interval (a, b)

Finding Absolute Extrema

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Absolute ExtremaCritical ValuesDerivativeInterval AnalysisMathematicsFactoringEducationalMax MinCalculusTrinomialsProfessor Monty