Distance and Displacement using Position and Velocity

turksvids

21 Sept 201908:35

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses the concepts of distance and displacement in the context of motion, focusing on their calculation without the use of calculus integrals. It defines displacement as the straight-line distance from the start point, which includes magnitude and direction, making it a vector quantity. Distance, on the other hand, is the sum of the absolute values of all displacements during the motion. The script uses a position function to illustrate how to find displacement by simple substitution. To calculate distance, the process involves identifying turning points where velocity is zero, which requires finding derivatives. An example is provided using the position function X(t) = sin(2t) - t, with t ranging from 0 to π/2, to demonstrate how to find both displacement and distance traveled. The video concludes by emphasizing that while displacement is easier to calculate, distance involves more work, including finding turning points and summing the absolute values of displacements.

Takeaways

📏 Displacement is the straight-line distance from the start to the end point, including direction.
🔄 Distance is the total path length traveled, regardless of direction, and is always a positive value.
📍 Displacements can be negative, indicating direction relative to the starting point.
🔢 Distance is calculated as the sum of the absolute values of displacements for each segment of the journey.
🚫 Velocity must be zero at a turning point, which is crucial for calculating distance traveled.
📈 To find distance, first determine when and where the object changes direction by finding when velocity equals zero.
🧮 Use derivatives to find turning points, which are essential for calculating distance.
📐 The position function is used to find displacement by substituting the endpoint and starting point into the function.
📊 For distance, create a table with time (T) and position (X of T) values at the start, end, and any turning points.
⛓ The process of finding distance involves breaking down the journey into segments and summing the absolute displacements.
🧷 Memorizing the unit circle is beneficial for understanding the sine function and its values at different angles.
📋 Writing out each step clearly is important for understanding and checking the work, especially when dealing with distance calculations.

Q & A

What is displacement in the context of the video?
-Displacement is the distance and direction from the starting point to the final position of a particle after its journey. It is a vector quantity with both magnitude and direction.
How is displacement calculated?
-Displacement is calculated by subtracting the initial position from the final position of the particle. It is the net change in position from the start to the end of the journey.
What does a negative displacement value indicate?
-A negative displacement value indicates that the final position is in the opposite direction from the starting point, for example, to the left or below the starting point.
What is the difference between distance and displacement?
-Distance is the sum of the absolute values of all the displacements during the journey, regardless of direction, while displacement is the straight-line distance from the start to the end point with direction considered.
How is distance calculated when a particle moves back and forth in a straight line?
-Distance is calculated by summing the absolute values of the displacements for each leg of the motion, which means you add up all the distances covered in each direction without considering the direction.
Why is it necessary to find when velocity is equal to zero when calculating distance?
-Velocity being equal to zero indicates a turning point where the direction of motion changes. These points are important to identify the different legs of motion for calculating the total distance traveled.
What is the relationship between position and velocity?
-Velocity is the derivative of position with respect to time. It is the rate of change of position, indicating how the position changes over time.
How does the video determine the turning points of the motion?
-The video determines the turning points by finding the times when the velocity (the derivative of the position function) is equal to zero.
What is the significance of the unit circle in the context of the sine function?
-The unit circle helps in remembering the values of the sine function at different angles. It is used to quickly find the sine of specific angles like 0, π/2 (90 degrees), π, and so on.
How does the video approach the calculation of distance traveled?
-The video approaches the calculation of distance traveled by first finding the turning points, then creating a table with the starting point, ending point, and turning points, and finally summing the absolute values of the displacements on each leg of the journey.
What is the final result for the distance traveled in the example problem provided in the video?
-The final result for the distance traveled in the example problem is √3 + π/6 units.
Why is it said that distance traveled is more complicated than displacement?
-Distance traveled is more complicated than displacement because it involves finding the absolute values of all displacements at each leg of the journey, including identifying turning points and summing these values, whereas displacement is a straightforward calculation of the initial and final positions.

Outlines

00:00

📐 Understanding Displacement and Distance

This paragraph introduces the concepts of distance and displacement. Displacement is defined as the straight-line distance from the start to the end point, including direction, making it a vector quantity. Distance, on the other hand, is the total length of the path traveled by an object, regardless of direction, and is a scalar quantity. The paragraph emphasizes that displacement is easier to calculate and contrasts it with distance, which requires summing the absolute values of displacements for each segment of the journey. An example is provided to illustrate the calculation of displacement and distance for a particle moving in a straight line with changes in direction.

05:03

🔍 Calculating Displacement and Distance with Position and Velocity

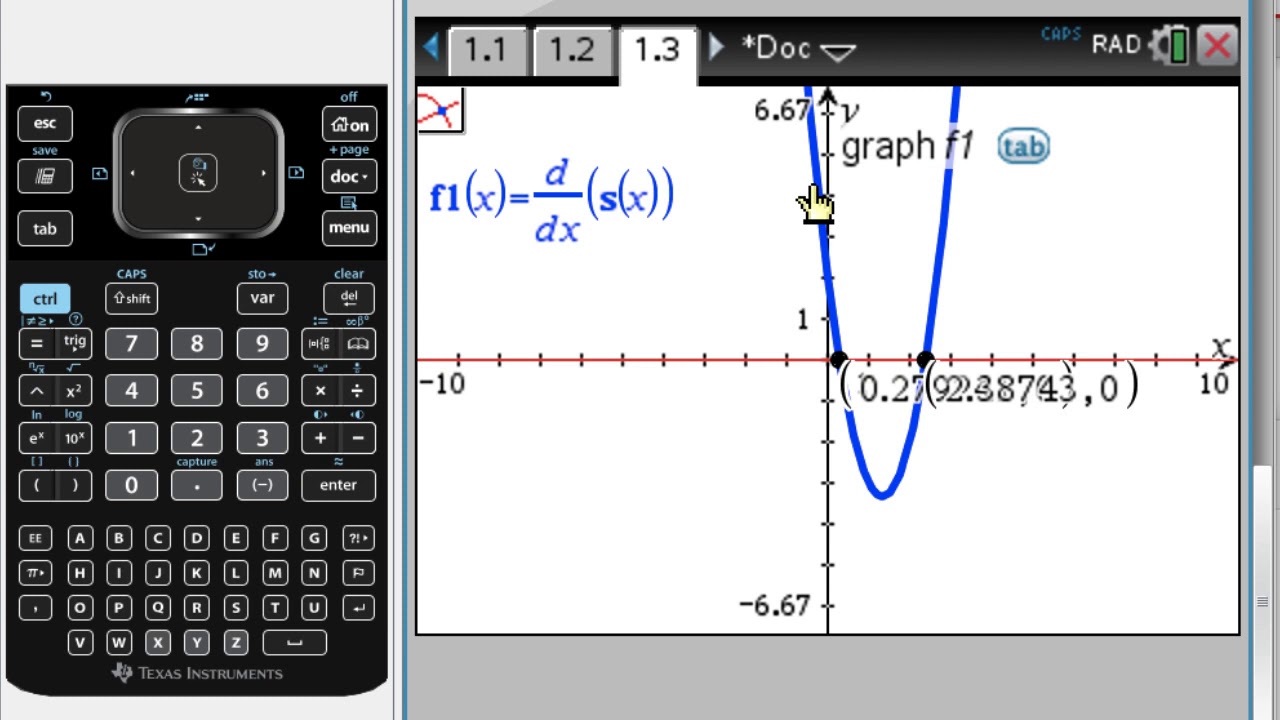
The second paragraph delves into calculating displacement and distance using a given position function over a specific time interval. It explains that to find displacement, one must evaluate the position function at the start and end points of the interval and subtract the two. For distance, the process is more complex and involves identifying when the object changes direction, which occurs when the velocity is zero. The paragraph outlines the steps to find the turning points by taking the derivative of the position function to get the velocity function, and then solving for when the velocity equals zero. The example provided uses a position function X(t) = sin(2t) - t, with t ranging from 0 to π/2, to calculate both displacement and distance traveled. The solution involves finding the derivative, identifying the turning point within the given interval, creating a table with key values of t and X(t), and summing the absolute values of displacements between these points to find the total distance traveled.

Mindmap

Keywords

💡Displacement

Displacement is a vector quantity that represents the shortest distance from the initial to the final position of an object, along with the direction of that distance. In the video, it is used to describe how far an object is from its starting point after a journey, and it is calculated by subtracting the starting position from the final position. For example, a displacement of negative six units indicates that the object is six units to the left or below the starting point.

💡Distance

Distance is a scalar quantity that measures the total length of the path traveled by an object, regardless of its direction. It is calculated by summing the absolute values of all displacements during the motion. In the video, distance is distinguished from displacement by the fact that it accounts for all the movements made by an object, even if they are in opposite directions, and is used to calculate the total path length covered.

💡Velocity

Velocity is a physical quantity that describes the rate of change of an object's position with respect to time. It is a vector quantity that includes both the speed (magnitude) and direction of the object's motion. In the video, velocity is crucial for determining when an object changes direction, which is when its velocity is zero. This is important for calculating the distance traveled, as the object can only change direction at these points.

💡Position Function

A position function is a mathematical representation that describes the position of an object as a function of time. It is often denoted as X(t), where X is the position vector and t is time. In the video, the position function X(t) = sin(2t) - t is given, and it is used to calculate both displacement and distance traveled by substituting different values of t into the function.

💡Derivative

The derivative of a function is a measure of the rate at which the function's value changes with respect to its variable. In the context of the video, the derivative of the position function (V(t) = X'(t)) is used to find the velocity function, which is essential for determining the turning points of the object's motion.

💡Turning Points

Turning points on a graph are the points where the direction of the function changes, typically from increasing to decreasing or vice versa. In the video, finding the turning points involves setting the velocity function equal to zero to determine when the object changes direction. These points are crucial for calculating the distance traveled, as they mark the segments of the path where the object moves in a particular direction.

💡Unit Circle

The unit circle is a circle with a radius of one unit, centered at the origin of a coordinate system. It is used in trigonometry to define the sine and cosine functions. In the video, the unit circle is referenced to find the values of sine for specific angles, such as sine of PI/3 and sine of PI, which are used in the calculation of displacement and distance.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for determining the derivative of a composite function. It states that the derivative of a function composed of two functions is the product of the derivative of the outer function and the derivative of the inner function. In the video, the chain rule is used to find the derivative of the position function involving the sine function.

💡Power Rule

The power rule is a basic rule in calculus that allows for the differentiation of polynomial functions. It states that the derivative of x^n, where n is a constant, is n*x^(n-1). In the video, the power rule is used to find the derivative of the term -t in the position function, resulting in -1.

💡Absolute Value

The absolute value of a number is its non-negative value, essentially its distance from zero on the number line, regardless of direction. It is used in the calculation of distance traveled to ensure that all displacements, whether positive or negative, contribute to the total path length. In the video, absolute values are taken of the differences in position to calculate the distance traveled on each segment of the object's path.

💡Interval

In mathematics, an interval refers to a continuous segment of a function's domain. It is often used to specify the range of values over which a function is considered. In the video, the interval from zero to PI/2 is used to define the time period over which the displacement and distance are calculated for the given position function.

Highlights

Distance and displacement are discussed using position and velocity without calculus integrals

Displacement is the distance from the start point with magnitude and direction, a vector quantity

Distance is the sum of absolute values of displacements on each leg of motion

Example given of calculating displacement and distance for a particle moving left and right

Displacement is the final position minus the initial position

Distance involves adding the absolute values of displacements for each segment of motion

Turning points where velocity is zero are key to finding distance traveled

Derivatives are used to find turning points by setting velocity equal to zero

Example problem involves finding displacement and distance on interval from 0 to π/2

Position function X(t) = sin(2t) - t is given

Displacement is found by evaluating X(π/2) - X(0)

Distance traveled involves finding turning points within the interval and summing absolute displacements

Table is constructed with turning points and endpoints to organize calculations

Absolute value of displacement is calculated for each segment between turning points

Final distance traveled is found by summing the absolute displacements for each segment

Distance traveled is more complex to calculate than displacement

Key takeaway is to find velocity, turning points within the interval, and sum absolute displacements for distance

Transcripts

Browse More Related Video

Displacement and Distance Traveled On Calculator

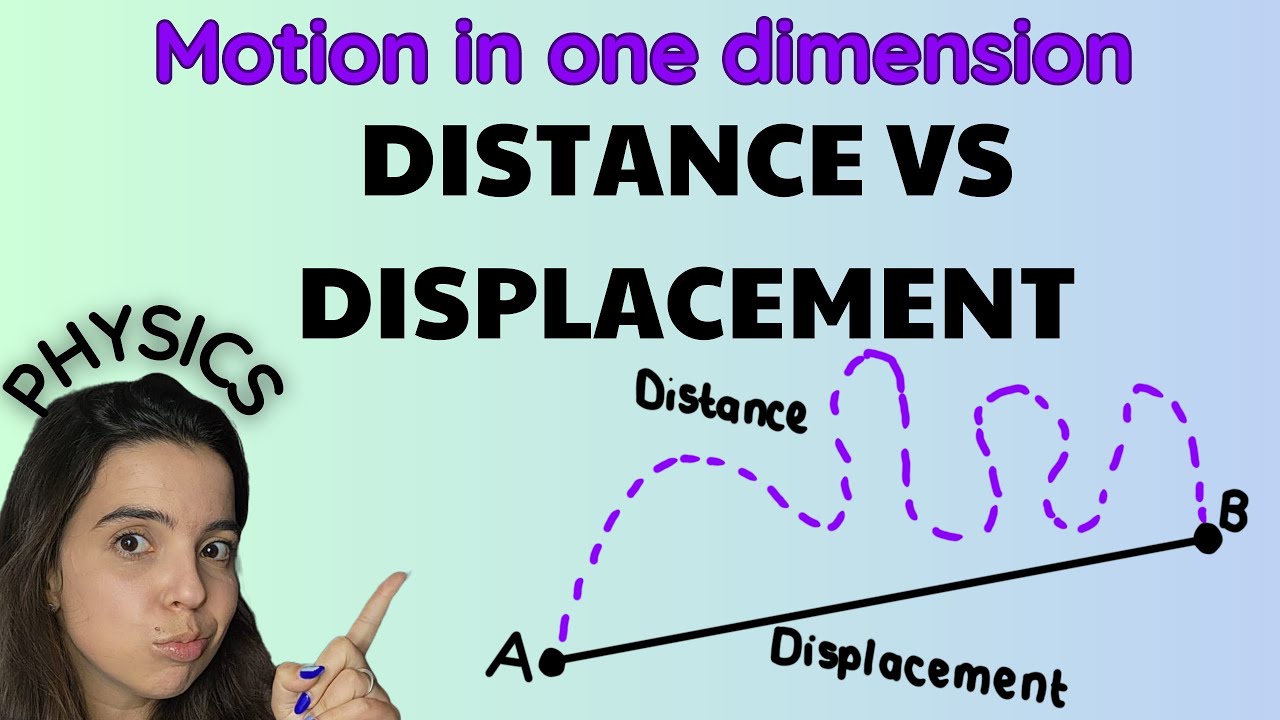
Displacement and Distance: Motion in One Dimension

Describing MOTION: DISTANCE and DISPLACEMENT || GRADE 7 SCIENCE _ PHYSICS

Displacement and Velocity - How is it different from Distance and Speed? | Physics

Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained

Distance Versus Displacement

Distance and Displacement using Position and Velocity

Takeaways

Q & A

What is displacement in the context of the video?

How is displacement calculated?

What does a negative displacement value indicate?

What is the difference between distance and displacement?

How is distance calculated when a particle moves back and forth in a straight line?

Why is it necessary to find when velocity is equal to zero when calculating distance?

What is the relationship between position and velocity?

How does the video determine the turning points of the motion?

What is the significance of the unit circle in the context of the sine function?

How does the video approach the calculation of distance traveled?

What is the final result for the distance traveled in the example problem provided in the video?

Why is it said that distance traveled is more complicated than displacement?