Distance vs. Displacement & Speed vs. Velocity | Kinematics Explained
TLDRThis engaging video script explores the fundamental concepts of distance and displacement, as well as speed and velocity, using a relatable example of a Lego character moving along a number line. The script clarifies that distance traveled is a scalar quantity, measured as the sum of all movements without regard to direction, akin to an odometer that continuously increases. In contrast, displacement is a vector quantity, representing the change in position from start to finish, which includes direction. The video also distinguishes between speed, a scalar that measures the total distance traveled over time, and velocity, a vector that factors in displacement over time, including direction. The script emphasizes that both speed and velocity are average values and highlights the conditions under which distance and displacement can be equivalent, particularly when an object moves in a straight line without changing direction. This insightful explanation demystifies common physics terms and provides a clear understanding of their applications and differences.
Takeaways
- 📏 **Distance vs. Displacement**: Distance is the total path length traveled by an object, while displacement is the straight-line change in position from the starting point to the final position.
- 🔄 **Direction in Displacement**: Displacement is a vector quantity, which means it has both magnitude and direction, indicated by a positive or negative sign.
- 📊 **Distance as a Scalar**: Distance is a scalar quantity, only having magnitude without direction, and is always a positive value.
- ⏱️ **Speed and Time**: Speed is the total distance traveled divided by the time taken, and it is a scalar quantity, always positive.
- 🚀 **Velocity and Time**: Velocity is the displacement divided by the time taken, and it is a vector quantity, which means it includes information about direction.
- 🔁 **Reversing Direction**: When an object changes direction, its displacement can be negative, indicating the direction of travel is opposite to the defined positive direction.
- 🔢 **Calculating Average Speed and Velocity**: Both speed and velocity can be calculated as averages over a period of time, indicated by a bar over the variable (e.g., 𝑣̄ for average velocity).
- 📈 **Positive and Negative Values**: In one-dimensional motion, positive values typically represent movement to the right and negative to the left, though this can be defined differently based on the context.
- ⏩ **Continuous Motion**: The odometer of a car, similar to distance traveled, continuously increases regardless of the direction of travel.
- 🔄 **Interchangeable Terms**: In certain scenarios, such as straight-line motion without change in direction, distance and displacement can be the same, making speed and velocity also equal.
- 🚦 **Speed Limit Analogy**: Speed is never negative as it represents the rate of motion without direction, unlike velocity, which can be negative if the direction of motion is opposite to the defined positive direction.
- 🧭 **Understanding Scalars and Vectors**: Scalars like distance and speed provide less information than vectors like displacement and velocity, which include directional information.
Q & A
What is the difference between distance and displacement?
-Distance is the total path length traveled by an object, regardless of direction, while displacement is the straight-line distance from the starting point to the final position, with a direction indicated by its sign (positive or negative).
How is displacement calculated in one-dimensional motion?
-Displacement is calculated as the final position minus the initial position. It is a vector quantity, meaning it has both magnitude and direction.
What is the relationship between a scalar and a vector?
-A scalar is a quantity with only magnitude, like distance, and no direction. A vector, on the other hand, has both magnitude and direction, like displacement and velocity.
How is speed defined and what is its relationship with distance?
-Speed is defined as the distance traveled divided by the time taken. It is a scalar quantity and always has a positive value, regardless of the direction of motion.
What is velocity and how does it differ from speed?
-Velocity is defined as the displacement divided by the time taken. Unlike speed, velocity is a vector quantity, which means it includes information about both the rate of motion and the direction of motion.
When can distance and displacement be considered the same?
-Distance and displacement can be the same when an object moves in a straight line without changing direction, such as moving from one point to another in a single direction.
Why are speed and velocity often represented with an average?
-Speed and velocity are often represented with an average (indicated by a line over the symbol, like v̄ for average velocity) because they describe the overall rate and direction of motion over a period of time or distance traveled.
How does the direction of motion affect the calculation of displacement?
-The direction of motion affects the calculation of displacement because displacement is a vector quantity. If an object moves to the right (positive direction), the displacement is positive, and if it moves to the left (negative direction), the displacement is negative.
What is the significance of the negative sign in displacement?
-The negative sign in displacement indicates that the object has moved in the opposite direction from the defined positive direction, typically to the left in one-dimensional motion.
Can you provide an example of when speed might not fully describe motion?
-An example where speed does not fully describe motion is when an object is moving back and forth in a straight line. Speed would only tell you how fast it is moving, but not the change in direction, which is important for understanding the object's displacement.
Why is it important to distinguish between speed and velocity when analyzing motion?
-It is important to distinguish between speed and velocity because speed only provides information about how fast an object is moving, while velocity provides both the rate and the direction of motion, which is crucial for understanding the object's change in position over time.
How can the terms 'speed' and 'velocity' be used interchangeably without causing confusion?
-The terms 'speed' and 'velocity' can be used interchangeably when the motion being analyzed is in a single direction without any change in direction, as in such cases, both the magnitude of speed and the magnitude of velocity (considering the direction as positive) will be the same.
Outlines
📏 Understanding Distance and Displacement
This paragraph introduces the concepts of distance and displacement using a Lego guy as an example. Distance is the total path length traveled, measured by a fitness tracker, and is a scalar quantity. Displacement, on the other hand, is the change in position from the starting point and is a vector quantity, indicating direction. The Lego guy moves 5 meters to the right and then 7 meters to the left, resulting in a total distance of 12 meters and a displacement of -2 meters, indicating he ended up 2 meters to the left of his starting point.
🚀 Clarifying Speed and Velocity
The second paragraph explains the difference between speed and velocity. Speed is a scalar that measures the total distance traveled over time and is always a positive value. Velocity is a vector that measures displacement over time and includes direction. Using the Lego guy's movement, the average speed is calculated as 2 m/s over a period of 6 seconds, covering a distance of 12 meters. The average velocity is calculated as -1/3 m/s, reflecting the direction of the final position relative to the starting point. The paragraph also clarifies that both speed and velocity are average values and can sometimes be used interchangeably when an object moves in a straight line without changing direction.
Mindmap
Keywords
💡Distance
💡Displacement
💡Speed
💡Velocity
💡Scalar
💡Vector
💡Average Speed
💡Average Velocity
💡Direction
💡Position
💡Time
Highlights
The difference between distance and displacement, as well as speed and velocity, is explained using a Lego guy on a number line.
Distance traveled is the sum of all steps taken by an object, measured like a Fitbit or car odometer.
Displacement is defined as the change in position from the initial to the final position, represented as a vector with direction.
Distance is a scalar quantity, having no direction, while displacement is a vector quantity with direction.
The concept of scalars and vectors in physics is introduced, with examples of their application.
Speed is calculated as the total distance traveled divided by the time taken, and is always a positive value.
Velocity is the displacement divided by time, and includes direction, making it a vector quantity.
Average speed and average velocity are represented by a bar over the respective symbols (v̄ for velocity, v for speed).
The direction of velocity indicates the direction of movement, with positive indicating rightward movement and negative indicating leftward movement.
In certain scenarios, distance and displacement can be the same, such as when an object moves in a straight line without changing direction.
The terms speed and distance, and displacement and velocity, are sometimes used interchangeably in physics, which can be confusing.
The conditions under which distance and displacement are the same are explained, emphasizing the importance of direction in displacement.
The importance of understanding the context in which distance and displacement, and speed and velocity, are used is emphasized.
The use of a Lego guy as an example provides a visual and relatable way to understand abstract physical concepts.
The transcript uses a step-by-step approach to explain the concepts, making the content accessible to a wide audience.
The explanation clarifies the common misconceptions and confusion between the terms distance/displacement and speed/velocity.
The transcript provides a clear distinction between scalar and vector quantities in the context of one-dimensional motion.
The concept of average speed and average velocity is introduced, highlighting the importance of time in their calculation.
Transcripts
Browse More Related Video

Displacement and Velocity - How is it different from Distance and Speed? | Physics
Velocity and speed: Motion in One Dimension
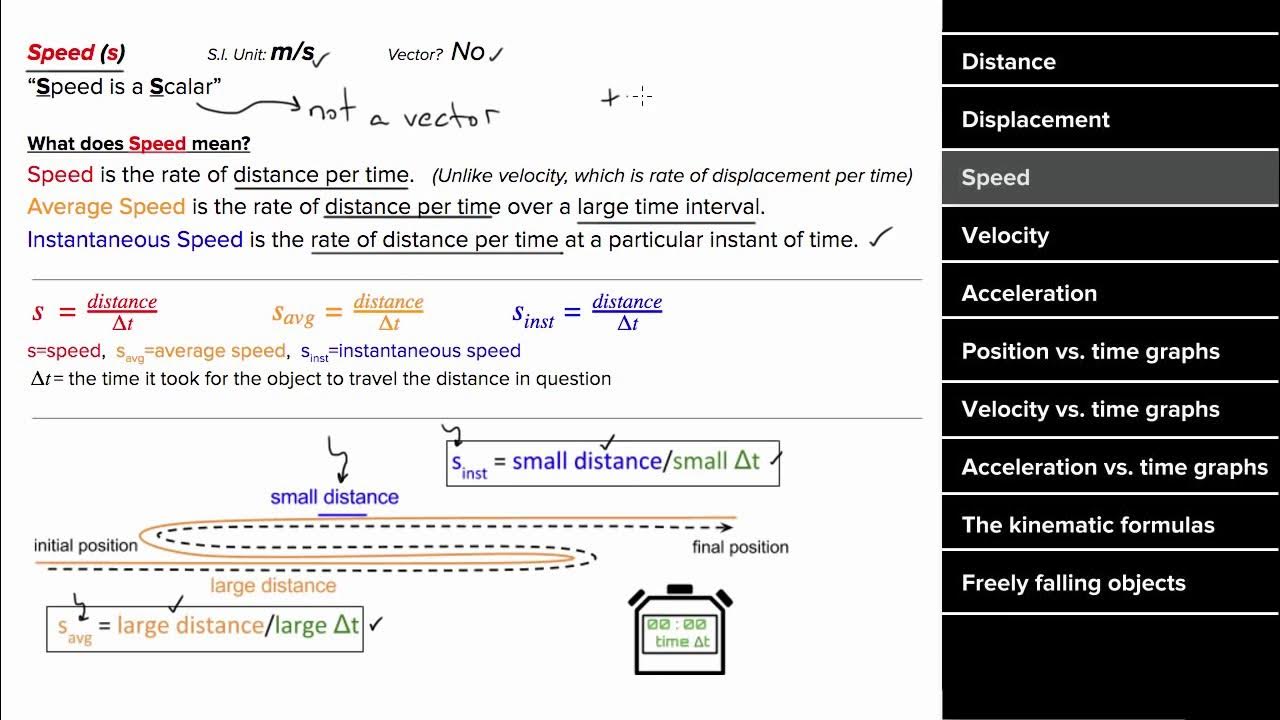
AP Physics 1 review of 1D motion

10 - Learn Distance and Displacement in Physics (Displacement Formula Vs. Distance Formula)

Distance, Displacement, Speed and Velocity

What is Velocity? - Full Concept of Velocity - Physics | Infinity Learn
5.0 / 5 (0 votes)
Thanks for rating: