Describing MOTION: DISTANCE and DISPLACEMENT || GRADE 7 SCIENCE _ PHYSICS
TLDRThis engaging video script delves into the fundamental concepts of motion in physics, focusing on distance and displacement. It begins by emphasizing the importance of understanding motion, which is a common yet complex phenomenon. The script outlines the learning target, which includes describing motion as a change in position, differentiating between distance and displacement, and measuring the total distance and displacement. The video explains that motion is relative and requires a reference point to define an object's movement. It distinguishes between scalar and vector quantities, highlighting that distance is a scalar (magnitude only), while displacement is a vector (magnitude and direction). The script uses a practical example of a boy's walk to illustrate the calculation of total distance and displacement, demonstrating how to apply the concepts using the Cartesian plane. The video concludes with the boy's displacement being zero, as he returns to his starting point, reinforcing the concept that displacement is the straight-line change in position. The script also encourages viewers to subscribe and stay informed about similar educational content.
Takeaways
- 📐 **Motion Description**: Motion is described relative to a reference point, involving both distance and direction from the reference point.
- 🔄 **Change in Position**: An object is considered to be in motion when it changes its position from an initial to a final position.
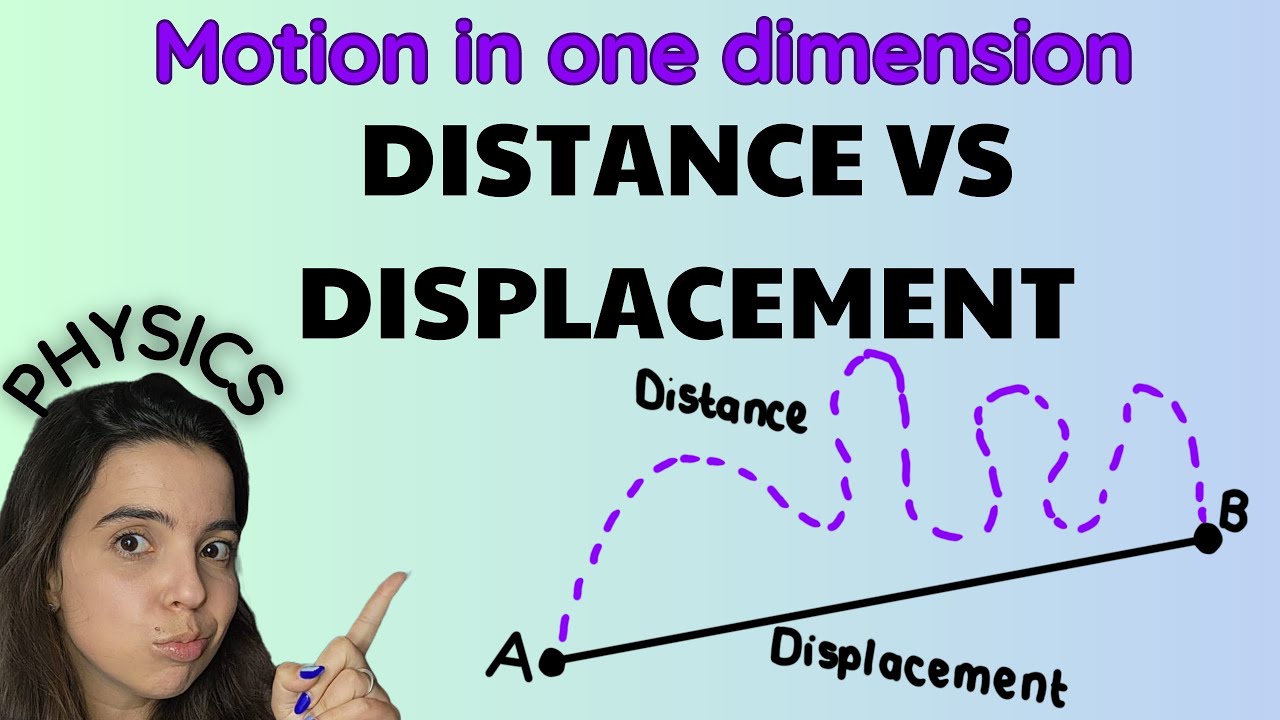
- 📏 **Distance vs. Displacement**: Distance is a scalar quantity, measuring the total path length traveled, while displacement is a vector quantity that considers both magnitude and direction.
- 🧭 **Direction Consideration**: Displacement focuses on the straight-line distance between the initial and final positions, taking into account the direction of travel.
- 📊 **Scalar and Vector**: Scalar quantities have only magnitude (e.g., 10 meters), whereas vector quantities have both magnitude and direction (e.g., 10 meters North).
- 🛤️ **Total Distance Calculation**: The total distance is found by summing the lengths of each individual segment of the path taken.
- ↗️ **Cartesian Plane for Displacement**: The Cartesian plane, with its X and Y axes, helps in plotting and understanding the direction components of displacement.
- 🔢 **Displacement Calculation**: Displacement is calculated by considering the direction (positive or negative) along each axis and summing these directional movements.
- 🔄 **Example Walk Path**: The example given involves a boy walking in a path that returns him to the starting point, resulting in a total displacement of zero.
- 🔀 **Understanding Motion**: Understanding motion in physics requires knowledge of distance, displacement, speed, velocity, and acceleration.
- 📈 **Learning Target**: The video's learning target is to describe motion as a change in position, differentiate between distance and displacement, and measure total distance and displacement.
Q & A
What are the three quantities related to motion in physics?
-The three quantities related to motion in physics are distance, displacement, and acceleration.
How is motion described in physics?
-Motion in physics is described relative to something else, which is the reference point, and it involves understanding the object's change in position, including both distance and direction.
What is the difference between a scalar and a vector quantity?
-Scalar quantities have only magnitude, such as distance and speed, while vector quantities have both magnitude and direction, such as displacement and velocity.
How do you differentiate distance from displacement?
-Distance is the total length of travel by an object and is a scalar quantity, whereas displacement is the straight line distance between the initial and final position and is a vector quantity considering both magnitude and direction.
What is the role of a reference point in describing motion?
-A reference point is essential to describe motion as it provides a fixed position to compare the object's movement against. It helps to determine if the object is moving towards or away from the reference point and in which direction.
How is the total distance calculated for an object's path?
-The total distance is calculated by adding the lengths of each individual segment of the path traveled by the object.
How is displacement calculated when an object returns to its starting position?
-When an object returns to its starting position, the total displacement is zero because the final position coincides with the initial position, regardless of the path taken.
What is the Cartesian plane and how is it used in calculating displacement?
-The Cartesian plane is a two-dimensional coordinate system formed by the intersection of the X and Y axes. It is used to plot the motion of an object and calculate displacement by considering the direction and magnitude of movement along each axis.
What are the signs assigned to the directions on the Cartesian plane?
-On the Cartesian plane, North is positive for the Y-axis, South is negative; East is positive for the X-axis, and West is negative.
In the example given, why is the total displacement of the boy zero?
-The total displacement of the boy is zero because he ends up at the same position where he started (point A), meaning the net change in position is zero despite the various directions he moved in.
What is the significance of understanding the difference between distance and displacement in physics?
-Understanding the difference between distance and displacement is significant because it allows for accurate descriptions of an object's motion, taking into account not just how far it has traveled but also the direction of that travel.
How can one enhance their understanding of motion in physics?
-One can enhance their understanding of motion in physics by studying the concepts of distance, displacement, and acceleration, and by practicing problems that involve calculating these quantities for different types of motion.
Outlines
📐 Understanding Motion and Position
The first paragraph introduces the concept of motion and its importance in physics. It explains that motion is relative and requires a reference point to describe an object's change in position. The video's learning target is to describe motion, differentiate between distance and displacement, and measure the total distance and displacement. The paragraph also distinguishes between scalar and vector quantities, with distance being a scalar (magnitude only) and displacement being a vector (magnitude and direction). An example is given to illustrate the difference: a boy's walk is analyzed in terms of the total distance and displacement covered.
📏 Calculating Total Distance and Displacement
The second paragraph provides a mathematical approach to calculating the total distance and displacement of the boy's walk. It explains that total distance is the sum of all individual distances traveled, which in this case is 16 meters. For displacement, the direction of movement is considered using the Cartesian plane, where north and east are positive directions, and south and west are negative. The boy's walk is plotted on the Cartesian plane, and by adding the vectors (3m North, 5m East, 3m South, 5m West), it is determined that the total displacement is zero because the boy ends up at the starting point.
🎓 Conclusion and Engagement
The third paragraph is a brief closing segment that thanks the viewers for watching and encourages new subscribers to subscribe and enable notifications for future videos. It includes a musical element, suggesting a conclusion to the educational content.
Mindmap
Keywords
💡Motion
💡Distance
💡Displacement
💡Scalar Quantity
💡Vector Quantity
💡Point of Reference
💡Initial and Final Position
💡Cartesian Plane
💡Direction
💡Speed
💡Acceleration
Highlights
Motion is described relative to something else, requiring an understanding of quantities related to it.
Learning about motion in physics involves understanding distance, displacement, speed, velocity, and acceleration.
Describing motion involves noting an object's position, which includes distance from a reference point and direction.
An object is considered moving if it changes its position relative to a reference point.
Distance traveled by an object is measured with respect to a point of reference.
Displacement is the change in position of an object from its original position.
Scalar quantities have magnitude only, while vector quantities have magnitude and direction.
Distance is a scalar quantity and is the total length of travel by an object.
Displacement is a vector quantity and is the straight-line distance between the initial and final position.
The difference between distance and displacement is that distance is the length of travel between two points, while displacement is the shortest distance between two points.
An example is given where a boy walks various distances in different directions, illustrating how to calculate total distance and displacement.
Total distance is calculated by adding the lengths of individual travels.
Total displacement considers the direction of movement and is calculated using the Cartesian plane for direction.
The Cartesian plane uses a coordinate system with positive and negative directions corresponding to North, South, East, and West.
In the example, the boy's total displacement is calculated to be zero, as he returns to his initial position.
Understanding the difference between scalar and vector quantities is crucial for accurately describing motion.
The video concludes with an invitation to subscribe for more educational content on similar topics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: