AP Calculus Multiple Choice Practice Test (2020 AP CED Problems)
TLDRThis video script presents a comprehensive review of 22 multiple-choice questions from AP Calculus, covering a range of topics including limits, derivatives, integrals, and differential equations. The instructor uses a step-by-step approach to solve each problem, applying various mathematical rules and theorems such as L'Hôpital's rule, the product and chain rules, and the second fundamental theorem of calculus. The video also touches on more advanced concepts like Taylor polynomials and the LaGrange error bound, providing insights into how to tackle complex calculus problems. The script is designed to prepare students for the AP exam by reinforcing key concepts and building problem-solving skills.
Takeaways
- 📚 The video covers 22 multiple-choice questions from AP Calculus, derived from the course exam description available online.
- 🔢 Problem 1 involves applying L'Hôpital's rule to find the limit of a trigonometric function as x approaches zero.
- 📉 For question 2, the concept of differentiability is explored by examining the continuity and derivatives of a piecewise function.
- ⚙️ Question 3 deals with the product rule and linearity of differentiation when finding the derivative of a function defined as a product of two other functions.
- 🧮 In question 4, implicit differentiation is used to find the derivative of a relationship between x and y variables.
- 🚀 Question 5 relates to the rate of change of a cylinder's volume with respect to time, given the rates of change of its radius and height.
- 📏 Question 6 focuses on the calculation of a definite integral using summations and right Riemann sums, with a focus on the correct interval for Δx.
- 📈 Question 7 examines the behavior of an accumulation function, specifically when the function H is increasing based on the graph of its derivative.
- 🧬 The video demonstrates U-substitution and the process of elimination in solving integrals, as shown in question 8.
- 📐 Question 9 involves identifying a differential equation that matches a given slope field, a common task in differential calculus.
- 🏞️ For question 10, the area of a region bounded by certain curves is calculated using integration with respect to y, due to the form of the equations given.
- 🎯 The video concludes with advanced calculus topics suitable for Calculus BC, such as integration by parts, series convergence, and Taylor polynomials.
Q & A
What is the first step in solving the limit problem as X approaches zero of (1 - cos^2(2x)) / (2x^2)?
-The first step is to substitute X with zero, which leads to an indeterminate form of 0/0, indicating the need to apply L'Hôpital's rule.
Why is L'Hôpital's rule applied in the first limit problem?
-L'Hôpital's rule is applied because the direct substitution results in an indeterminate form of 0/0, suggesting that the limit can be found by taking the derivatives of the numerator and denominator instead.
What is the final answer to the first limit problem?
-The final answer to the first limit problem is 1, after applying L'Hôpital's rule and simplifying the expression using known limits.
At what value of x is the function F not differentiable in question number two?
-The function F is not differentiable at x = 2, because the left and right derivatives at this point are not equal, indicating a discontinuity in the derivative.
What is the method used to find the derivative of H(x) in question three?
-The method used to find the derivative of H(x) is the product rule, as H(x) is defined as the product of two functions, f(x) and g(x).
What is the approach to solving the implicit differentiation problem in question four?
-The approach involves differentiating both sides of the given equation with respect to x, using the power rule, product rule, and chain rule as necessary, and then solving for dy/dx.
How is the rate of change of the volume of a cylinder with respect to time found in question five?
-The rate of change of the volume with respect to time is found by differentiating the volume formula V = πr^2h with respect to time, using the given rates of change for the radius and height.
What is the equivalent to the definite integral from two to six of the square root of x dx in question six?
-The equivalent expression is found by evaluating the right Riemann sum, using the correct delta x and evaluation points, which leads to the selection of the correct answer choice.
What is the interval on which the function H is increasing in question seven?
-The function H is increasing on the intervals [0, 1] and [3, 7]. This is determined by finding the derivative of H and analyzing where it is positive.
What is the method to solve the integral in question eight?
-The method to solve the integral is by using a U-substitution, where U is chosen to be the quantity under the square root, and then integrating with respect to U.
How is the area of the region R in question ten found?
-The area of region R is found by integrating the function x = e^y from y = 1 to y = 2, as the region is bounded by the graph of x = e^y, x = 10, and the horizontal lines y = 1 and y = 2.
What is the approach to determine the number of points of inflection for the function F in question 14?
-The approach is to graph the second derivative of the function F and count the number of relative extrema, as points of inflection occur where the second derivative changes sign.
Outlines
📚 AP Calculus Multiple Choice Questions Overview
The video introduces a series of 22 multiple choice questions from AP Calculus, sourced from the course's exam description available online. The presenter provides a link for further reference and begins solving the first problem involving limits and L'Hôpital's rule. The approach to solving calculus problems and the presenter's methodical explanation set the stage for the rest of the video.
🔍 Continuity and Differentiability of Function F
The second problem discusses the differentiability of a piecewise function F. The presenter evaluates the function's continuity at specific points and calculates the left and right derivatives to determine differentiability. The conclusion is that the function is not differentiable at x=2, leading to the selection of answer choice B.
📈 Application of Product Rule and Chain Rule in Calculus
The presenter tackles a table of values for functions f and g, and their derivatives, to find the derivative of a new function H. By applying the product rule and considering the given values, the solution leads to answer choice A. The video then moves on to implicit differentiation and the calculation of the rate of change for a cylinder's volume, with the presenter carefully guiding viewers through each step.
🧮 Definite Integrals and Riemann Sums
The focus shifts to definite integrals and Riemann sums, with the presenter simplifying the problem by recognizing it as a right Riemann sum. By evaluating the given options and using the properties of definite integrals, the correct answer, choice C, is identified. The video also covers the concept of accumulation functions and their derivatives in the context of AP Calculus.
📉 Analysis of a Particle's Acceleration and Velocity
The video presents a problem involving the velocity and acceleration of a particle moving along a straight line. The presenter uses the given velocity function to find the acceleration at a specific time, T=1.2, showcasing the application of differentiation in physics. The process involves substituting values and performing calculations, ending with the selection of answer choice C.
🤔 Must-Be-True Statements and Inflection Points
The presenter addresses a 'must be true' problem, which typically involves the intermediate value theorem or the mean value theorem. By analyzing the given derivative values and using process of elimination, the presenter determines that there exists a value C where the derivative equals a specific amount, leading to answer choice D. The video also covers finding points of inflection for a function defined by a derivative, using calculus concepts to arrive at the correct answer.
🍯 Calculating the Volume of Poured Honey
The video presents a problem involving the calculation of honey volume poured through a funnel over time. By integrating the given rate function, the presenter finds the total volume of honey poured from time T=0 to T=3. The integration process is demonstrated, leading to the selection of answer choice D.
🧮 Integration by Parts and Logistic Differential Equations
The presenter uses integration by parts to find the integral of a product of functions, identifying the correct approach and applying the method to obtain the answer, which is A. The video then covers the logistic differential equation, emphasizing the importance of memorizing key formulas and applying them to find the limit of a function as time approaches infinity, resulting in the selection of answer choice K.
📐 Parametric Equations and Second Derivatives
The video delves into parametric equations, showing how to find the second derivative of a curve defined by X(T) and Y(T). The presenter advises starting with the first derivative and then finding the second, emphasizing the importance of understanding the process. The correct answer, A, is selected after performing the necessary calculus operations.
🔢 Convergence Tests and Maclaurin Series
The presenter discusses conditional convergence, applying tests to determine the convergence of series. By evaluating the absolute value of terms and using the alternating series test, the presenter identifies the conditionally convergent series, leading to the selection of answer choice B. The video concludes with the Maclaurin series for the derivative of an exponential function, using differentiation to find the correct series representation, which corresponds to answer choice D.
🌐 Polar Coordinates and Taylor Polynomials
The video covers the area calculation of a shaded region defined by a polar curve. By graphing and finding the bounds, the presenter sets up an integral to find the area, which is solved to find the correct answer, A. The final problem involves Taylor polynomials and the Lagrange error bound for a function with given derivative constraints. The presenter calculates the error bound and identifies the smallest value of K that satisfies the condition, leading to the selection of answer choice A.
Mindmap
Keywords
💡L'Hôpital's Rule
💡Derivative
💡Implicit Differentiation
💡Product Rule
💡Definite Integral
💡Riemann Sum
💡Accumulation Function
💡Differential Equation
💡Logistic Differential Equation
💡Taylor Polynomial
💡Maclaurin Series
💡Polar Coordinates
Highlights
The video covers 22 multiple-choice questions from AP Calculus, derived from the course exam description available online.
For problem one, l'Hôpital's rule is applied to find the limit as X approaches zero of a trigonometric function.
The solution to problem one concludes with the final answer being one, using the known limit of sine function.
In problem two, the function F's differentiability is questioned, with the conclusion that it is not differentiable at x equals two.
Problem three involves calculating the derivative of a function H, defined as a combination of functions f and g, using the product rule.
The method of implicit differentiation is used in problem four to solve for dy/dx in an equation involving x and y.
The rate of change of the volume of a cylinder is determined in problem five, with respect to time, given the rates of change of radius and height.
Problem six deals with the evaluation of a definite integral representing the area under a square root function from 2 to 6.
The video explains how to determine the interval on which the function H is increasing using its derivative in problem seven.
An integral involving a square root and a linear function is solved in problem eight, with a focus on avoiding common mistakes.
Problem nine involves identifying the differential equation that corresponds to a given slope field.
The area of a region bounded by certain curves is calculated in problem ten using integration with respect to Y.
The limit of a function as X approaches one from the right is evaluated in problem eleven, showcasing a straightforward limit calculation.
The acceleration of a particle at a specific time is calculated in problem twelve using given velocity and time.
Problem thirteen involves analyzing a table of function values to determine which statement must be true based on the data provided.
The number of points of inflection for a function F on a given interval is determined in problem fourteen using calculus concepts.
The volume of honey poured through a funnel over a three-minute period is calculated in problem fifteen using integration.
Problem sixteen involves using integration by parts to find the value of an integral involving a function f and its derivative.
The logistic differential equation is applied in problem seventeen to find the limit of a function as time approaches infinity.
Problem eighteen requires finding the second derivative of a curve defined by parametric equations.
Conditional convergence of a series is determined in problem nineteen using the alternating series test.
The Maclaurin series for the derivative of a given function is derived in problem twenty using series expansion and differentiation.
The area of a shaded region defined by a polar curve is calculated in problem twenty-one using graphical and integral methods.
Problem twenty-two involves finding the smallest value of K for which the LaGrange error bound guarantees a certain level of accuracy for a Taylor polynomial approximation.
Transcripts
Browse More Related Video
AP Calculus AB/BC Multiple Choice Practice Test (2012 AP CED Problems)
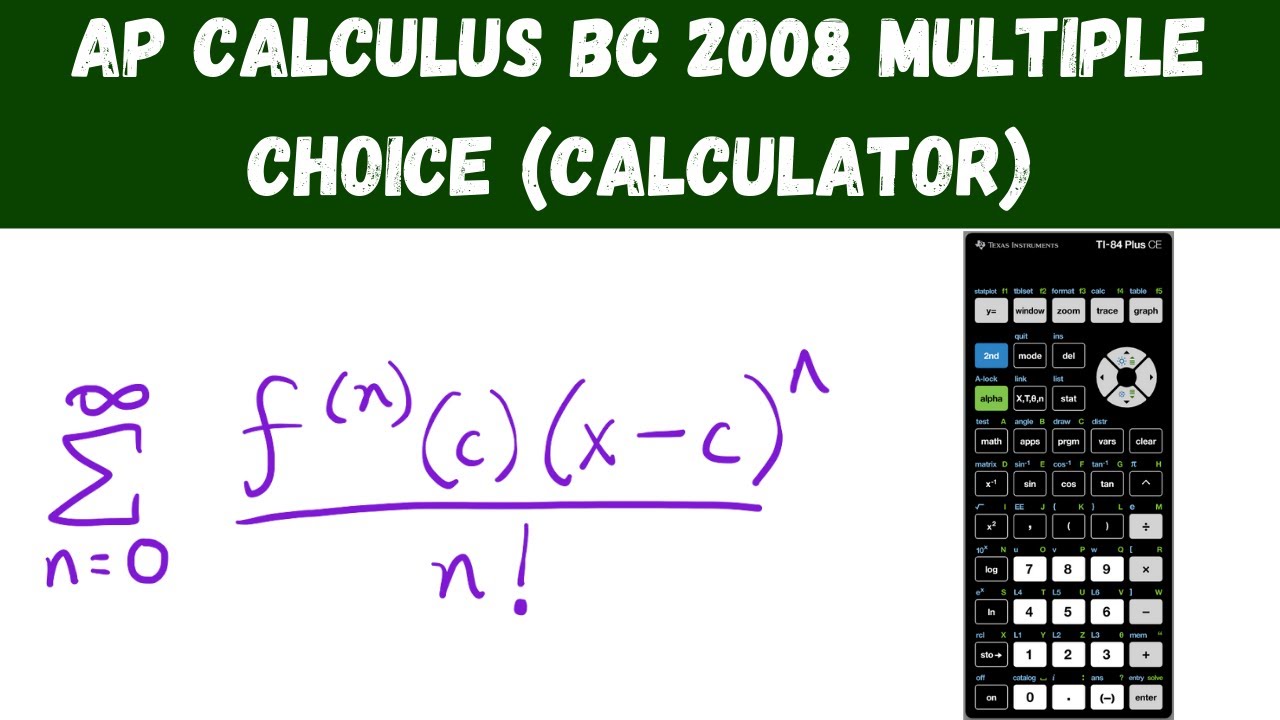
AP Calculus BC 2008 Multiple Choice (calculator) - Questions 76 - 92
2019 Multiple choices 1,2

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28

AP Calculus: EVERYTHING YOU NEED TO KNOW

What We Must Know for Calculus AB
5.0 / 5 (0 votes)
Thanks for rating: