AP Calc AB & BC Practice MC Review Problems #3
TLDRThe video script presents a comprehensive review session on multiple-choice calculus problems. The presenter approaches each problem methodically, often opting to solve them from first principles rather than relying on memorized formulas. The session covers a range of topics including rate of change, slope fields, exponential decay, limits, concavity and convexity, implicit differentiation, and the mean value theorem. The presenter emphasizes the importance of understanding the underlying concepts and provides strategies for tackling complex problems, such as drawing unit diagrams and using sign charts. The script concludes with a problem-solving approach to finding the minimum value of a function on a closed interval, highlighting the candidate's theorem. Throughout the video, the presenter maintains an engaging tone, encouraging viewers to attempt the problems first and then refer back to the video for clarification.
Takeaways
- 😀 The video focuses on solving multiple-choice review problems related to calculus.
- 🤔 When confused, drawing diagrams and visualizing units can help understand the problem better.
- 📝 Techniques like finding the slope of tangent lines and using derivatives are employed to solve calculus problems.
- 🔍 Analyzing graphs and understanding slope fields aid in identifying differential equations.
- 🤓 Understanding concepts like rate of change, concavity, and derivatives is crucial for problem-solving.
- 🧠 Techniques such as separation of variables and power rules are utilized for differential equations.
- 📊 Sign charts and graphical analysis assist in determining concavity, increasing/decreasing functions, and critical points.
- 🎯 Applying the fundamental theorem of calculus helps find values of functions given their derivatives.
- ⚖️ The candidates test is used to find absolute extrema on closed intervals.
- 🍀 The video concludes with a summary of problem-solving strategies and wishes the viewer good luck.
Q & A
What is the rate at which the depth of the snow is changing at T equals one hour, given the model s(T) = (1/2)T^2 + 150 + (1/4)T for snow depth in inches?
-To find the rate of change of snow depth with respect to time, you differentiate the given function s(T) with respect to T. The derivative, s'(T), will give the rate of change in inches per hour. For T = 1 hour, you substitute T with 1 in the derivative to get the rate at that specific time.
How can you determine the differential equation represented by a given slope field?
-You can determine the differential equation by looking for horizontal asymptotes, where the slope does not change along a horizontal line, indicating that the x variable has no impact on the slope. This allows you to eliminate options with an x term. Then, by testing specific values or looking for points where the slope is zero, you can distinguish between the remaining options.
What is the expression for P(T), the amount of oil left in the well at time T in years, given the rate of oil being pumped is proportional to the amount of oil left?
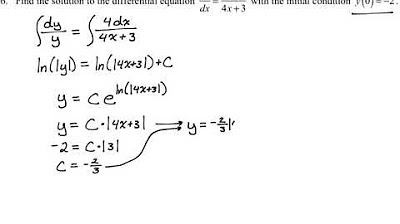
-The rate of change of the amount of oil, dP/dt, is given by a constant K times the amount of oil left, P. This is a first-order linear differential equation, which can be solved by separation of variables. The solution form is P = Ce^(KT), where C is a constant determined by the initial condition (P(0) = 2 million gallons).
How do you find the instantaneous velocity of a particle at a specific time T, given its position function Y(T)?
-The instantaneous velocity of a particle at time T is the first derivative of its position function with respect to time, Y'(T). You calculate this by differentiating Y(T) with respect to T.
What is the largest open interval on which the function F(x) = 2x^3 + (11/2)x^2 - 10x is both concave down and decreasing?
-To find this interval, first determine the first and second derivatives of F(x). The first derivative, F'(x), will help identify where the function is decreasing (F'(x) < 0), and the second derivative, F''(x), will help identify where the function is concave down (F''(x) < 0). The interval where both conditions are met is the solution.
How do you express dy/dx in terms of y, given the equation 4x - 3(sec y)^2 = 3?
-This is an implicit differentiation problem. You differentiate both sides of the equation with respect to x, treating y as a function of x. The derivative of the left side involves the chain rule, and the right side becomes zero since it's a constant. You then solve for dy/dx.
What is the average velocity of a particle over the interval from T=1 to T=4, given its position function Y(T) = √(T^3 + 1)?
-The average velocity over the interval [T1, T2] is the change in position divided by the change in time, which is (Y(T2) - Y(T1)) / (T2 - T1). For the given interval from T=1 to T=4, you substitute these values into the position function and calculate the average rate of change.
What is the value of H(-2) if the graph of H', the first derivative of H, consists of two line segments and a semi-circle, and H(4) is given as 3?
-This is a fundamental theorem of calculus problem. You integrate H' from -2 to 4 and use the given value H(4) to find H(-2). The integral will involve calculating the area under the line segments and the semi-circle.
What is the minimum value of the function F(x) = x^3 - 9x^2 + 24x + 2 on the interval from -1 to 5?
-To find the minimum value, you first find the critical points by setting the first derivative, F'(x), to zero and solving for x. Then, evaluate the function at the critical points and the endpoints of the interval. The smallest value among these evaluations is the minimum value of the function on the interval.
How do you find the derivative dy/dx of the function y = cos^2(f(x))^4?
-You apply the chain rule for derivatives. The derivative of y with respect to x involves differentiating the outer function (cosine squared) and then the inner function (f(x) to the fourth power), and multiplying them by their respective derivatives.
What technique did the speaker use to solve problems when confused with many units involved?
-The speaker uses a technique of drawing a simple representation to get a sense of the units involved, which helps in understanding and solving the problem.
What is the strategy for solving a differential equation represented by a slope field with multiple choices?
-The strategy involves identifying horizontal asymptotes, eliminating options with x terms if the slope does not change along a horizontal line, and then comparing the remaining options by testing specific values or looking for zero slopes.
How does the speaker approach solving a problem with an initial condition in a differential equation?
-The speaker uses separation of variables to solve the differential equation, then applies the initial condition to find the constant of integration, and finally solves for the particular solution.
What is the process to determine the largest open interval where a function is concave down and decreasing?
-You find the first and second derivatives of the function. The first derivative determines where the function is decreasing (negative first derivative), and the second derivative determines where the function is concave down (negative second derivative). The interval where both conditions are true is the solution.
How does the speaker approach finding dy/dx in terms of y for an implicit differentiation problem?
-The speaker differentiates both sides of the equation with respect to x, treating y as a function of x, and then solves for dy/dx by rearranging the terms.
What is the approach to find the value of a function at a specific point if the graph of its derivative is given?
-The approach is to use the Fundamental Theorem of Calculus, integrating the derivative function from a known point to the point in question and using the given value to find the unknown value of the function.
How does the speaker find the minimum value of a function on a closed interval?
-The speaker finds the critical points by setting the first derivative to zero and also considers the endpoints of the interval. Then, the function is evaluated at these points to determine the minimum value.
Outlines
📚 Introduction to Multiple-Choice Review Problems
The video begins with an introduction to a series of multiple-choice review problems. The presenter explains that the problems are not from actual exams but are modeled after their difficulty level. They provide a link to the problems in the video description and offer guidance on how to approach the problems. The first problem involves calculating the rate of change of snow depth over time using a given mathematical model.
🔍 Analyzing a Differential Equation's Slope Field
The second paragraph discusses a problem involving a slope field for a differential equation. The presenter uses the process of elimination by identifying horizontal asymptotes and eliminating options with variables that should not affect the slope. The correct differential equation is determined by testing specific points and their corresponding slopes.
📉 Deriving an Expression for Oil Depletion Over Time
The third paragraph focuses on a differential equation representing the rate at which oil is pumped from a well, proportional to the amount of oil left. The presenter solves the differential equation using separation of variables and applies initial conditions to find constants. The problem involves calculating the amount of oil left in the well after a certain number of years.
🤔 Evaluating Limits and False Statements in a Multiple-Choice Context
In the fourth paragraph, the presenter tackles a multiple-choice question about evaluating limits. The strategy involves using substitution and focusing on whether the limit exists from the left and right. The presenter emphasizes the importance of finding a false statement among the options and correctly identifies the answer.
📈 Finding the Interval of Concavity and Decreasing Function
The fifth paragraph deals with identifying the interval where a given function is both concave down and decreasing. The presenter calculates the first and second derivatives, creates a sign chart, and uses it to determine the interval where both the first and second derivatives are negative.
🧮 Solving for the Derivative in Terms of Another Variable
The sixth paragraph involves an implicit differentiation problem where the derivative dy/dx is sought in terms of y. The presenter carefully applies the chain rule and simplifies the expression to find the derivative in terms of y, considering the relationship between secant, tangent, and cosine functions.
🚀 Applying Mean Value Theorem to a Particle's Motion
The seventh paragraph discusses a problem related to the instantaneous and average velocity of a particle moving along the y-axis. The presenter applies the mean value theorem to find the time at which the instantaneous velocity equals the average velocity over a given interval.
📐 Calculating the Derivative of a Composite Function
In the eighth paragraph, the presenter calculates the derivative of a composite function involving cosine squared. The derivative is found by applying the chain rule and considering the derivatives of the cosine and power functions.
🧷 Determining a Function's Value Using the Fundamental Theorem of Calculus
The ninth paragraph involves using the fundamental theorem of calculus to find the value of a function at a given point, given the graph of its derivative. The presenter calculates the integral of the derivative over an interval and uses geometric interpretation to find the function's value.
🔢 Finding the Minimum Value of a Function on a Closed Interval
The final paragraph focuses on finding the minimum value of a function on a closed interval. The presenter uses the candidate's test to identify critical points and endpoints, evaluates the function at these points, and determines the minimum value from the results.
Mindmap
Keywords
💡Multiple-choice review problems
💡Spiral curriculum
💡Derivative
💡Differential equations
💡Slope field
💡Concavity
💡Chain rule
💡Mean value theorem
💡Fundamental theorem of calculus
💡Critical points
💡Secant and Tangent
Highlights
The video provides a review of multiple-choice calculus problems without answer choices, as they are considered annoying to create.
Problems are not from actual exams but are based on their difficulty, with a link to the problems in the video description.
The first problem involves finding the rate of change of snow depth over time using a given mathematical model.
A technique for dealing with confusion in problems with many units is to draw a diagram to understand the units involved.
The solution to the first problem involves finding the derivative, representing the slope of the tangent line to the graph of the function.
The second problem involves identifying the correct differential equation from a given slope field by eliminating options with variables that do not match the field.
A strategy for multiple-choice calculus problems is to look for points where the slope is zero to help identify the correct differential equation.
The third problem deals with the rate at which oil is pumped from a well, modeled by a differential equation relating the rate of change of oil to the amount of oil left.
The solution to the oil well problem involves separating variables and solving for the constant and the rate constant using initial conditions.
The fourth problem is a multiple-choice question about the existence of limits of functions, which requires understanding of limits and their properties.
The solution process for limit problems may involve using substitution and analyzing the behavior of the function as it approaches certain values.
The fifth problem involves finding the largest open interval where a function is both concave down and decreasing by analyzing its first and second derivatives.
The sixth problem requires finding the derivative of an implicit function, which involves applying the chain rule and understanding the derivative of a constant.
The seventh problem is about finding the time at which the instantaneous velocity of a particle equals the average velocity over a given interval, which involves calculating derivatives and averages.
The eighth problem involves finding the derivative of a composite function, which requires understanding the derivative of a squared function and the chain rule.
The ninth problem is a fundamental theorem of calculus problem that requires finding the value of a function at a point given its derivative over an interval.
The tenth problem involves finding the minimum value of a function on a closed interval, which is a candidate's theorem problem requiring finding critical points and endpoints.
The video concludes with a summary of the solutions to the calculus problems, aiming to help viewers prepare for similar questions on the AP exam.
Transcripts
Browse More Related Video

AP Calc AB & BC Practice MC Review Problems #2
AP Calc AB & BC Practice MC Review Problems #5
AP Calc AB & BC Practice MC Review Problems #6

AP Calc AB & BC Practice MC Review Problems #7

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92

AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92
5.0 / 5 (0 votes)
Thanks for rating: