Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity
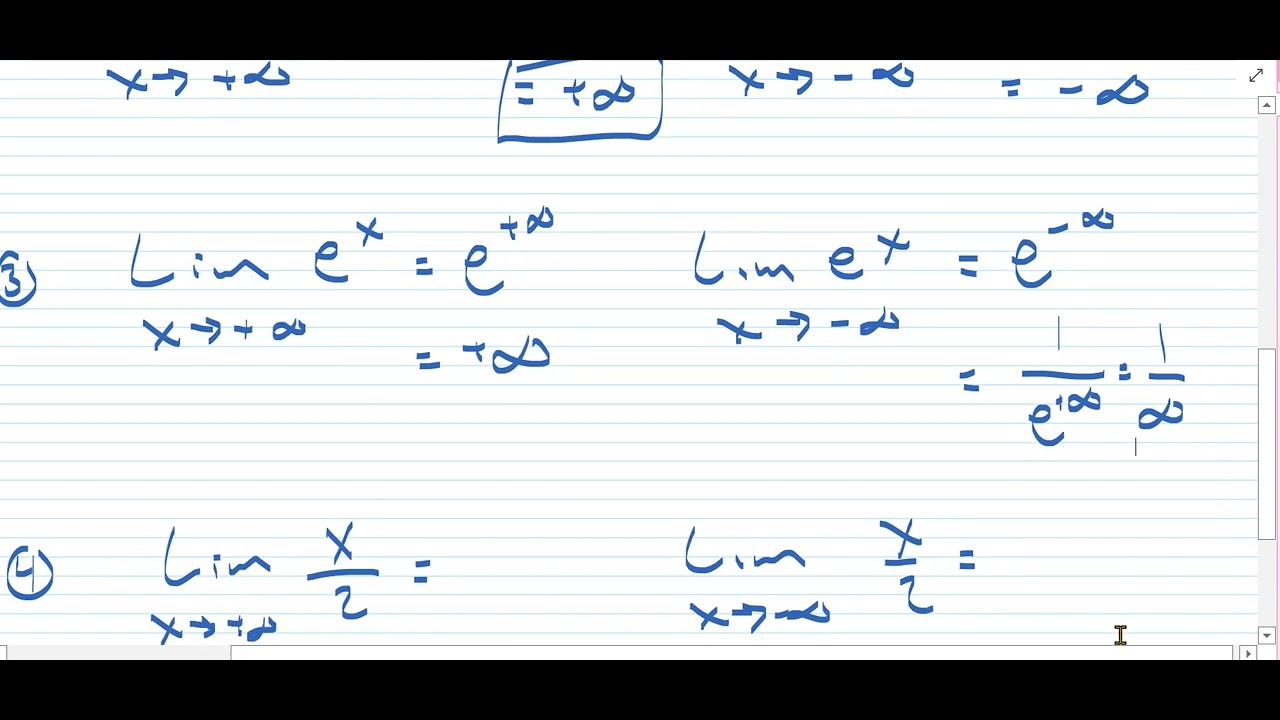
TLDRThe video script delves into the concept of limits in calculus, particularly as X approaches infinity or negative infinity. It explains that for any positive number K, the limit of 1/X^K as X approaches infinity or negative infinity is zero. The script uses graphical representations to illustrate this behavior, showing how the value of a function's reciprocal decreases as the function's value increases without bound. It also covers the process for determining limits at infinity, emphasizing the importance of dividing by the highest power term from the denominator. Several examples are provided to demonstrate how to apply these concepts to find limits, including the use of multipliers and the simplification of terms. The script further explores the behavior of functions as they approach vertical asymptotes, discussing how to determine if a limit exists and the concept of continuity in functions. It concludes with examples of determining continuity for piecewise functions and rational functions, highlighting the conditions for a function to be considered continuous at a point.
Takeaways
- 📚 The basic rule for limits as X approaches infinity states that for a positive number K, the limit of 1 over X to the power of K is 0.
- 📈 As X approaches infinity or negative infinity, the end behavior of a function can be observed by looking at the graphs and how they approach zero.
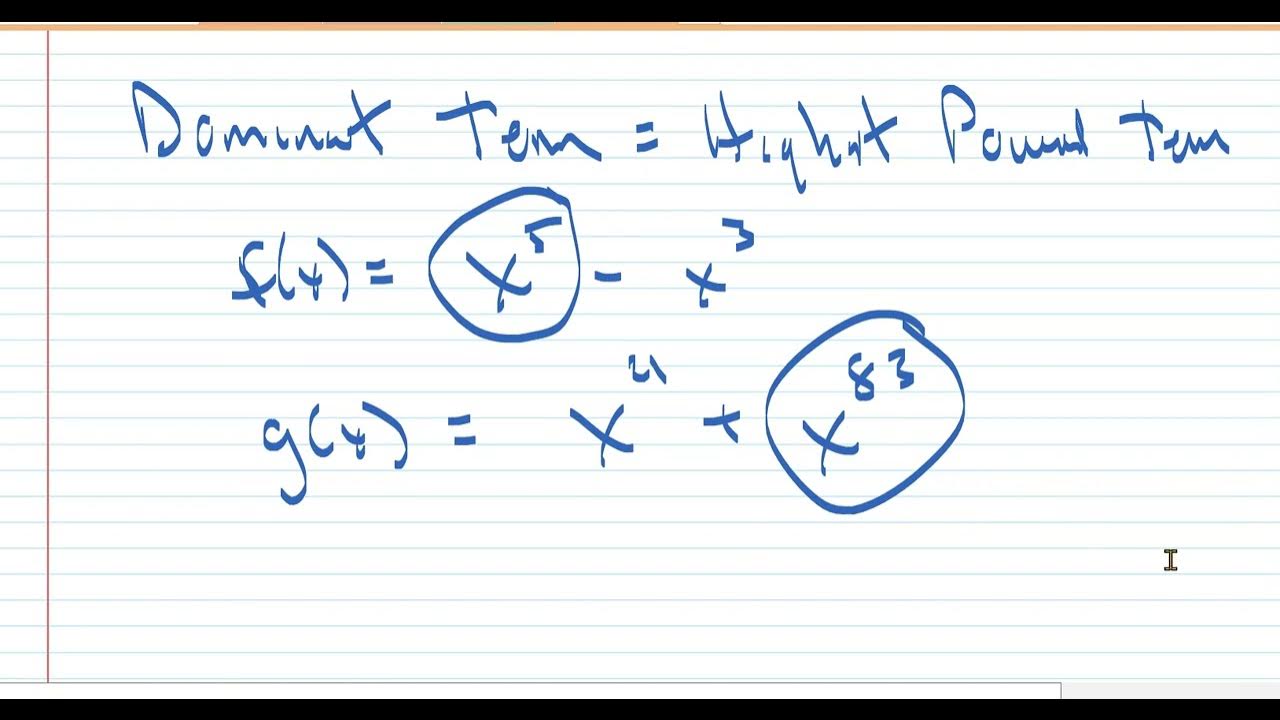
- 🔢 When finding limits at infinity, divide each term in the numerator and denominator by the highest power term from the denominator and simplify.
- 📉 For rational functions, the limit as X approaches infinity will often involve terms that approach 0, except for constant terms.
- 🤔 To determine continuity, a function must have a defined value at a point, an existing limit as X approaches that point, and the function value must equal the limit.
- 🚫 A function is discontinuous where it fails the criteria for continuity, such as having an undefined value or a gap in the graph.
- 📌 A vertical asymptote indicates that the limit does not exist at a certain point, as the function increases or decreases without bound on either side of that point.
- 🛠️ Polynomials are continuous everywhere because they do not have any points where the denominator is zero or any undefined points.
- 🔑 To find a value (like K in a piecewise function) that ensures continuity, equate the left and right limits at the point of interest and solve for the unknown.
- 📋 When analyzing piecewise functions for continuity, ensure that the function values and limits from both sides of any breakpoint are equal to confirm continuity at that point.
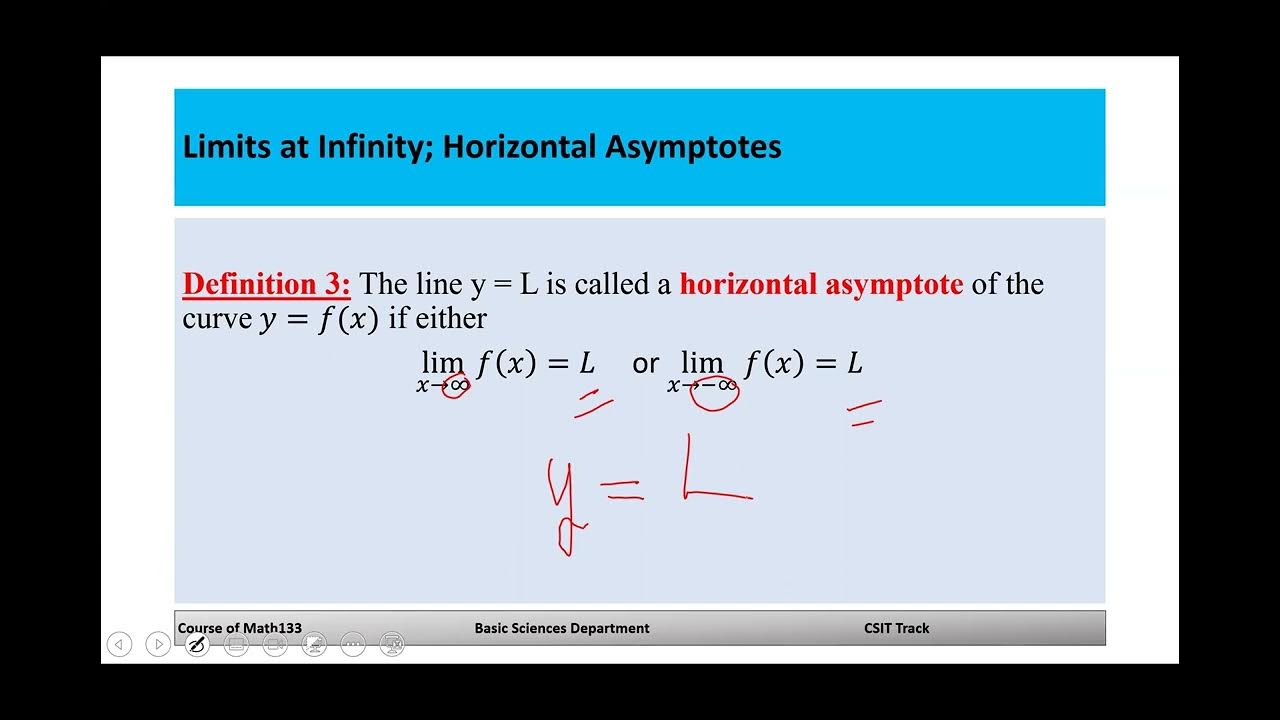
- ∞ A function that increases or decreases without bound as X approaches a certain value has a limit of infinity or negative infinity, respectively, indicating a vertical asymptote.
Q & A
What is the basic rule for the limit as X approaches infinity of 1 over X to the K power?
-The basic rule states that for K greater than zero, the limit as X approaches infinity of 1 over X to the K power is equal to 0. This also holds true as X approaches negative infinity.
How does the behavior of the function y equals 1 over X as X approaches positive and negative infinity?
-As X approaches both positive and negative infinity, the value of y equals 1 over X gets closer and closer to zero. This is indicative of the end behavior of the function.
What is the result of the limit as X goes to infinity of 4 over X cubed?
-The limit as X goes to infinity of 4 over X cubed is 0. This is derived by applying the rule that the limit of 1 over X to the K power as X approaches infinity is 0, where K is 3 in this case.
How do you determine limits at infinity for a rational function?
-To determine limits at infinity for a rational function, you divide each term in the numerator and denominator by the highest power term X to the K from the denominator, simplify each term, and then take the limit of each term, knowing that the limit of 1 over X to the K where K is positive is equal to 0.
What is the end behavior of the function 4x squared minus 3x plus 2 over 5 plus 6x minus x squared as X approaches infinity?
-The end behavior of the function as X approaches infinity is that it levels off at negative 4, creating a horizontal asymptote at y equals negative 4.
How does the profit function for producing and settling X thousands of toothpicks behave as production increases without bounds?
-As production increases without bounds, the average profit per thousand toothpicks approaches $5.00, as determined by taking the limit as X goes to infinity of the average profit function.
What does it mean for a function to be continuous?
-A function is considered continuous if it is defined at a point, the limit as X approaches that point exists, and the limit as X approaches the point is equal to the function value at that point. It implies the function can be drawn without lifting the pen from the paper, indicating a connected graph without breaks.
What is the criterion for a rational function to be continuous?
-A rational function is continuous everywhere it is defined, as long as its denominator is not equal to zero. Any point where the denominator equals zero is a point of discontinuity.
How do you determine the value of K for a piecewise function to be continuous at a certain point?
-To determine the value of K for a piecewise function to be continuous at a certain point, you need to ensure that the left and right limits at that point are equal, and that this common value is also equal to the function value at that point from both pieces of the function.
What is the condition for a limit to be considered as approaching infinity or negative infinity?
-A limit is considered as approaching infinity if the function's value increases without bound as X approaches a certain value C. Conversely, a limit approaches negative infinity if the function's value decreases without bound as X approaches C.
How do you identify if a function has a vertical asymptote at a certain point?
-A function has a vertical asymptote at a certain point if the function's value increases or decreases without bound as X approaches that point. This behavior is observed when the function cannot be evaluated at that point due to division by zero or other undefined operations.
Outlines
📚 Understanding Limits at Infinity
This paragraph introduces the concept of limits as a variable approaches infinity or negative infinity. It discusses the basic rule that for a positive number K, 1 over X to the power of K approaches zero as X approaches either positive or negative infinity. The paragraph uses graphical representation and algebraic manipulation to explain the behavior of functions at extreme values of X.
🔍 Example Calculations and Limit Procedures
The second paragraph provides examples of calculating limits at infinity. It demonstrates how to apply algebraic rules to isolate the highest power term from the denominator and simplify the expression before taking the limit. The process is illustrated with specific examples, showing how to handle different types of functions and polynomials.
📈 Analyzing End Behavior and Asymptotes
This part of the script delves into the end behavior of functions, specifically discussing horizontal and slant asymptotes. It explains how the behavior of a function as X approaches infinity or negative infinity can be determined by examining the non-zero terms of the function. The concept of profit functions and their implications on average profit per unit are also explored.
🤔 Dealing with Infinite Limits and Discontinuities
The fourth paragraph addresses infinite limits, explaining when a function increases or decreases without bound as it approaches a certain value. It also covers how to determine if a limit does not exist due to differing behaviors on either side of a point. The concept of continuity is introduced, emphasizing the importance of a function's ability to be graphed without interruption.
📉 Evaluating Limits from the Left and Right
This section focuses on how to find the left and right limits of a function when it is undefined at a certain point. It provides a method to determine the sign of the function near the point of discontinuity and how this can be used to infer the behavior of the function as it approaches that point.
🔗 Simplifying Functions and Testing Limits
The script continues with an example of simplifying a function and testing its limits from both sides of a discontinuity. It shows how factoring can help in reducing the complexity of the function and how to use one-sided limits to determine the behavior of the function at points of discontinuity.
🔁 Continuity Criteria and Function Evaluation
The sixth paragraph discusses the criteria for a function to be considered continuous. It outlines three conditions that must be met for a function to be classified as continuous at a point. The concept is applied to determine the continuity of different functions, emphasizing the importance of evaluating the function and its limit at the point in question.
📋 Determining Continuity Across Intervals
This part of the script explains how to determine where a function is continuous across an interval. It highlights that rational functions are continuous everywhere except where the denominator is zero. The process of finding the domain of continuity for a function is demonstrated, showing how to identify points of discontinuity.
🔍 Piecewise Functions and Continuity
The eighth paragraph deals with piecewise functions, explaining how to determine their continuity. It emphasizes the need to check the function value and the limit at the points where the function definition changes. The concept of one-sided limits is introduced for piecewise functions, and an example is provided to illustrate the process.
🎢 Finding Values for Continuous Piecewise Functions
The final paragraph focuses on finding the value of a constant that makes a piecewise function continuous across its entire domain. It demonstrates how to equate the left and right limits at the point of transition between different parts of the function to find the value of the constant that ensures continuity.
Mindmap
Keywords
💡Limit
💡Infinity
💡End Behavior
💡Reciprocal
💡Rational Function
💡Continuous Function
💡Asymptote
💡Piecewise Function
💡Polynomial
💡One-Sided Limits
💡Undefined Limit
Highlights
The limit as X approaches infinity of 1 over X to the K power is equal to 0 for positive K, which is a fundamental rule for understanding end behavior of functions.
End behavior of functions is discussed through the graphical representation of y = 1/X and y = 1/X^2, illustrating the concept of limits as X approaches infinity or negative infinity.
The reciprocal of a number approaching infinity gets smaller and eventually approaches zero, explained through the example of increasing values like a million, billion, and trillion.
A step-by-step process for determining limits at infinity is provided, emphasizing the division by the highest power term from the denominator.
Example problem demonstrates how to find the limit as X goes to infinity of 4/X^3, showcasing the application of the basic limit rule for reciprocal functions.
The concept of horizontal asymptotes is introduced, explaining the behavior of functions as X increases without bound, using the example of a profit function for toothpick production.
A method for finding the limit as X approaches a specific value is explained, which involves examining the signs of the function on the left and right sides of the target number.
A diagram is provided to help remember the process for determining limits, especially useful for identifying whether the limit approaches infinity, negative infinity, or does not exist.
The criteria for a function to be considered continuous are outlined, including the function being defined, the limit existing, and the limit being equal to the function value at that point.
A piecewise function's continuity is explored through examples, emphasizing the need to check the function value and limit at the transition points.
A polynomial function's continuity is discussed, noting that polynomials are continuous everywhere except where the denominator is zero in rational functions.
The concept of a vertical asymptote is explained, showing how functions can increase without bound as they approach a certain value, leading to discontinuity.
An example is given to find the value of K that makes a piecewise function continuous for all values of X, using the criteria of continuity to solve for K.
The importance of understanding the behavior of functions at discontinuities is highlighted, showing how to determine if a function is continuous at a given point.
The use of one-sided limits is explained in the context of piecewise functions, which is crucial for determining continuity at certain points.
A comprehensive approach to analyzing the continuity and limits of functions is presented, combining algebraic manipulation with graphical analysis.
The video concludes with a summary of key points, reinforcing the importance of understanding limits and continuity in calculus for both theoretical and practical applications.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: