Limits at infinity of quotients (Part 2) | Limits and continuity | AP Calculus AB | Khan Academy

Khan Academy
22 Jan 201304:33
EducationalLearning
32 Likes 10 Comments

TLDRThis educational script explores the concept of finding limits as x approaches infinity or negative infinity through several examples. It emphasizes understanding which terms dominate in a function as x grows in magnitude. For instance, in one case, the limit simplifies to 3 as x approaches infinity by focusing on the highest degree terms in both the numerator and denominator. Another example illustrates how a function approaches a horizontal asymptote of y=0 as x approaches negative infinity. Additionally, the script demonstrates how a function can become unbounded, highlighting the importance of the highest degree terms in determining the behavior of functions at infinity. This explanation aims to clarify the approach to limits, reinforcing the idea through multiple scenarios.

Takeaways
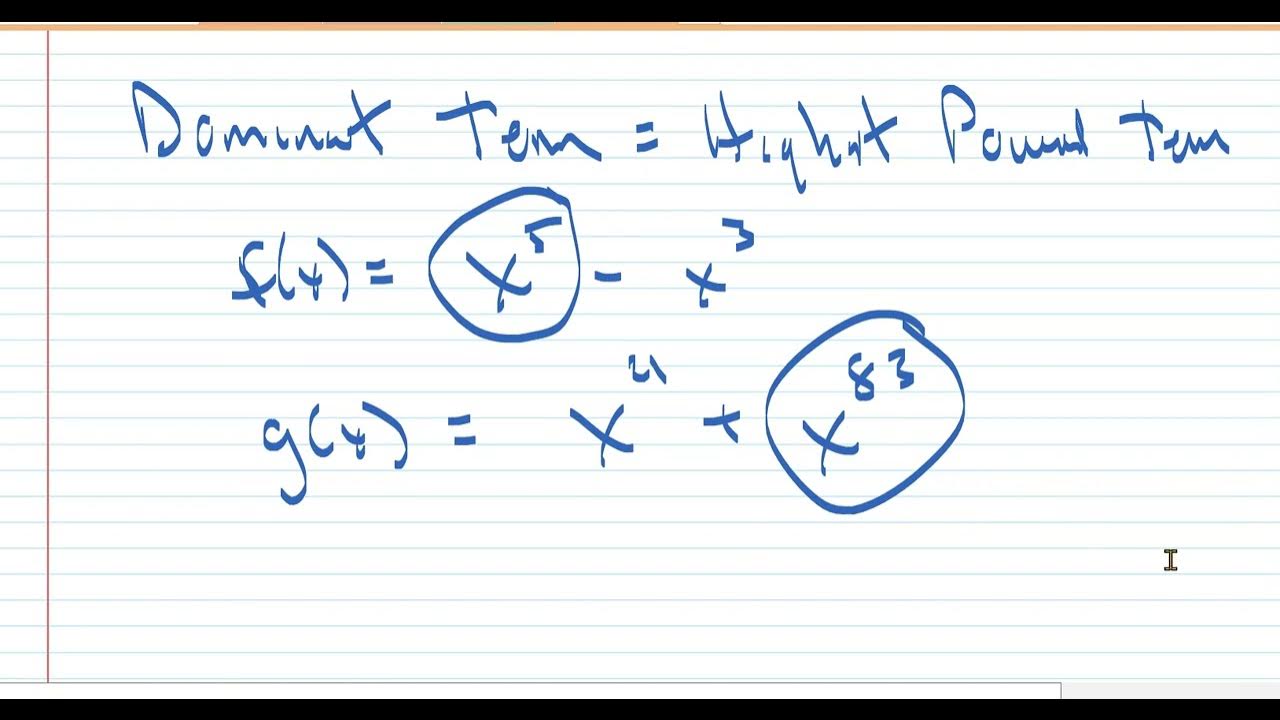
  • 🌟 When finding the limit of a function as x approaches infinity, identify the terms that will dominate the behavior of the function.
  • 🔢 For the function 9x^7 - 17x^6 + 15√x / (3x^7 + 1000x^5 - log2(x)), the dominant term in the numerator is 9x^7 and in the denominator is 3x^7, leading to a limit of 9/3, which is 3.
  • 📉 As x approaches negative infinity, the dominant terms change, resulting in a limit of 1/2x for the function 3x^3 / 6x^4, which simplifies to 0 as the absolute value of x grows larger.
  • 🚀 In the example with 4x^4 in the numerator and 250x^3 in the denominator, the limit as x approaches infinity is unbounded, or infinity, because the numerator grows faster than the denominator.
  • 🌐 The concept of limits at infinity can be applied to functions with various combinations of polynomial, radical, and logarithmic terms.
  • 📈 Understanding the behavior of functions at infinity or negative infinity can help in predicting the long-term behavior of the function without necessarily obtaining an exact numerical value.
  • 🧠 The process of simplification by canceling out common factors or terms is crucial in finding limits, as demonstrated with the first function where x^7 canceled out.
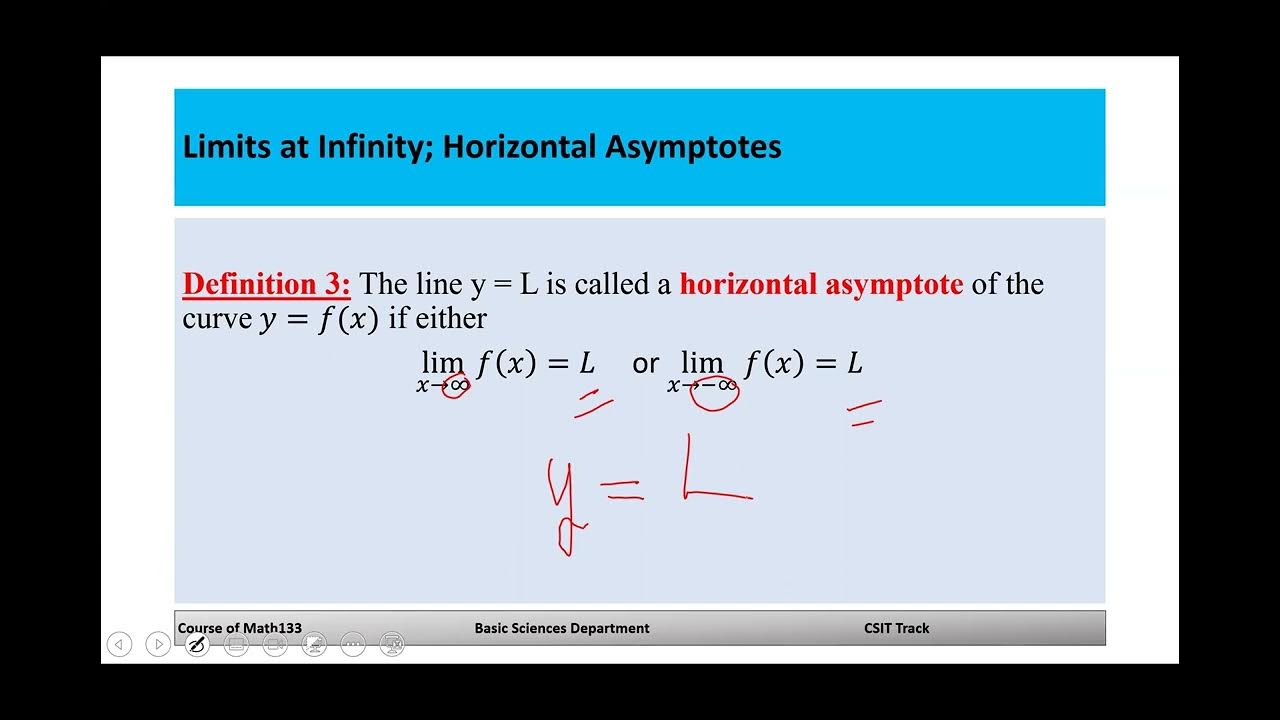
  • 📊 When graphing functions, identifying horizontal asymptotes (y = 0 in the second example) can provide insights into the behavior of the function as x approaches infinity or negative infinity.
  • 🔍 To verify the theoretical findings, it is encouraged to graph the functions or test them with specific values to see if the practical results align with the theoretical limits.
  • 📚 The principles of limits are fundamental in calculus and understanding them is essential for solving more complex problems and applications in various fields.
Q & A

  • What is the main concept discussed in the script?

    -The main concept discussed in the script is finding the limit of functions as x approaches infinity or negative infinity by identifying the dominating terms in the function.

  • How does the script illustrate the process of finding the limit as x approaches infinity?

    -The script illustrates the process by examining a complex function and determining which terms will grow the fastest as x increases without bound, thus becoming the dominating terms that dictate the limit.

  • What is the dominating term in the numerator of the first example function?

    -The dominating term in the numerator of the first example function is 9x to the seventh, as it grows faster than the other terms in the numerator.

  • What is the dominating term in the denominator of the first example function?

    -The dominating term in the denominator of the first example function is 3x to the seventh, as it grows faster than the other terms in the denominator, such as 1000x to the fifth and the log base 2 of x.

  • What is the limit of the first example function as x approaches infinity?

    -The limit of the first example function as x approaches infinity is 3, obtained by dividing the dominating term in the numerator (9x to the seventh) by the dominating term in the denominator (3x to the seventh) and canceling out the x to the seventh term.

  • How does the script explain the behavior of the function as x approaches negative infinity?

    -The script explains that the behavior of the function as x approaches negative infinity can be understood using the same principle of identifying dominating terms. It provides an example where the function approaches a horizontal asymptote, y equals 0, as the absolute value of x increases without bound.

  • What happens when the degree of the terms in the numerator is higher than the degree of the terms in the denominator?

    -When the degree of the terms in the numerator is higher than the degree of the terms in the denominator, the function will grow without bound as x approaches infinity, resulting in an unbounded limit, or infinity.

  • What is the limit of the function as x approaches infinity for the second example given in the script?

    -The limit of the second example function as x approaches infinity is infinity because the highest degree term in the numerator (4x to the fourth) is higher than the highest degree term in the denominator (250x to the third), leading to an unbounded function.

  • How can one verify the behavior of the function as x approaches infinity or negative infinity?

    -One can verify the behavior of the function by graphing it or plugging in values of x that are very large or very small, respectively, to observe the function's behavior and confirm the theoretical findings.

  • What is the significance of simplifying the problem by focusing on dominating terms?

    -Focusing on dominating terms simplifies the problem by allowing us to ignore the less significant terms that do not greatly affect the limit as x approaches very large or very small values. This makes the process of finding limits more manageable and less complex.

  • How does the script relate the concept of limits to the degrees of terms in a function?

    -The script relates the concept of limits to the degrees of terms by showing that the limit of a function as x approaches infinity or negative infinity is determined by the highest degree terms in the numerator and the denominator. The function will approach infinity or zero depending on whether the numerator's highest degree term grows faster or slower than the denominator's highest degree term.

Outlines
00:00
🌟 Understanding Limits at Infinity

This paragraph introduces the concept of finding limits of functions as x approaches positive or negative infinity. It emphasizes the importance of identifying the dominant terms in a function to determine its behavior at infinity. The example provided involves a complex function with various terms, including exponents and logarithms. The key takeaway is to focus on the fastest growing terms in the numerator and denominator to find the limit. As x approaches infinity, the function simplifies to the ratio of the highest power terms, resulting in a finite limit of 3. The discussion also extends to negative infinity, where the function approaches 0, highlighting the principles of limit analysis in calculus.

Mindmap
Keywords
💡limit
In the context of the video, 'limit' refers to the value that a function approaches as the input (x) gets arbitrarily large or small. It is a fundamental concept in calculus, used to describe the behavior of functions at their boundaries or as inputs tend towards infinity or negative infinity. The video script discusses finding limits by identifying the dominant terms in a function as x approaches these extreme values.
💡infinity
The term 'infinity' is used in the video to describe a concept that represents an unbounded quantity or value that is larger than any real number. It is employed when discussing the limits of functions, specifically when a function grows without bound as the input (x) approaches a very large or negative value. The script illustrates this by showing that certain functions tend towards infinity as x becomes infinitely large.
💡negative infinity
Similar to 'infinity,' 'negative infinity' refers to an idealized number representing a limit that is infinitely small or negative. In the video, this concept is used to analyze the behavior of functions as their input (x) approaches extremely negative values. The script explains that by identifying the dominant terms, one can determine that a function will tend towards zero when the negative infinity is approached, given the specific function's structure.
💡numerator
The 'numerator' is the top part of a fraction, which when divided by the denominator gives the value of the fraction. In the context of the video, the numerator is a part of the function that is being analyzed for its behavior as x approaches infinity or negative infinity. Dominant terms in the numerator can help determine the limit of the function.
💡denominator
The 'denominator' is the bottom part of a fraction that dictates the value of the fraction when divided by the numerator. In the video, the denominator, along with its dominant terms, is crucial for understanding how the function behaves as x approaches extreme values. The relationship between the numerator and the denominator determines whether the limit of the function is infinity, a finite number, or zero.
💡dominant terms
In the context of the video, 'dominant terms' refer to the parts of a function that grow or shrink at the fastest rate when the variable (x) approaches certain values, such as infinity or negative infinity. Identifying these terms is key to finding the limit of a function, as they dictate the function's behavior and ultimately its limit. The script provides examples of how to recognize and use dominant terms to simplify the process of finding limits.
💡asymptote
An 'asymptote' is a line that a function approaches but never actually reaches, typically as the input (x) becomes very large or very small. In the video, the concept of an asymptote is used to describe the behavior of a function when it tends towards a specific value (in this case, zero) as x approaches negative infinity. The horizontal asymptote is illustrated by the function's behavior when the denominator grows faster than the numerator.
💡degree of terms
The 'degree of terms' in a function refers to the highest power of the variable present in that term. In the video, the degree of terms is crucial for determining the limit of a function as x approaches infinity. The script explains that if the numerator has a higher degree than the denominator, the function will tend towards infinity, while a lower degree in the numerator compared to the denominator will result in a limit of zero.
💡logarithm
A 'logarithm' is the inverse operation to exponentiation, where the logarithm of a number is the exponent to which another fixed value, the base, must be raised to produce that number. In the video, the logarithm base 2 of x is a term in one of the functions discussed. The script implies that as x approaches infinity, the logarithmic term will grow slower compared to terms with higher powers of x and thus will not be a dominant term in determining the function's limit.
💡simplify
The term 'simplify' in the context of the video refers to the process of reducing a complex function to a more manageable form to make it easier to analyze or find its limit. Simplification often involves canceling out terms, reducing fractions, or focusing on the dominant terms of the function. The script emphasizes the importance of simplification in determining the behavior of functions as x approaches extreme values.
Highlights

The concept of finding limits of functions as x approaches infinity or negative infinity is discussed.

A crazy function with multiple terms is presented to demonstrate the concept.

The importance of identifying dominating terms in a function to determine its limit is emphasized.

For the numerator, 9x to the seventh is identified as the dominating term.

In the denominator, 3x to the seventh is the dominating term over 1000x to the fifth and log base 2 of x.

The limit as x approaches infinity for the given function is determined to be 3.

The process is repeated for a function as x approaches negative infinity.

At negative infinity, the dominating terms in the numerator and denominator are 3x to the third and 6x to the fourth, respectively.

The limit at negative infinity results in an approach to 0.

The concept of horizontal asymptote is introduced, with y equal to 0 being the horizontal asymptote in this case.

Another function is considered to find its limit as x approaches infinity.

The highest degree terms in the numerator and denominator are 4x to the fourth and 250x to the third.

The limit as x approaches infinity for this function is determined to be infinity due to the higher degree term in the numerator.

The relationship between the degrees of terms in the numerator and denominator and the limit of the function is discussed.

A function with a higher degree in the numerator than the denominator will approach infinity as x approaches infinity.

A function with a higher degree in the denominator than the numerator will approach 0 as x approaches infinity.

The method of simplifying complex limit problems by focusing on dominating terms is highlighted as useful.

Transcripts

Browse More Related Video

Limits at infinity of quotients (Part 1) | Limits and continuity | AP Calculus AB | Khan Academy

Calculus:Limits of Rational Functions

Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity

Computing Limits at Infinity for Rational Functions

Math 133 Lecture 2 6

Limits at infinity of quotients with square roots (even power) | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusLimitsInfinityDominant TermsFunction AnalysisMathematicsEducationCritical ThinkingSeries and SequencesHigher Degree Terms