How To Find The Limit At Infinity

The Organic Chemistry Tutor
9 Sept 201713:13
EducationalLearning
32 Likes 10 Comments

TLDRThis lesson delves into the concept of limits at infinity for various functions. It explains how to determine the limit as x approaches positive or negative infinity for functions like x squared, x cubed, and polynomials. The script also covers rational functions, discussing how to simplify them to find the limit and emphasizing that bottom-heavy functions approach zero while top-heavy functions approach infinity. Examples are provided to illustrate the process, including the method of dividing by higher powers of x to simplify and find the limit.

Takeaways
  • 🌟 The limit of a function as x approaches infinity can be determined by considering what happens to the function as x becomes very large.
  • 📈 For functions like x squared or x cubed, as x approaches infinity, the function also approaches infinity, either positive or negative depending on the sign of x.
  • 🔢 When dealing with polynomial functions, insignificant terms can be ignored when evaluating limits at infinity, focusing on the term with the highest degree.
  • 👉 For example, the limit of 5 plus 2x minus x cubed as x approaches negative infinity simplifies to the limit of negative x cubed, which is negative infinity.
  • 🤔 When evaluating limits of rational functions, if the degree of the numerator is less than the degree of the denominator, the limit as x approaches infinity is zero.
  • 📊 To find the limit of a rational function with the same degree in the numerator and denominator, divide the coefficients of the leading terms to find the limit.
  • 🛑 If a rational function is top heavy (numerator degree greater than denominator degree), the limit as x approaches infinity is the leading coefficient of the numerator times infinity.
  • 📈 For bottom-heavy rational functions, the limit can be found by multiplying the numerator and denominator by a power of x that makes the denominator approach zero, simplifying the expression.
  • 📚 When the highest degree terms in the numerator and denominator cancel each other out, the limit is the ratio of the remaining coefficients.
  • 🔍 To illustrate the process, the limit of 5x minus 7x cubed over 2x squared plus 14x cubed minus 9 as x approaches negative infinity is negative one half.
Q & A

  • What happens to the function x squared as x approaches infinity?

    -As x approaches infinity, the function x squared also approaches infinity, because squaring a large number results in an even larger number.

  • What is the limit of x squared as x approaches negative infinity?

    -The limit of x squared as x approaches negative infinity is also positive infinity, because squaring a negative number results in a positive value, and the principle is the same as for positive infinity.

  • How does the limit change for the function x cubed when x approaches infinity?

    -For positive x cubed, the limit as x approaches infinity is positive infinity, as cubing a large number results in an even larger positive number. However, for negative x cubed, the limit is negative infinity due to the odd power.

  • What is the limit of the expression 5 plus 2x minus x cubed as x approaches negative infinity?

    -The limit of the expression 5 plus 2x minus x cubed as x approaches negative infinity is positive infinity. The terms 5 and 2x are insignificant compared to the negative x cubed, which dominates the expression as x becomes very large.

  • How does the limit of a rational function with a higher degree in the numerator compare to the limit of a function with a higher degree in the denominator?

    -A rational function with a higher degree in the numerator (top heavy) will have a limit of infinity as x approaches infinity, whereas a function with a higher degree in the denominator (bottom heavy) will have a limit of zero.

  • What is the limit of one over x as x approaches infinity?

    -The limit of one over x as x approaches infinity is zero. As the denominator (x) becomes larger, the value of the fraction becomes smaller, approaching zero.

  • How can you find the limit of a rational function with the same degree in the numerator and denominator as x approaches infinity?

    -You can find the limit by dividing the coefficients of the highest degree terms in the numerator and denominator. The limit will be the ratio of these two coefficients.

  • What is the limit of the expression 5x minus 7x cubed over 2x squared plus 14x cubed minus 9 as x approaches negative infinity?

    -The limit of this expression as x approaches negative infinity is negative one half (-1/2). This is found by multiplying the numerator and denominator by one over x cubed and simplifying the expression.

  • How do you simplify a rational function to find its limit at infinity?

    -To simplify a rational function for the limit at infinity, you can eliminate insignificant terms and focus on the terms with the highest degree. For the limit at negative infinity, you can also multiply the numerator and denominator by a power of x that matches the highest degree term to simplify the expression.

  • What is the limit of the function 5x plus 6x squared divided by 3x minus 8 as x approaches infinity?

    -The limit of this function as x approaches infinity is positive infinity. The significant term in the numerator is 6x squared, which is more influential than 5x, and the significant term in the denominator is 3x, resulting in a ratio of 2x as x approaches infinity.

  • What happens to the limit of a rational function when the degree of the numerator is greater than the degree of the denominator?

    -When the degree of the numerator is greater than the degree of the denominator, the limit of the rational function as x approaches infinity is infinity. Conversely, if the degree of the denominator is greater, the limit is zero.

Outlines
00:00
🌌 Understanding Limits at Infinity

This paragraph introduces the concept of limits at infinity, specifically focusing on how to determine the limit of a function as x approaches positive or negative infinity. It explains the behavior of simple functions like x squared and x cubed as x becomes very large or very small, and how to handle polynomial and rational functions with different degrees in the numerator and denominator. The key takeaway is that the limit of a function at infinity depends on the highest degree term and whether the function is 'bottom heavy' (denominator has a higher degree) or 'top heavy' (numerator has a higher degree).

05:03
📚 Calculating Limits of Rational Functions

This section delves into the specifics of calculating limits for rational functions at infinity. It explains the process of simplifying expressions by focusing on the most significant terms and ignoring the insignificant ones. The paragraph provides a method for handling functions with the same degree in the numerator and denominator by dividing coefficients and for those with different degrees by multiplying the numerator and denominator by a power of x that matches the highest degree term. The summary emphasizes the importance of recognizing the degree of the terms and the behavior of the function as x approaches infinity or negative infinity.

10:07
🚀 Applying the Concepts to Complex Examples

The final paragraph applies the concepts learned to more complex examples of rational functions, demonstrating how to simplify expressions and determine the limit at infinity. It shows how to handle both 'bottom heavy' and 'top heavy' functions, providing a step-by-step approach to simplify the expressions and calculate the limits. The paragraph reinforces the idea that the limit behavior is determined by the most significant terms and the overall degree of the function, leading to conclusions of positive or negative infinity based on the function's structure.

Mindmap
Keywords
💡Limit at Infinity
The concept of a 'limit at infinity' refers to the value that a function or sequence approaches as the input (often denoted as 'x') tends towards an infinitely large or negative value. In the context of the video, this concept is used to determine what happens to functions like x squared or x cubed as x becomes infinitely large or negative. For example, as x approaches infinity for the function x squared, the value of the function also approaches infinity, because squaring a large number results in an even larger number.
💡Polynomial Function
A polynomial function is a mathematical function that involves a sum of powers of a variable multiplied by coefficients. The degree of the polynomial is the highest power of the variable in the function. In the video, the concept is used to analyze the behavior of polynomials at infinity, where higher-degree terms dominate the behavior of the function. For instance, when considering the expression 5 plus 2x minus x cubed, the term negative x cubed is more significant as x approaches infinity, and the other terms become insignificant.
💡Rational Function
A rational function is a function that is expressed as the quotient or fraction of two polynomial functions. The behavior of a rational function at infinity depends on the degrees of the numerator and the denominator. If the denominator's degree is higher (bottom heavy), the function approaches zero as the variable approaches infinity. The video provides examples of rational functions and how to determine their limits at infinity by either simplifying or multiplying the numerator and denominator by a suitable power of the variable to make the behavior clear.
💡Bottom Heavy
In the context of the video, 'bottom heavy' refers to a rational function where the denominator's degree is greater than the numerator's degree. As the variable approaches infinity, the value of such a function tends towards zero because the denominator grows faster than the numerator. The video illustrates this with examples like one over x, which approaches zero as x becomes infinitely large, because the denominator's growth in value outpaces the numerator's.
💡Top Heavy
Conversely, a 'top heavy' rational function is one where the numerator's degree is greater than or equal to the denominator's degree. In such cases, as the variable approaches infinity, the function tends towards a value determined by the ratio of the leading coefficients of the numerator and the denominator. For example, the video explains that a function like 5x plus 6x squared divided by 3x minus 8 simplifies to 2x as x approaches infinity, because the higher-degree terms in the numerator dominate.
💡Insignificant Terms
In the context of evaluating limits at infinity, 'insignificant terms' are those that do not affect the limit's value because they are less dominant compared to other terms in the function. When a function approaches infinity, higher-degree terms have a more significant impact on the function's value. In the video, it is explained that terms like 5 and 2x become insignificant when compared to a term like negative x cubed, which has a higher degree and thus a more substantial influence on the function's behavior as x approaches infinity.
💡Leading Coefficients
The leading coefficients are the coefficients of the term with the highest degree in a polynomial or rational function. These coefficients play a crucial role in determining the behavior of the function, especially when evaluating limits at infinity. If the function is top heavy, the ratio of the leading coefficients of the numerator and the denominator determines the limit as x approaches infinity. The video explains that for a function like 5 plus 2x minus 3x cubed over four x squared plus nine x minus seven, the leading coefficients of the numerator (-3) and the denominator (4) give the final answer of negative three-fourths times negative infinity, which simplifies to positive infinity.
💡Negative Infinity
Negative infinity is a concept used to describe a value that is infinitely small or less than any negative number. In the context of the video, it is used to illustrate the limit of functions that decrease without bound as the variable approaches negative or positive infinity. For example, when the video discusses the limit of x squared as x approaches negative infinity, it explains that the result is positive infinity because squaring a negative number results in a positive value, and as the magnitude of x increases, the value of x squared also increases without bound.
💡Simplifying Expressions
Simplifying expressions involves reducing mathematical expressions to their most straightforward form, often by canceling out common factors, combining like terms, or reducing powers. In the context of the video, simplifying is crucial for determining the limits of functions at infinity. By eliminating insignificant terms and focusing on the most dominant terms, one can more easily identify the behavior of the function as the variable becomes very large or very small.
💡Dominant Terms
Dominant terms in a polynomial or rational function are those with the highest powers that primarily influence the function's behavior, especially as the variable approaches extreme values like infinity or zero. In the video, dominant terms are used to determine the limit of a function at infinity. For example, when the degree of the numerator is higher than the denominator, the function's limit is determined by the leading term of the numerator, as seen when discussing the limit of 5x plus 6x squared divided by 3x minus 8, where the 6x squared term is dominant.
Highlights

The limit of a function as x approaches infinity can be determined by understanding how the function behaves with very large values of x.

For the function x squared, as x approaches infinity, the value also approaches infinity, indicating that the limit is positive infinity.

Similarly, the limit of x squared as x approaches negative infinity is also positive infinity, since squaring a negative number results in a positive value.

For the function x cubed, the sign of the limit depends on whether x is approaching from the positive or negative side, resulting in positive or negative infinity, respectively.

When dealing with polynomial functions, insignificant terms can be ignored when determining limits at infinity, focusing on the term with the highest degree.

The limit of an expression like 5 plus 2x minus x cubed as x approaches negative infinity simplifies to negative infinity, as the dominant term is negative x cubed.

For rational functions, when the degree of the denominator exceeds the degree of the numerator, the limit as x approaches infinity is zero.

An example of a rational function limit is one over x, which approaches zero as x approaches infinity because the denominator grows without bound.

To find the limit of a rational function with a bottom-heavy numerator and denominator, you can multiply the top and bottom by a power of x that corresponds to the degree of the denominator.

When the degrees of the numerator and denominator are the same, the limit can be found by dividing the coefficients of the highest degree terms.

For a rational function with a top-heavy numerator and denominator, you can simplify the expression by removing insignificant terms and focusing on the most significant terms.

The limit of a function with a top-heavy rational expression as x approaches infinity is determined by the most significant term in the numerator divided by the most significant term in the denominator.

In the case of a rational function with the same degree in the numerator and denominator, multiplying the numerator and denominator by a suitable power of x can help in simplifying the expression to find the limit.

When the numerator is heavier than the denominator in a rational function, the limit as x approaches infinity is positive infinity.

For rational functions with the same degree in the numerator and denominator, the limit can be found by simplifying the expression and considering the highest degree terms.

The limit of a rational function can also be found by evaluating the expression with a very large value of x to see the behavior of the function.

Understanding the behavior of polynomial and rational functions at infinity is crucial for analyzing their limits and can be done by focusing on the highest degree terms.

Transcripts

Browse More Related Video

Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity

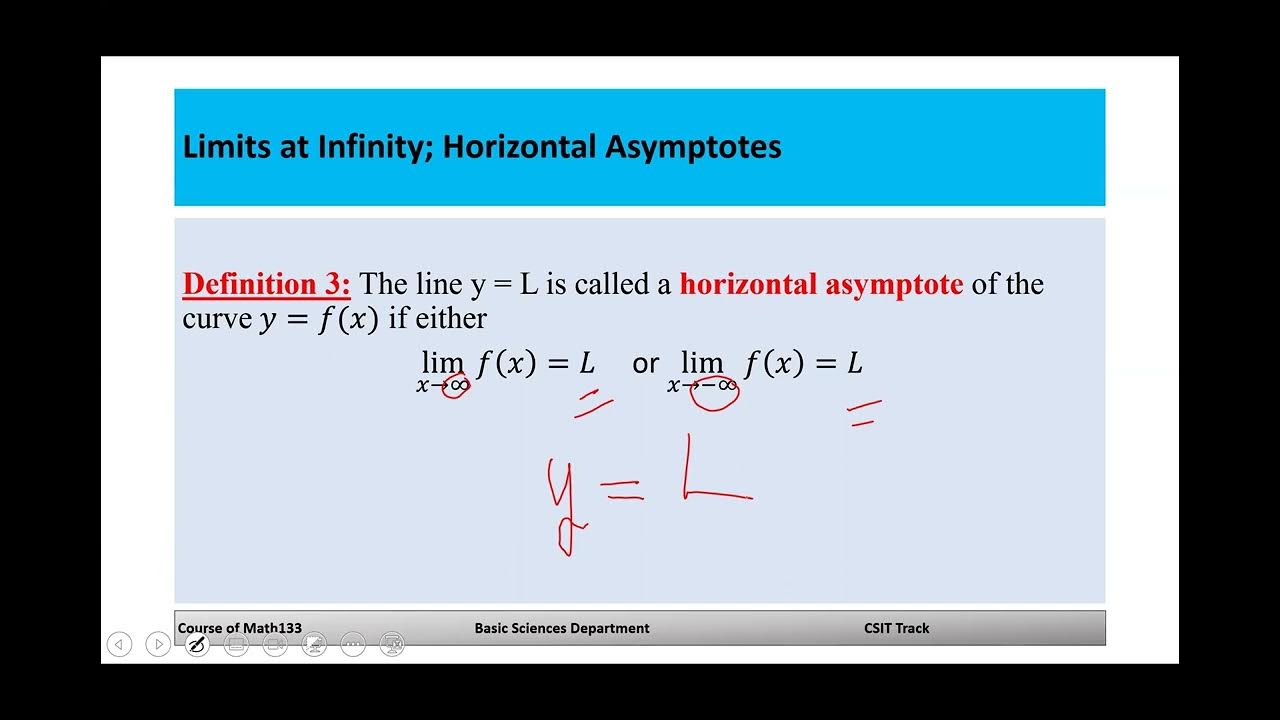
Math 133 Lecture 2 6

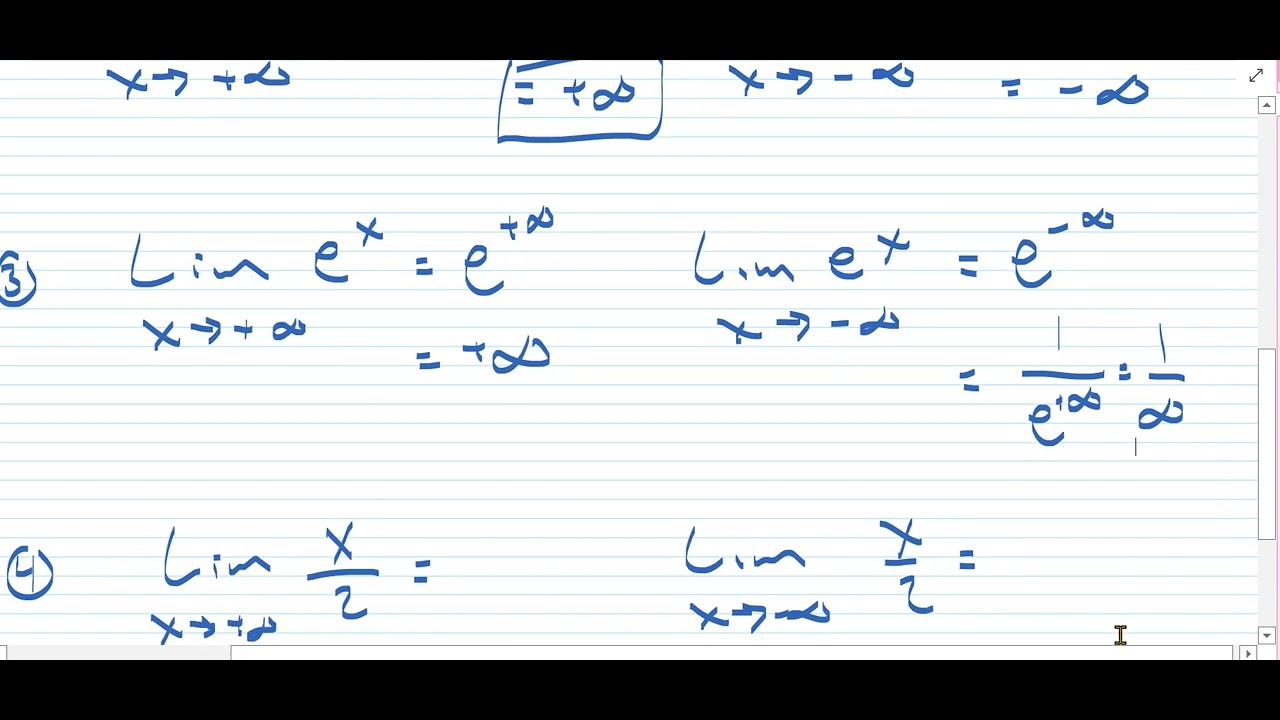
Calculus: Limits at Infinity-Algebraic

Finding limits at infinity using squeeze theorem | Squeeze or Sandwich Theorem - Calculus

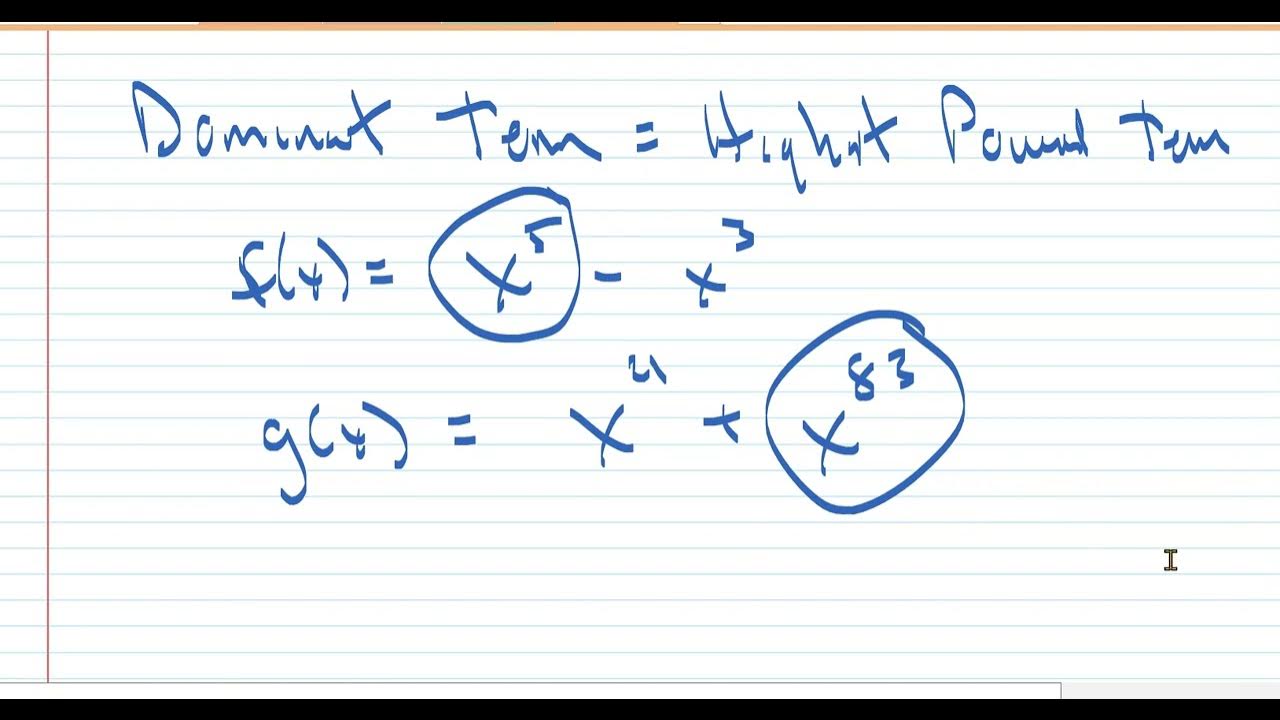
Calculus:Limits of Rational Functions

Introduction to infinite limits | Limits and continuity | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusLimitsInfinityPolynomialsRational FunctionsEducationLearningAlgebraFunctions