Calculus: Limits at Infinity-Algebraic

Sun Surfer Math

1 Feb 202206:13

EducationalLearning

32 Likes 10 Comments

TLDRThe video script presents an algebraic exploration of limits at infinity, contrasting with the traditional graphical approach. It begins with the concept of direct substitution, illustrating how multiplying infinity by a constant or adding a finite number to infinity yields infinity. The script further explains that exponential functions like e^x tend to positive infinity as x approaches positive infinity and to zero as x approaches negative infinity due to the reciprocal relationship. The video also touches on the behavior of limits involving division by a constant and the intricacies of limits of rational functions, where the rates of growth of the numerator and denominator determine the limit. The presenter encourages viewers to watch another video for a deeper understanding of limits of rational functions.

Takeaways

📚 Direct substitution is used to find limits at infinity algebraically.
🔢 Multiplying infinity by a constant (like 3) results in infinity, regardless of the direction (positive or negative).
➕ Adding a constant to infinity (like +5) retains the infinity value, with the sign of the infinity determining the result's sign.
📈 Exponential functions like e^x approach positive infinity as x approaches positive infinity.
📉 Raising e to a negative power results in 1 over e to the positive power, which approaches zero as the exponent becomes more negative.
💹 When the numerator of a fraction grows without bound and the denominator remains constant, the limit is infinity.
⤵️ Conversely, if the denominator grows without bound while the numerator remains constant, the limit is zero.
🔁 The direction (positive or negative) of infinity does not change the outcome for addition or multiplication by a constant.
🔄 Infinity minus infinity is not defined as zero; the rate at which the terms approach infinity matters.
🧮 To find the limit of rational functions where both numerator and denominator approach infinity, further analysis is required.
⏸ At the point where the denominator approaches infinity and the numerator does not, the limit of the function is zero.

Q & A

What is the algebraic approach to finding limits at infinity?
-The algebraic approach to finding limits at infinity involves direct substitution of infinity into the function and applying rules for operations with infinity, such as multiplication and addition.
What is the result when you multiply infinity by a constant, like 3?
-When you multiply infinity by a constant, the result is still infinity, regardless of whether the constant is positive or negative.
How does the sign affect the result when multiplying infinity by a negative number?
-Multiplying a positive infinity by a negative number results in negative infinity, as the rules for signs still apply.
What is the limit of x plus a constant (like 5) as x approaches positive infinity?
-The limit is still positive infinity because adding a finite number to infinity does not change its value.
What happens to the limit when x approaches negative infinity and you add 5 to it?
-The limit remains negative infinity because negative infinity is considered larger in magnitude than any finite number, thus retaining the negative sign.
What is the limit of e to the power of x as x approaches positive infinity?
-The limit is positive infinity, as the exponential function grows without bound in the positive direction.
How do you find the limit of e to the power of x as x approaches negative infinity?
-You rewrite e to the power of negative infinity as 1 over e to the power of positive infinity, which approaches zero because the denominator grows infinitely large.
What is the limit of a function where the numerator grows infinitely large and the denominator is a constant?
-The limit approaches infinity because the numerator's growth outpaces the constant denominator.
What is the limit of a function where the numerator is constant and the denominator grows infinitely large?
-The limit approaches zero because the constant numerator is dwarfed by the infinitely increasing denominator.
What is the correct approach to find the limit of a rational function where both the numerator and the denominator approach infinity at different rates?
-To find the limit of a rational function with both approaching infinity at different rates, one should watch a video on finding limits of rational functions for a detailed explanation and method.
Why might the result of infinity minus infinity not be zero?
-Infinity minus infinity might not be zero because the terms approaching infinity can have different rates of growth, which would affect the final result.
What is the general rule for adding or subtracting infinity by a finite number?
-The general rule is that adding or subtracting a finite number from infinity will not change its value; it remains infinity, with the sign determined by the direction of infinity (positive or negative).

Outlines

00:00

📚 Understanding Limits at Infinity: Algebraic Approach

This paragraph introduces the concept of limits at infinity from an algebraic perspective, contrasting it with the graphical approach previously discussed. The video demonstrates direct substitution to evaluate limits as x approaches positive or negative infinity. It covers scenarios such as multiplying infinity by a constant, adding a constant to infinity, and the behavior of exponential functions like e^x as x grows without bound. The explanation clarifies that multiplying infinity by a number or adding a number to infinity retains the sign and nature of infinity, while raising a number to the power of infinity results in positive infinity, and the inverse results in zero. The paragraph concludes with examples of limits involving exponential and polynomial functions.

05:02

🔍 Deep Dive into Limits of Rational Functions

The second paragraph delves into the specifics of calculating limits of rational functions as x approaches infinity. It addresses the common misconception that infinity minus infinity equals zero, explaining that the terms may approach infinity at different rates, thus the result is not straightforward. The paragraph encourages viewers to watch a dedicated video for understanding how to find limits of rational functions, which would provide the method to resolve such indeterminate forms and determine the actual limit of expressions like 2x^2 - 3x as x tends to infinity.

Mindmap

Keywords

💡Limits at Infinity

Limits at Infinity refer to the behavior of a function as its input (x) approaches an infinite value. In the video, the concept is approached algebraically rather than graphically, which is a common method to understand how a function behaves without necessarily plotting it. For instance, when x approaches positive or negative infinity, the behavior of expressions like 3x or x^2 is discussed.

💡Direct Substitution

Direct Substitution is a technique used to evaluate limits by substituting the value that the variable approaches into the function. It is a straightforward method that works well when the function is continuous at the point of interest. In the script, the presenter uses direct substitution to find the limit of 3x as x approaches infinity, resulting in infinity.

💡Positive Infinity

Positive Infinity is a concept that represents an unbounded quantity that grows without limit in the positive direction. It is used in calculus to describe the behavior of functions that increase indefinitely. In the video, the presenter substitutes positive infinity for x in expressions to determine their behavior as x grows without bound.

💡Negative Infinity

Negative Infinity, similar to Positive Infinity, represents an unbounded quantity that decreases without limit in the negative direction. It is used to describe the behavior of functions that decrease indefinitely. The video discusses what happens when you multiply or add to negative infinity, maintaining the negative direction of infinity.

💡Exponential Functions

Exponential Functions are mathematical functions where the variable is in the exponent. They are often written as e^x, where e is the base of the natural logarithm. The video explains that e^(positive infinity) tends towards positive infinity, while e^(negative infinity) approaches zero, demonstrating the explosive growth and rapid decay of exponential functions.

💡Exponent Rules

Exponent Rules are the mathematical principles governing operations on exponents, such as multiplication, division, and raising to a power. In the context of the video, the presenter uses exponent rules to transform e^(negative infinity) into 1/e^(positive infinity), which helps in evaluating the limit as it approaches zero.

💡Rational Functions

Rational Functions are functions that can be expressed as the quotient of two polynomials. The video hints at a separate discussion on finding limits of rational functions, which is important when the numerator and denominator both tend towards infinity at different rates, as in the case of 2x^2 - 3x as x approaches infinity.

💡Infinity Minus Infinity

Infinity Minus Infinity is a concept that arises when two expressions that both tend towards infinity are subtracted from one another. The video clarifies that this does not necessarily result in zero and that the rates at which the terms grow towards infinity must be considered, which is a topic for a different video.

💡Approaching Zero

Approaching Zero refers to a function's behavior where the output of the function gets arbitrarily close to zero as the input (x) tends towards a certain value. In the video, this is demonstrated with the limit of 1/x as x approaches infinity, where the function's value decreases without bound, getting infinitely close to zero.

💡Algebraic Approach

An Algebraic Approach involves using algebraic manipulation and substitution to solve mathematical problems. In the context of the video, the presenter uses an algebraic approach to evaluate limits at infinity by substituting infinity for x and performing operations to determine the resulting behavior of the function.

💡Graphical Approach

A Graphical Approach involves using visual representations, such as graphs or plots, to understand the behavior of functions. The video mentions that a graphical approach has been used previously but focuses on an algebraic method for this particular lesson, emphasizing a different way to conceptualize limits at infinity.

Highlights

The video discusses limits at infinity using an algebraic approach instead of a graphical one.

Direct substitution is used to evaluate limits as x approaches infinity.

When x approaches positive infinity, 3 times x equals positive infinity.

Approaching negative infinity results in negative infinity when multiplied by 3.

Adding a constant to infinity, such as 5, results in the same infinity.

Exponential functions, like e^x, approach positive infinity as x grows infinitely large in a positive direction.

Exponential functions approach zero when x approaches negative infinity due to the reciprocal nature.

A large numerator divided by a constant, like 2, approaches positive infinity.

A negative large numerator divided by a constant approaches negative infinity.

A constant divided by an infinitely large denominator approaches zero.

For rational functions, the rate at which the numerator and denominator grow towards infinity affects the limit.

The video suggests watching another video for limits of rational functions to understand when infinity minus infinity is not zero.

The concept of infinity and its mathematical treatment is explored with various algebraic examples.

Understanding the behavior of limits at infinity is crucial for advanced algebraic and calculus concepts.

The video provides a clear explanation of how to algebraically handle different scenarios involving infinity.

The algebraic approach to limits at infinity is contrasted with a graphical approach previously discussed.

The video emphasizes the importance of the rate of growth when dealing with limits involving infinity.

The video concludes with a teaser for further learning on limits of rational functions.

Transcripts

Browse More Related Video

Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity

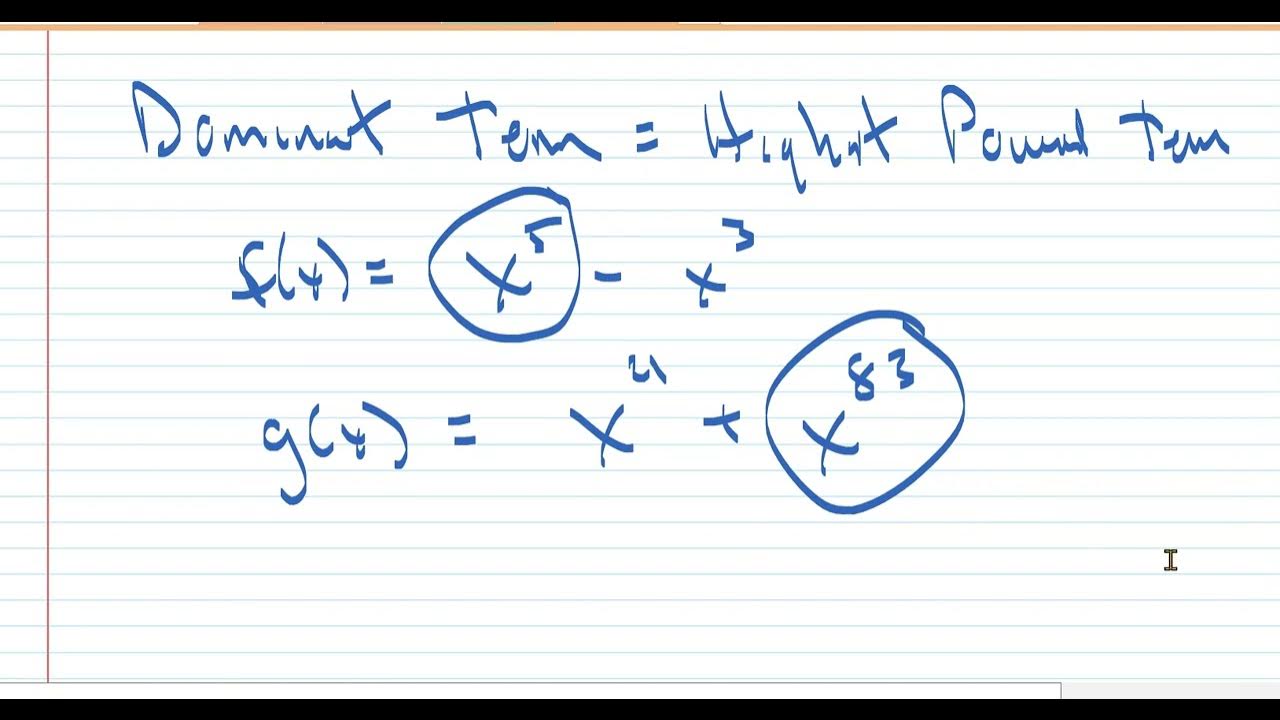
Calculus:Limits of Rational Functions

Limits at Infinity & Horizontal Asymptotes

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes

Finding limits at infinity using squeeze theorem | Squeeze or Sandwich Theorem - Calculus

How To Find The Limit At Infinity

Calculus: Limits at Infinity-Algebraic

Takeaways

Q & A

What is the algebraic approach to finding limits at infinity?

What is the result when you multiply infinity by a constant, like 3?

How does the sign affect the result when multiplying infinity by a negative number?

What is the limit of x plus a constant (like 5) as x approaches positive infinity?

What happens to the limit when x approaches negative infinity and you add 5 to it?

What is the limit of e to the power of x as x approaches positive infinity?

How do you find the limit of e to the power of x as x approaches negative infinity?

What is the limit of a function where the numerator grows infinitely large and the denominator is a constant?

What is the limit of a function where the numerator is constant and the denominator grows infinitely large?

What is the correct approach to find the limit of a rational function where both the numerator and the denominator approach infinity at different rates?

Why might the result of infinity minus infinity not be zero?

What is the general rule for adding or subtracting infinity by a finite number?