Calculus:Limits of Rational Functions
TLDRThis video script delves into the concept of limits of rational functions, particularly focusing on understanding them algebraically rather than graphically. It begins by contrasting the graphical approach to limits with an algebraic one, using the example of a function that tends towards infinity as x values increase or decrease without bound. The script then explores how different powers of x (x, x^2, x^3) behave as x approaches positive infinity, emphasizing that higher powers grow more rapidly. The key takeaway is identifying the dominant term in a rational function, which is the highest power of x present, as it dictates the function's behavior towards infinity. Several examples are provided to illustrate how to calculate limits by focusing on the dominant terms in both the numerator and the denominator. The script concludes by reinforcing the algebraic method's utility in determining the behavior of rational functions at infinity, offering a clear and engaging explanation suitable for viewers interested in calculus and mathematical analysis.
Takeaways
- 📈 The limit of a function as x approaches positive infinity can be determined by considering the behavior of the function as x becomes very large.
- 📉 When x approaches negative infinity, the function values tend towards negative infinity, indicating the function's behavior as x becomes very small.
- 🔢 Direct substitution can be used to find the limit of a function as x approaches infinity, but it's important to consider the growth rate of terms.
- 📊 Graphs can visually represent how a function's values increase or decrease towards positive or negative infinity.
- ⏱ As x grows without bound, polynomial terms with higher exponents grow faster than those with lower exponents.
- 🌟 The dominant term in a rational function is the highest powered term, which will determine the function's behavior as x approaches infinity.
- ➗ When finding limits of rational functions, we can often ignore lower order terms and focus on the dominant terms in the numerator and denominator.
- 🚫 Infinity minus infinity does not equal zero; it results in either positive or negative infinity, depending on the specific terms involved.
- ➗ Subtracting two terms that both approach infinity can result in a limit that is either positive or negative infinity, depending on their relative growth rates.
- 📖 Understanding the growth rate of terms is crucial for analyzing rational functions and their limits as x approaches infinity or negative infinity.
- ∞ The limit of a constant divided by an infinitely large term (approaching infinity) is zero, highlighting the behavior of rational functions as the denominator grows large.
Q & A
How does the limit of a rational function change when viewed from an algebraic perspective compared to a graphical perspective?
-From an algebraic perspective, the limit of a rational function is determined by identifying the dominant term in the numerator and denominator as x approaches a certain value, such as positive or negative infinity. This approach focuses on the behavior of the function as x grows without bounds, rather than its graphical representation.
What is the limit of the function f(x) as x approaches positive infinity if the function is represented by x^3 - x^2?
-The limit of the function f(x) as x approaches positive infinity for the expression x^3 - x^2 is positive infinity. This is because x^3 is the dominant term and it grows towards positive infinity faster than x^2.
How does the growth rate of x^n (where n is a positive integer) change as the exponent 'n' increases?
-As the exponent 'n' increases, the function x^n grows more quickly towards positive infinity. This is because higher exponents result in a faster rate of growth for the function as x becomes larger.
What is the dominant term in a rational function, and why is it important?
-The dominant term in a rational function is the term with the highest power of x in both the numerator and the denominator. It is important because as x approaches positive or negative infinity, the dominant term will determine the behavior of the function, overpowering all other terms.
How does the limit of a rational function change if the numerator and denominator have different dominant terms?
-If the numerator and denominator have different dominant terms, the limit of the rational function as x approaches positive or negative infinity will be determined by the ratio of these dominant terms. The limit will approach a constant value, positive infinity, negative infinity, or may tend towards zero, depending on the specific powers of the dominant terms.
What is the limit of x as x approaches positive infinity?
-The limit of x as x approaches positive infinity is positive infinity. This is because as x increases without bound, the function values also increase without limit towards positive infinity.
What is the limit of x^2 as x approaches positive infinity?
-The limit of x^2 as x approaches positive infinity is also positive infinity. This is because squaring a number that is increasing without bound results in an even larger number, also tending towards positive infinity.
What is the limit of x^3 as x approaches positive infinity?
-The limit of x^3 as x approaches positive infinity is positive infinity. Cubing a number that is increasing without bound results in a value that grows even faster towards positive infinity compared to x and x^2.
How does the concept of direct substitution apply to finding limits of functions as x approaches infinity?
-Direct substitution involves substituting the value that x is approaching (in this case, infinity) into the function to determine the limit. For functions like x, x^2, or x^3, direct substitution shows that as x grows without bound, the function values also grow without bound towards positive infinity.
What is the limit of the rational function (x^3 - x^2) / (x - 2) as x approaches positive infinity?
-The limit of the rational function (x^3 - x^2) / (x - 2) as x approaches positive infinity is positive infinity. This is because the dominant term in the numerator, x^3, grows much faster than the terms in the denominator, making the overall limit approach positive infinity.
What is the limit of the function x^5 as x approaches positive infinity?
-The limit of the function x^5 as x approaches positive infinity is positive infinity. This is consistent with the behavior of all functions of the form x^n (where n is a positive integer), which all tend towards positive infinity as x grows larger.
How does the limit of a rational function change if we consider x approaching negative infinity instead of positive infinity?
-The process of finding the limit as x approaches negative infinity is similar to that for positive infinity. The dominant term in the numerator and denominator will still determine the limit. However, the specific values and the behavior of the function may differ, leading to different limit values such as negative infinity or zero.
Outlines
📈 Understanding Limits of Rational Functions: Algebraic vs. Graphical
This paragraph introduces the concept of limits of rational functions, contrasting the algebraic approach with the graphical one. It explains how to determine the behavior of a function as x approaches positive or negative infinity by looking at the function's growth towards infinity. The paragraph also discusses how to identify the limit of basic polynomial functions (x, x^2, x^3) as x approaches positive infinity using direct substitution and how to compare their rates of growth.
📚 Dominant Terms in Rational Functions and Their Limits
The second paragraph delves into the importance of identifying dominant terms in rational functions when finding limits as x approaches infinity. It explains that the higher the exponent of the term, the faster the function grows towards infinity. The paragraph provides examples of rational functions and how to simplify them to their dominant terms to find the limit. It also illustrates the concept with the help of a graphing tool like Desmos to visualize the behavior of different terms.
🔍 Simplifying Rational Functions to Find Limits at Infinity
This paragraph focuses on the method of simplifying rational functions to their dominant terms to find the limit as x approaches positive infinity. It emphasizes that the highest powered term in the numerator and denominator is the dominant term, which will determine the limit of the function. The paragraph provides several examples of rational functions and demonstrates how to calculate their limits by focusing only on the dominant terms.
🔢 Limits at Infinity: Approaching Zero and Negative Infinity
The final paragraph discusses the behavior of limits as x approaches negative infinity, showing how to simplify expressions by canceling out terms and focusing on the dominant terms. It explains that when the denominator approaches negative infinity, the entire expression approaches zero. The paragraph provides an example of a rational function and demonstrates the process of finding its limit as x approaches negative infinity, resulting in the conclusion that the limit is zero.
Mindmap
Keywords
💡Rational Functions
💡Limits
💡Direct Substitution
💡Algebraic Perspective
💡Dominant Term
💡Positive Infinity
💡Negative Infinity
💡Exponential Growth
💡Graphical Perspective
💡Desmos
💡Subtracting Functions
Highlights
The video discusses the limits of rational functions from an algebraic perspective rather than a graphical one.
Positive x values approach positive infinity as you move further to the right on the x-axis.
Negative x values approach negative infinity as you move towards the left on the x-axis.
The function values increase without bound towards positive infinity as x approaches positive infinity.
The function values decrease without bound towards negative infinity as x approaches negative infinity.
Direct substitution can be used conceptually to find the limit as x approaches infinity for polynomials.
As x approaches positive infinity, x, x squared, and x cubed all tend towards positive infinity.
The rate of growth towards infinity is determined by the exponent; higher exponents grow faster.
X cubed grows faster towards infinity than x squared, which in turn grows faster than x.
When dealing with rational functions, the dominant term (highest power of x) is the most important for determining limits.
The limit of x cubed minus x squared over x, as x approaches positive infinity, is positive infinity.
Infinity minus infinity is not necessarily zero; it can be either positive or negative infinity.
The limit of a rational function can be found by looking at the dominant terms in the numerator and the denominator.
The limit of x squared minus x to the fifth over 2 x cubed, as x approaches positive infinity, is negative infinity.
The concept of dominant terms allows for simplification when finding limits of rational functions at infinity.
The limit of a constant over x as x approaches negative infinity is zero.
Understanding the behavior of functions at infinity is crucial for analyzing rational functions.
The video uses Desmos to visually demonstrate the behavior of different functions as they approach infinity.
Transcripts
Browse More Related Video

Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity

Limits at infinity of quotients (Part 2) | Limits and continuity | AP Calculus AB | Khan Academy
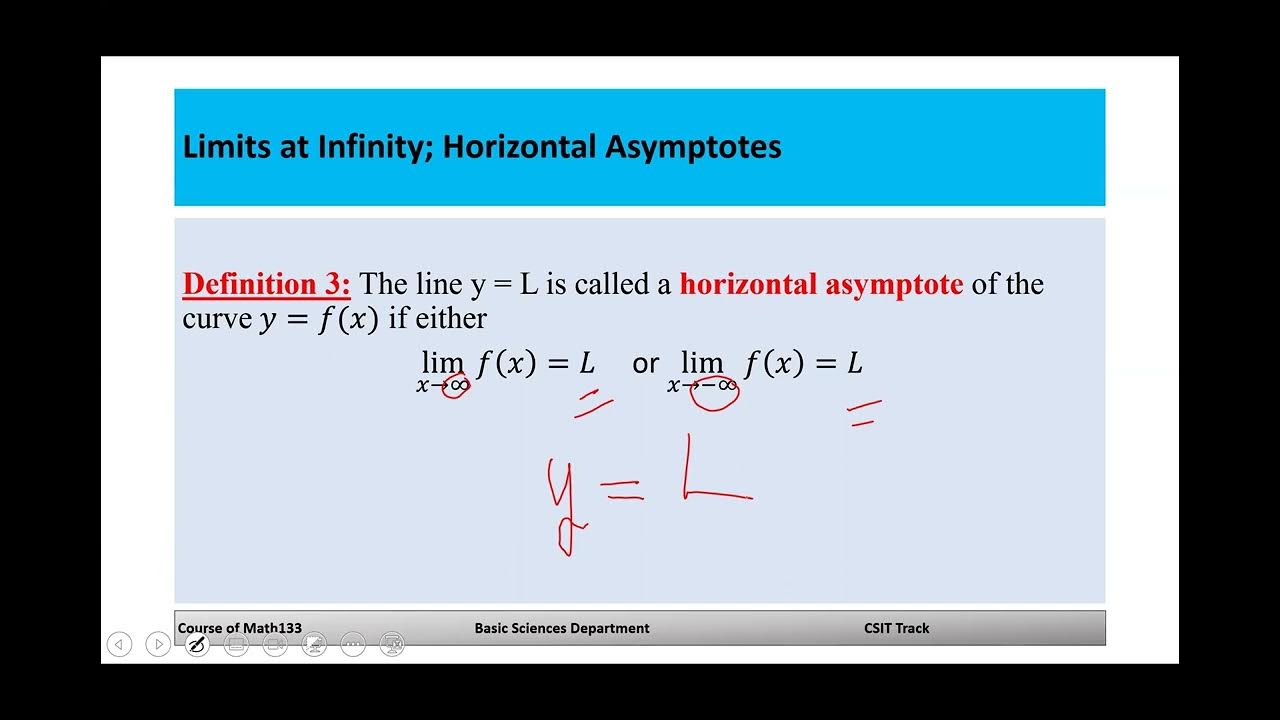
Math 133 Lecture 2 6
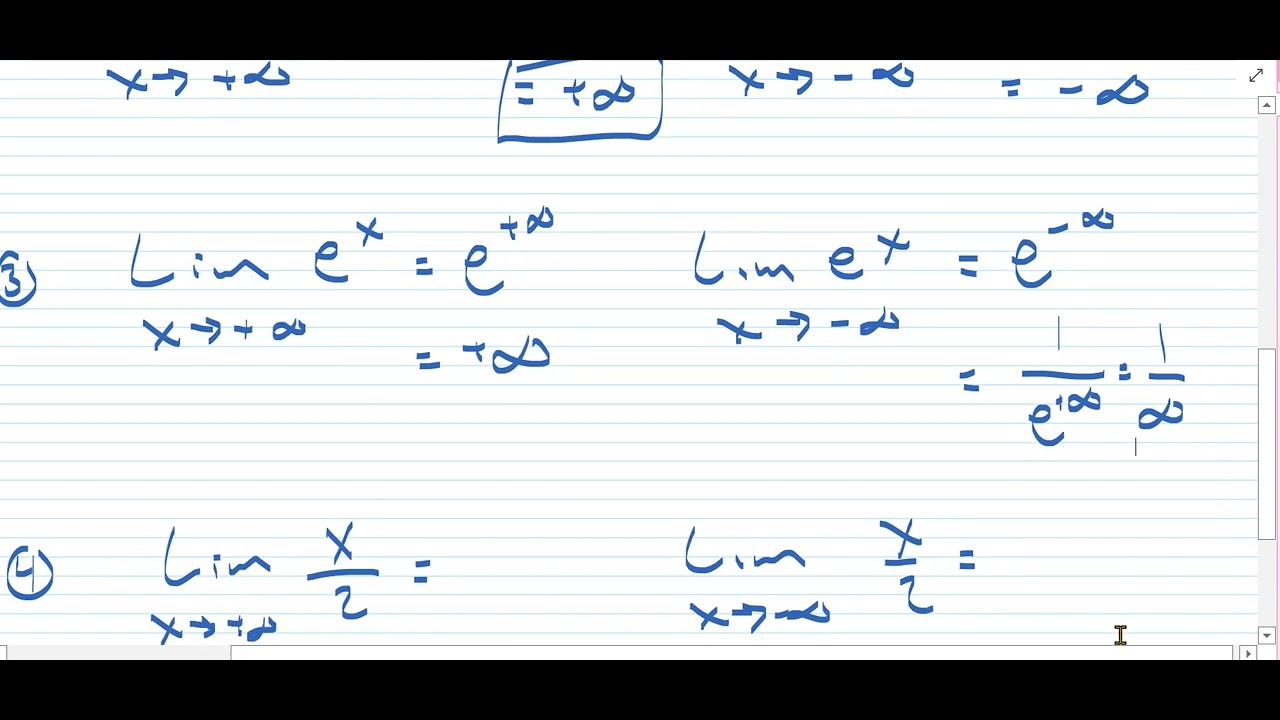
Calculus: Limits at Infinity-Algebraic

Computing Limits at Infinity for Rational Functions

Limits at infinity of quotients (Part 1) | Limits and continuity | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: