Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)

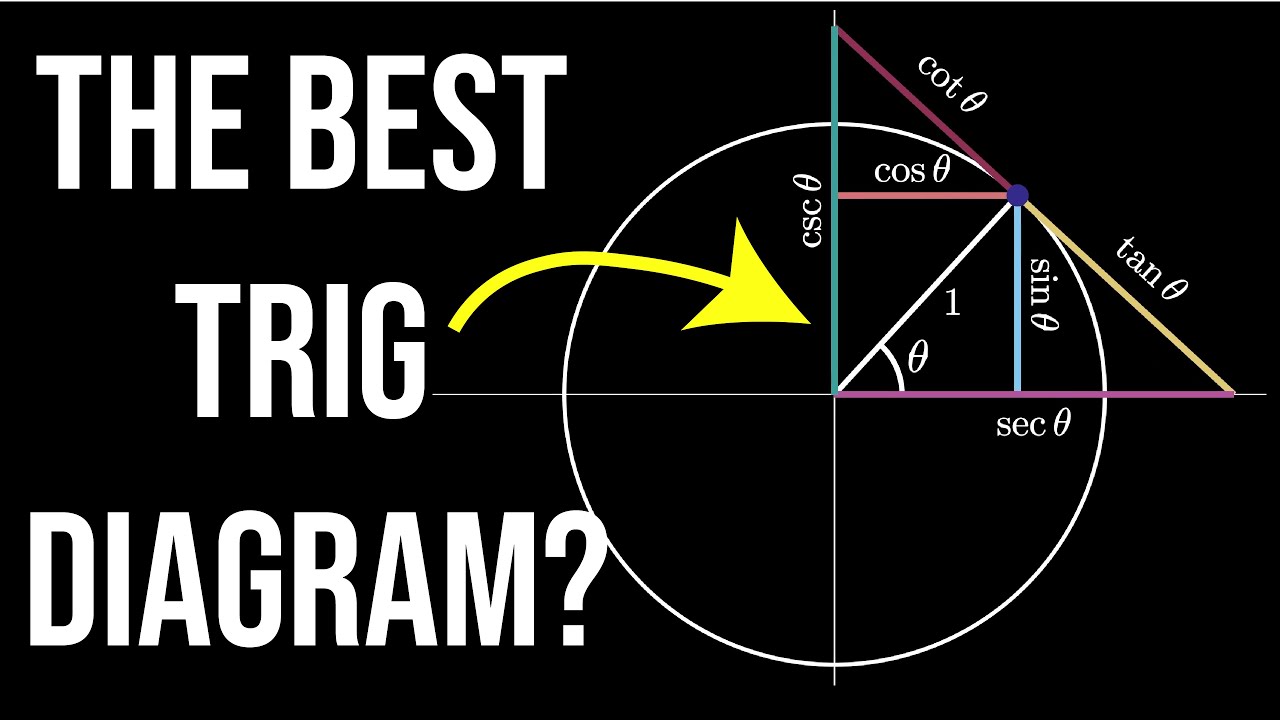
The video begins with an introduction to trigonometric functions, including sine, cosine, tangent, cosecant, secant, and cotangent. It emphasizes the interrelation of these functions and the concept of identities, which are mathematical relationships that allow for deeper understanding and extrapolation in trigonometry. The unit circle is introduced as a convenient tool for correlating angles with points on the circle, and thus with the trigonometric functions. Sine is related to the y-coordinate, and cosecant to its reciprocal, while cosine is associated with the x-coordinate, and secant with its reciprocal. Tangent and cotangent are defined in terms of ratios of these coordinates.

The second paragraph delves into the domain and range of the sine and cosine functions. The domain is the set of all possible input angles that yield a valid output, while the range is the set of all possible output values. For sine, the domain is all real numbers, as every angle results in a y-coordinate between -1 and 1, which is also the range. Similarly, cosine has a domain of all real numbers, with its range being the same interval on the x-axis. The importance of avoiding division by zero, which would result in an undefined value, is highlighted.

The third paragraph discusses the limitations in the domain of the cosecant and secant functions. Since cosecant is the reciprocal of sine, it is undefined where sine equals zero, which occurs at every multiple of pi. This leads to a domain that excludes these specific angles. The range of cosecant is all real numbers except for zero because the reciprocal of zero is undefined. The secant function, being the reciprocal of cosine, faces the same issue at every multiple of pi, leading to the same domain restrictions.

The fourth paragraph explores the domain and range of the tangent and cotangent functions. Tangent, being the ratio of y to x, is undefined where x equals zero, which is at pi/2 and 3pi/2 radians. This sets the domain of tangent to exclude these angles. The range of tangent is all real numbers because as x approaches zero, the ratio y/x can become infinitely large or small. Cotangent, the reciprocal of tangent, shares the same domain restrictions due to the same undefined nature at x equals zero.

The fifth paragraph explains the concept of periodicity in trigonometric functions. The period is the interval over which the outputs of the trigonometric functions repeat. For sine and cosine, the period is 2π, meaning their outputs repeat every 2π radians. For tangent, the period is π because it repeats every half rotation. The reciprocal functions, cosecant and secant, share the same period as their respective originals, sine and cosine, while cotangent has the same period as tangent.

The sixth paragraph demonstrates how to simplify angles that exceed one period of a trigonometric function using the concept of periodicity. By subtracting multiples of the period from the angle until the result lies within the fundamental interval of the unit circle, one can easily determine the value of the trigonometric function. This method is applicable to all trigonometric functions and is particularly useful for quickly finding the equivalent angle within the unit circle.

The seventh paragraph introduces a quick method for simplifying angles that involve more than one period of a trigonometric function. This method involves multiplying the denominator of the angle by the period of the respective trigonometric function and then finding the remainder of the numerator when divided by this product. The remainder becomes the new numerator, and the denominator remains the same, resulting in an equivalent angle on the unit circle.

The eighth paragraph discusses how to determine the quadrant of an angle based on the signs of the trigonometric functions. Since the signs of sine and cosecant are the same, as well as secant and cosine, and tangent and cotangent, one can deduce the quadrant by knowing the signs of these functions. For example, if the secant is negative and sine is positive, the angle must be in quadrant II. This understanding is crucial for applying trigonometric identities and solving problems involving angles.

The final paragraph teases the topic of reciprocal identities, which will be covered in the next video. It also provides a summary of the importance of understanding the interplay between the signs of trigonometric functions and their quadrants. The video concludes on a positive note, encouraging viewers to practice these concepts to solidify their understanding.