Trig 0.2 - Trig Functions Defined on the Unit Circle

Kimberly Brehm
19 Jun 202109:40
EducationalLearning
32 Likes 10 Comments

TLDRThe video script provides an insightful exploration into trigonometric functions on the unit circle, which is a circle with a radius of one centered at the origin. It explains how points on the unit circle satisfy the equation x^2 + y^2 = 1 and how trigonometric functions, such as sine, cosine, and tangent, relate to the coordinates of points on this circle. The script delves into the concept of standard position for angles and how it forms a right triangle, leading to the definition of sine as the y-coordinate over the hypotenuse (1), and cosine as the x-coordinate over the hypotenuse. It further clarifies the relationships between the reciprocal trigonometric functions. The video also demonstrates how to calculate the coordinates for specific angles like π/4 (45 degrees) using the properties of an isosceles right triangle. It emphasizes the utility of the unit circle in determining the values of trigonometric functions for any angle, including those not located on the circle, by using a calculator. The script concludes with a teaser for the next topic: the domains of the trigonometric functions.

Takeaways
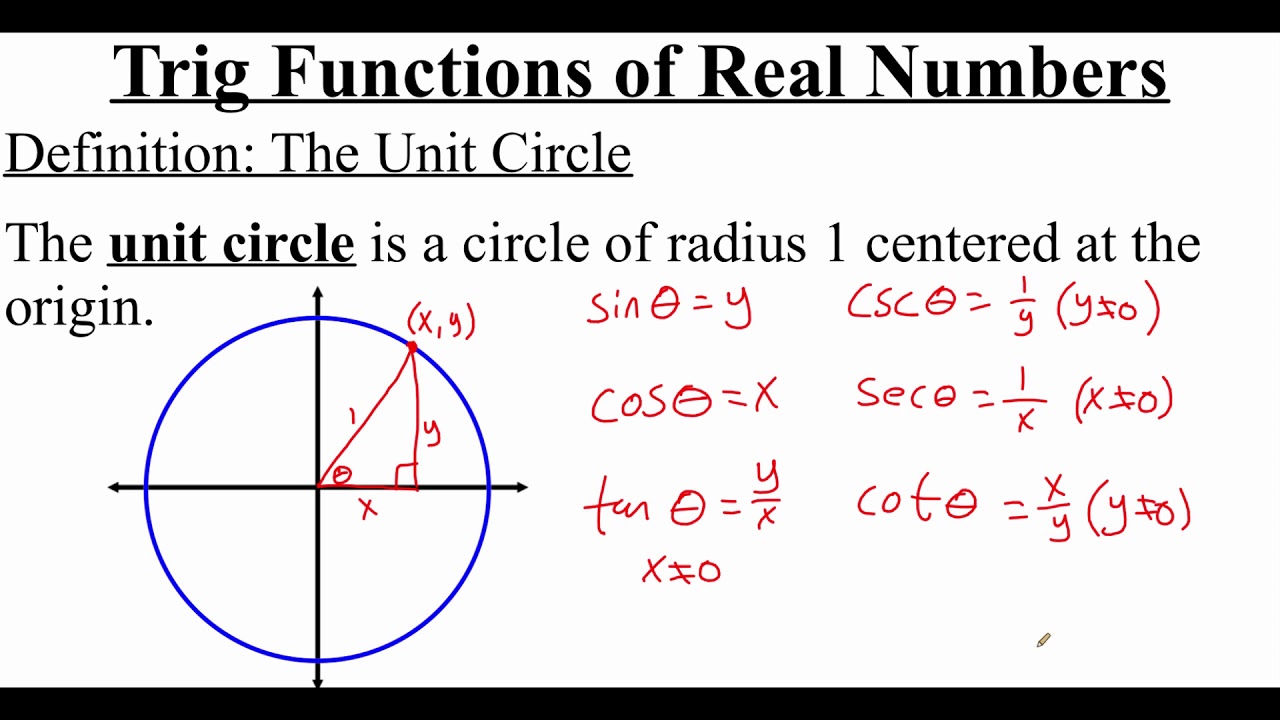
  • 📐 The unit circle is a circle with a radius of one, centered at the origin, where every point satisfies the equation x^2 + y^2 = 1.
  • 📍 Points on the unit circle can be represented as coordinates (cos(θ), sin(θ)), where θ is the angle formed.
  • 🔢 Sine of an angle (sin(θ)) is the y-coordinate of the point on the unit circle, and cosine (cos(θ)) is the x-coordinate.
  • 🤹 Tangent (tan(θ)) is the ratio of the y-coordinate to the x-coordinate, or sin(θ) / cos(θ).
  • 🔄 The reciprocal trigonometric functions, such as cosecant (csc(θ)) and secant (sec(θ)), are derived from the basic trigonometric functions.
  • 🧮 To find the coordinates for specific angles like π/4, one can use the properties of a 45-45-90 special right triangle, which simplifies to x = y on the unit circle.
  • ✅ The coordinates for π/4 are sqrt(2)/2, sqrt(2)/2 after rationalizing the denominator.
  • 🌐 The unit circle allows for quick evaluation of trigonometric functions for special angles without a calculator.
  • 🔄 For angles in different quadrants, the signs of the x and y coordinates change based on the quadrant, but the magnitudes remain the same.
  • 📊 To evaluate trigonometric functions for non-special angles, a calculator can be used, ensuring it is set to the correct mode (degrees or radians).
  • 📘 Understanding the unit circle and the relationships between the trigonometric functions and the coordinates of points on the circle is fundamental for evaluating these functions at various angles.
Q & A

  • What is the definition of the unit circle?

    -The unit circle is a circle with a radius of one, centered at the origin, where every point on the circle satisfies the equation x^2 + y^2 = 1.

  • How is the cosine of an angle on the unit circle defined?

    -The cosine of an angle (θ) on the unit circle is defined as the x-coordinate of the point where the terminal side of the angle intersects the circle.

  • What is the sine of an angle on the unit circle?

    -The sine of an angle (θ) on the unit circle is the y-coordinate of the point where the terminal side of the angle intersects the circle.

  • How do you find the tangent of an angle on the unit circle?

    -The tangent of an angle (θ) on the unit circle is found by taking the ratio of the y-coordinate to the x-coordinate of the point where the terminal side of the angle intersects the circle.

  • What are the reciprocal trigonometric functions?

    -The reciprocal trigonometric functions are cosecant (reciprocal of sine), secant (reciprocal of cosine), and cotangent (reciprocal of tangent).

  • How can you find the coordinates of a point on the unit circle for an angle of π/4?

    -For an angle of π/4, the coordinates on the unit circle are both equal to √2/2, resulting in the point (√2/2, √2/2), because it forms a 45-45-90 special right triangle.

  • What is the significance of the unit circle for evaluating trigonometric functions?

    -The unit circle allows for easy evaluation of trigonometric functions at special angles without the need for a calculator, by simply using the coordinates of the points where the angles intersect the circle.

  • How do the coordinates of points on the unit circle change as you move through different quadrants?

    -The coordinates change based on the quadrant: in the first quadrant both x and y are positive, in the second quadrant x is negative and y is positive, in the third quadrant both x and y are negative, and in the fourth quadrant x is positive and y is negative.

  • How can you find the value of sine for an angle of 5π/3?

    -For an angle of 5π/3, the sine value is the y-coordinate of the point on the unit circle, which is -√3/2.

  • What is the process for finding the value of a trigonometric function for a non-special angle?

    -For non-special angles, you would use a calculator, ensuring it is set to the correct mode (degrees or radians) for the given argument, and then input the function and the angle to find the value.

  • How can you find the value of the cosecant function for an angle of 5π/3 using a calculator?

    -Since cosecant is the reciprocal of sine, you would calculate it as 1 divided by the sine of the angle. For an angle of 5π/3, this would be 1 / (-√3/2), which equals -2.

  • What is the next topic to be discussed in the video series?

    -The next topic to be discussed is the domains of the trigonometric functions.

Outlines
00:00
📐 Understanding Trigonometric Functions on the Unit Circle

This paragraph introduces the concept of the unit circle, defined as a circle with a radius of one centered at the origin, satisfying the equation x^2 + y^2 = 1. The video explains how to use an angle in standard position to create a right triangle, which is then used to define trigonometric functions such as sine, cosine, and tangent in terms of the coordinates of points on the unit circle. The explanation covers how sine is the y-coordinate, cosine is the x-coordinate, and tangent is the ratio of the y-coordinate to the x-coordinate. It also touches on the reciprocal trigonometric functions like cosecant and secant. The paragraph concludes with an example of finding the coordinates for an angle of π/4 (45 degrees), which leads to the point (√2/2, √2/2) on the unit circle.

05:01
🧮 Evaluating Trigonometric Functions for Special Angles

The second paragraph delves into the values of trigonometric functions for special angles, specifically π/4, 3π/4, 5π/4, and 7π/4, highlighting that these angles share the same coordinate values but differ in their quadrant positions. The video demonstrates how to find the sine and secant for an angle of 5π/3 (300 degrees), which corresponds to the point (1/2, -√3/2) on the unit circle. It also shows how to calculate cosine and tangent for an angle of -5π/6 (-150 degrees), which is equivalent to 7π/6. The paragraph emphasizes the utility of the unit circle for evaluating trigonometric functions at special angles without the need for a calculator. Lastly, it mentions that for non-special angles, a calculator must be used, and it provides an example of how to use a calculator for such cases, including converting between degrees and radians.

Mindmap
Keywords
💡Unit Circle
The unit circle is a circle with a radius of one, centered at the origin of a coordinate system. It is fundamental in defining trigonometric functions for all angles, as every point on the unit circle can be described by coordinates (x, y) that satisfy the equation x^2 + y^2 = 1. In the video, the unit circle is used to define the trigonometric functions sine, cosine, and tangent in terms of the coordinates of points on the circle.
💡Trigonometric Functions
Trigonometric functions, such as sine, cosine, and tangent, are mathematical functions that relate the angles of a triangle to the lengths of its sides. In the context of the video, these functions are defined for any angle using the unit circle, with sine as the y-coordinate, cosine as the x-coordinate, and tangent as the ratio of the y-coordinate to the x-coordinate of a point on the circle.
💡Right Triangle
A right triangle is a triangle with one angle measuring 90 degrees. It is used in the video to explain how the trigonometric functions sine and cosine are derived from the sides of a triangle relative to an angle θ. Specifically, sine is the ratio of the length of the side opposite the angle to the hypotenuse, and cosine is the ratio of the length of the adjacent side to the hypotenuse.
💡Standard Position
Standard position refers to the way an angle is placed in a coordinate system for the purpose of trigonometric analysis. In the video, the initial ray of an angle in standard position lies along the positive x-axis, and the terminal ray extends from there to form the angle θ. This positioning is crucial for drawing a right triangle and applying trigonometric functions to it.
💡Sine
Sine is a trigonometric function that, in the context of a right triangle, represents the ratio of the length of the side opposite an angle to the length of the hypotenuse. In the video, sine is also defined for any angle on the unit circle as the y-coordinate of the point where the terminal side of the angle intersects the circle, making it a key function for evaluating angles.
💡Cosine
Cosine is another trigonometric function that corresponds to the ratio of the adjacent side to the hypotenuse in a right triangle. In the video, it is defined on the unit circle as the x-coordinate of the point where the terminal side of an angle intersects the circle, complementing the definition of sine.
💡Tangent
Tangent is a trigonometric function that represents the ratio of the sine to the cosine of an angle. It is the ratio of the opposite side to the adjacent side in a right triangle. In the video, the tangent is defined for any angle on the unit circle as the ratio of the y-coordinate to the x-coordinate of the point where the terminal side intersects the circle.
💡Cosecant, Secant, Cotangent
These are reciprocal trigonometric functions defined as the reciprocals of sine, cosine, and tangent, respectively. In the video, cosecant is mentioned as 1/sine, secant as 1/cosine, and cotangent as the reciprocal of tangent (1/tangent). These functions are used to find the angles when the side lengths of a triangle are known.
💡Special Angles
Special angles are angles that have common and easily remembered values for the trigonometric functions. In the video, angles such as π/4 (45 degrees), π/6 (30 degrees), and π/3 (60 degrees) are discussed as examples of special angles. These angles are used to find the coordinates on the unit circle and to evaluate trigonometric functions without a calculator.
💡Quadrants
Quadrants refer to the four equal regions that the coordinate plane is divided into by the two axes. In the video, the concept of quadrants is used to understand the signs of the x and y coordinates for points on the unit circle as the angle θ varies. For example, in the first quadrant, both x and y are positive, while in the second quadrant, x is negative and y is positive.
💡Calculator
A calculator is an electronic device used for performing arithmetic operations and computations. In the context of the video, a calculator is mentioned as a tool for evaluating trigonometric functions for angles that are not special angles on the unit circle. The video explains how to use a calculator in both degree and radian modes to find the values of cosine and other trigonometric functions.
Highlights

The unit circle is a circle of radius one centered at the origin where every point satisfies x^2 + y^2 = 1

Trig functions can be defined on the unit circle using the coordinates (cos θ, sin θ) of a point

Sine is the y-coordinate of a point on the unit circle, or opposite side divided by hypotenuse in a right triangle

Cosine is the x-coordinate of a point on the unit circle, or adjacent side divided by hypotenuse in a right triangle

Tangent is sine divided by cosine, or opposite side divided by adjacent side in a right triangle

Cosecant is the reciprocal of sine (1/sin), and secant is the reciprocal of cosine (1/cos)

Cotangent is the reciprocal of tangent (1/tan), or x/y flipped to y/x

For a 45 degree angle (π/4 radians), the x and y coordinates on the unit circle are equal

Using the Pythagorean theorem, the coordinates for π/4 are (√2/2, √2/2)

The coordinates for other quadrants can be found by memorizing the first quadrant and knowing the sign of x and y

The unit circle allows you to easily evaluate trig functions for special angles without a calculator

For angles outside the special angles, you can use a calculator in the correct degree or radian mode

Cosecant can be found as the reciprocal of sine (1/sin) using a calculator

The domains of the trig functions will be discussed in the next video

This video provides a great way to visually understand and evaluate trig functions for special angles on the unit circle

The unit circle connects the concepts of trig functions, right triangles, and angles in a geometric way

The video uses clear examples and illustrations to explain the concepts in an accessible manner

Understanding the unit circle is key to mastering trigonometry and evaluating trig functions

Transcripts

Browse More Related Video

4.3.4 Trigonometric Functions of Real Numbers

Trigonometric Functions and the Unit Circle (Precalculus - Trigonometry 6)

30-60-90 Triangles - Special Right Triangle Trigonometry

Trigonometry: Unit Circle

Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)

Unit Circle Definition of Trig Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryUnit CircleAnglesSine FunctionCosine FunctionTangent FunctionRight TriangleSpecial AnglesTrig FunctionsMathematicsEducational VideoCalculus