Solve related rates falling ladder
TLDRThe video script presents a mathematical problem involving a ladder falling against a wall. It emphasizes the importance of understanding the given information, visualizing the problem, and identifying the correct relationships and derivatives to solve for the rate at which the ladder moves when it reaches a certain distance from the wall. The solution employs the Pythagorean theorem and concepts of precalculus and geometry, ultimately deriving the ladder's speed as it approaches the friend standing six feet away.
Takeaways
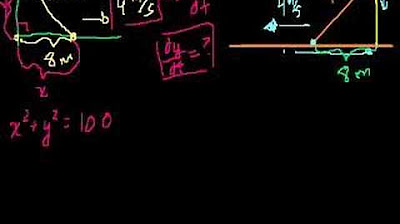
- 📌 The problem involves a ladder falling down a wall at a rate of four feet per second.
- 📏 The ladder is initially 10 feet long and leans against a wall with a friend standing six feet away from the wall.
- 🤔 The goal is to determine the speed at which the ladder is moving when it reaches the friend.
- 📐 The situation can be modeled as a right triangle problem, utilizing precalculus and geometry concepts.
- 🧐 The Pythagorean theorem is applicable since the ladder, the distance from the wall, and the distance the friend is standing form a right triangle.
- 📈 The rate of change (derivative) is important to find out, which in this case is the speed of the ladder as it moves away from the wall.
- 🔢 The derivative relationship can be expressed as 2x * dx/dt + 2y * dy/dt = 2z * dz/dt, where x, y, and z represent the distances along the wall, ground, and ladder's length respectively.
- 📌 To solve for the rate of change of x (dx/dt), it is necessary to know the values of x, y, and their derivatives with respect to time.
- 🤓 At the moment the ladder reaches the friend, x is 6 feet. Using the Pythagorean theorem, y can be determined to be 8 feet.
- 📉 Since the ladder is falling, the rate of change of y (dy/dt) is negative and equal to the rate of the ladder falling, which is -4 feet per second.
- 🔍 The final calculation for the rate of change of x (dx/dt) when the ladder is six feet from the wall is 16/3 feet per second, considering the negative direction of the ladder's movement.
Q & A
What is the scenario described in the transcript?
-The scenario involves a person standing at the top of a 10-foot ladder leaning against a wall. The ladder starts to fall down the wall at a rate of 4 feet per second, and the person's friend is standing 6 feet away from the wall. The goal is to determine the speed at which the ladder is moving when it reaches the friend.
Why is the ladder considered to be in a right triangle configuration?
-The ladder forms a right triangle with the wall and the ground because it is leaning against the wall and makes a right angle with the ground. This configuration allows the application of the Pythagorean theorem and the use of trigonometric functions to solve the problem.
What is the significance of identifying the right triangle in this problem?
-Identifying the right triangle is crucial because it enables the use of the Pythagorean theorem to relate the distances involved in the problem. It also allows for the application of trigonometric functions, which can provide additional methods to solve the problem.
What are the two distances that are changing when the ladder falls?
-The two distances that change are the vertical distance the ladder falls down the wall and the horizontal distance the base of the ladder moves away from the wall.
How is the rate of the ladder falling related to the derivative in the mathematical model?
-The rate at which the ladder is falling is equivalent to the derivative with respect to time of the vertical distance it covers. This derivative represents the instantaneous rate of change of the position of the ladder.
What does the equation 2x * dx/dt + 2y * dy/dt = 2z * dz/dt represent?
-This equation is a differential form of the Pythagorean theorem applied to the right triangle formed by the ladder, the wall, and the ground. It represents the relationship between the rates of change of the distances x, y, and z (the ladder's position along the wall, the ground, and its length, respectively).
Why is the length of the ladder (z) considered to be constant in this problem?
-The length of the ladder is considered constant because it does not change as the ladder falls. This is a given fact in the problem statement, and thus, dz/dt (the rate of change of the ladder's length) is zero.
What is the value of y when the ladder is 6 feet away from the wall?
-Using the Pythagorean theorem with the given values (x = 6 feet, z = 10 feet), we find that y (the height of the ladder on the wall) is 8 feet. This is calculated by solving 10^2 = 6^2 + y^2, which gives y^2 = 100 - 36, resulting in y = 8 feet.
How is the negative sign used in the equation to represent the direction of the ladder's movement?
-The negative sign is used to indicate that the ladder is moving in the downward direction along the wall. In mathematical terms, a negative value for a derivative often represents a decrease or movement in the negative direction of the axis.
What is the final calculation for the rate of change of x (dx/dt) when x is 6 feet?
-By solving the derived equation with the known values (12 * dx/dt - 64 = 0), we find that dx/dt equals 16/3 feet per second. This represents the speed at which the ladder is moving when it is 6 feet away from the wall.
Why is it important to include units in the final answer?
-Units are essential in the final answer because they provide context to the numerical value, indicating what physical quantity is being measured. In this case, the units would be feet per second, signifying the speed of the ladder's movement.
Outlines
🔍 Introduction to the Ladder Problem
This paragraph introduces a physics problem involving a ladder falling against a wall. The scenario describes a ladder leaning against a wall, with one person at the top and another friend standing six feet away from the wall. The ladder begins to fall at a rate of four feet per second, and the goal is to determine the speed at which the ladder moves when it reaches the friend. The discussion delves into the concept of distances changing over time and the importance of understanding the geometry of the situation, specifically highlighting the relevance of right triangles in solving the problem. The Pythagorean theorem and the potential use of trigonometric functions are mentioned as tools for solving the problem.
📚 Derivatives and the Rate of Change
The second paragraph focuses on the mathematical approach to the ladder problem, emphasizing the need to find the derivative with respect to time to determine the rate of change. The explanation involves setting up a relationship between the distances (x, y, and z) and using this to find the derivative. The paragraph introduces the equation 2x(dx/dt) + 2y(dy/dt) = 2z(dz/dt) to represent the rates of change. It then proceeds to solve for the specific rate (dx/dt) when x equals six feet, which is the distance to the friend. The solution involves applying the Pythagorean theorem to find the value of y and then substituting known values into the derivative equation to isolate and solve for dx/dt.
🧮 Solving for the Ladder's Speed
In the final paragraph, the problem's solution is completed by calculating the speed of the ladder as it reaches the friend. The paragraph reiterates the need to consider the direction of the ladder's movement (downward in this case, hence negative values) and the fixed length of the ladder (10 feet, which does not change). The solution process involves substituting the values into the previously derived equation, taking into account the negative rate for the downward movement, and solving for dx/dt. The final answer for the ladder's speed is given as 16/3 feet per second, with the reminder to include units in a real-world application for accurate results.
Mindmap
Keywords
💡ladder
💡wall
💡rate
💡distance
💡triangle
💡Pythagorean theorem
💡precalculus
💡derivative
💡trigonometric functions
💡special right triangles
💡unit circle
Highlights
The problem involves a ladder falling down a wall at a rate of four feet per second.
The ladder is initially leaning against a wall with a friend standing six feet away from the wall.
The goal is to determine the speed at which the ladder is moving when it reaches the friend.
The scenario is described as a right triangle problem, which allows for the application of the Pythagorean theorem.
The ladder has a fixed length of 10 feet.
The rate of the ladder falling is given as four feet per second.
The problem requires finding the derivative to calculate the rate of change of distance.
The relationship between the changing distances is expressed as x^2 + y^2 = z^2, where z is the fixed length of the ladder.
The derivative relationship is expressed as 2x(dx/dt) + 2y(dy/dt) = 2z(dz/dt) to find the rates of change.
When the ladder is six feet from the wall, the derivative calculation involves plugging in x = 6 into the derivative equation.
The height y of the ladder when it's six feet from the wall is found to be 8 feet using the Pythagorean theorem.
The rate of change of y with respect to time dy/dt is determined to be negative four feet per second, indicating downward movement.
The length of the ladder dz/dt is zero since the ladder's length does not change.
Solving the derivative equation yields the rate of change of x, which is the speed at which the ladder moves when it hits the friend.
The final calculated speed of the ladder when it hits the friend is 16/3 feet per second.
The problem demonstrates the application of precalculus and geometry to real-world scenarios.
The solution process emphasizes the importance of visualizing the problem and understanding the relationships between the variables.
The use of derivatives to find rates of change in physical scenarios is a key concept in calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: