Related Rates - Sliding Ladder Problem
TLDRThe video script presents a detailed exploration of a sliding ladder problem, a common type of related rates problem. The scenario involves a 5-meter ladder sliding down a wall with the bottom moving away from the wall at a rate of 0.8 meters per second. The challenge is to find the velocity of the top of the ladder at T=2 seconds, given that the bottom is 1.5 meters from the wall at T=0 seconds. The solution process involves setting up a right triangle with variable lengths for the horizontal (X) and vertical (Y) sides, and the constant length of the ladder as the hypotenuse (5 meters). By applying related rates and the Pythagorean theorem, the video demonstrates how to calculate the rate of change of the top of the ladder (dy/dt) at the specified time. The key steps include differentiating the equation with respect to time, applying the chain rule, and substituting known values to solve for dy/dt. The video emphasizes the importance of understanding the signs of the rates and using a calculator for the final computation, providing a comprehensive guide to solving related rates problems in trigonometry.
Takeaways
- 📐 The problem involves a sliding ladder, a common type of related rates problem, where the ladder slides down a wall and away from it simultaneously.
- 🏗️ A five-meter ladder is used, with the ladder's length remaining constant throughout the problem, simplifying calculations.
- 📏 The bottom of the ladder slides away from the wall at a rate of 0.8 meters per second, which is a given constant rate.
- ⏱️ At time T=0, the bottom of the ladder is 1.5 meters from the wall, and we are interested in finding the velocity of the top of the ladder at T=2 seconds.
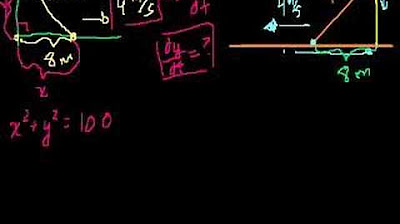
- 🔢 We define variables for the horizontal distance (X), the vertical distance (Y), and use the Pythagorean theorem to relate them in the right triangle formed by the ladder, the wall, and the ground.
- 📈 As time passes, X increases, which means DX/DT is positive and equal to 0.8 m/s. Understanding the direction of change is crucial for related rates problems.
- 🧮 The goal is to find the rate of change of the top of the ladder (dy/dt) at T=2 seconds, which requires differentiating the equation relating X and Y with respect to time.
- 🔄 Applying the chain rule, we differentiate the Pythagorean theorem equation to find the relationship between dx/dt, dy/dt, and d(X^2 + Y^2)/dt.
- ✅ Since the ladder's length is constant, d(X^2 + Y^2)/dt simplifies to 0, which helps in solving for dy/dt.
- 🔢 After differentiating and substituting the known values, we can solve for dy/dt, which represents the velocity of the top of the ladder.
- 📐 The final step involves substituting the values of X, DX/DT, and the expression for Y into the equation for dy/dt to find the specific rate at T=2 seconds.
- 📊 The unit for dy/dt should be in meters per second (m/s), matching the units of dx/dt, ensuring the solution is dimensionally consistent.
Q & A
What is the main topic of the video?
-The video discusses a sliding ladder problem, which is a common type of related rates problem in mathematics.
What is the length of the ladder in the problem?
-The ladder in the problem is 5 meters long.
At what rate does the bottom of the ladder slide away from the wall?
-The bottom of the ladder slides away from the wall at a rate of 0.8 meters per second.
At what time is the velocity of the top of the ladder to be found?
-The velocity of the top of the ladder is to be found at T equals 2 seconds.
What is the initial distance of the bottom of the ladder from the wall at T equals zero seconds?
-At T equals zero seconds, the bottom of the ladder is 1.5 meters from the wall.
What is the variable used to represent the horizontal distance from the wall in the triangle?
-The variable used to represent the horizontal distance from the wall is X.
What is the variable used to represent the vertical distance from the ground in the triangle?
-The variable used to represent the vertical distance from the ground is Y.
Why is it important to get the signs of the rates correct in related rates problems?
-The signs of the rates are crucial because they indicate the direction of the change (e.g., whether the ladder is sliding away from or towards the wall), which affects the calculation of the rate of change for other variables.
What theorem is used to relate the sides of the right triangle formed by the ladder, the wall, and the ground?
-The Pythagorean theorem is used to relate the sides of the right triangle, stating that the square of the hypotenuse (the ladder's length) is equal to the sum of the squares of the other two sides (X and Y).
What is the expression for the derivative of the ladder's length squared with respect to time?
-The derivative of the ladder's length squared (25 meters squared) with respect to time is 0, because the ladder's length is constant and does not change over time.
How is the final expression for dy/dt simplified before plugging in the values?
-The expression for dy/dt is simplified by applying the chain rule to the derivative of the Pythagorean theorem equation and then isolating dy/dt on one side of the equation.
What should be the units for dy/dt after solving the problem?
-The units for dy/dt should be meters per second (m/s), which is the same as the units for dx/dt, representing the velocity.
Outlines
📐 Sliding Ladder Problem Introduction
The video begins with an introduction to a sliding ladder problem, a common type of related rates problem. A 5-meter ladder slides down a wall while its bottom moves away from the wall at a rate of 0.8 meters per second. The goal is to find the velocity of the top of the ladder at t=2 seconds, given that the bottom is 1.5 meters from the wall at t=0 seconds. The problem is more complex than typical ladder problems because it requires understanding the changing positions within a triangle as time progresses.
Mindmap
Keywords
💡Sliding Ladder Problem
💡Related Rates
💡Velocity
💡Right Triangle
💡Pythagorean Theorem
💡Derivative
💡Chain Rule
💡Constants
💡Time Variable (t)
💡Initial Conditions
💡Rate of Change
Highlights
The video discusses a sliding ladder problem, a common type of related rates problem.
A five-meter ladder slides down a wall while the bottom slides away at a rate of 0.8 meters per second.
The problem involves finding the velocity of the top of the ladder at T equals two seconds.
The bottom of the ladder is initially 1.5 meters from the wall at T equals zero seconds.
The length of the ladder remains constant at five meters, simplifying the problem.
The rate of change of the bottom's distance from the wall (DX/DT) is positive 0.8 meters per second.
The direction of the ladder's movement affects the sign of the rate in related rates problems.
At T equals 2, the bottom's distance (X) from the wall is calculated to be 3.1 meters.
Pythagorean theorem is used to relate the horizontal (X), vertical (Y), and hypotenuse (ladder length) of the triangle formed.
The derivative dY/dt, representing the rate of change of the top of the ladder, is the primary unknown to solve for.
Differentiating the Pythagorean relationship with respect to time (t) is key to finding dY/dt.
The derivative of the ladder's constant length results in zero, simplifying the differentiation process.
Substituting known values and applying the chain rule allows solving for dY/dt.
Careful consideration of the units ensures that dY/dt is measured in meters per second, matching DX/DT.
The final step involves using a calculator to find the numerical value of dY/dt.
The video emphasizes the importance of getting the signs of the rates correct in related rates problems.
Triangles are ubiquitous in related rates problems, even in those that initially seem unrelated to geometry.
The video provides a step-by-step approach to solving the sliding ladder problem, from setting up the triangle to differentiating and solving for dY/dt.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: