Solving a falling ladder problem using related rates
TLDRThe video script discusses a mathematical problem involving a 50-foot ladder leaning against a wall. As the base of the ladder is pulled away from the wall at a rate of 3 feet per second, the video aims to determine the rate at which the top of the ladder is falling when the base is 30 feet from the wall. By using the Pythagorean theorem and differentiating with respect to time, the problem is solved to find that the top of the ladder falls at a rate of 9.4 feet per second.
Takeaways
- 📏 The problem involves a 50-foot ladder leaning against a wall with its base being pulled away at a rate of 3 feet per second.
- 🏢 The scenario takes place at a building where the ladder's base starts to move away from the wall.
- 📐 The question asks for the rate at which the top of the ladder is falling when the base is 30 feet from the wall.
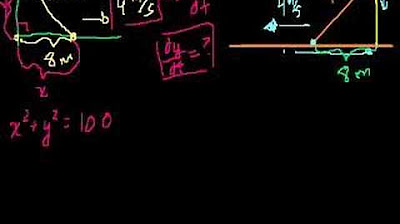
- 🎨 A visual representation or drawing of the ladder, wall, and ground is recommended to better understand the problem.
- 📚 The Pythagorean theorem is essential in solving this problem, with the ladder forming a right-angled triangle with the wall and ground.
- 📈 The ladder's length (L) is 50 feet, the base (B) is moving to 30 feet from the wall, and the height (H) can be calculated as 40 feet using the Pythagorean theorem.
- 🔄 Differentiating the Pythagorean equation with respect to time gives us the relationship between the rates of change of the base, height, and ladder's length.
- 📉 The rate of change of the base (db/dt) is given as 3 feet per second, and the problem states that the ladder's length doesn't change (dl/dt = 0).
- 📌 The key equation to solve is 2b * (db/dt) + 2h * (dh/dt) = 2l * (dl/dt), where b, h, and l are the base, height, and ladder's length, respectively.
- 🧠 By substituting the known values and solving for (dh/dt), we find the rate of the top of the ladder falling.
- 📉 The calculated rate at which the top of the ladder is falling when the base is 30 feet from the wall is -9.4 feet per second.
Q & A
What is the length of the ladder mentioned in the transcript?
-The length of the ladder mentioned in the transcript is 50 feet.
How fast is the base of the ladder being pulled away from the wall?
-The base of the ladder is being pulled away from the wall at a rate of 3 feet per second.
What is the Pythagorean triple that the speaker refers to in the context of the ladder's height?
-The Pythagorean triple referred to is 3-4-5, which helps determine that the height of the ladder is 40 feet.
What is the equation derived from the Pythagorean theorem that the speaker uses?
-The equation derived from the Pythagorean theorem is b^2 + h^2 = l^2, where b is the distance from the wall to the base of the ladder, h is the height of the ladder, and l is the length of the ladder.
How does the speaker differentiate the Pythagorean equation with respect to time?
-The speaker differentiates the equation with respect to time as follows: d/dt(b^2 + h^2) = d/dt(l^2), which simplifies to 2b(db/dt) + 2h(dh/dt) = 2l(dl/dt).
At what distance from the wall is the base of the ladder when the speaker is solving the problem?
-When the speaker is solving the problem, the base of the ladder is 30 feet from the wall.
What is the rate at which the top of the ladder is falling when the base is 30 feet from the wall?
-The top of the ladder is falling at a rate of -9.4 feet per second when the base is 30 feet from the wall.
Why is the change in the ladder's length with respect to time considered zero in the problem?
-The change in the ladder's length with respect to time is considered zero because the ladder is not changing in length, only its position relative to the wall is changing.
What does the negative sign in the rate of the top of the ladder falling indicate?
-The negative sign indicates that the top of the ladder is moving downward, or falling, as the base is being pulled away from the wall.
What is the significance of the speaker's recommendation to draw a picture to understand the problem better?
-Drawing a picture is significant as it visually represents the problem, making it easier to understand the spatial relationships between the ladder's base, height, and length, and how these change over time.
How does the speaker ensure that the final equation correctly represents the rate of change of the ladder's height?
-The speaker ensures this by differentiating the Pythagorean equation with respect to time, substituting the known values, and solving for the unknown rate of change of the ladder's height.
Outlines
📏 Mathematical Problem Explanation
The paragraph discusses a mathematical problem involving a 50-foot ladder leaning against a wall. The base of the ladder is being pulled away from the wall at a rate of 3 feet per second. The speaker is trying to determine how fast the top of the ladder is falling when the base is 30 feet from the wall. The speaker uses the Pythagorean theorem to relate the lengths of the ladder, the distance from the wall, and the height of the ladder. The speaker then sets up a differential equation to find the rate of change of the height with respect to time and solves it to find the answer.
🔢 Derivative Calculation for Ladder Problem
In this paragraph, the speaker continues to work through the ladder problem by focusing on the derivative calculations. The speaker acknowledges that the length of the ladder does not change, so its derivative with respect to time is zero. The base of the ladder is moving at a known rate, which is 3 feet per second, so the derivative of the base with respect to time is -3 feet per second. The speaker then sets up the equation involving the derivatives of the base and height with respect to time and the derivative of the ladder's length, which is zero. After solving the equation, the speaker finds that the top of the ladder is falling at a rate of -9.4 feet per second when the base is 30 feet from the wall.
Mindmap
Keywords
💡50-foot ladder
💡pulling away
💡rate of change
💡Pythagorean theorem
💡differentiate with respect to time
💡related rates
💡base
💡height
💡length
💡derivative
💡negative rate
Highlights
A 50-foot ladder is leaning against a wall with its base pulled away at a rate of 3 feet per second.
The problem is to find out how fast the top of the ladder is falling when the base is 30 feet from the wall.
The ladder has a length (L) of 50 feet, which is given as a starting point for the problem.
A visual representation or drawing can help understand the problem better, by visualizing the ladder, base, and height.
The Pythagorean theorem is applied to relate the lengths of the ladder, the distance from the wall to the base (b), and the height (h).
The height (h) is calculated to be 40 feet based on the Pythagorean triple 3-4-5, corresponding to the given lengths.
A differential equation is formed using the Pythagorean theorem and differentiating with respect to time (t).
The rate of change of the base (db/dt) is given as 3 feet per second, which is a key parameter in the problem.
The length of the ladder (L) is constant and does not change with respect to time, so its rate of change (dl/dt) is zero.
The equation simplifies to finding the rate of change of the height (dh/dt) when the base is 30 feet from the wall.
By solving the differential equation, the rate at which the top of the ladder is falling is determined.
The final answer is a negative rate of change, indicating the top of the ladder is falling at a rate of 9.4 feet per second.
The problem-solving approach involves understanding the geometry of the situation and applying calculus to find rates of change.
The problem demonstrates the practical application of mathematical concepts in real-world scenarios, such as the movement of a ladder.
The process emphasizes the importance of visualizing and understanding the problem before attempting to solve it mathematically.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: