Related Rates - The Ladder Problem
TLDRThis video script presents a mathematical problem involving a 17-foot ladder sliding away from a building. It calculates the rate at which the top of the ladder slides down the wall, the rate of change of the area formed by the ladder, and the rate of change of the angle between the ladder and the ground. Using concepts from calculus, such as the Pythagorean theorem, differentiation, and trigonometric functions, the video provides a step-by-step solution to each part of the problem, resulting in detailed explanations and final answers.
Takeaways
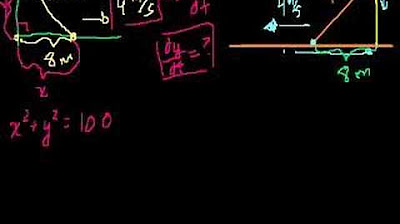
- 📏 A 17-foot ladder is leaning against a building with its foot 8 feet from the base.
- 🏃♂️ The ladder is sliding away from the building at a rate of 3 feet per second.
- 📐 The Pythagorean theorem is used to relate the lengths x, y, and z (17) of the right triangle formed.
- 🔄 As the ladder slides, both x (distance from the building) and y (height on the wall) change, with dx/dt being 3 ft/s and an unknown dy/dt.
- 🧭 Differentiating the Pythagorean equation with respect to time yields an equation to solve for dy/dt.
- 📉 The value of y (height on the wall) is found to be 15 feet using the Pythagorean theorem.
- 📌 The rate of the top of the ladder sliding down the wall, dy/dt, is calculated to be -8/5 ft/s.
- 📐 The area formed by the ladder is changing, and its rate of change (dA/dt) is calculated using the formula for the area of a right triangle and the product rule.
- 🔢 The rate of change of the area (dA/dt) is found to be 161/10 square feet per second.
- 📐 The angle between the ladder and the ground (θ) is related to x, y, and z using trigonometric functions.
- 🌟 The rate of change of the angle (dθ/dt) is determined to be -1/5 radians per second, avoiding the quotient rule by using sine and cosine.
Q & A
What is the length of the ladder mentioned in the problem?
-The length of the ladder is 17 feet.
How far is the foot of the ladder from the base of the building initially?
-The foot of the ladder is initially 8 feet away from the base of the building.
At what rate is the ladder sliding away from the building?
-The ladder is sliding away from the building at a rate of 3 feet per second.
What is the Pythagorean theorem and how is it applied in this problem?
-The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In this problem, it is applied to relate the lengths x, y, and z (the length of the ladder) of the right triangle formed by the ladder, the ground, and the wall.
What is the value of y (the height of the ladder on the wall) when x is 8 and z is 17?
-Using the Pythagorean theorem, we find that y is the square root of (z squared - x squared), which is the square root of (17^2 - 8^2) or 15 feet.
How is the rate of change of y with respect to time (dy/dt) calculated?
-The rate of change of y with respect to time (dy/dt) is calculated by differentiating the equation z^2 = x^2 + y^2 with respect to time and solving for dy/dt. The result is -24/15 feet per second.
What is the formula for the area of a right triangle?
-The area of a right triangle is given by half the product of its base and height, or (1/2) * base * height.
How is the rate of change of the area (dA/dt) with respect to time calculated in this problem?
-The rate of change of the area (dA/dt) is calculated by differentiating the formula for the area of a right triangle with respect to time using the product rule, which results in (1/2) * (dx/dt * y + x * dy/dt). By plugging in the given values, we get dA/dt as 161/10 square feet per second.
What is the angle between the ladder and the ground, and how is it denoted in the problem?
-The angle between the ladder and the ground is denoted by theta in the problem.
Which trigonometric function is used to relate theta to x, y, and z, and why is it chosen?
-The sine function is used to relate theta to x, y, and z because it involves a variable (y) and a constant (z), making it simpler to differentiate using the power rule rather than the quotient rule which would be needed if tangent was used.
How is the rate of change of the angle theta with respect to time (dθ/dt) calculated?
-The rate of change of the angle theta with respect to time (dθ/dt) is calculated by differentiating the sine function with respect to time using the chain rule and the constant multiple rule, resulting in dθ/dt being -1/5 radians per second.
What are the units for the rate of change of the angle (dθ/dt), and why is this important?
-The units for dθ/dt are radians per second. This is important because it provides context for the magnitude and type of change occurring, which is crucial for understanding the physical situation described in the problem.
Outlines
📐 Mathematical Analysis of a Moving Ladder
This paragraph introduces a problem involving a 17-foot ladder sliding away from a building at a rate of 3 feet per second. The initial setup includes the ladder forming a right triangle with the building and the ground, where the foot of the ladder is 8 feet from the building. The goal is to determine the rate at which the top of the ladder is sliding down the wall, represented by dy/dt. The problem is approached by using the Pythagorean theorem to relate the lengths of the ladder (x, y, and constant z) and then differentiating the equation with respect to time to find the unknown derivative. The solution involves calculating the current value of y using the theorem and then using the given dx/dt to solve for dy/dt, resulting in a negative value of -8/5 feet per second.
📐 Deriving the Rate of Change of the Triangle's Area
The second paragraph focuses on calculating the rate of change of the area formed by the ladder at a given instant. The area of a right triangle is given by the formula 1/2 * base * height, which in this case are x and y respectively. The task involves differentiating this formula with respect to time to find da/dt. By applying the product rule and using the previously calculated dx/dt and the constant value of y, the derivative is determined. The units for the variables are established as feet for x and y, and seconds for time. The final calculation results in a value of 161/10 square feet per second, indicating the rate of change of the area.
📐 Finding the Rate of Change of the Angle Between the Ladder and Ground
The final paragraph addresses the challenge of finding the rate at which the angle between the ladder and the ground is changing at that instant. The angle, denoted as theta, is related to the variables x, y, and z using trigonometric functions. The paragraph discusses the principles of SOHCAHTOA and selects sine to relate theta to the variables, as it involves a constant and a variable, simplifying the differentiation process. The derivative of sine theta is found using the chain rule and the constant multiple rule. The calculation yields a value of -1/5 radians per second for dθ/dt, indicating the rate of change of the angle.
Mindmap
Keywords
💡Ladder
💡Pythagorean Theorem
💡Differentiation
💡Rate of Change
💡Right Triangle
💡Hypotenuse
💡Trigonometry
💡Chain Rule
💡Constant Multiple Rule
💡Quotient Rule
💡SOHCAHTOA
💡Area
Highlights
A 17-foot ladder is leaning against a building with its foot 8 feet from the base.
The ladder is sliding away from the building at 3 feet per second.
The goal is to find out how fast the top of the ladder is sliding down the wall.
The Pythagorean theorem is used to relate the lengths of the ladder, building, and the distance from the base.
The derivative of the Pythagorean equation with respect to time is used to find the rate of change.
The value of y (height of the ladder on the wall) is calculated to be 15 feet using the Pythagorean theorem.
The rate of change of y (dy/dt) is found to be -24/15 feet per second, indicating the top of the ladder is sliding down.
The area formed by the ladder is a right triangle, with the area calculated as 1/2 * base * height.
The rate of change of the area (dA/dt) is determined using the product rule and given as 161/10 square feet per second.
The angle between the ladder and the ground (theta) is analyzed using trigonometric functions.
The rate of change of the angle (dθ/dt) is calculated to be -1/5 radians per second.
The problem involves the use of calculus concepts, including differentiation and the chain rule.
The problem demonstrates the practical application of mathematics in real-world scenarios.
The solution process is clearly explained, making it an excellent example of mathematical problem-solving.
The use of trigonometric identities (SOHCAHTOA) is highlighted in solving the problem.
The problem showcases the importance of correctly identifying the variables and constants in a mathematical model.
The solution involves the use of both algebraic and geometric methods.
The problem is a comprehensive example of the application of the Pythagorean theorem in a dynamic situation.
The final answers are provided with their respective units, emphasizing the importance of dimensional analysis.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: