Projectile launched off a cliff at an angle
TLDRIn this engaging video, the presenter, Physics Ninja, tackles the problem of a projectile launched at an angle from a 15-meter high cliff with an initial velocity of 20 m/s at a 25-degree angle. The video meticulously calculates the time the projectile is in the air (2.8 seconds), the horizontal distance it travels (51 meters), and its final velocity (26.3 m/s) at impact. Additionally, the presenter explores the maximum height reached by the projectile, finding it to be 18.7 meters, using two different kinematic approaches. The step-by-step breakdown of the physics equations and the clear explanation make this a valuable resource for those interested in understanding projectile motion.
Takeaways
- 📐 The problem involves a projectile launched at an angle from a cliff with given initial velocity, angle, and cliff height.
- 🕒 The time of flight is calculated using the vertical motion and quadratic equation, resulting in a positive solution of 2.8 seconds.
- 🏹 The horizontal distance (X) traveled by the projectile is determined by multiplying the initial horizontal velocity component (18.1 m/s) by the time of flight (2.8 s), equaling 51 meters.
- 🚀 The final velocity components are calculated separately for the X and Y directions, with the X component being the initial horizontal velocity and the Y component being the initial vertical velocity minus the acceleration due to gravity over time.
- 🌐 The magnitude of the final velocity is found using the Pythagorean theorem, combining the X and Y components, resulting in 26.3 m/s.
- 📈 The angle of the final velocity vector with respect to the horizontal is calculated using the tangent function and the velocity components, yielding 46.2 degrees.
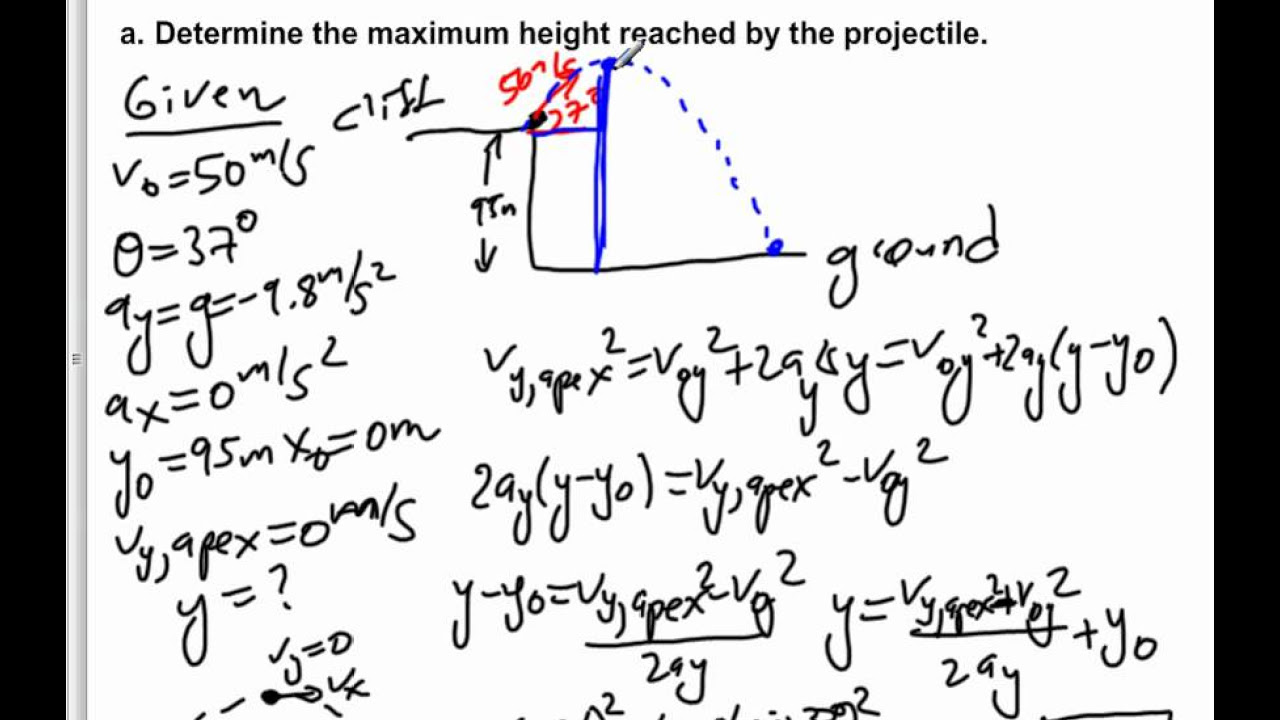
- 🔝 The maximum height reached by the projectile is determined in two ways: either by setting the final vertical velocity to zero and solving for time, then substituting back into the displacement equation, or by using a kinematic equation relating initial velocity, acceleration, and vertical displacement.
- 🏞️ The height calculated using the first method is 18.7 meters, and using the second method, the displacement (Delta Y) is found to be 3.7 meters, confirming the same result as the first method when considering the initial cliff height.
- 🎯 The analysis is based on the principles of projectile motion, considering both horizontal and vertical components of velocity and displacement.
- 🤔 The problem-solving approach emphasizes the importance of understanding the separate horizontal and vertical motions in projectile problems and how they interact over time.
- 💡 The script provides a comprehensive walkthrough of the calculations, offering clear explanations and steps that can be followed for similar physics problems.
Q & A
What is the initial velocity of the projectile launched?
-The initial velocity of the projectile is 20 meters per second.
What angle is the projectile launched at?
-The projectile is launched at an angle of 25 degrees.
What is the height of the cliff from which the projectile is launched?
-The height of the cliff is 15 meters.
How do you calculate the time the projectile is in the air?
-The time the projectile is in the air is calculated using the vertical motion equation, which is a quadratic equation in time. By solving this equation, you find the positive solution which represents the time of flight.
What is the horizontal distance the projectile travels from the cliff?
-The horizontal distance the projectile travels is calculated by multiplying the initial velocity in the X direction (20 m/s) by the total time of flight (2.8 seconds), resulting in approximately 51 meters.
How is the final velocity of the projectile determined?
-The final velocity of the projectile is determined by calculating both the X and Y components of the velocity, and then using the Pythagorean theorem to find the magnitude of the velocity vector. The angle of the velocity vector with respect to the horizontal is found using the tangent function.
What is the maximum height reached by the projectile?
-The maximum height reached by the projectile can be found using two approaches: one by setting the final vertical velocity to zero in the vertical motion equation, and the other by using the kinematic equation that relates initial velocity, acceleration, and displacement. Both methods yield a maximum height of approximately 18.7 meters.
How is the vertical component of the projectile's velocity at the top of its trajectory calculated?
-The vertical component of the projectile's velocity at the top of its trajectory is calculated by using the vertical velocity equation, which gives the velocity as the initial vertical velocity minus the product of gravitational acceleration and time. At the top, the vertical velocity is zero, so the time can be found by dividing the initial vertical velocity by gravitational acceleration.
What is the significance of the quadratic equation in solving the projectile motion problem?
-The quadratic equation is significant in solving the projectile motion problem because it represents the vertical motion of the projectile. Solving this equation gives the time of flight, which is essential for calculating other parameters such as the horizontal distance traveled and the final velocity.
Why is the positive solution of the quadratic equation in time considered in the context of the projectile motion?
-In the context of the projectile motion, the positive solution of the quadratic equation in time is considered because it represents the actual time it takes for the projectile to travel from the cliff to the ground. The negative solution does not make physical sense in this scenario as time cannot be negative.
How does the horizontal component of the projectile's initial velocity affect the distance traveled?
-The horizontal component of the projectile's initial velocity directly affects the distance traveled because, in the absence of air resistance and horizontal acceleration, the horizontal velocity remains constant. The distance traveled is calculated by multiplying the horizontal component of the initial velocity by the time of flight.
What is the role of gravitational acceleration in the projectile motion calculations?
-Gravitational acceleration (denoted as 'g' or 9.8 m/s^2 on Earth) plays a crucial role in the projectile motion calculations as it is the acceleration acting on the projectile in the vertical direction. It is used in the equations to determine the vertical motion, which in turn affects the total time of flight, the final vertical velocity, and the maximum height reached by the projectile.
Outlines
🚀 Projectile Motion: Launch and Trajectory
This paragraph introduces the problem of projectile motion at an angle. The speaker, Physics Ninja, revisits a previous problem involving a horizontally launched projectile and builds upon it by considering a launch angle of 25 degrees from the horizontal. The initial velocity is given as 20 meters per second, and the height of the cliff is 15 meters. The focus is on calculating the time the projectile is in the air, its horizontal distance from the cliff, and its final velocity. The approach involves analyzing the vertical motion first due to the unknown horizontal displacement at the point of impact. The projectile's trajectory is described as a parabolic arc, and the time of flight is determined by setting up a quadratic equation in terms of time, yielding a positive solution of 2.8 seconds.
📏 Calculating Horizontal Distance and Final Velocity
In this paragraph, the speaker continues the analysis by calculating the horizontal distance traveled by the projectile. Using the initial velocity in the horizontal direction and the time of flight calculated earlier, the horizontal distance (X) is found to be approximately 51 meters. The final velocity of the projectile is then determined by calculating its components in the X and Y directions. The X component remains constant due to the lack of acceleration in the horizontal plane, while the Y component is calculated considering the initial vertical velocity and the acceleration due to gravity. The final velocity is found using the Pythagorean theorem, resulting in a magnitude of 26.3 meters per second. Additionally, the angle of the final velocity vector with respect to the horizontal is calculated to be approximately 46.2 degrees.
🔝 Maximum Height and Alternative Calculation Methods
The final paragraph addresses the calculation of the projectile's maximum height (WiMAX). Two methods are presented for this calculation. The first method involves using the vertical displacement equation and knowing the time at which the vertical velocity becomes zero at the peak of the trajectory. The second method uses an alternative kinematic equation, taking into account the initial horizontal velocity and the vertical displacement. Both methods lead to the conclusion that the projectile reaches a maximum height of 18.7 meters above the ground. The speaker encourages viewers to subscribe to the channel and reach out with any questions, offering help with physics problems.
Mindmap
Keywords
💡Projectile Motion
💡Horizontal Launch
💡Angle of Launch
💡Initial Velocity
💡Time of Flight
💡Trajectory
💡Final Velocity
💡Acceleration Due to Gravity
💡Quadratic Equation
💡Horizontal Distance
💡Maximum Height
Highlights
The physics problem involves a projectile launched at an angle from a cliff.
Initial velocity is 20 meters per second at a 25-degree angle from horizontal.
The height of the cliff is 15 meters.
The time the projectile is in the air is calculated to be 2.8 seconds.
The horizontal distance from the cliff is approximately 51 meters.
The final velocity magnitude is 26.3 meters per second.
The angle of the final velocity vector with respect to the horizontal is 46.2 degrees.
The maximum height reached by the projectile is 18.7 meters.
Two methods are used to calculate the maximum height: one using the vertical position equation and the other using a kinematic equation.
The vertical component of the initial velocity is calculated using the sine of the launch angle.
The horizontal component of the initial velocity is determined by the cosine of the launch angle.
The acceleration due to gravity is considered in the vertical motion equations.
The Pythagorean theorem is used to find the magnitude of the final velocity vector.
The tangent function is used to find the angle of the final velocity vector.
The problem-solving approach involves breaking down the motion into horizontal and vertical components.
The quadratic formula is used to solve for time in the vertical motion equation.
The horizontal motion is not considered when calculating time in the air because the horizontal displacement is unknown.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: