Marginal Cost and Average Total Cost
TLDRThis video script presents a comprehensive guide to understanding marginal cost, average cost, and how to determine the production level that minimizes average cost. It begins by explaining how to calculate the total cost, average cost, and marginal cost given a cost function and a production level of 200 units. The script then demonstrates how to find the production level that results in the lowest average cost by setting the marginal cost equal to the average cost. The video concludes with the calculation of the minimum average cost and verifies the result by comparing average costs at different production levels. The detailed explanation is designed to help viewers grasp the concepts and apply them to real-world scenarios.
Takeaways
- 📈 The total cost function is given by c(x) = 1500 + 5x + 0.01x^2.
- 🔢 To find the total cost at a production level of 200 units, substitute x with 200 in the cost function: 1500 + 5(200) + 0.01(200^2) = 2900.
- 💰 The average cost per unit is calculated by dividing the total cost by the number of units produced: 2900 / 200 = $14.50.
- 📈 The marginal cost is the first derivative of the cost function: c'(x) = 5 + 0.02x.
- 🔄 At a production level of 200 units, the marginal cost is 5 + 0.02(200) = $9 per unit.
- 📊 The marginal cost represents the additional cost of producing one extra unit.
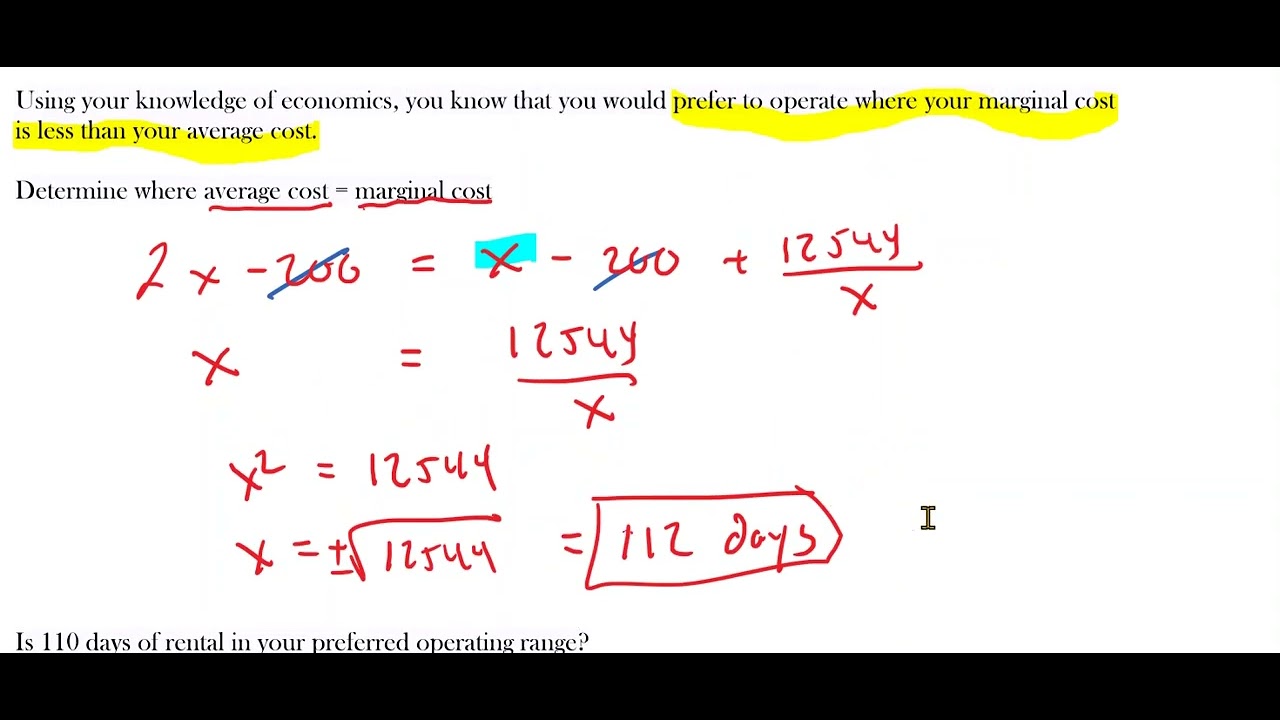
- 🔧 To minimize the average cost, set the marginal cost equal to the average cost and solve for x.
- 📐 The production level that minimizes the average cost is approximately 387 units.
- 💹 The minimum average cost at a production level of 387 units is $12.75 per unit.
- 🔄 To verify the minimum average cost, compare average costs at production levels below and above 387 units.
- 🎓 Understanding these concepts is crucial for making informed decisions regarding production levels and cost management.
Q & A
What is the given cost function in the video?
-The given cost function is c(x) = 1500 + 5x + 0.01x^2.
How is the total cost calculated for producing 200 units?
-The total cost for producing 200 units is calculated by substituting x = 200 into the cost function: 1500 + 5(200) + 0.01(200)^2 = 2900.
What is the average cost per unit when producing 200 units?
-The average cost per unit is the total cost divided by the number of units. For 200 units, it is 2900/200 = 14.50 dollars per unit.
How do you find the marginal cost for producing 200 units?
-The marginal cost is the first derivative of the cost function, evaluated at the production level of 200 units. It is c'(x) = 5 + 0.02x, and at x = 200, it equals 5 + 0.02(200) = 9 dollars per unit.
What does the marginal cost represent in the context of the video?
-The marginal cost represents the additional cost of producing one extra unit. It tells us how much it will cost to produce one more item.
How is the production level that minimizes the average cost determined?
-The production level that minimizes the average cost is found by setting the marginal cost equal to the average cost and solving for x. In this case, it is determined by the equation 0.02x = 1500/x, which yields x = 387 units.
What is the minimum average cost per unit?
-The minimum average cost per unit is found by plugging the production level that minimizes the average cost (387 units) back into the average cost function, resulting in 12.75 dollars per unit.
How are the average costs verified to ensure that the calculated minimum is indeed the lowest?
-The average costs are verified by calculating the average costs at production levels slightly below and above the calculated minimum (387 units). If the calculated values are higher than the minimum, it confirms that the minimum has been correctly identified.
What is the total cost of producing one extra unit beyond 200 units?
-The total cost of producing one extra unit beyond 200 units is found by calculating the cost for 201 units, which is 1500 + 5(201) + 0.01(201)^2 = 2909.01. The difference between this and the cost for 200 units (2900) is approximately 9 dollars.
What is the significance of the difference between the total costs at 200 and 201 units of production?
-The difference between the total costs at 200 and 201 units of production represents the additional cost of producing that one extra unit, which is the definition of marginal cost.
How does the video script illustrate the relationship between marginal cost and average cost?
-The video script illustrates that the average cost is minimized when the marginal cost is equal to the average cost. This is shown through the process of setting the marginal cost function equal to the average cost function and solving for the production level that yields the minimum average cost.
Outlines
📈 Calculating Marginal and Average Costs
This paragraph introduces the concept of marginal and average costs in the context of a given cost function, c(x) = 1500 + 5x + 0.01x^2. It explains how to calculate the total cost for producing 200 units by substituting x with 200, resulting in a total cost of $2,900. The average cost per unit is then determined by dividing the total cost by the number of units, yielding $14.50 per unit. Additionally, the marginal cost is defined as the first derivative of the cost function and is calculated for 200 units, resulting in an additional cost of $9 per unit. The significance of marginal cost in understanding the cost of producing one extra unit is highlighted.
📉 Finding the Production Level for Minimum Average Cost
This paragraph focuses on the process of finding the production level that minimizes the average cost. It establishes the principle that average cost is minimized when marginal cost equals average cost. The average cost function is derived by dividing the total cost function by x, leading to an equation that is solved to find the optimal production level, x = 387 units. This production level is then rounded to the nearest whole number, as it represents physical units of production.
💰 Determining the Minimum Average Cost
The final paragraph calculates the minimum average cost by substituting the optimal production level of 387 units into the average cost function. The calculation results in a minimum average cost of $12.75 per unit. To confirm that this indeed represents the minimum average cost, average costs are also calculated for production levels below and above 387 units (350 and 420 units, respectively), which are found to be higher than the minimum. This validates the production level of 387 units as the point at which the average cost is minimized.
Mindmap
Keywords
💡Marginal Cost
💡Average Cost
💡Total Cost
💡Production Level
💡Derivative
💡First Derivative
💡Cost Function
💡Minimum Average Cost
💡Economic Efficiency
💡Optimization
💡Solving Equations
Highlights
The video discusses the problem of marginal cost and average costs in the context of a given cost function.
The cost function is given as c(x) = 1500 + 5x + 0.01x^2.
The total cost of producing 200 units is calculated by substituting x with 200 in the cost function.
The total cost at a production level of 200 units is found to be $2,900.
The average cost per unit is determined by dividing the total cost by the number of units.
The average cost to produce one unit of the item at a production level of 200 units is $14.50.
The marginal cost is defined as the first derivative of the cost function with respect to x.
The marginal cost for producing 200 units is calculated to be $9 per unit.
Marginal cost represents the additional cost of producing one extra unit.
The total cost to produce 201 units is calculated to further illustrate the concept of marginal cost.
The minimum average cost production level is found by setting the marginal cost equal to the average cost.
The production level that minimizes the average cost is approximately 387 units.
The minimum average cost per unit at the optimal production level is $12.75.
The minimum average cost is verified by comparing average costs at production levels below and above 387 units.
The video provides a comprehensive guide on calculating average cost, marginal cost, and finding the minimum average cost.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: