Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3
TLDRThis video tutorial guides viewers on how to calculate the area between two curves, specifically y = x^3 - 3x^2 and y = x - 3, which intersect at three points: (-1, -4), (1, -2), and (3, 0). The presenter emphasizes the importance of finding intersection points to understand the graph's behavior. The area under consideration is divided into two regions due to the nature of the curves' intersections. To find the total area, the video suggests two methods: using a calculator with absolute value integration from -1 to 3, or manually calculating two separate integrals for each region and summing them. The manual approach involves finding antiderivatives and evaluating them at the bounds of integration. The presenter demonstrates the process for both integrals, ultimately revealing the total area to be 8, derived from the sum of the areas of the two regions.
Takeaways
- 📐 **Finding Intersection Points**: To understand the area between two curves, first find the points where they intersect by setting the two equations equal to each other.
- 🔍 **Factoring by Grouping**: Solve the resulting equation by factoring it, which can help in finding the x-values for the intersection points.
- 📈 **Graphing the Functions**: Plot the intersection points on a graph to visualize the regions bounded by the curves and understand their interactions.
- 📌 **Identifying Key Points**: Key points such as (-1, -4), (1, -2), and (3, 0) are crucial for graphing the curves and understanding the bounded regions.
- 🔺 **Understanding the Shape of Curves**: Recognize the general shape of the cubic and linear functions to better visualize their graph and the regions they enclose.
- 🚫 **Avoiding Absolute Values**: When calculating areas without a calculator, it's more efficient to work with separate integrals rather than using absolute values.
- 🧮 **Calculating Separate Integrals**: Break down the problem into two separate integrals, one for each region where one curve is above the other, and solve them individually.
- ✅ **Simplifying Expressions**: When evaluating integrals, simplify expressions by combining like terms and using the properties of algebra to make calculations easier.
- 📝 **Evaluating at Boundaries**: For each integral, evaluate the antiderivative at the upper and lower bounds of the region to find the area.
- 🔄 **Combining Results**: Add the results of the two separate integrals to get the total area enclosed by the curves.
- 📉 **Visualizing the Area**: Even with a calculator, it's beneficial to visualize the area on the graph to understand the problem better and ensure accuracy in calculations.
- 🎯 **Accuracy Over Speed**: While calculators can provide quick results, taking the time to understand the problem and work through the integrals manually can lead to a more accurate and educational solution.
Q & A
What is the main topic of the video?
-The video is about finding the area between two curves, specifically y = x^3 - 3x^2 and y = x - 3, where there are multiple regions involved due to the curves intersecting at different points.
How does the video suggest to start solving the problem?
-The video suggests starting by finding the intersection points of the two curves by setting y equal to y and solving the resulting equation.
What are the intersection points found in the video?
-The intersection points are found to be at x = 3, x = -1, and x = 1.
How does the video approach the graphing of the curves?
-The video approaches graphing by first plotting the intersection points and then sketching the curves, noting that the cubic curve has a bounce at x = 0 and the linear curve is a straight line.
What are the two regions that the video identifies for calculating the area?
-The two regions identified are the area where the cubic curve is on top and the area where the linear curve is on top.
What is the method suggested for calculating the area without a calculator?
-The video suggests calculating the area by working out two separate integrals for each region and then adding them up at the end.
What is the alternative method for calculating the area with a calculator?
-The alternative method is to use the absolute value of the difference between the two functions over the interval from the first to the last intersection point.
How does the video simplify the integrals for calculation?
-The video simplifies the integrals by distributing negative signs and separating the integrals into two parts, one from negative one to one and the other from one to three.
What is the final area calculated in the video?
-The final area calculated in the video is 8, which is the sum of the areas of the two separate regions.
Why does the video recommend not simplifying everything in the integrals?
-The video recommends not simplifying everything because it can be avoided by directly substituting the bounds of integration, which simplifies the process and avoids unnecessary algebra.
What does the video imply about the importance of sketching the region?
-The video implies that sketching the region is crucial for understanding the problem and determining the correct approach to finding the area between the curves.
How does the video handle the issue of multiple intersection points?
-The video handles multiple intersection points by identifying each point, plotting them, and then breaking down the area calculation into separate integrals for each region between the intersection points.
Outlines
📐 Finding Intersection Points for Area Calculation
The video begins with the objective of finding the area between two curves, y = x^3 - 3x^2 and y = x - 3, which intersect in multiple regions. The presenter emphasizes the importance of identifying intersection points to understand the graph's behavior. By setting the two equations equal to each other, the presenter algebraically solves for the points where x = 3, x = -1, and x = 1. These points are then used to sketch the curves and identify two distinct regions where one curve lies above the other. The presenter suggests using a calculator for an absolute value integral approach or manually calculating the area by summing two separate integrals, corresponding to the regions where each curve is on top.
🧮 Calculating the Area Between Curves Manually
The presenter chooses to calculate the area between the curves manually by setting up two separate integrals, one for each region where a different curve is on top. The first integral is from x = -1 to x = 1, where the cubic function is above the linear one, and the second integral is from x = 1 to x = 3, where the linear function is above the cubic. The presenter then finds the antiderivative for each integral and evaluates them between the bounds, simplifying the expressions step by step. The final area is obtained by adding the results of both integrals, which in this case, both yield the same value of 4, leading to a total area of 8. The presenter concludes by reflecting on the process, noting that while the sketch may not have been perfect, the method of breaking down the problem into separate integrals for distinct regions was effective.
Mindmap
Keywords
💡Area between curves
💡Intersection points
💡Factoring by grouping
💡Antiderivatives
💡Definite integral
💡Absolute value
💡Linear function
💡Cubic function
💡Graph
💡Multiple regions
💡Integration
Highlights
The video focuses on finding the area between two curves with multiple regions involved, specifically y = x^3 - 3x^2 and y = x - 3.
The approach starts by finding the intersection points of the two curves to understand their interaction.
The intersection points are found by setting y equal to y and solving the resulting equation.
The algebraic manipulation involves factoring by grouping and factoring further to find the intersection points at x = 3, x = -1, and x = 1.
The graph of the cubic function has a bounce at x = 0 and the linear function is a straight line.
The area between the curves is divided into two regions, one where the cubic is on top and another where the linear curve is on top.
To find the total area, two separate integrals are calculated and then added together.
The first integral is calculated from x = -1 to x = 1 where the cubic curve is above the linear curve.
The second integral is calculated from x = 1 to x = 3 where the linear curve is above the cubic curve.
The antiderivative of the first integral is found and evaluated from -1 to 1, resulting in an area of 4.
The antiderivative of the second integral is found and evaluated from 1 to 3, also resulting in an area of 4.
The total area between the curves is the sum of the two calculated areas, which is 8.
An alternative method using absolute value in the integral can be used with a calculator for a quicker result.
However, the video recommends working out the integrals by hand for a better understanding of the problem.
The process involves simplifying the integral expressions and evaluating them at the bounds.
The video emphasizes the importance of sketching the region and finding intersection points for a clearer understanding.
The final result confirms that both regions have the same area, and the total area is accurately calculated as 8.
The video provides a comprehensive guide to dealing with areas between curves with multiple regions and intersection points.
Transcripts
Browse More Related Video

Area Between y=x^3 and y=3x-2 | MIT 18.01SC Single Variable Calculus, Fall 2010
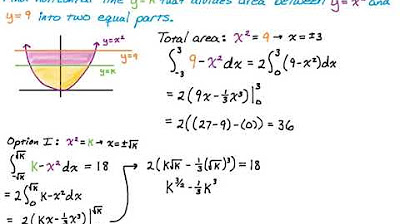
Finding a Horizontal Line (y=k) to Divide a Region into Two Equal Parts Using Calculus

Area Between Curves: Integrating with Respect to y (Example 2)

Area Between Curves: y = cos(pi*x), y = 4x^2 -1
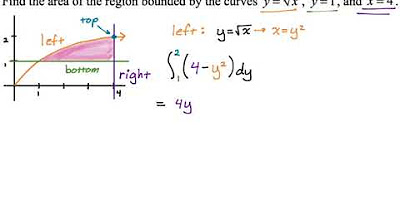
Area Between Curves: Integrating with Respect to y (Example 3)
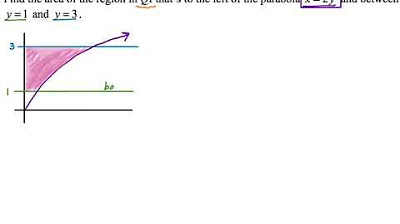
Area Between Curves: Integrating with Respect to y (Example 1)
5.0 / 5 (0 votes)
Thanks for rating: