Marginal and Average Cost
TLDRThe video script discusses the concepts of marginal cost, average cost, and marginal average cost in the context of business economics. It explains that marginal cost is the derivative of the cost function, representing the additional cost of producing one more unit, while average cost is the total cost divided by the number of units produced. Using examples, the script illustrates how to calculate these costs at specific production levels, such as 110 days of rental or 17 hours of operation. It also highlights the economic principle that it's preferable to operate when marginal cost is less than average cost, as this indicates that producing an additional unit will reduce the average cost. The script further introduces the concept of marginal average cost, which is the derivative of the average cost function, indicating how the average cost changes with each additional unit produced. The examples provided show calculations for total cost, average cost, marginal cost, and marginal average cost, emphasizing the importance of understanding these cost dynamics for efficient business operations.
Takeaways
- 📈 Marginal Cost is the derivative of the cost function and represents the additional cost of producing one more item.
- 🔢 Average Cost is calculated by dividing the total cost of producing x number of items by the number of items.
- ✅ To find the marginal cost at a specific point, evaluate the marginal cost function at that point.
- 💡 The average cost function can be simplified to help find the average cost at a specific number of items or days.
- 💲 At 110 days of rental, the marginal cost is $20, indicating the additional cost of renting one more cabin.
- 📊 The average cost at 110 days of rental is $24.03, which is derived from the total cost divided by 110 days.
- 🆚 Marginal cost is preferred to be less than average cost for economic efficiency, as it drives down the average cost.
- 🟢 To find where average cost equals marginal cost, set the average cost function equal to the marginal cost and solve for x.
- 🔍 At 112 days, the average cost equals the marginal cost, which is a key point for operational decision-making.
- 📉 Operating below 110 days is favorable since the marginal cost is less than the average cost in that range.
- 🧮 The total cost at 17 hours is calculated using the cost function without needing to find a derivative.
- 📌 The average cost at 17 hours is $2.87, which is close to the marginal cost of $2.88, indicating a slight difference.
Q & A
What is the marginal cost and how is it derived?
-Marginal cost is the derivative of the cost function, representing the additional cost of producing one more item. It is derived by taking the derivative of the total cost function with respect to the quantity produced.
How is the average cost function defined?
-The average cost function is defined as the total cost of producing 'x' number of items divided by the number of items 'x'. It simplifies to a function where the total cost is divided by the quantity.
What does it mean when the marginal cost is less than the average cost?
-When marginal cost is less than the average cost, it indicates that the cost of producing one additional unit is less than the average cost of all units produced so far. This situation is generally preferred in economics as it suggests that the average cost will decrease with further production.
How do you calculate the marginal cost at a specific quantity?
-To calculate the marginal cost at a specific quantity, you first find the marginal cost function by taking the derivative of the total cost function. Then, you evaluate this function at the specific quantity of interest.
What is the significance of finding where the average cost equals the marginal cost?
-Finding where the average cost equals the marginal cost is significant because it identifies the point at which the average cost is minimized. It is a key concept in cost analysis and economic decision-making.
How do you determine the preferred operating range for a company?
-A company's preferred operating range is determined by where the marginal cost is less than the average cost. Operating in this range means that the cost of producing an additional unit will reduce the overall average cost.
What is the formula for calculating the total cost at a specific number of hours?
-The total cost at a specific number of hours is calculated using the cost function, which is a function of time (or hours in this case). You simply substitute the specific number of hours into the cost function to find the total cost.
How is the average cost at a given time calculated?
-The average cost at a given time is calculated by taking the cost function, which represents the total cost as a function of time, and dividing it by the number of hours.
What is the marginal average cost and how is it found?
-The marginal average cost is the derivative of the average cost function. It indicates how the average cost changes when producing one more unit. It is found by differentiating the average cost function with respect to the quantity.
Why is it important to understand the relationship between marginal cost and average cost?
-Understanding the relationship between marginal cost and average cost is important because it helps in making economic decisions about production levels. It can indicate optimal production points and inform pricing strategies.
What does it imply if the marginal cost and average cost are very close in value?
-If the marginal cost and average cost are very close in value, it suggests that the cost of producing an additional unit is nearly the same as the average cost of all units produced. This can be a sign of operating near an economically efficient point.
Outlines
📊 Understanding Cost Functions: Marginal and Average Costs
This paragraph introduces the concepts of marginal cost, average cost, and marginal average cost. It explains that marginal cost is the derivative of the cost function, representing the additional cost of producing one more item. The average cost is calculated by dividing the total cost by the number of items produced. The video provides an example where the cost function is given by x^2 - 200x + 544, and the marginal cost function is derived as 2x - 200. It then calculates the marginal cost at 110 days of rental as $20. The average cost function is simplified to x - 200 + (12,544/x), and the average cost at 110 days of rental is found to be $24.03. The video concludes by discussing the economic principle that it's preferable to operate when the marginal cost is less than the average cost and shows how to find the point where the two costs are equal.
🔍 Calculating Total and Average Costs at 17 Hours
The second paragraph focuses on calculating the total cost and average cost at 17 hours of operation using a given cost function. The total cost at 17 hours is found by substituting 17 into the cost function, resulting in $48.80. The average cost is then calculated by dividing the cost function by the number of hours (h), yielding an average cost of $2.87 at 17 hours. The paragraph also introduces the concept of marginal cost as the derivative of the cost function, which in this case is 3 - (1/h) - 8. The marginal cost at 17 hours is calculated to be $2.88, which is nearly the same as the average cost, indicating that operating at this point is efficient as the cost of an additional hour slightly raises the average cost.
📉 Marginal Average Cost and Its Impact on Operational Decisions
The third paragraph delves into the concept of marginal average cost, which is the derivative of the average cost function and indicates how the average cost changes with one more unit of production. The average cost function from the previous example is simplified to 3 - log(h) - (h/h), and its derivative, the marginal average cost, is calculated at 17 hours, resulting in $0.12. This value suggests a slight increase in the average cost with each additional hour of operation. The video emphasizes the importance of understanding the relationship between marginal cost and average cost for making informed operational decisions, particularly when aiming to minimize costs and maximize efficiency.
Mindmap
Keywords
💡Marginal Cost
💡Average Cost
💡Cost Function
💡Derivative
💡Economic Efficiency
💡Total Cost
💡Natural Logarithm
💡Marginal Average Cost
💡Optimal Operating Range
💡Revenue
💡Production Quantity
Highlights
Marginal cost is the derivative of the cost function and represents the additional cost of producing one more item
Average cost is the total cost of producing x items divided by the number of items
The marginal cost function is found by taking the derivative of the cost function, which is 2x - 200 in this example
The marginal cost at 110 days of rental is calculated to be $20
The average cost function is derived by dividing the total cost function (x^2 - 200x + 544) by the number of days x
The average cost at 110 days of rental is found to be $24.03
It is preferable to operate when marginal cost is less than average cost, as it brings down the average cost
The point where average cost equals marginal cost can be found by setting the two functions equal to each other and solving for x
At 112 days of rental, the average cost equals the marginal cost
Operating at 110 days is within the preferred range since marginal cost is less than average cost when x < 112
The total cost at 17 hours is found by evaluating the cost function 3h - ln(h) - 8 at h = 17, which gives $48.80
The average cost at 17 hours of operation is calculated to be $2.87
The marginal cost function is found by taking the derivative of the cost function, which is 3 - 1/h - 8
The marginal cost at 17 hours is found to be $2.88, which is very close to the average cost
Operating when marginal cost is less than average cost is desirable as it decreases the average cost
The marginal average cost tells you how the average cost changes when you produce one more unit
The marginal average cost is found by taking the derivative of the average cost function
At 17 hours, the marginal average cost is calculated to be $0.12
Transcripts
Browse More Related Video

Marginal Revenue, Average Cost, Profit, Price & Demand Function - Calculus
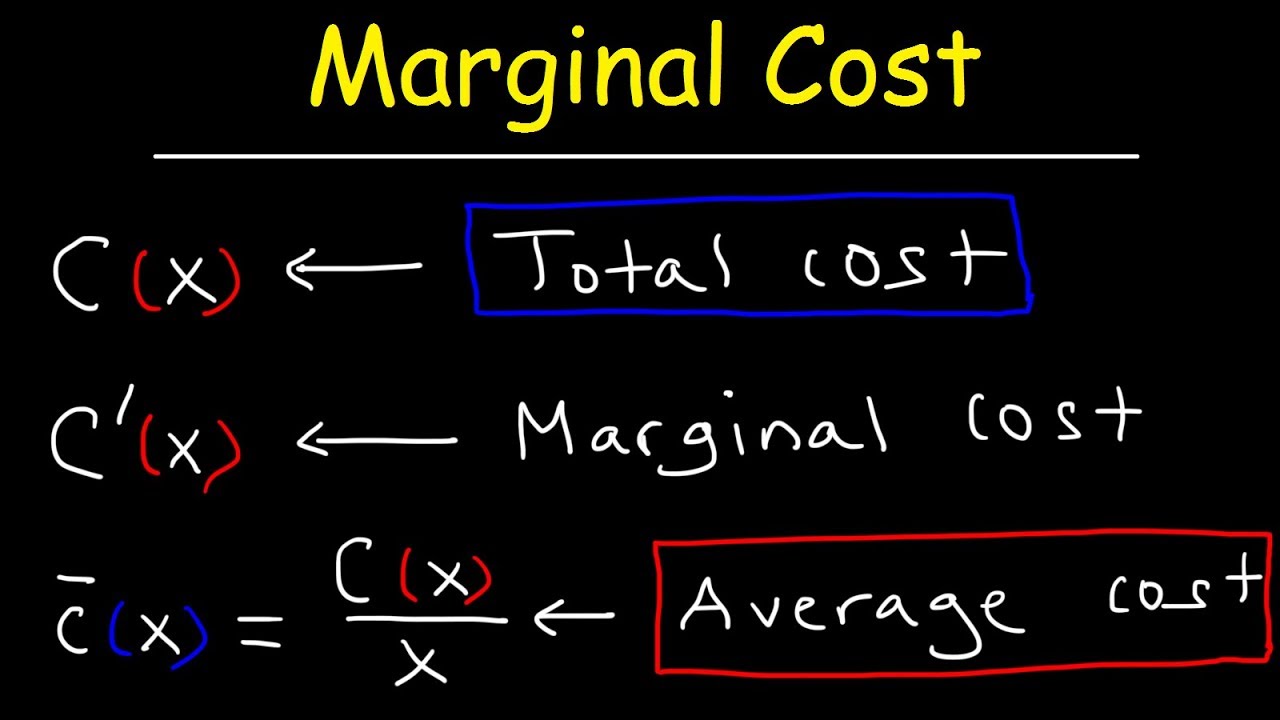
Marginal Cost and Average Total Cost
Business Calculus - Math 1329 - Section 2.5 - Marginal Analysis and Differentials

Marginal cost & differential calculus | Applications of derivatives | AP Calculus AB | Khan Academy

Marginal Cost, Marginal Revenue, and Marginal Profit
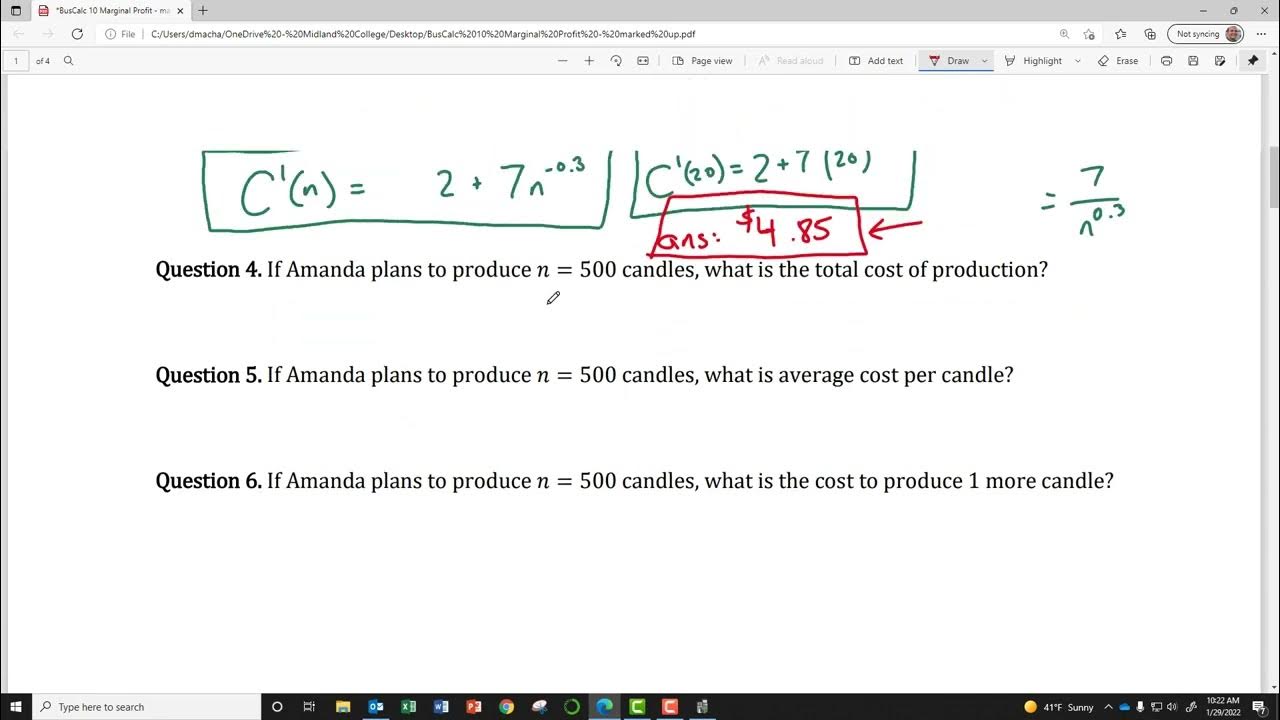
BusCalc 10 Marginal Profit
5.0 / 5 (0 votes)
Thanks for rating: