Math 1325 Lecture 12 4
TLDRThis lecture focuses on applying integrals to business problems, specifically looking at how profit is calculated as revenue minus cost. The concept of marginal profit, revenue, and cost is introduced, with the marginal values being the derivatives of their respective functions. The lecture demonstrates how to find the total cost function given a marginal cost function and fixed costs. It also covers how to maximize profit by setting marginal revenue equal to marginal cost. The process involves integrating the marginal functions to find the original revenue and cost functions, using these to calculate profit, and then finding the production level that maximizes profit. The summary also highlights a common mistake where students may calculate profit but fail to directly answer the specific question asked, such as the level of production for maximum profit.
Takeaways
- 💰 **Profit Fundamentals**: Profit is calculated as revenue minus cost, and it can be positive or negative, with negative profit indicating a loss.
- 📈 **Marginal Analysis**: The marginal profit, revenue, and cost are derived from taking the derivative of their respective functions, indicating the instantaneous change in profit, revenue, or cost.
- ∫ **Integration of Marginals**: By integrating marginal profit, revenue, or cost, one can find the original profit, revenue, or cost functions.
- 🧮 **Total Cost Function**: The total cost function can be found by integrating the marginal cost function and adding a constant that represents fixed costs.
- 🔍 **Fixed Costs**: Fixed costs are expenses that do not change with production levels and are included in the cost function as a constant.
- 🎯 **Profit Maximization**: Profit is typically maximized when marginal revenue equals marginal cost, although this is not always the case.
- 📉 **Revenue and Cost Functions**: The revenue and cost functions can be found by integrating their marginal counterparts and applying relevant constraints, such as fixed costs or production quantities.
- 🤔 **Maximizing Profit**: To find the maximum profit, one must determine the production level where marginal revenue equals marginal cost and then calculate the profit function at that point.
- 📌 **Zero Revenue Concept**: Revenue is zero when no units are sold, which is a concept that should be inherent in understanding revenue functions.
- 🔢 **Algebraic Manipulation**: Finding profit functions and their maxima involves algebraic manipulation of integrated revenue and cost functions.
- 🔑 **Key to Profit Function**: The profit function is derived by subtracting the total cost function from the total revenue function, with careful attention to algebraic signs.
- 📚 **Attention to Detail**: It's crucial to pay attention to the specific question being asked, as demonstrated by the mistake of calculating profit instead of production level for profit maximization.
Q & A
What is the basic formula for profit from a business perspective?
-The basic formula for profit from a business perspective is profit equals revenue minus cost.
What does a negative profit indicate in business?
-A negative profit indicates a loss, meaning the expenses are greater than the revenue, potentially leading to debt.
How is marginal profit related to the profit function?
-Marginal profit is related to the profit function as it is the derivative of the profit function, indicating the instantaneous change in profit.
What is the integral of the marginal cost function used for?
-The integral of the marginal cost function is used to find the original total cost function.
How is the fixed cost related to the cost function in the given example?
-In the given example, the fixed cost is related to the cost function as C(0) equals the fixed cost, which is $10,000 per month, representing the cost incurred regardless of production.
What is the condition for maximizing profit in terms of marginal revenue and marginal cost?
-The condition for maximizing profit is when the marginal revenue equals the marginal cost.
What is the relationship between the profit function and the cost function given the marginal revenue and marginal cost?
-The profit function is calculated as the integral of the marginal revenue minus the integral of the marginal cost.
What is the significance of setting marginal revenue equal to marginal cost?
-Setting marginal revenue equal to marginal cost is a method to find the point where profits are maximized, although it does not always guarantee the absolute maximum profit.
What is the process to find the maximum profit in the given business problem?
-To find the maximum profit, first, set the marginal revenue equal to the marginal cost to find the quantity of items that maximize profit. Then, integrate the marginal revenue and marginal cost to find the revenue and cost functions. Subtract the cost function from the revenue function to get the profit function, and evaluate it at the quantity found earlier.
Why might a business sell items at a loss?
-A business might sell items at a loss to enter a new market, be more competitive by underpricing, or to introduce a product or service to potential customers, with the expectation of reaching a volume where profits can be made.
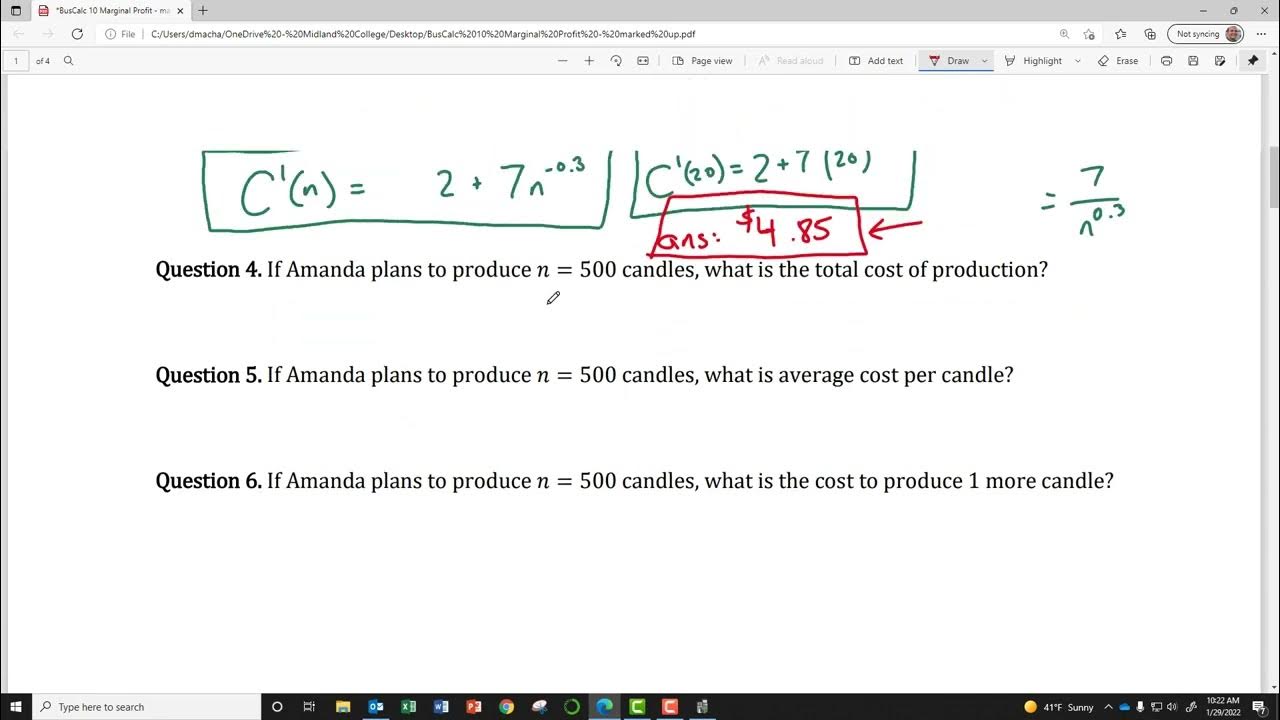
What is the formula for the total cost function in the given example with marginal cost as 3x + 50 and fixed costs of $10,000?
-The total cost function is C(x) = (3/2)x^2 + 50x + 10,000.
How does the shape of the profit function's graph (parabola) indicate the nature of the maximum profit?
-If the profit function's graph is an upside-down parabola (frown), the maximum profit occurs at the vertex of the parabola, which can be found using the formula -b/(2a) where 'b' and 'a' are the coefficients of the quadratic and linear terms, respectively.
Outlines
📚 Introduction to Business Applications of Integrals
The first paragraph introduces the topic of applying integrals to business problems. It emphasizes the fundamental business formula where profit equals revenue minus cost, and how this formula can be applied to personal finance as well. The paragraph also explains the concept of marginal profit, revenue, and cost, which are derived from their respective functions. The integrals of these marginal functions are identified as the original profit, revenue, and cost functions. An example is provided to illustrate how to find the total cost function given a marginal cost function and fixed costs.
🔍 Maximizing Profit with Marginal Analysis
The second paragraph delves into the concept of maximizing profit by equating marginal revenue and marginal cost. It presents a problem where the marginal revenue and cost functions are given, along with the cost to produce a certain number of items. The paragraph outlines the steps to find the profit function by integrating the marginal revenue and cost functions, and then uses this to determine the maximum profit. The process involves setting the marginal revenue equal to the marginal cost to find the production level that maximizes profit, integrating to find the original revenue and cost functions, and then calculating the profit function. The maximum profit is then found by evaluating the profit function at the production level that maximizes profit.
📉 Understanding Profit and Loss in Business Strategy
The third paragraph discusses a scenario where a business might sell products at a loss. It explores reasons why a company might choose to do so, such as entering a new market or becoming more competitive. The paragraph also presents another problem involving a company's weekly production and sales, where the marginal revenue and cost functions are provided, along with the total cost for a specific number of units produced. The goal is to determine the production level that maximizes profit. The solution involves finding the profit function by integrating the marginal revenue and cost, and then identifying the production level that corresponds to the maximum profit. The paragraph emphasizes the importance of carefully reading the question and providing the exact answer requested.
Mindmap
Keywords
💡Integrals
💡Profit
💡Revenue
💡Cost
💡Marginal Profit
💡Marginal Revenue
💡Marginal Cost
💡Derivative
💡Fixed Costs
💡Variable Costs
💡Maximum Profit
💡Quadratic Equation
Highlights
The lecture focuses on applying integrals to business problems, specifically looking at profit maximization through marginal revenue and cost analysis.
Profit is defined as revenue minus cost, which can be positive or negative and is relatable to personal finance.
Marginal profit, revenue, and cost are found by taking the derivative of their respective functions, which represent instantaneous changes.
Integrals are used to find the original profit, revenue, and cost functions from their marginal counterparts.
The total cost function for a product is derived by integrating the marginal cost function and accounting for fixed costs.
Fixed costs are included in the cost function and are independent of production levels.
Profit maximization often occurs where marginal revenue equals marginal cost, although it's not a guaranteed rule.
The profit function is calculated by subtracting the cost function from the revenue function.
The revenue and cost functions are derived from their marginal functions using integration and considering specific given values.
The maximum profit is found by setting marginal revenue equal to marginal cost and solving for the quantity of goods.
The profit function is a quadratic equation, and its maximum value can be found using the vertex formula.
Businesses may operate at a loss initially for strategic reasons, such as entering a new market or becoming competitive.
The weekly production level for profit maximization is determined by setting marginal revenue equal to marginal cost and solving for the number of units.
The profit function is derived by integrating the marginal revenue and subtracting the integrated marginal cost.
To find the maximum profit, the profit function is evaluated at the production level where marginal revenue equals marginal cost.
It's crucial to pay attention to the specific question asked, as calculations may lead to different answers depending on the context.
The lecture emphasizes the importance of understanding the concepts behind the calculations and applying them correctly to business scenarios.
Transcripts
Browse More Related Video

Marginal Revenue, Average Cost, Profit, Price & Demand Function - Calculus

Marginal Cost, Marginal Revenue, and Marginal Profit

Math 1325 Lecture 9 9 - Applications
BusCalc 10 Marginal Profit
Business Calculus - Math 1329 - Section 2.5 - Marginal Analysis and Differentials
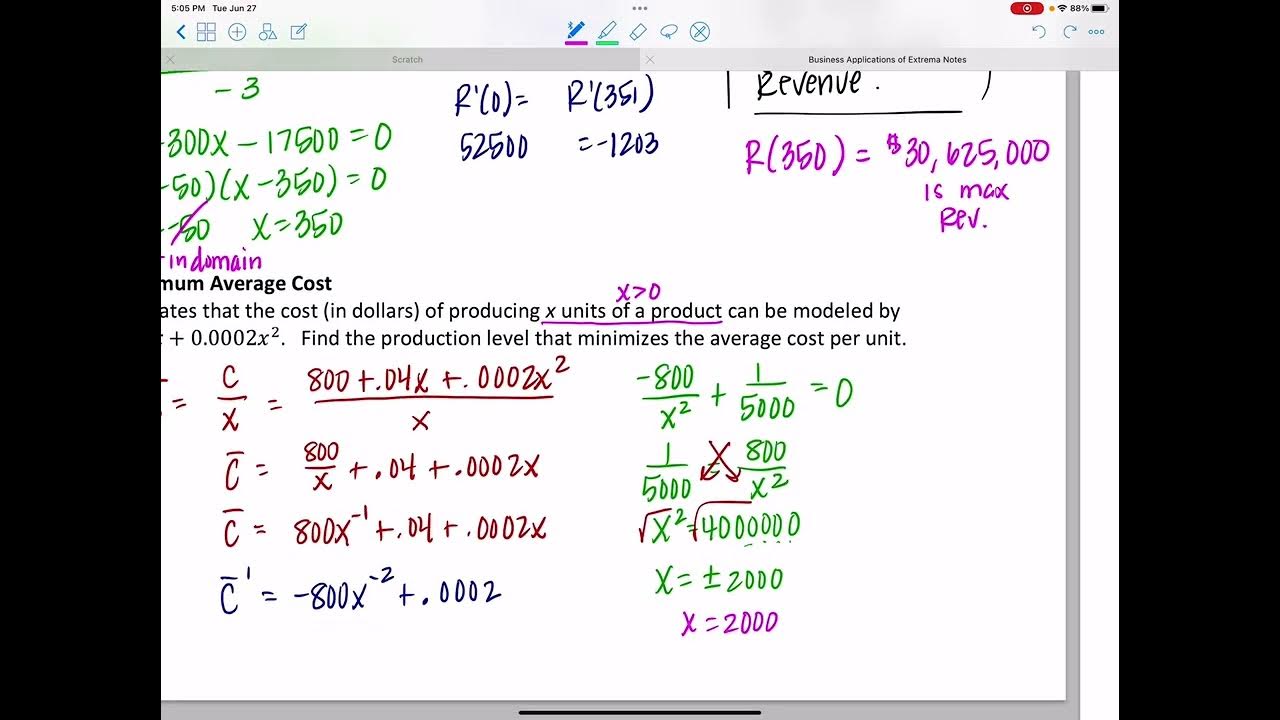
Business Applications of Extrema
5.0 / 5 (0 votes)
Thanks for rating: