Finding a Horizontal Line (y=k) to Divide a Region into Two Equal Parts Using Calculus

turksvids
28 Jan 201906:19
EducationalLearning
32 Likes 10 Comments

TLDRIn this video, the presenter tackles a calculus problem involving finding a horizontal line y = K that equally divides the area between y = x^2 and y = 9. The approach begins with visualizing the region and identifying the intersection points at x = ±3. The total area under y = x^2 from x = -3 to x = 3 is calculated using integration, resulting in 36 square units. Next, the presenter explores two methods to find the value of K. The first method involves integrating with respect to x from the bounds -√(K) to √(K), using symmetry to simplify the calculation, and solving for K to find it equals 27^(2/3). The second, deemed more straightforward by the presenter, integrates with respect to y, considering x as √(y) and -√(y), and solving the resulting integral to again find K as 27^(2/3). The video concludes by emphasizing the utility of integrating with respect to y for certain problems, offering viewers two effective strategies for solving calculus area problems.

Takeaways
  • 📐 The problem involves finding a horizontal line y = K that divides the area between y = x^2 and y = 9 into two equal parts.
  • 🔍 The video begins by suggesting the use of a figure to visualize the problem and understand the region to be divided.
  • 🏁 Intersection points of y = x^2 and y = 9 are at x = -3 and x = 3, which are used as bounds for the integrals.
  • ⚖️ The total area under the curve y = x^2 from x = -3 to x = 3 is calculated to be 36 using symmetry and integration.
  • 🔑 The area under the curve y = K from x = -√K to x = √K is set to be half of the total area, which is 18, to find the value of K.
  • 🧮 The integral for the area under y = K is evaluated using symmetry and the reverse power rule, leading to an equation in terms of K.
  • 🗜️ Simplifying the equation results in K^(3/2) - (1/3)K^(3/2) = 9, which is then solved for K.
  • 💡 The solution for K is found to be (27/2)^(2/3), which is the value that divides the region into two equal parts.
  • 🔄 An alternative method is presented, integrating with respect to y, using x = √y and x = -√y as the bounds for the integral.
  • 📈 The integral with respect to y is easier to handle and results in a simpler equation: 4/3 * K^(3/2) = 18.
  • 📊 The final value of K is the same using both methods, demonstrating the versatility in solving calculus problems.
  • 🎓 The video emphasizes the importance of being comfortable with both dx and dy methods in calculus as they can simplify different types of problems.
Q & A

  • What is the primary goal of the video?

    -The primary goal of the video is to use calculus to find a horizontal line y = K that divides the area between y = x^2 and y = 9 into two equal parts.

  • Why is it helpful to start with a figure when solving this problem?

    -Starting with a figure helps visualize the problem, understand the region to be divided, and identify the intersection points between y = x^2 and y = 9.

  • What are the intersection points of y = x^2 and y = 9?

    -The intersection points are at x = -3 and x = 3.

  • How does one find the total area of the region between y = x^2 and y = 9?

    -The total area is found by integrating from -3 to 3, using the top curve y = 9 and the bottom curve y = x^2, and applying symmetry to simplify the calculation.

  • What is the result of the total area calculation?

    -The total area is 36 square units.

  • How does one find the area of the region below the horizontal line y = K?

    -The area is found by integrating from the bounds -√K to √K with the top curve y = K and the bottom curve y = x^2.

  • What is the equation that represents the area below the horizontal line y = K?

    -The equation is 2/3 * K^(3/2) - 1/3 * K^(3/2) = 9, after applying symmetry and simplifying.

  • What is the value of K that divides the region into two equal parts?

    -The value of K is (27/2)^(2/3).

  • What is the alternative approach to solving the problem by integrating with respect to Y?

    -The alternative approach involves considering x as a function of y (x = √y and x = -√y) and integrating from 0 to K with the integrand (√y - (-√y)) or 2√y.

  • How does the integration with respect to Y simplify the problem?

    -Integrating with respect to Y simplifies the problem by avoiding dealing with the square root of K directly and using the power rule more straightforwardly.

  • What is the final equation obtained by the alternative integration method?

    -The final equation is 4/3 * K^(3/2) = 18, which is used to find the value of K.

  • Why is it beneficial to think in terms of dy when solving calculus problems?

    -Thinking in terms of dy is beneficial because it can simplify the process of integration, especially for problems where the integration variable is more naturally expressed in terms of the dependent variable.

Outlines
00:00
📐 Calculus Problem: Dividing an Area into Equal Parts

The video begins with an introduction to a calculus problem that involves finding a horizontal line y=K that divides the area between y=x^2 and y=9 into two equal parts. The presenter suggests starting with a visual representation of the problem for clarity. The intersection points of y=x^2 and y=9 are identified as -3 and 3, and a new line y=K is introduced to split the area equally. Two different methods are proposed to solve the problem. The first step in both methods is to calculate the total area under the curve y=9 minus the area under y=x^2 from -3 to 3. Using symmetry, the integral is simplified and evaluated to find the total area is 36 square units. The video then moves on to finding the area under the curve y=K, which is done by setting up an integral with bounds determined by the points where x^2 equals K. The integral is evaluated by using symmetry and the power rule, leading to an equation that is solved for K, yielding K = (27/2)^(2/3). The presenter also discusses an alternative method of integrating with respect to Y, which is considered a more straightforward approach by some, and involves integrating from 0 to K and solving for K using a similar process.

05:02
🧮 Alternative Integration Method: Integrating with Respect to Y

The second paragraph introduces an alternative approach to solving the calculus problem by integrating with respect to Y. This method is presented as potentially easier for some viewers, as it involves integrating the area under the curve from 0 to K, considering x as a function of Y (x = √Y and x = -√Y). The integral to be solved is ∫ from 0 to K (Y^(1/2) - (-Y)^(1/2)) dY, which simplifies to 2/3 * Y^(3/2) evaluated from 0 to K. Setting this equal to half of the total area (18), the presenter applies the power rule in reverse to find K. After evaluating the integral and simplifying, the solution for K is found to be the same as in the first method, K = (27/2)^(2/3). The presenter emphasizes the usefulness of integrating with respect to Y, as it can make certain problems more manageable.

Mindmap
Keywords
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and the accumulation of quantities. In the video, calculus is used to find a horizontal line that divides a given region into two equal parts, which is a problem involving the calculation of areas under curves. The script mentions using calculus to set up and solve integrals, which is a fundamental technique in calculus.
💡Horizontal Line
A horizontal line in mathematics is a straight line parallel to the x-axis, and it is often represented by the equation y = K, where K is a constant. In the context of the video, the horizontal line y = K is sought to bisect the area between y = x^2 and y = 9, which is a geometric problem that requires the application of calculus.
💡Area
Area, in mathematics, refers to the amount of space enclosed within a surface or boundary. The primary goal of the video is to find a line that divides the area under two curves into two equal parts. The script discusses calculating the total area under the curves and then finding the area under one curve in terms of K to establish an equation to solve for K.
💡Integration
Integration is a mathematical process that is the reverse of differentiation and is used to find the accumulated value of a function over an interval. In the video, integration is used to calculate the areas under the curves y = x^2 and y = 9, and to find the value of K that divides the total area into two equal parts.
💡Intersection Points
Intersection points are the points where two or more curves, lines, or geometric shapes meet. In the script, the intersection points of y = x^2 and y = 9 are found to be at x = -3 and x = 3, which are crucial for setting up the integrals to calculate the areas of interest.
💡Symmetry
Symmetry in mathematics refers to a property of an object or system where it remains unchanged under a specific transformation, such as reflection or rotation. The video script uses the concept of symmetry to simplify the calculation of the total area by integrating from 0 to 3 and then multiplying by 2, taking advantage of the even nature of the region.
💡Power Rule
The power rule is a fundamental theorem in calculus that allows for the integration of polynomial functions. It states that the integral of x^n, where n is a constant, is x^(n+1)/(n+1) + C. In the video, the power rule is used to simplify the integral expressions when calculating areas under the curves.
💡Bounds
In calculus, bounds refer to the limits of an interval over which an integral is evaluated. The script discusses finding the bounds for the integrals based on the intersection points of the curves and the horizontal line y = K to set up the integrals correctly.
💡Definite Integral
A definite integral is an integral that has a specific start and end point, known as the limits of integration. The video involves setting up and evaluating definite integrals to find the areas under the curves y = x^2 and y = 9 between the specified bounds.
💡Reverse Power Rule
The reverse power rule is a term used to describe the integration technique for powers of x. It is the counterpart to the power rule for differentiation. In the script, the reverse power rule is applied to integrate functions of the form x^n, which simplifies the process of finding antiderivatives.
💡dy
In calculus, dy often represents an infinitesimal change in the variable y. When integrating with respect to y, as an alternative approach suggested in the video, it allows for a different perspective of the problem, which can sometimes simplify the integrals. The script mentions thinking in terms of dy as a valuable skill for solving certain calculus problems.
Highlights

The video uses calculus to find a horizontal line that divides a region into two equal parts.

The problem involves finding the line y = K that splits the area between y = x^2 and y = 9 equally.

A visual representation of the problem is recommended to understand the region's division.

Intersection points of y = x^2 and y = 9 are at x = -3 and x = 3.

The total area of the region between y = x^2 and y = 9 is calculated using integration.

Symmetry is used to simplify the calculation of the total area.

The integral from -3 to 3 of (9 - x^2) with respect to x is evaluated.

The total area is found to be 36 square units.

The area under the curve y = x^2 and above the line y = K is expressed in terms of K.

The intersection points where x^2 = K are found to be ±√K.

The area under y = K and above y = x^2 is calculated using integration from -√K to √K.

The area under y = K is set to be half of the total area, which is 18.

Integration with respect to Y is introduced as an alternative method.

The region is re-visualized with x = √y and x = -√y for integration with respect to Y.

The integral from 0 to K of (√y - -√y) with respect to y is evaluated.

The integral simplifies to 2/3 times K to the three-halves, which equals 18.

Solving for K, the value is found to be 27/2 to the 2/3.

Two different approaches to solving the problem are demonstrated.

The video emphasizes the importance of using calculus and symmetry in problem-solving.

The skill of integrating with respect to Y is highlighted as beneficial for certain types of problems.

Transcripts

Browse More Related Video

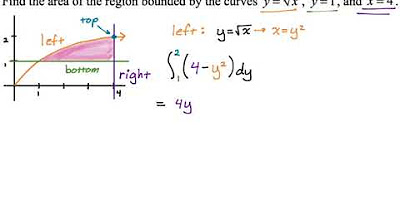
Area Between Curves: Integrating with Respect to y (Example 3)

Area Between y=x^3 and y=3x-2 | MIT 18.01SC Single Variable Calculus, Fall 2010

Area Between Curves: Integrating with Respect to y (Example 2)

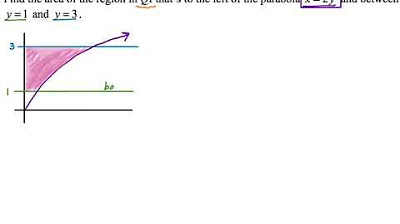
Area Between Curves: Integrating with Respect to y (Example 1)

2012 AP Calculus AB Free Response #2

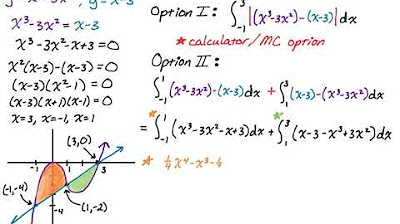
Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegralsArea DivisionMath ProblemsSymmetryPower RuleGeometryProblem SolvingEducational VideoMathematical Analysis