High School Physics: Significant Figures

Dan Fullerton
28 Jun 201103:44
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the concept of significant figures (sig figs), emphasizing their importance in accurately representing the precision of numerical measurements. It explains the process of determining the number of significant figures by first noting all certain digits and then estimating an additional one. The script also clarifies how to read significant figures, highlighting that all nonzero digits are significant, zeros between significant figures are significant, and zeros to the left of the first significant digit are not. The application of these rules is demonstrated through examples, and viewers are encouraged to practice by measuring and expressing the length of a hand, the mass of a pencil, and the time to pat one's head 20 times in significant figures.

Takeaways
  • 📏 The concept of significant figures (sig figs) is introduced as a way to express the precision of numerical measurements.
  • 🔢 The goal is to determine how accurately we know a numerical value by using the correct number of significant figures.
  • 📝 To measure with sig figs, record as many digits as you can with certainty and then estimate one additional digit.
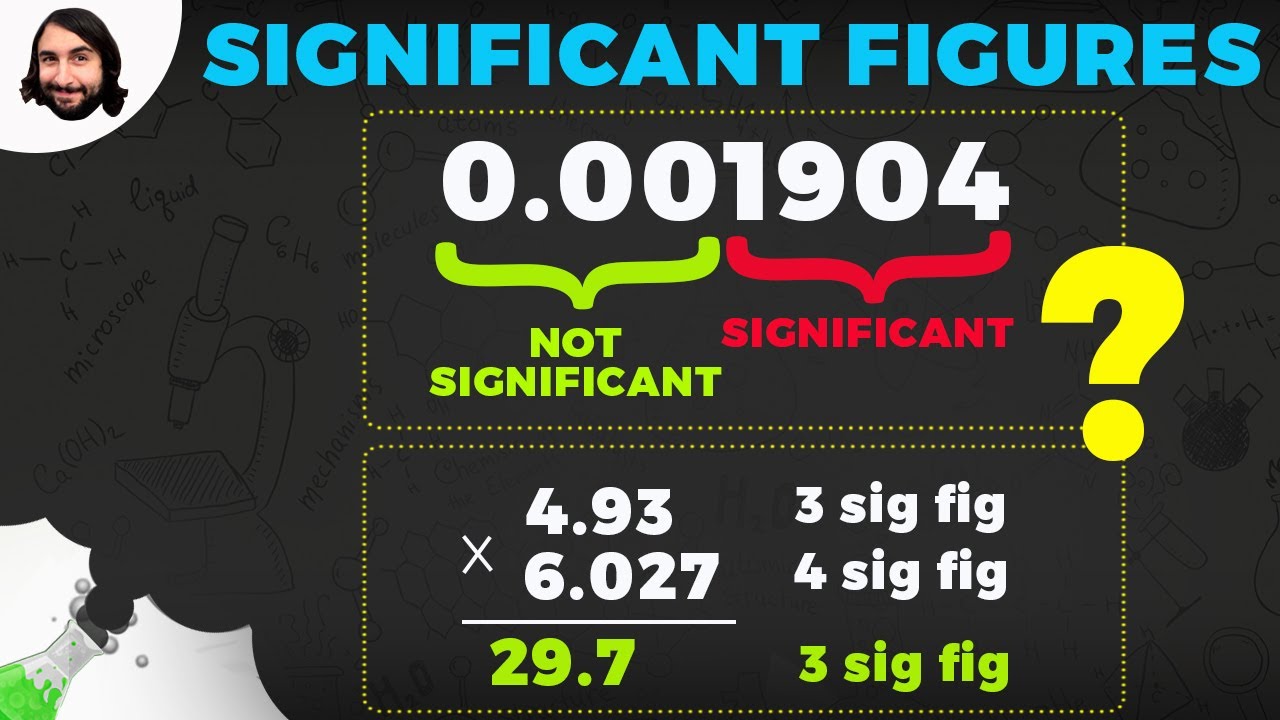
  • 🔍 Reading significant figures involves understanding that all nonzero digits are significant, and zeros between nonzero digits are also significant.
  • 👉 Zeros to the left of significant digits are not significant, while zeros to the right are significant.
  • 🌰 Examples provided in the script help clarify the rules for counting significant figures in various numerical expressions.
  • 📐 The importance of practice is emphasized by encouraging the audience to measure and express the length of their hand, the mass of a pencil, and the time it takes to pat their head 20 times using significant figures.
  • 🎓 The script suggests that using significant figures enhances the accuracy and precision of numerical data.
  • 📈 Scientific notation is mentioned as a context where zeros to the right of significant figures are considered significant.
  • 💡 The script concludes by directing the audience to a plus physics comm for more information, indicating a resource for further learning.
  • 🤔 The exercise of measuring and expressing everyday quantities in significant figures promotes a practical understanding of the concept.
Q & A

  • What is the main purpose of using significant figures in measurements?

    -The main purpose of using significant figures is to express the accuracy and level of certainty of a measured value.

  • How can you determine the number of significant figures in a measurement?

    -You start by writing down as many digits as you can with absolute certainty, and then estimate one more figure if possible. The total number of these digits becomes the number of significant figures in your measurement.

  • What is the rule for reading significant figures in a number presented in scientific notation?

    -In scientific notation, all nonzero digits are significant, and all digits between any nonzero digits are also significant.

  • How do you treat zeros when counting significant figures?

    -Zeros to the left of significant figures are not significant, but zeros to the right of significant figures are significant.

  • What is the difference in significance between the values 43.74 kilometers and 40,334.27 kilometers?

    -The value 43.74 kilometers has four significant figures, while the value 40,334.27 kilometers has more significant figures due to its precision to several decimal places.

  • How many significant figures are there in the measurement '40,334.2.5 grams'?

    -There are five significant figures in this measurement.

  • What is the correct interpretation of zeros in the value '0.0083 seconds'?

    -In the value '0.0083 seconds', there are two significant figures because the zeros to the left of the first significant digit (8) are not counted.

  • How would you express the value '1.200 times 10 to the third kilograms' in terms of significant figures?

    -This value has four significant figures, as the zeros to the right of the significant figure '8' are also counted in scientific notation.

  • What is the practical exercise suggested in the script for practicing significant figures?

    -The script suggests measuring the length of your hand, the mass of a pencil, and the time it takes to pat your head 20 times, and then expressing these measurements in significant figures.

  • What resource is recommended for more information on significant figures?

    -For more information on significant figures, the script recommends checking out a plus physics comm.

  • Why is it important to understand and use significant figures correctly?

    -Understanding and using significant figures correctly is important because it communicates the precision of a measurement and prevents the misinterpretation of data.

Outlines
00:00
📏 Understanding Significant Figures

This paragraph introduces the concept of significant figures, emphasizing their importance in accurately representing numerical values. It explains that significant figures indicate the level of precision and certainty in a measurement. The process of determining significant figures is outlined, starting with noting all known digits and then estimating an additional digit if possible. The paragraph also delves into the method of reading significant figures, highlighting that all nonzero digits are significant, zeros between significant figures are significant, and zeros to the left of the first significant figure are not significant. The explanation is supported with examples to illustrate the rules for counting significant figures in various numerical expressions, including those in scientific notation.

Mindmap
Keywords
💡Significant Figures (Sig Figs)
Significant figures are the digits in a number that are known with a certain level of precision or exactness. They indicate the reliability of a measured value by showing how accurately the number is known. In the context of the video, sig figs are used to express numeric quantities accurately, such as measuring the height of a garden gnome in various ways, from 14 cm to 14.3827482 cm, to demonstrate the concept of certainty in measurement.
💡Measurement
Measurement is the process of determining the size, amount, or degree of something by comparing it with a standard or unit. In the video, measurement is central to understanding significant figures, as it is through measurement that we can determine the accuracy and precision of a value, such as the height of a garden gnome or the length of a hand.
💡Precision
Precision refers to the degree of exactness or the level of detail to which a measurement is accurate. It is closely related to significant figures, as both concepts deal with the reliability of numerical data. The video emphasizes the importance of precision in measurements, explaining how to read and write numbers with the correct number of significant figures to convey the known precision of the measurement.
💡Scientific Notation
Scientific notation is a way of expressing numbers that are too large or too small to be conveniently written in decimal form. It is typically written as a product of a number between 1 and 10 and a power of 10. In the video, scientific notation is used as an example to illustrate how to count significant figures, emphasizing that all nonzero digits are significant and zeros to the right of significant figures are also significant.
💡Zero
The digit zero plays a specific role in significant figures. It is not considered significant unless it is to the right of a nonzero digit or it is the only nonzero digit in the number. The video clarifies that zeros to the left of significant figures are not significant, but zeros to the right are, which affects how we count significant figures in numbers like 43.74 km or 40,334,000.0025 grams.
💡Estimation
Estimation in the context of significant figures involves making an educated guess about an additional digit in a measurement when the exact value is uncertain. The video explains that after writing down as many digits as can be known with certainty, one should estimate one more figure to determine the correct number of significant figures in a measurement.
💡Nonzero Digits
Nonzero digits are any digits in a number that are not zero. According to the rules explained in the video, all nonzero digits in a number are considered significant when determining the number of significant figures. This rule is applied when counting significant figures in both standard and scientific notation.
💡Certainty
Certainty in the context of the video refers to the level of confidence in the accuracy of a measurement or a number. Significant figures are a way to express this certainty, with more significant figures indicating a higher known precision. The video discusses how to express numeric quantities with the correct number of significant figures to convey the known level of certainty.
💡Practice
Practice, as mentioned in the video, is the act of applying the learned concepts to real-world situations. The video encourages viewers to practice measuring objects like the length of their hand, the mass of a pencil, and the time it takes to pat their head 20 times, and then expressing these measurements in significant figures to reinforce their understanding of the concept.
💡Accuracy
Accuracy is the closeness of a measured value to the true or actual value. In the video, accuracy is related to significant figures, as the correct number of sig figs helps to convey how accurately a number is known. The video emphasizes the importance of accuracy in scientific measurements and how significant figures contribute to expressing this accuracy.
Highlights

The introduction of significant figures (sig figs) and their importance in expressing the accuracy of numerical values.

The method of determining the number of significant figures by writing down as many digits as possible with absolute certainty and estimating one additional figure.

The example of measuring a garden gnome's height in various ways to illustrate the concept of significant figures.

The rule for reading significant figures in scientific notation: all nonzero digits are significant, and all digits between nonzero digits are also significant.

The explanation that zeros to the left of significant figures are not significant, while zeros to the right are significant.

An example of determining the number of significant figures in the value 'forty-three point seven four kilometers', resulting in four significant figures.

The example of counting significant figures in 'forty, three four thousand three hundred and 2.5 grams', which contains five significant figures.

The clarification that zeros to the left of significant figures in a number like 'point zero zero eight three seconds' do not count as significant, resulting in two significant figures.

The example of expressing the value 'one point two zero zero times 10 to the third kilograms' with four significant figures in scientific notation.

The comparison between writing a value as '1.2 times 10 to the third kilograms' versus 'one point two zero zero times 10 to the third kilograms', highlighting the difference in the number of significant figures expressed.

The practical application of measuring and expressing the length of one's hand, the mass of a pencil, and the time to pat one's head 20 times using significant figures.

The encouragement to practice measuring and expressing data in significant figures for better accuracy and understanding.

The reference to a plus physics comm for more information on the topic of significant figures.

Transcripts

Browse More Related Video

Measurement and Significant Figures

What are Significant Figures, Precision & Accuracy in Chemistry & Physics?

Significant Figures Step by Step | How to Pass Chemistry

College Physics 1: Lecture 3 - Significant Figures and Scientific Notation

Rounding and Order of Operations Used in Significant Figures

2.1 Significant Figures | High School Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Numerical PrecisionMeasurement TechniquesScientific NotationAccuracy in DataEducational ContentPhysics FundamentalsMathematical ConceptsEstimation SkillsPractical ApplicationPlus Physics