Rounding and Order of Operations Used in Significant Figures
TLDRThe video script provides an essential guide to rounding numbers and significant figures (sig figs) in both math and chemistry. It emphasizes the importance of proper rounding to avoid errors that could impact exam scores. The rules for rounding are explained, highlighting the need to look at the digit to the right of the target rounding place to decide whether to round up or down. The script then delves into the rules for determining significant figures, with examples illustrating each rule. It also covers the significance of zeros in different contexts. The video further explains the application of significant figures in operations like addition, subtraction, multiplication, and division, stressing the importance of adhering to the least number of decimal places or significant figures. The script concludes with a discussion on the order of operations (PEMDAS) and how it applies to chemistry problems, providing examples to solidify understanding. The summary also encourages viewers to practice these concepts with the provided problems and video explanations.
Takeaways
- 📝 Knowing how to round numbers is crucial in math and chemistry to avoid losing points on exams.
- 🔢 When rounding, always look at the digit to the right of the place you are rounding to determine if you should round up or down.
- 📉 If the digit is less than 5, round down, and if it's 5 or greater, round up.
- 🧪 In chemistry, you often round to the appropriate number of significant figures (sig figs) rather than just to a certain decimal place.
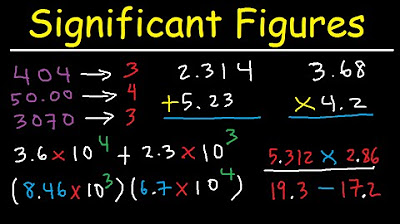
- 📍 Rule 1: All non-zero numbers are significant.
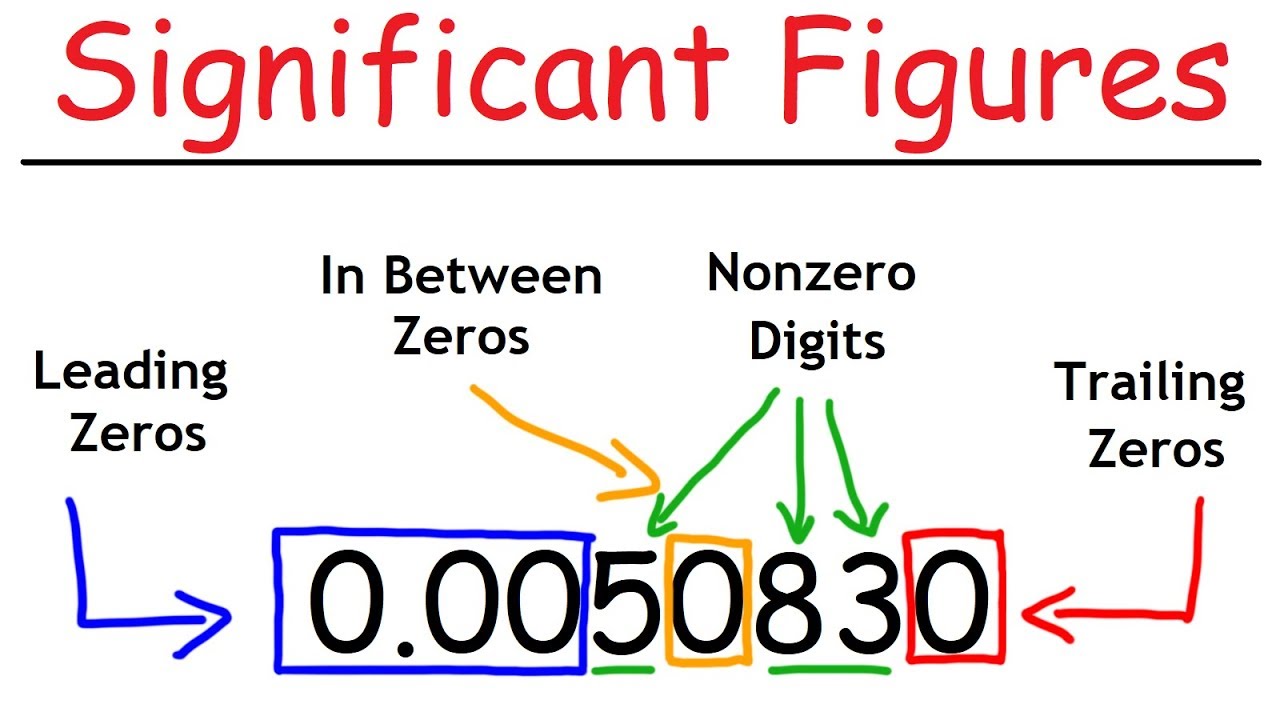
- 📍 Rule 2: Zeros between non-zero numbers are significant.
- 📍 Rule 3: Zeros after a decimal point are significant.
- 📍 Rule 4: Coefficients in scientific notation are significant, but the exponent is not.
- 📍 Rule 5: Leading zeros in a number without a decimal are not significant.
- 📍 Rule 6: Zeros within a number without a decimal are not significant unless they are between non-zero digits.
- ➕ When adding or subtracting, round to the least number of decimal places.
- ✖️ When multiplying or dividing, round to the least number of significant figures.
- 🔢 For complex problems, follow the order of operations (PEMDAS) and apply the rounding rules at each step.
- ✅ Always round at the end of a calculation, not after each step.
- 🔗 Practice problems are available with step-by-step video explanations through a link in the description.
Q & A
What is the primary importance of rounding numbers correctly in math and chemistry?
-Rounding numbers correctly is crucial because incorrect rounding can lead to loss of points in exams and inaccurate results in calculations.
When rounding a number, what should you consider to decide whether to round up or down?
-You should look at the digit to the right of the place value you are rounding to. If it's less than 5, round down, and if it's 5 or greater, round up.
What is the rule for significant figures when it comes to zeros that are in the middle of non-zero numbers?
-Zeros that are between non-zero numbers are considered significant figures.
How are significant figures determined when a number is in scientific notation?
-In scientific notation, only the coefficient (the number in front of the exponent) is considered for significant figures, not the exponent itself.
When rounding a number to a certain number of significant figures, what is the common mistake made and how can it be avoided?
-A common mistake is to round the number down too much, significantly changing the value of the original number. To avoid this, ensure that you do not round to a point where the value of the number is altered beyond recognition, like confusing $341 with $34.
What is the rule for adding and subtracting numbers in terms of significant figures?
-When adding or subtracting, you must round to the least number of decimal places present in all the numbers involved in the operation.
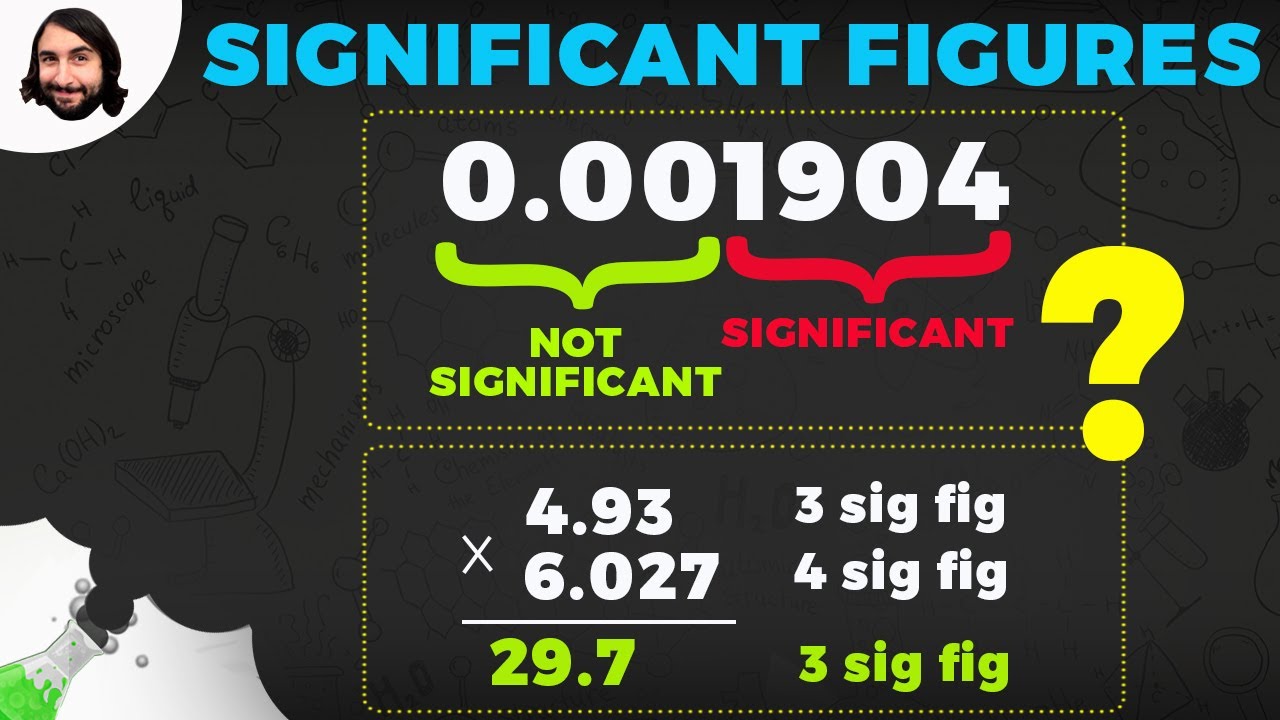
How do you handle significant figures when multiplying or dividing numbers?
-For multiplication and division, you round to the least number of significant figures between the two numbers being multiplied or divided.
What is the basic order of operations in mathematics known as?
-The basic order of operations is known as PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right).
When performing calculations that involve a combination of addition, subtraction, multiplication, and division, what should you remember to do at the end of the calculation?
-You should remember to round to the appropriate number of significant figures at the very end of the calculation, after performing all operations according to PEMDAS.
How do you approach a calculation that requires both multiplication and division according to PEMDAS?
-According to PEMDAS, you should perform multiplication and division operations from left to right, regardless of their order in the sequence.
What is the significance of understanding the order of operations (PEMDAS) in the context of chemistry calculations?
-Understanding PEMDAS is crucial in chemistry as it ensures the correct sequence of operations is followed, which directly affects the accuracy of significant figures in the final answer.
How can you practice the concepts of rounding and significant figures with problems that are explained step-by-step?
-You can find practice problems with step-by-step video explanations by using the link provided in the video description.
Outlines
📚 Rounding Numbers and Significant Figures in Math and Chemistry
This paragraph introduces the importance of rounding numbers correctly in mathematics and chemistry to avoid losing points on exams. It explains the basic rules for rounding to the nearest whole number by looking at the digit to the right of the target rounding place. The concept of significant figures (sig figs) is introduced as crucial for chemistry problems, with rules outlined for determining them. Common mistakes are highlighted, such as not changing the value of the original number when rounding to sig figs. The paragraph concludes with an example of rounding a number to two sig figs and the option to express the number in scientific notation for the same purpose.
🔢 Significant Figure Rules and Arithmetic Operations
The second paragraph delves into the rules for significant figures in arithmetic operations. It emphasizes that when adding or subtracting, results should be rounded to the least number of decimal places present in the operands. An example is provided to illustrate this process. For multiplication and division, the rule is to round to the least number of significant figures. An example demonstrates this by identifying the significant figures of the operands, performing the operation, and then rounding to the least number of significant figures. The paragraph also reviews the basic order of operations (PEMDAS) and provides examples of how to apply it correctly, cautioning against common mistakes like performing addition before division.
🧪 Applying Order of Operations and Significant Figures in Chemistry
The final paragraph focuses on the application of order of operations and significant figure rules in chemistry problems. It advises to always perform calculations according to the order of operations and to consider significant figure rules throughout the process. An example is given where multiplication and subtraction are combined, showing how to round results according to the least number of significant figures or decimal places as appropriate. The paragraph concludes with an encouragement to practice these concepts with provided problems and to return for further instruction in subsequent videos.
Mindmap
Keywords
💡Rounding
💡Significant Figures (Sig Figs)
💡Scientific Notation
💡Order of Operations (PEMDAS)
💡Decimal Places
💡Coefficient
💡Leading Zeros
💡Zero as a Placeholder
💡Multiplication and Division
💡Addition and Subtraction
💡Contextualizing Numbers
Highlights
Rounding numbers is crucial in math and chemistry to avoid losing points on exams.
When rounding, always consider the digit to the right of the rounding place to decide whether to round up or down.
If the digit to the right is less than five, round down; if it's five or more, round up.
In chemistry, rounding is often to the appropriate number of significant figures (sig figs), which is critical for the accuracy of chemistry problems.
Rule one for significant figures: Every non-zero number is significant.
Rule two: Zeros between non-zero numbers are significant.
Rule three: Zeros after a decimal point are always significant.
Rule four: Coefficients in scientific notation are significant, but the exponent is not.
Rule five: Leading zeros in a number without a decimal are not significant.
Rule six: Zeros within a whole number are not significant unless there's a decimal point.
Common mistake: Not rounding to the correct number of significant figures can drastically change the value of a number.
When adding or subtracting, round to the least number of decimal places.
For multiplication and division, round to the least number of significant figures.
PEMDAS is the order of operations for solving mathematical problems: Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).
In complex problems, apply PEMDAS and significant figure rules in sequence, considering the operation being performed.
Always round at the very end of a calculation to maintain the correct number of significant figures.
Practical exercises are available through the provided link for additional practice on rounding and significant figures.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: