Significant Figures Step by Step | How to Pass Chemistry

Melissa Maribel
24 Jul 201704:56
EducationalLearning
32 Likes 10 Comments

TLDRIn this video, Melissa Maribel explains six rules for determining significant figures, or sig figs, which help gauge the accuracy of measurements. Key rules include: non-zero numbers and zeros between them are significant, zeros after a decimal are significant, leading zeros are not, and zeros in large numbers without a decimal are not significant. For addition and subtraction, focus on the least decimal places; for multiplication and division, consider the least sig figs. Melissa provides examples and practice problems to reinforce these concepts, aiming to help students master Chemistry with ease.

Takeaways
  • 🔢 Significant figures (sig figs) are the digits in a number that indicate its precision and accuracy.
  • 💡 Rule 1: All non-zero digits are considered significant.
  • 🌟 Rule 2: Zeros between non-zero digits are significant.
  • 📉 Rule 3: Zeros after a decimal point are significant.
  • 🌌 Rule 4: Zeros in scientific notation are significant.
  • ❌ Rule 5: Leading zeros are not significant.
  • 🔍 Rule 6: Zeros in large numbers without a decimal point are not significant.
  • ➕ When adding or subtracting, align the decimal points and round to the least number of decimal places.
  • ✂️ For multiplication and division, round to the number with the fewest significant figures.
  • 📚 Practice is key; the video offers additional practice problems and step-by-step solutions.
  • 👍 Encouragement to like and subscribe for more educational content.
  • 🎓 The channel aims to help students succeed in Chemistry.
Q & A

  • What are significant figures and why are they important?

    -Significant figures, or sig figs, are the digits within a number that help determine the accuracy of a data value or measurement. They are important because they indicate the precision of the measurement and prevent misrepresentation of data.

  • What is the first rule for determining significant figures in a number?

    -The first rule states that all non-zero numbers are significant. Every digit in a non-zero number is considered significant.

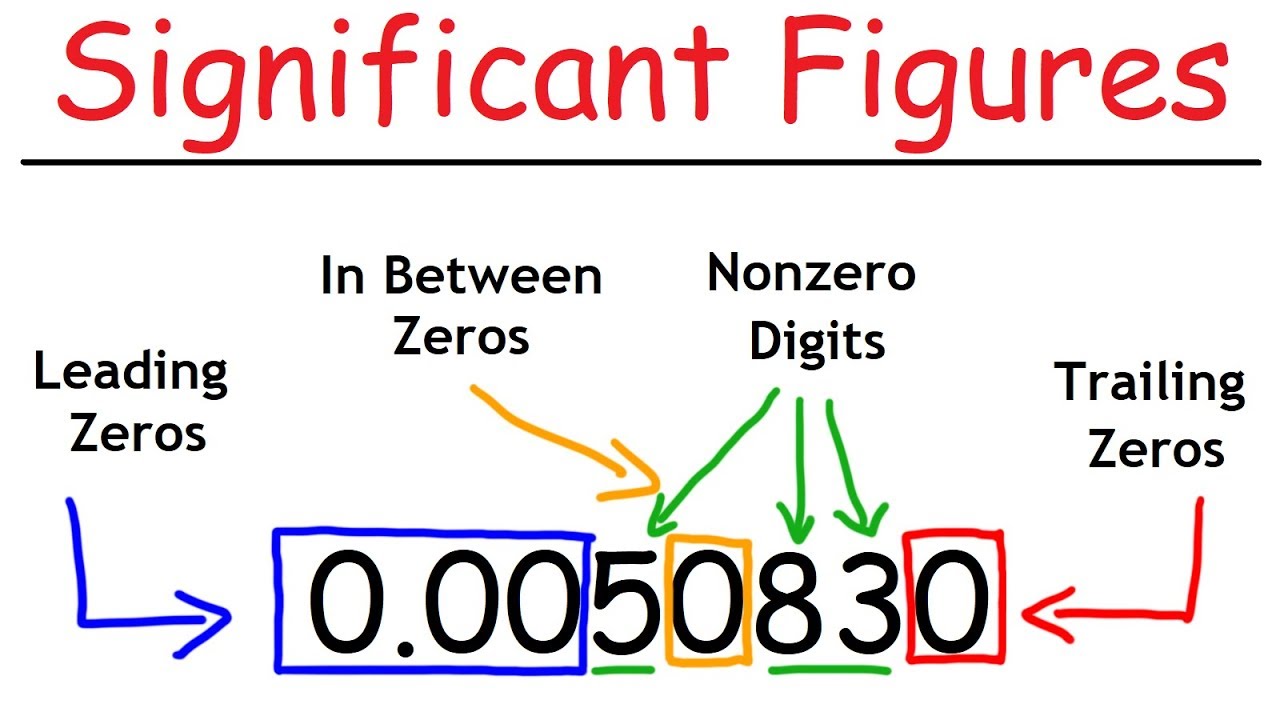
  • How do you treat zeros that are in the middle of non-zero numbers?

    -Any zeros that are in the middle of non-zero numbers are significant. For example, in the number 5208, the zeros between the 5 and the 8 are significant.

  • Are zeros after the decimal point always significant?

    -Yes, zeros after the decimal point are always significant. They indicate precision up to a certain place value.

  • How are zeros treated in scientific notation?

    -In scientific notation, any zeros that are part of the coefficient (the number before the multiplication sign) are significant.

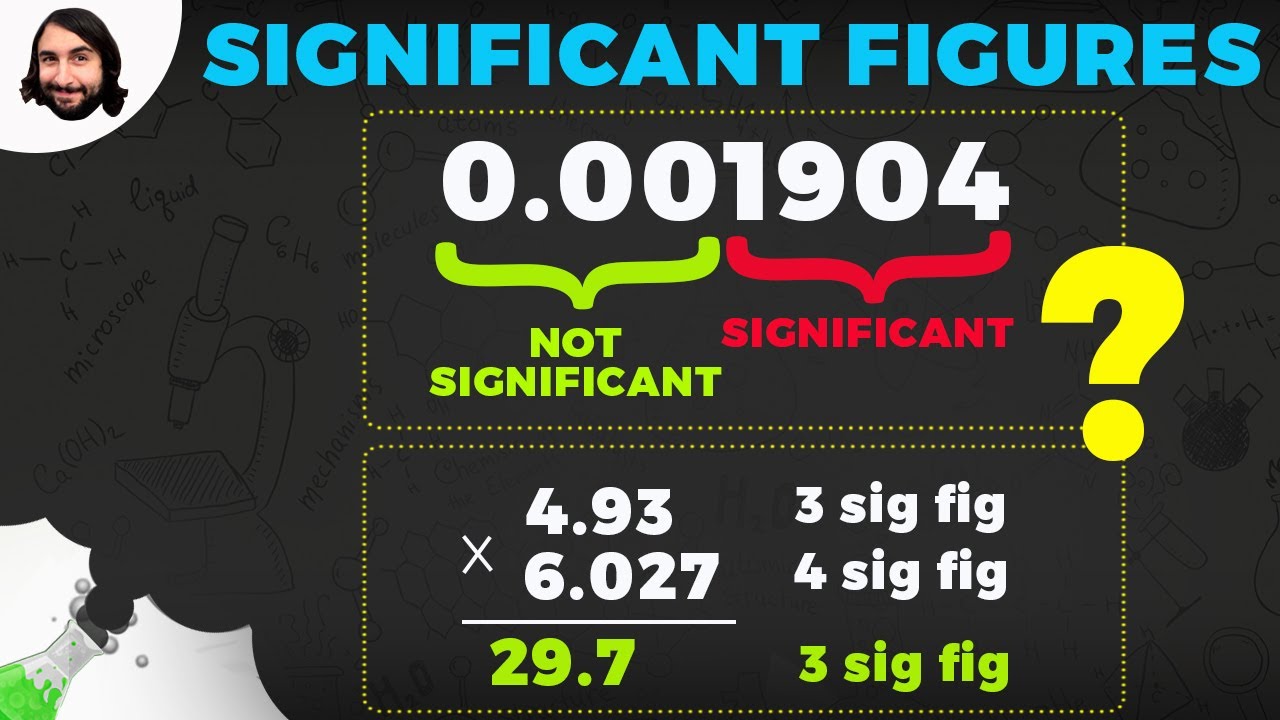
  • What about leading or beginning zeros in a number, are they significant?

    -Leading or beginning zeros in a number are not significant. They are placeholders and do not contribute to the accuracy of the measurement.

  • How do you determine the significance of zeros in a large number without a decimal?

    -Zeros in a large number without a decimal are not significant. Only the non-zero digits count towards the significant figures.

  • When adding and subtracting numbers, what determines the number of significant figures in the result?

    -When adding and subtracting, you look for the least amount of decimal places among the numbers involved. The result should be rounded to the same number of decimal places as the number with the least.

  • In multiplication and division, how do you determine the number of significant figures in the result?

    -When multiplying and dividing, you look for the least amount of significant figures among the numbers involved. The result should be rounded to the same number of significant figures as the number with the least.

  • What should you do if there is a zero in the last place of a number after multiplication?

    -If there is a zero in the last place after multiplication, and there is no decimal, you should consider the significant figures based on the non-zero digits and ignore the trailing zero.

  • Where can I find additional practice problems and step-by-step answers related to significant figures?

    -Additional practice problems and step-by-step answers can be found in the description box of the video, where a link is provided.

Outlines
00:00
📚 Understanding Significant Figures

In this educational video, Melissa Maribel introduces the concept of significant figures and their importance in determining the accuracy of data values or measurements. She explains six key rules for identifying significant figures, including the significance of non-zero numbers, zeros between non-zero digits, zeros after a decimal point, and zeros in scientific notation. She also clarifies that leading zeros and zeros within large numbers without a decimal are not significant. Additionally, Melissa provides guidance on how to handle significant figures during arithmetic operations like addition, subtraction, multiplication, and division, emphasizing the importance of rounding to the correct number of significant figures. The video concludes with an invitation for viewers to practice with provided problems and to subscribe for more educational content.

05:00
🎥 Behind-the-Scenes Bloopers

This section captures a light-hearted moment from the video production, where Melissa Maribel is interrupted by the cameraman regarding her hair. She playfully denies any action and thanks a 'random hand' for assistance, adding a touch of humor to the educational content. The blooper ends with Melissa laughing and flipping her hair, showcasing the casual and friendly tone of the video series.

Mindmap
Keywords
💡Significant Figures
Significant figures, often abbreviated as 'sig figs', are the digits in a number that carry meaningful information about the precision of a measurement or data value. In the video, they are the core concept, as the entire script revolves around explaining how to determine and work with them. For example, the script explains that all non-zero digits are significant, and zeros between non-zero digits are also significant, as seen in the number '508' where both zeros are considered significant.
💡Decimal Place
The decimal place refers to the position in a number that comes after the decimal point and is used to indicate the precision of the number. In the context of the video, zeros immediately following the decimal point are considered significant. For instance, in the number '5.000', all three zeros are significant because they come after the decimal place.
💡Scientific Notation
Scientific notation is a way of expressing very large or very small numbers in the form of a × 10^n, where a is a number greater than or equal to 1 and less than 10, and n is an integer. In the video, it is mentioned that any zeros and the coefficient in scientific notation are significant figures. For example, the number '8.52 × 10^3' has four significant figures: '8', '5', '2', and the zero.
💡Leading Zeros
Leading zeros are zeros that appear at the beginning of a number and do not affect its value. The video emphasizes that leading zeros are not considered significant in determining the accuracy of a number. For example, the number '0.04' has only one significant figure, the '4', despite the presence of two leading zeros.
💡Large Number
A large number, in the context of significant figures, refers to a number without a decimal point that has trailing zeros. The video clarifies that zeros within a large number without a decimal are not significant. For example, in the number '4000', only the '4' is significant, and the three trailing zeros are not.
💡Rounding
Rounding is the process of adjusting a number to the nearest value with a certain number of significant figures. The video explains that when adding and subtracting, you look for the least amount of decimal places to determine how to round the final answer, such as rounding '5004' to '5488' to maintain four significant figures.
💡Multiplication
In the context of significant figures, multiplication involves determining the total number of significant figures in the result by considering the number with the least significant figures. The video illustrates this by showing that when multiplying numbers with different amounts of significant figures, you round to the number with the fewest, such as rounding the result of multiplying '3.14' and '2' to three significant figures.
💡Division
Division, like multiplication, requires attention to significant figures when calculating results. The video suggests that when dividing, you should also consider the number with the least significant figures to determine the precision of the result, ensuring that the final answer reflects the accuracy of the least precise number.
💡Practice Problems
Practice problems are exercises provided to help viewers apply and reinforce their understanding of significant figures. The video encourages viewers to try practice problems to check their understanding and offers a link in the description for additional practice with step-by-step solutions.
💡Accuracy
Accuracy in the context of significant figures refers to the degree of closeness of a measured or calculated value to the true value. The video's theme revolves around understanding significant figures to ensure the accuracy of scientific measurements and calculations. For example, knowing which digits are significant helps in correctly interpreting the precision of a measurement like '3.1415' versus '3.14'.
💡Chemistry
Although not explicitly defined in the script, the mention of 'pass Chemistry' suggests that the video's content is particularly relevant to the field of chemistry, where precision in measurements is crucial. The video aims to help students understand significant figures to improve their performance in chemistry, a subject where such concepts are fundamental.
Highlights

Significant figures determine the accuracy of a data value or measurement.

All non-zero numbers are significant.

Zeros between non-zero numbers are significant.

Zeros after the decimal point are significant.

Zeros in scientific notation are significant.

Leading zeros are not significant.

Zeros in large numbers without a decimal are not significant.

When adding and subtracting, find the least amount of decimal places.

Align decimals when adding and rounding to the least decimal place.

Rounding involves looking at the number to the right of the rounding place.

When multiplying and dividing, find the least amount of significant figures.

Count significant figures in each number before multiplication or division.

After multiplying, remove unnecessary decimal places.

Practice problems are available in the description box.

A recap of significant figures and their rules is provided.

The importance of understanding significant figures for accuracy in Chemistry.

Engagement with the audience to provide help in Chemistry.

A humorous moment with a hair flip and a random hand.

Transcripts

Browse More Related Video

Measurement and Significant Figures

Rounding and Order of Operations Used in Significant Figures

What are Significant Figures, Precision & Accuracy in Chemistry & Physics?

Significant Figures - A Fast Review!

High School Physics: Significant Figures

2.1 Significant Figures | High School Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Significant FiguresEducational ContentChemistry BasicsAccuracy in NumbersScientific NotationDecimal PlacesMathematics RulesMeasurement PrecisionStudent GuidanceLearning Tips