AP Physics Workbook 2.J Modified Atwood Machines

Mr.S ClassRoom
23 Apr 202009:17
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript discusses a modified Atwood machine scenario from the AP Physics workbook, focusing on dynamics and the interaction of forces. It describes an object (Big M) hit by a bat, moving on a rough surface under the influence of gravity, friction, and tension from a hanging mass (M). The session explains how to draw Freebody diagrams for two different motion scenarios: the object's initial rightward movement and subsequent leftward return. It emphasizes the direction of frictional force relative to the object's motion and how the forces contribute to the system's overall acceleration. The analysis concludes with a discussion on deriving the system's acceleration and sketching a velocity-time graph, highlighting the impact of friction and tension forces on the object's velocity.

Takeaways
  • 📚 The session discusses a modified Atwood machine involving two masses, with one mass (Big M) being struck by a bat and moving on a rough surface.
  • 🏎️ The block with mass Big M starts at rest at position x=0 and moves to the right with an initial speed V₀ after being hit by the bat.
  • 📈 The block experiences friction as it slides on the rough surface, which causes it to slow down and eventually stop at position x=d after time T=2t.
  • 🔄 Upon stopping, the block returns to its original position x=0 due to the pull of the hanging mass M.
  • 🎨 Two Freebody diagrams are used to represent the forces acting on the block at different points in its motion - one when the block is moving to the right and another when it starts moving to the left.
  • ⚖️ Vertical forces on the block include the normal force (upward) and gravitational force (downward), while horizontal forces include tension (leftward) and friction (opposite to the direction of motion).
  • 🔧 The friction force is always opposite to the direction of the block's motion, and the tension force is always in the direction of the string's pull.
  • 📊 The acceleration of the system is derived by considering the net force (sum of horizontal forces) divided by the total mass (M + m) of the system.
  • 📉 The block's velocity-time graph is characterized by an initial decrease due to friction, followed by a less steep negative slope as the block returns to its starting position.
  • 🤔 The acceleration is greatest before the block reaches x=d because the tension and friction forces are in the same direction, adding up to increase the magnitude of the negative acceleration.
  • 📝 The script provides an example of how to calculate and understand the forces and acceleration in a modified Atwood machine scenario, which is useful for AP Physics exam preparation.
Q & A

  • What is the basic setup of an Atwood machine?

    -An Atwood machine consists of two masses, one on a table and one hanging mass, connected by a string that passes over a pulley.

  • How does the modified Atwood machine in the transcript differ from the basic setup?

    -The modified Atwood machine has a block of mass 'Big M' that is hit by a bat, initiating its movement, and it's connected to a hanging mass 'M' via a string over an ideal pulley.

  • What are the forces acting on the moving block in the horizontal direction?

    -The horizontal forces acting on the moving block are the force of tension (to the left) and the friction force (opposite to the direction of the block's movement).

  • What is the significance of the coefficient of kinetic friction in this scenario?

    -The coefficient of kinetic friction represents the roughness of the surface the block is sliding on, causing it to slow down and eventually stop at a certain position.

  • Why does the block return to its original position after stopping at x = d?

    -The block returns to its original position due to the tension force exerted by the hanging mass, which pulls the block back towards the left.

  • How does the direction of the friction force change when the block's motion changes?

    -When the block's motion changes from right to left, the friction force changes direction from left to right, opposing the new direction of motion.

  • What is the relationship between the direction of the string's tension force and the block's motion?

    -The tension force is always in the direction of the string's pull, which is opposite to the block's motion. In the given scenario, the tension force is to the left because the string is being pulled down by the hanging mass.

  • How does the magnitude of friction force compare in the two scenarios described in the script?

    -In the first scenario, the friction force has a larger magnitude because it is acting in the same direction as the tension force, adding to the overall deceleration. In the second scenario, the friction force has a smaller magnitude as it is acting against the tension force, reducing the overall acceleration.

  • What is the acceleration of the system in the described scenario?

    -The acceleration of the system is negative, calculated by the net force (negative FT + friction force) divided by the total mass (M + m), where 'M' is the mass of the hanging mass and 'm' is the mass of the moving block.

  • How does the velocity of the block change over time according to the script?

    -The block's velocity starts at V_nought, decreases due to friction until it stops at x = d, and then becomes negative as it slides back towards the original position. The acceleration changes the velocity, with a greater deceleration before reaching x = d and a smaller deceleration afterward.

  • What should be considered when sketching a graph of the block's velocity as a function of time?

    -When sketching the graph, the positive direction should be to the right, and the forces acting on the block should be labeled. The velocity starts high, decreases as it moves to the right due to friction, stops at x = d, and then decreases less rapidly as it moves back to the left.

Outlines
00:00
📚 Introduction to AP Physics Workbook - Dynamics and Atwood Machine

This paragraph introduces the AP Physics workbook focusing on Unit 2, Dynamics. It explains a modified Atwood machine scenario where a mass (Big M) is hit by a bat, initiating its motion to the right with an initial speed (V naught). The mass slides on a rough surface, experiencing friction, and is connected to a hanging mass (M) via a string over an ideal pulley. The front end of the block stops at point x equals d after time T equals 2t. The paragraph also discusses the forces acting on the system, including normal force, gravity, tension, and friction, and sets the stage for drawing Freebody diagrams to analyze the motion.

05:02
📈 Analysis of Forces and Acceleration in the Atwood Machine

This paragraph delves into the analysis of forces in the Atwood machine, including the force normal, force of friction, and tension force. It explains how these forces are calculated and combined to derive the system's gravitational acceleration. The paragraph emphasizes the direction of friction force relative to the object's motion and how the tension force always acts in the direction of the string's pull. It also discusses the system's acceleration, highlighting that the greatest acceleration occurs before the block reaches x equals d. The paragraph concludes with an explanation of how to derive the acceleration for the two scenarios and how to answer related questions effectively.

Mindmap
Keywords
💡Atwood Machine
An Atwood Machine is a simple experimental setup used to study the dynamics of pulleys and friction. It typically consists of two masses connected by a string that passes over a pulley. In the video, a modified version of this machine is discussed, where one mass is struck by a bat, initiating motion. The Atwood Machine is central to the video's theme as it is the apparatus through which the principles of dynamics and friction are explored.
💡Dynamics
Dynamics is a branch of physics that deals with the motion of objects and the forces that cause these motions. In the context of the video, dynamics is the core concept being explored, as it relates to the movement of the masses in the Atwood Machine and how they are affected by external forces such as friction and tension.
💡Coefficient of Kinetic Friction
The coefficient of kinetic friction is a value that represents the frictional force between two surfaces in motion relative to each other. It quantifies the amount of friction that resists the motion of an object. In the video, this coefficient is crucial in determining how the rough surface affects the sliding motion of the block in the Atwood Machine.
💡Freebody Diagrams
Freebody diagrams are graphical representations that show all the forces acting on an object in isolation. They help in visualizing and analyzing the forces involved in a physical scenario. In the video, Freebody diagrams are used to represent the forces on the block in the Atwood Machine during its motion to the right and left.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. It is a vector quantity that indicates how quickly an object speeds up, slows down, or changes direction. In the video, the concept of acceleration is used to analyze the motion of the block in the Atwood Machine and how it is affected by the forces acting upon it.
💡Tension Force
Tension force is the force transmitted through a string, rope, or cable when it is pulled tight by the masses or objects it connects. In the video, the tension force in the string plays a critical role in the interaction between the two masses in the Atwood Machine, causing one mass to pull the other and affecting the motion of the system.
💡Friction Force
Friction force is the resistive force that opposes the relative motion or tendency of such motion of two surfaces in contact. It arises from the interactions between the surfaces and acts to prevent or slow down the motion. In the video, the friction force is a key factor in slowing down the block's motion on the rough surface of the Atwood Machine.
💡Velocity
Velocity is a vector quantity that describes the speed of an object in a given direction. It is a fundamental concept in physics used to describe motion. In the video, velocity is used to analyze the change in the block's motion over time in the Atwood Machine, particularly how it starts, decreases, and becomes negative as it moves in opposite directions.
💡Gravitational Acceleration
Gravitational acceleration, often denoted by 'g', is the acceleration experienced by an object due to the force of gravity. It is approximately 9.81 m/s^2 near the Earth's surface. In the context of the video, gravitational acceleration is not directly discussed, but it is an underlying concept that would affect the weight of the masses in the Atwood Machine and thus the tension in the string.
💡Force of Normal
The force of normal is the reactive force exerted by a surface as a response to a force being applied to it. It is a perpendicular force that acts against the weight of an object resting on the surface. In the video, the force of normal is one of the vertical forces acting on the block in the Atwood Machine, balancing out the force of gravity.
Highlights

The session focuses on dynamics, specifically using a modified Atwood machine to explore motion and forces.

In the modified Atwood machine, a block of mass 'Big M' is struck by a bat, initiating its motion.

The block starts at position x=0 with an initial speed V_naught and moves on a rough surface, introducing friction.

The block is connected to a hanging mass 'M' through a string that passes over an ideal pulley.

The block reaches point x=d and stops after time T=2t, indicating a change in motion due to the hanging mass.

The block's motion includes both rightward and leftward movements, influenced by the forces acting upon it.

Friction always opposes the direction of motion, pointing in the opposite direction to the movement of the object.

The tension force is always in the direction of the string's pull, which can vary depending on the object's motion.

The acceleration of the system is derived from the forces acting on the block, considering both vertical and horizontal components.

The block experiences the greatest acceleration before reaching x=d, where the force of tension and friction act in the same direction, increasing the acceleration's magnitude.

The velocity of the block is a function of time, starting at V_naught and decreasing due to friction, then becoming negative as it slides back.

The graph of the block's velocity versus time will show an initial decrease in velocity due to friction, followed by a less steep negative slope as it returns.

Understanding the interplay between forces such as tension, friction, and gravity is crucial for analyzing the system's motion.

The modified Atwood machine provides a practical application for studying dynamics and the effects of different forces on motion.

The session's exploration of the modified Atwood machine offers insights into the principles of dynamics, with potential applications in various fields of physics and engineering.

The detailed analysis of the block's motion and the forces acting on it serves as a valuable example for students learning about dynamics and problem-solving techniques.

Transcripts

Browse More Related Video

AP Physics Workbook 2.E Newton's Second and Third Laws

Free Body Diagrams - Tension, Friction, Inclined Planes, & Net Force

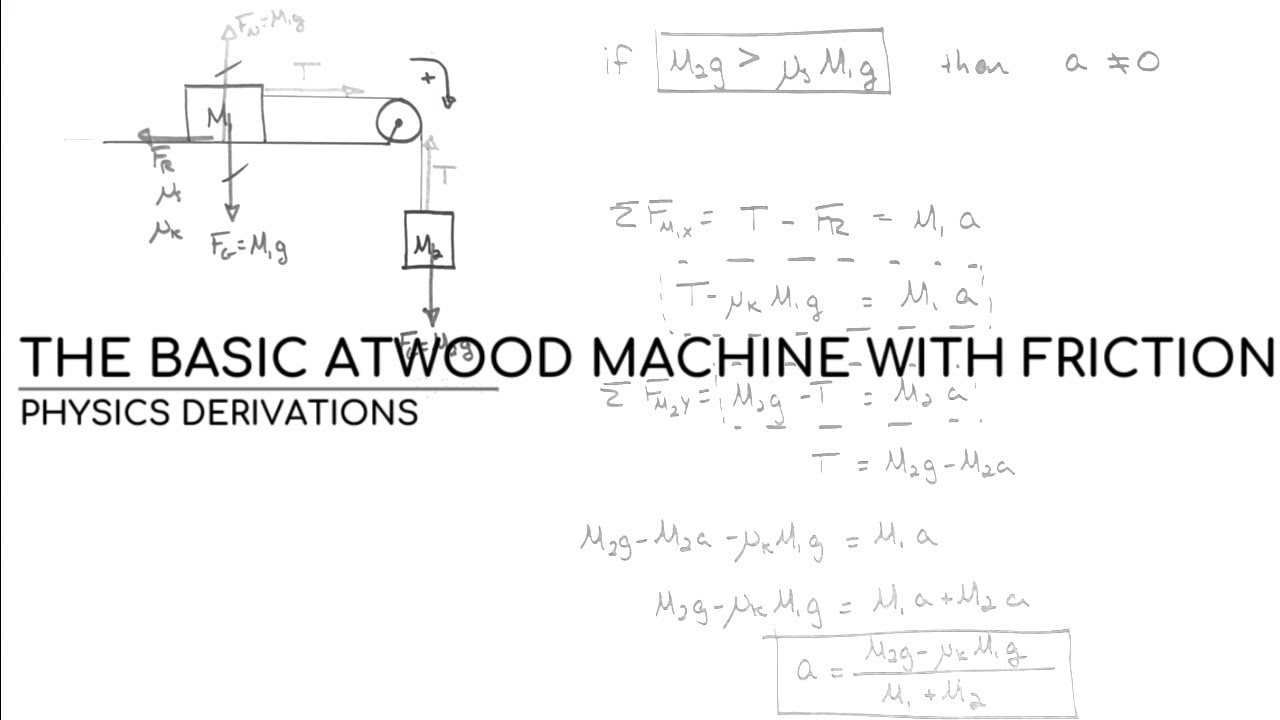
The Basic Atwood Machine With Friction

AP Physics Workbook 2.K Acceleration of Systems

GCSE Physics Revision "Newton's First Law of Motion"

AP Physics Workbook 2.F Direction of Friction

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationAtwoodMachineForceAnalysisFrictionAccelerationAPWorkbookModifiedSystemDynamicsFreebodyDiagramsKineticFric