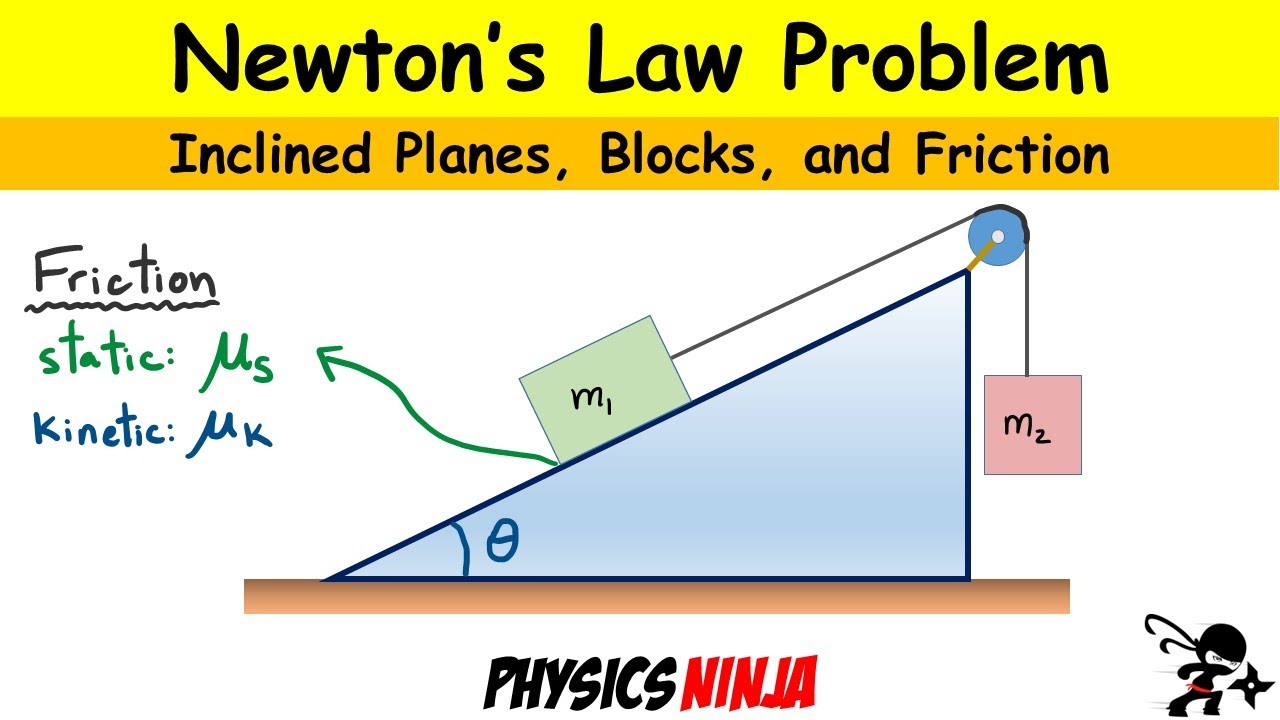
The Basic Atwood Machine With Friction

INTEGRAL PHYSICS
28 Sept 202012:01
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores the dynamics of a modified Atwood machine with the introduction of friction between the block and the horizontal surface. It explains the conditions for the system to move and calculates the acceleration of the system if it does. The script details the forces acting on both blocks, the role of static and kinetic friction, and uses Newton's second law to derive the equations for the system's motion. The analysis is aimed at understanding the balance of forces and the resultant acceleration, highlighting the impact of varying friction coefficients on the system's behavior.

Takeaways
  • 📚 The Atwood machine is a classic physics problem involving two masses connected by a string over a pulley.
  • 🔄 This analysis introduces friction into the Atwood machine scenario, specifically between the block on the surface and the horizontal plane.
  • 🔢 Two coefficients of friction are considered: the static friction (μs) and the kinetic friction (μk).
  • 🚦 A positive direction of motion is established for the analysis, with the block on the surface moving to the right and the hanging block moving downward.
  • ⚖️ The system will move if the weight of the hanging block (m2g) is greater than the static friction force between the surface block and the horizontal surface.
  • 📈 Newton's second law is applied to each block to derive equations for the forces acting on them and their resulting accelerations.
  • 🔄 The tension in the string is an unknown variable (T), which is the same at both ends of the string in this frictionless pulley model.
  • 📌 When the system is at rest, the forces are balanced, and the weight of the hanging block equals the frictional force opposing it.
  • 🚀 If m2g > μs * m1g, the system will accelerate, and the problem transitions from static to kinetic friction as the block starts moving.
  • 📝 The acceleration of the system is found by solving the two equations derived from Newton's second law for the two blocks.
  • 🔍 Reducing the kinetic friction coefficient (μk) to zero simplifies the problem to the basic, frictionless Atwood machine.
Q & A

  • What is the basic Atwood machine setup described in the script?

    -The basic Atwood machine setup described in the script consists of a block on a horizontal surface connected by a massless string that runs over a frictionless pulley to a second block hanging from the string.

  • What is the main difference between the basic Atwood machine and the one described in the script?

    -The main difference is that the Atwood machine described in the script includes friction between the block on the horizontal surface and the surface itself, which is not present in the basic Atwood machine.

  • What are the two types of friction mentioned in the script, and how do they differ?

    -The two types of friction mentioned are static friction (μs) and kinetic friction (μk). Static friction is the frictional force when the block is not moving, while kinetic friction comes into play once the block starts sliding.

  • How does the presence of friction affect the system's potential to move?

    -The presence of friction affects the system's potential to move by introducing a resistance that must be overcome for the system to start moving. If the weight of the hanging block (m2g) is greater than the static friction force (μs times the normal force), the system will accelerate.

  • What are the positive directions of motion established for the blocks in the script?

    -The positive direction of motion for the block on the horizontal surface is to the right, and for the hanging block, it is downward.

  • What are the forces acting on the hanging block in the script?

    -The forces acting on the hanging block are gravity (m2 times g acting downward) and the tension in the string acting upward (denoted as T).

  • What is the relationship between the tensions at the两端 of the string in a frictionless pulley system?

    -In a frictionless pulley system, the tensions at the两端 of the string are always the same because the string has no mass and there is no friction.

  • How does the friction force acting on the block on the horizontal surface differ when the system is at rest versus when it is moving?

    -When the system is at rest, the friction force is static (μs times the normal force). When the system is moving, the friction force becomes kinetic (μk times the normal force).

  • What are the conditions for the system to start moving?

    -The system will start moving if the weight of the hanging block (m2g) is greater than the static friction force (μs times the normal force).

  • How is the acceleration of the system determined in the presence of friction?

    -The acceleration of the system is determined by applying Newton's second law to each block, considering the net force acting on them, and solving the resulting equations simultaneously.

  • What happens to the problem if the coefficient of kinetic friction (μk) is reduced to zero?

    -If the coefficient of kinetic friction (μk) is reduced to zero, the problem simplifies to the basic frictionless Atwood machine, where the presence of friction is no longer a factor.

Outlines
00:00
📚 Introduction to the Atwood Machine with Friction

This paragraph introduces the basic concept of an Atwood machine, which is a system consisting of two blocks connected by a massless string over a frictionless pulley. The unique aspect of this machine is the introduction of friction between the block on the horizontal surface and the surface itself. The coefficient of friction is described with two different values: one for static friction (μs) and one for kinetic friction (μk). The goal of this section is to derive an equation to determine if the system will move and, if so, to calculate the system's acceleration as it moves forward. The positive direction of motion is established, with the first block moving to the right and the hanging block moving downward, and an analysis of the forces acting on each block is conducted to understand the dynamics of the problem.

05:00
🔍 Analyzing the Conditions for System Motion

In this paragraph, the analysis focuses on the conditions required for the system to move. It explains that if the friction is large enough to prevent the top block from moving, the system will remain in a state of equilibrium with forces balanced. The condition for the system to move is that the weight of the hanging block (m2g) must be greater than the static friction force (μs times the normal force). If this condition is met, the system will accelerate. The paragraph also discusses the transition from static to kinetic friction once the system starts moving and how this affects the overall problem. The equations for the forces acting on each block are set up, but the focus is on finding the acceleration of the system rather than calculating the tension in the string.

10:05
🧮 Calculating System Acceleration with Friction

This paragraph delves into the calculation of the system's acceleration if the conditions for motion are met. It emphasizes the role of static friction in determining whether the system will accelerate and kinetic friction in calculating the actual acceleration. The net force acting on the system is determined by the weight of the hanging block (m2g) acting in the positive direction and the friction force (μk times m1g) acting in the negative direction. The resulting equation shows the net force acting on the system, which causes acceleration. The paragraph concludes by noting that if friction is reduced to zero, the problem simplifies to the basic frictionless Atwood machine. The conditions for motion and the actual acceleration for motion have been determined, providing a comprehensive understanding of the Atwood machine with friction.

Mindmap
Keywords
💡Atwood Machine
The Atwood Machine is a classic physics experiment that demonstrates the concepts of mechanical advantage and the conservation of energy. In the video, the Atwood Machine consists of two blocks connected by a massless string over a frictionless pulley. The main theme revolves around analyzing the system's motion when friction is introduced, which adds complexity to the analysis.
💡Friction
Friction is the force that resists the relative motion or tendency of such motion of two surfaces in contact. In the context of the video, friction is introduced between the block on the horizontal surface and the surface itself, with two types considered: static friction, which prevents the block from starting to move, and kinetic friction, which acts when the block is in motion.
💡Coefficient of Friction
The coefficient of friction is a dimensionless scalar value that represents the ratio of the frictional force between two bodies and the normal force between them. It is symbolized by mu (μ) and comes in two forms: μs for static friction and μk for kinetic friction. In the video, these values are crucial for determining whether the system will move and for calculating the system's acceleration.
💡Tension
Tension is a force that is transmitted through a string, rope, cable, or wire when it is pulled tight by forces acting from opposite ends. In the Atwood Machine, tension in the string plays a critical role in the motion of the two blocks. The video explains that tension is a reaction to the weights of the blocks and the frictional force acting on the system.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. It is a vector quantity that describes how quickly an object speeds up, slows down, changes direction, or some combination of these. In the video, the goal is to find the acceleration of the system when one block is on a frictional surface and the other hangs from the string connected to the first block.
💡Static Friction
Static friction is the frictional force that acts on objects in contact but not moving relative to each other. It resists the initiation of sliding motion between two surfaces. In the video, static friction is the force that must be overcome for the block on the horizontal surface to start moving.
💡Kinetic Friction
Kinetic friction, also known as dynamic friction, is the frictional force that resists the relative motion of two surfaces in contact while they are in motion. It is usually less than the maximum static friction but still opposes the motion. In the video, kinetic friction is what acts on the block once it starts moving.
💡Newton's Second Law
Newton's Second Law of Motion states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. It is commonly expressed as F = ma, where F is the net force, m is the mass, and a is the acceleration. The video uses this law to analyze the forces on each block and to derive the acceleration of the system.
💡Positive Direction
A positive direction is a chosen direction in which motion or a vector quantity is considered positive. In physics problems, it is a convention to select a positive direction to simplify the analysis of motion and forces. In the video, the positive direction is defined as to the right for the block on the surface and downward for the hanging block.
💡Equilibrium
Equilibrium in physics refers to a state in which all forces acting on an object are balanced, resulting in no acceleration. In the context of the video, if the friction is large enough to prevent the block on the surface from moving, the system is in equilibrium as the forces balance out and there is no net force causing motion.
💡Net Force
Net force is the vector sum of all the individual forces acting on an object. It is the force that is responsible for changing the motion of the object according to Newton's Second Law. In the video, the net force on the system is the difference between the gravitational force exerted by the hanging block and the frictional force resisting the motion of the block on the surface.
Highlights

Introduction to the basic Atwood machine with friction.

Establishing a positive direction of motion for the system.

Deriving an equation to determine if the system will move under the influence of friction.

Calculating the acceleration of the system when it moves forward.

Explaining the role of the coefficient of friction, with mu_s for static and mu_k for kinetic friction.

Analyzing the forces acting on each block in the presence of friction.

Describing the balance of forces when the system is at rest due to sufficient friction.

Condition for the system to start moving: m2g must be greater than the static friction force.

Using Newton's second law to find the acceleration of block m1 in the x-axis.

Applying Newton's second law to block m2 in the y-axis to find the system's acceleration.

Rearranging the equation for tension and substituting it to find the system's acceleration.

Understanding the role of static friction in the initiation of motion.

Identifying kinetic friction as the force to consider when calculating the actual acceleration.

The net force on the system determines the acceleration when m2g overcomes friction.

The impact of reducing mu_k to zero on the problem's simplification to a frictionless Atwood machine.

Summarizing the conditions for motion and the actual acceleration of the system.

Transcripts

Browse More Related Video

AP Physics Workbook 2.F Direction of Friction

AP Physics Workbook 2.J Modified Atwood Machines

AP Physics 1 Dynamics Review

AP Physics Workbook 2.E Newton's Second and Third Laws

2022 AP Physics 1 Free Response #1

Incline Plane Problems with Friction

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsProblemAtwoodMachineFrictionalForceMechanicalSystemNewtonsLawsKineticFrictionStaticFrictionTensionAnalysisAccelerationCalculationEducationalContent