AP Physics Workbook 2.E Newton's Second and Third Laws
TLDRThis AP Physics workbook solution delves into Unit 2 on dynamics, specifically focusing on Newton's second and third laws. The video outlines a scenario involving two blocks, m1 and m2, being pushed across a smooth surface without friction. The narrator explains how to draw forces on each block, emphasizing the absence of frictional force and the different gravitational forces due to varying masses. The explanation includes the force applied by the push, the reaction forces between the blocks, and the effect of these forces on the blocks' acceleration. The solution also explores how adding a third mass affects the system's acceleration, demonstrating the inverse relationship between total mass and acceleration. Through detailed guidance, the video aids students in understanding the fundamental principles of dynamics and the interconnectedness of forces and motion.
Takeaways
- 📚 The scenario involves two blocks, m1 and m2, being pushed across a smooth surface with an external force F, where m2 > m1.
- 🚫 Friction between the surface and the blocks is negligible, so there is no frictional force to consider in the analysis.
- 📈 Newton's second law is applied to derive the acceleration of the system, with the formula F_push = (m1 + m2) * a_x.
- 🔄 Newton's third law is evident as m2 exerts an equal and opposite force on m1, which is represented as F_2 on m1.
- 🤔 The force applied by the person (F_push) is not the only force acting on m1; m2 also exerts a force on m1, which is crucial for understanding the system's dynamics.
- 🔽 The addition of a third mass (m3) to the system results in a decrease in the overall acceleration due to the increased total mass affecting the denominator of the acceleration formula.
- 📐 The relationship between mass and acceleration is inversely proportional, meaning as the mass of the system increases, the acceleration decreases.
- 🌟 The acceleration of the individual blocks is the same as the acceleration of the system, which is derived from the summation of all internal forces.
- 📝 The quantitative reasoning section guides through the steps of deriving the acceleration for block two by applying Newton's second law to the system.
- 🎯 The key to solving the problem is understanding the interplay between the forces acting on the blocks and how the total mass of the system affects the resulting acceleration.
Q & A
What is the main topic of the video?
-The main topic of the video is the application of Newton's second and third laws of motion in the context of two blocks being pushed across a surface.
What is the scenario presented in the video?
-The scenario involves two blocks of different masses, m1 and m2, being pushed across a smooth surface by an external force F. The mass of block 2 (m2) is greater than that of block 1 (m1), and both blocks begin at rest.
Why is the frictional force neglected in this scenario?
-The frictional force is neglected because the surface is described as being smooth enough to ignore its effect on the motion of the blocks.
How does Newton's third law come into play in this scenario?
-Newton's third law states that for every action, there is an equal and opposite reaction. In this case, as the person applies a force F to move block 1 (m1), block 2 (m2) exerts an equal and opposite force on block 1 due to the third law.
What forces act on block 1 (m1) in the scenario?
-Block 1 (m1) experiences the force of gravity and the normal force, as well as the push force F applied by the person and the equal and opposite force exerted by block 2 (m2) as per Newton's third law.
How does the mass of the blocks affect the gravitational and normal forces?
-The gravitational force and the normal force are proportional to the mass of the blocks. Since block 2 (m2) has a greater mass than block 1 (m1), it experiences a greater gravitational force and normal force.
What is the quantitative reasoning behind deriving the acceleration of block 2 (m2)?
-The quantitative reasoning involves applying Newton's second law, which states that the sum of all internal forces on a system equals the mass of the system times its acceleration. By setting up the equation with the given masses and the applied force, one can solve for the acceleration of block 2 (m2).
What happens to the acceleration when a third mass is added to the system?
-When a third mass is added, the total mass of the system increases, which results in a decrease in acceleration, due to the inverse proportional relationship between acceleration and mass.
How is the acceleration of the individual blocks related to the system's acceleration?
-The acceleration of the individual blocks is the same as the system's acceleration because they are all part of the same system and are accelerated together.
What is the final conclusion regarding the effect of mass on the system's acceleration?
-The final conclusion is that the system's acceleration will decrease when the mass of the system increases, due to the inverse proportional relationship between mass and acceleration.
How can the principles discussed in the video be applied to real-world scenarios?
-The principles of Newton's second and third laws can be applied to various real-world scenarios involving forces and motion, such as in designing mechanical systems, understanding vehicle dynamics, and analyzing the effects of different masses on the motion of objects.
Outlines
📚 Introduction to Newton's Second and Third Laws
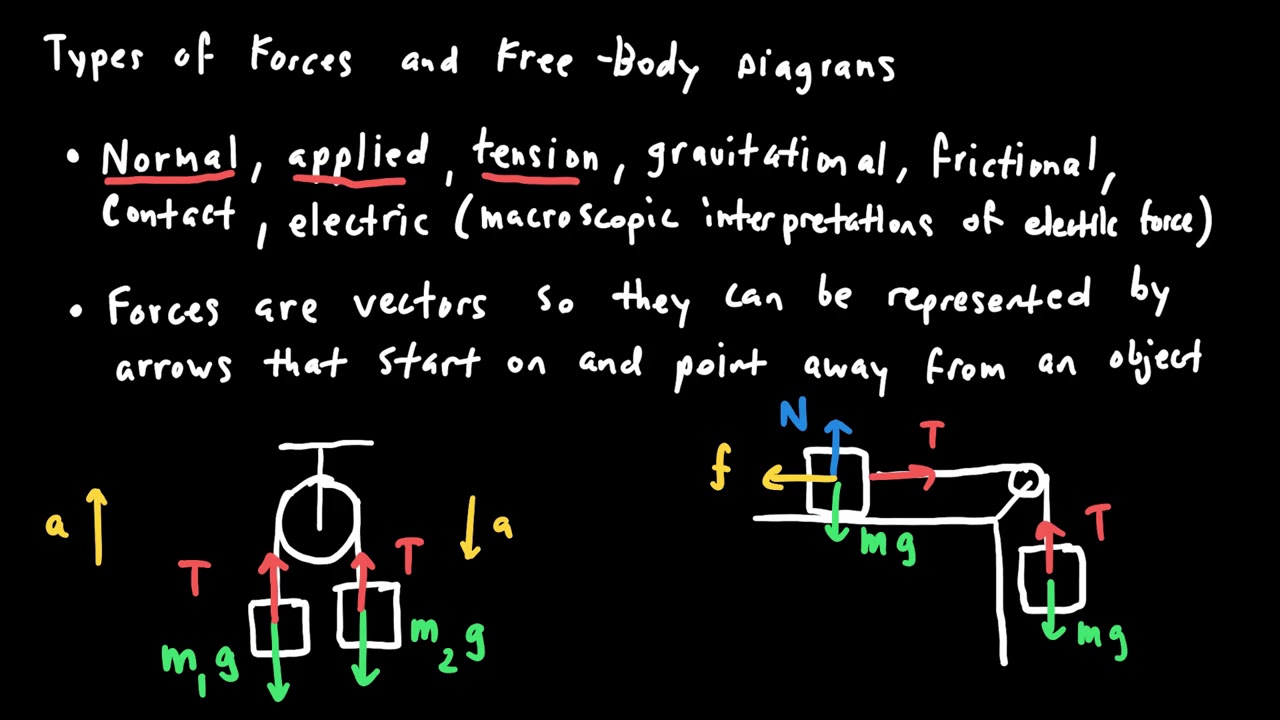
This paragraph introduces the topic of the video, which is focused on unit 2 dynamics, specifically section 2.8 concerning Newton's second and third laws. The scenario involves two blocks being pushed across a smooth surface by an external force F. The blocks, with masses m1 and m2 (m2 > m1), start from rest, and the frictional force is negligible. The task is to draw the forces acting on each block, remembering that friction is not present. The video explains the forces acting on both blocks, including gravity and the normal force, and how these forces relate to the masses of the blocks. It also delves into Newton's third law, explaining the interaction forces between the two blocks, and sets up the groundwork for the quantitative analysis to follow.
🔍 Derivation and Analysis of Acceleration with an Additional Mass
The second paragraph continues the discussion by focusing on the quantitative reasoning behind the acceleration of block 2. It outlines the steps to derive the acceleration using Newton's second law, which states that the net force on a system equals the mass of the system times its acceleration. The video explains how to calculate the system's acceleration by considering the total mass (m1 + m2) and the applied force (F_push). It then addresses the effect of adding a third mass (m3) to the system, explaining that the acceleration will decrease due to the inverse proportional relationship between mass and acceleration. The summary emphasizes that as the system's mass increases, the acceleration decreases, which is true for the individual blocks as well.
Mindmap
Keywords
💡Newton's Second Law
💡Newton's Third Law
💡Friction
💡External Force
💡Mass
💡Acceleration
💡System
💡Inertia
💡Force Diagram
💡Gravitational Force
💡Normal Force
Highlights
The scenario involves two blocks being pushed across a surface with an external force F, with block 2 having a greater mass than block 1.
The surface is smooth enough to neglect the frictional force between the surface and the blocks.
The initial condition of the blocks is at rest.
Gravity and normal forces act on both blocks, with the normal force and gravity being smaller on the block with less mass (m1).
Newton's third law is applied to explain the equal and opposite forces between the two blocks.
The force applied by the person (F_push) is to the right on m1, and the reaction force (F2 on 1) acts on m1 due to m2.
There is no force pushing to the left on the larger block (m2), but there is a force to the right due to the reaction force from m1 (F1 on 2).
The summation of all internal forces of the system equals the mass of the system times its acceleration, as per Newton's second law.
The mass of the system is the sum of the masses of the two blocks (m1 + m2).
The acceleration of the system (ax) is derived by solving for it using the given forces and masses.
The acceleration of block two (a2) is found to be equal to the acceleration of the system (ax).
The addition of a third mass (m3) to the system results in a decrease in the system's acceleration due to the increased total mass.
There is an inverse proportional relationship between the acceleration of the system and its mass.
As the mass of the system increases, the acceleration decreases for the individual blocks as well.
The acceleration of the system remains the same for the individual blocks, even with the addition of a third mass.
The addition of a third mass (m3) inversely affects the acceleration, causing it to decrease.
The comprehensive analysis of the forces and masses provides a clear understanding of the dynamics involved in the system.
The practical application of Newton's laws allows for the prediction and calculation of the system's behavior under varying conditions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: