Introduction to Population Models and Logistic Equation (Differential Equations 31)
TLDRThe video delves into the application of differential equations in modeling population dynamics, focusing on two key scenarios: when birth rates decrease as population grows (logistic growth) and when birth rates are directly proportional to population size. It explains how these scenarios lead to different outcomes, such as carrying capacity, population explosion, or extinction, providing a mathematical framework to understand population changes over time.
Takeaways
- 📈 The video discusses the application of differential equations in modeling population growth and decline, focusing on the relationship between birth rates, death rates, and population changes over time.
- 🔢 The script introduces the concept of equilibrium solutions, critical points, and stability in the context of population dynamics, emphasizing how these factors interplay in determining population trends.
- 🐰 The discussion includes two common scenarios: one where the birth rate decreases as the population increases (logistic growth), and another where the birth rate is directly proportional to the population (exponential growth or decline).
- 🌿 The logistic growth model is explained, where an initial birth rate minus a birth rate proportional to the population results in a logistic equation that describes how populations approach a carrying capacity.
- 💥 The exponential growth scenario is also explored, where a birth rate proportional to the population without a decreasing factor can lead to population explosion or extinction depending on the initial population size.
- 📉 The video emphasizes the importance of understanding how populations work and are modeled by differential equations, as this knowledge is crucial for predicting and managing population changes.
- 🔍 The script provides a detailed mathematical breakdown of how the rate of population change is calculated and how it can lead to different outcomes based on the interplay between birth and death rates.
- 🌐 The content is relevant for those interested in fields such as ecology, biology, and environmental science, where understanding population dynamics is essential.
- 🎓 The video serves as an educational resource for individuals learning about differential equations and their real-world applications, particularly in the context of population biology.
- 📚 The script is a transcript from an educational video, which means it is designed to be accompanied by visual aids and examples that would be present in the actual video content.
Q & A
What is the primary focus of the video?
-The primary focus of the video is to explore the applications of differential equations in modeling population growth and decline, including specific techniques like equilibrium solutions, stability, and critical points.
How do differential equations model population changes?
-Differential equations model population changes by considering the birth rates and death rates, and how these rates are related to the population size and time. The change in population is expressed as the difference between the birth rate and death rate multiplied by the population.
What are the two common ways of population growth and decline discussed in the video?
-The two common ways of population growth and decline discussed are when the birth rate decreases as the population increases (logistic growth) and when the birth rate is directly proportional to the population size (exponential growth or decline).
What is the logistic equation?
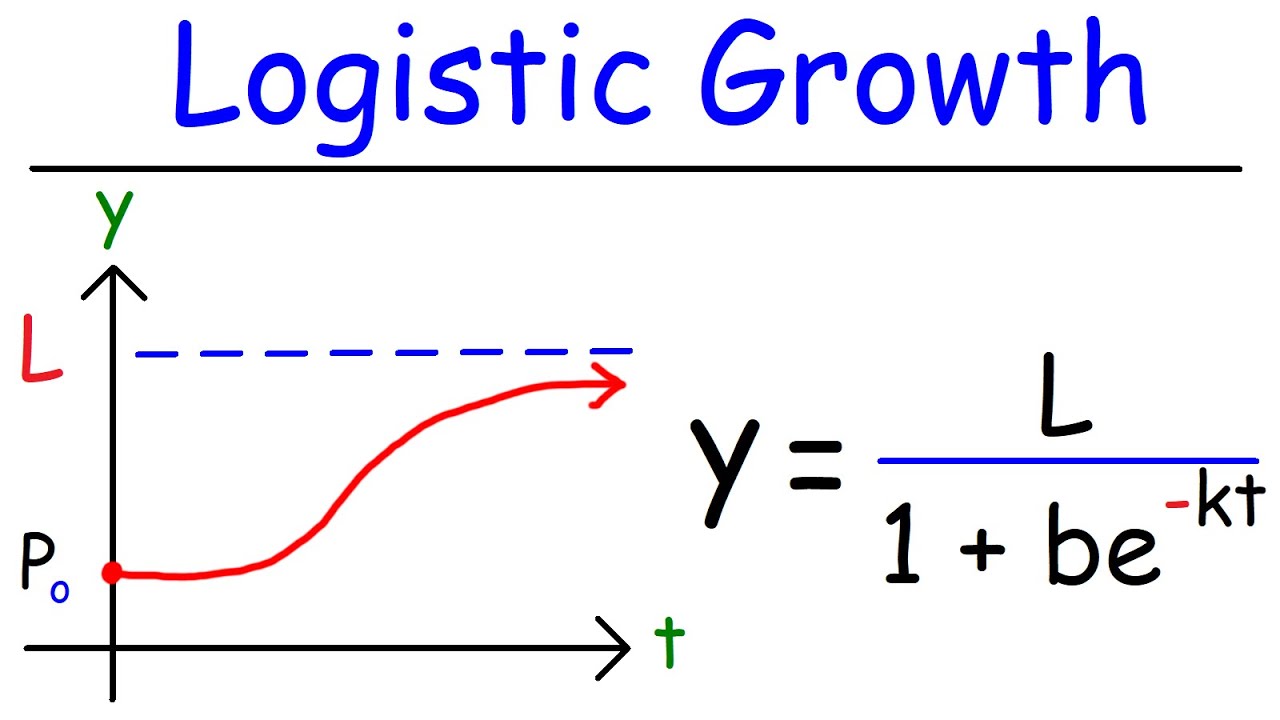
-The logistic equation is a model of population growth that takes into account the carrying capacity or limiting population (M). It is represented as dP/dt = rP(1 - P/M), where r is the intrinsic growth rate and P is the population size.
What happens when the initial population is less than the carrying capacity (M)?
-When the initial population is less than the carrying capacity (M), the population will increase until it reaches M, at which point the growth stops and the population stabilizes at the carrying capacity level.
What happens when the initial population is greater than the carrying capacity (M)?
-When the initial population is greater than the carrying capacity (M), the population will decrease until it reaches M, at which point the population stabilizes at the carrying capacity level.
What occurs in the case of population growth where the birth rate is directly proportional to the population size?
-In the case where the birth rate is directly proportional to the population size, the population will either experience an unbounded growth (explosion) if the initial population is above a certain threshold, or it will go extinct if the initial population is below the threshold.
What is the significance of the threshold (M) in the context of population growth?
-The threshold (M) represents a critical point in population growth. If the initial population is above M, the population will eventually explode without bound. If the initial population is below M, the population will decline towards extinction.
How does the concept of equilibrium solutions relate to population models?
-Equilibrium solutions in population models refer to the stable states where the population neither grows nor declines. These solutions are important for understanding the long-term behavior of populations and can represent carrying capacity or other stable conditions.
What is the role of critical points in population dynamics?
-Critical points in population dynamics are the specific values of the population size at which the growth rate changes its sign. They can indicate the onset of growth or decline and are crucial for predicting the behavior of populations over time.
Outlines
📚 Introduction to Differential Equations and Population Modeling
The video begins with an introduction to the use of differential equations in modeling population dynamics. It explains that while previous videos focused on solving differential equations, this one will explore specific applications, such as population growth and decline. The video sets the stage for discussing equilibrium solutions, stability, critical points, and how these concepts apply to real-world population scenarios. It emphasizes the importance of understanding how populations work and how they can be modeled mathematically, starting with the basics of how populations change due to birth and death rates.
📈 Understanding Population Change through Birth and Death Rates
This paragraph delves into the specifics of how populations change over time, focusing on the relationship between birth rates, death rates, and population dynamics. It explains that the change in population is represented by the difference between the birth rate and death rate, and how these rates are proportional to the population size and time. The video clarifies the concept of birth and death rates per unit of population per unit of time, and how these rates can vary depending on the population size and available resources.
📊 Two Key Cases of Population Dynamics
The speaker introduces two critical cases of population dynamics: one where the birth rate decreases as the population increases, leading to a logistic growth model, and another where the birth rate is directly proportional to the population, which can result in population explosion or extinction. The paragraph discusses the implications of these two scenarios, highlighting the importance of understanding how birth and death rates interact with the carrying capacity of the environment to shape population trends.
🌿 Logistic Equation and Population Thresholds
This section focuses on the logistic equation, which models population growth when there is a carrying capacity limit. The video explains how the logistic equation is derived when the birth rate decreases as the population increases, and how it leads to an equilibrium population size (M). It also discusses the concepts of limiting population (when starting below the threshold) and carrying capacity (when starting above the threshold), and how these relate to the stability and sustainability of populations.
💥 Population Explosion and Extinction Scenarios
The video explores scenarios where birth rates are directly proportional to the population size, leading to either population explosion or extinction. It explains how, in the absence of a decreasing birth rate with increasing population, the population can grow without bound (explosion) if the initial population is above a certain threshold (M), or decrease towards zero (extinction) if the initial population is below this threshold. The speaker emphasizes the mathematical reasoning behind these outcomes and their ecological implications.
🧠 Deep Dive into Differential Equations and Population Modeling
The speaker concludes by summarizing the key points discussed in the video, emphasizing the relationship between birth rates, death rates, and population change. It reiterates the importance of understanding the logistic equation and the concept of a threshold (M) in population dynamics. The video also previews upcoming content, which will include more detailed examples and exploration of population explosion and extinction, as well as further examination of the logistic equation.
Mindmap
Keywords
💡Differential Equations
💡Population Growth
💡Birth Rate
💡Death Rate
💡Equilibrium Solutions
💡Stability
💡Critical Points
💡Carrying Capacity
💡Logistic Equation
💡Extinction
Highlights
The video discusses the application of differential equations in modeling population growth and decline.
The presenter introduces the concept of equilibrium solutions, stability, and critical points in population dynamics.
Population changes are generally due to birth rates and death rates, which can be expressed as births minus deaths.
Birth and death rates are defined per unit of population per unit of time, making them dependent on both population size and time.
The rate of population change can be modeled by the difference between the birth rate and death rate times the population size.
Two common cases of population dynamics are explored: one where the birth rate decreases as the population increases, and another where the birth rate is proportional to the population size.
The logistic equation is derived for the case where the birth rate decreases as the population increases, leading to a carrying capacity or limiting population.
The concept of a threshold population size is introduced, where if the initial population is greater than the threshold, a population explosion occurs, and if less, the population heads towards extinction.
The video emphasizes the importance of understanding how population dynamics are modeled by differential equations for predicting future population trends.
The presenter uses mathematical derivations to explain why populations reach a carrying capacity or experience extinction or explosion based on birth and death rates.
The logistic equation is a key mathematical model used to describe population growth when resources are limited.
The video provides a detailed explanation of how the logistic equation is derived and its implications for population dynamics.
The presenter explains the concept of a threshold in population dynamics, which acts as a tipping point for either growth or decline.
The video demonstrates how the relationship between birth and death rates can lead to different outcomes for population growth, such as bounded growth, explosion, or extinction.
The presenter uses the concept of partial fractions to solve a differential equation related to population dynamics.
The video concludes with a discussion on how the logistic equation can be used to predict population behavior over time.
Transcripts
Browse More Related Video

Population Growth and Decline (Differential Equations 35)

Birth Rates and Death Rates in Differential Equations (Differential Equations 33)
Logistic Growth Function and Differential Equations

Worked example: Logistic model word problem | Differential equations | AP Calculus BC | Khan Academy

Basic Population Models in Differential Equations (Differential Equations 32)

Introduction to Time Rate of Change (Differential Equations 5)
5.0 / 5 (0 votes)
Thanks for rating: