How Schrodinger Came Up With His Famous Equation (But EASIER)

Parth G
14 Nov 202310:35
EducationalLearning
32 Likes 10 Comments

TLDRThe video script offers an intuitive derivation of the Schrödinger equation, a cornerstone of quantum physics, starting from the classical electromagnetic wave equation. It explains how the equation's solutions relate to the wave-particle duality and the mass-energy equivalence. The script then transitions to the Klein-Gordon equation for massive particles and shows how the Schrödinger equation emerges when considering non-relativistic scenarios. This derivation connects the abstract quantum mechanics with classical physics, providing a logical and mathematical pathway to understanding the Schrödinger equation.

Takeaways
  • 🌟 The Schrödinger equation is a fundamental equation in quantum physics with a derivation that is not widely discussed.
  • 🎓 The video aims to provide an intuitive derivation of the Schrödinger equation, step by step.
  • 🔍 The derivation is not identical to the one Schrödinger used but is based on principles from classical physics and the mass-energy equivalence relation.
  • 📈 The electromagnetic wave equation and its sinusoidal solutions are used as a starting point to understand wave behavior.
  • 🌊 A specific solution of the electromagnetic wave equation reveals the relationship between a photon's energy and momentum.
  • 💡 The mass-energy equivalence relation (E=mc²) is a key component in understanding the relationship between energy and momentum for massless particles like photons.
  • 🚀 The Klein-Gordon equation is introduced as the wave equation for particles with mass and is derived from the mass-energy equivalence relation.
  • 📉 By considering non-relativistic scenarios (low speeds), the Klein-Gordon equation can be simplified to the Schrödinger equation.
  • 🌐 The Schrödinger equation describes the wave functions of particles with mass and is applicable in scenarios where relativistic effects are negligible.
  • 🧠 The derivation of the Schrödinger equation from classical wave equations and the mass-energy equivalence shows it is not a product of mere conjecture but has a theoretical basis.
  • 📚 The video encourages further exploration of these concepts through linked resources and suggests that there are multiple ways to derive the Schrödinger equation.
Q & A

  • What is the Schrödinger equation?

    -The Schrödinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time.

  • Why is the derivation of the Schrödinger equation important to understand?

    -Understanding the derivation of the Schrödinger equation provides insight into the theoretical foundations of quantum physics and helps clarify that it is not a concept pulled out of thin air, but rather a result of logical mathematical progressions.

  • What is the electromagnetic wave equation?

    -The electromagnetic wave equation describes the behavior of electromagnetic waves, such as light, x-rays, and radio waves, within the universe.

  • How is the electromagnetic wave equation related to the Schrödinger equation?

    -The electromagnetic wave equation serves as a starting point for deriving the Schrödinger equation. A specific solution of the electromagnetic wave equation is used to establish a relationship between energy and momentum, which is then applied to particles with mass to derive the Schrödinger equation.

  • What is the significance of the mass-energy equivalence relationship in the context of the Schrödinger equation?

    -The mass-energy equivalence relationship, represented by E=mc², is a fundamental principle that relates the energy of a photon to its momentum. This relationship is crucial in deriving the Schrödinger equation, as it is used to establish a similar relationship for particles with mass.

  • What is the Klein-Gordon equation?

    -The Klein-Gordon equation is a relativistic wave equation used to describe particles with mass that are free to move, meaning they can have momentum.

  • How does the Klein-Gordon equation lead to the Schrödinger equation?

    -By applying the mass-energy equivalence relationship to the Klein-Gordon equation and considering non-relativistic scenarios (low speeds), the equation simplifies to the Schrödinger equation.

  • What are the limitations of the Schrödinger equation in dealing with relativistic effects?

    -The Schrödinger equation is not suitable for dealing with relativistic effects, which become noticeable at high speeds. For such cases, more advanced equations like the Klein-Gordon and Dirac equations are required.

  • How does the video script help in understanding the logical progression from classical physics to quantum mechanics?

    -The video script walks through the derivation of the Schrödinger equation starting from a classical wave equation, highlighting the logical steps and mathematical relationships that connect classical physics concepts to the principles of quantum mechanics.

  • What is the significance of the wave-particle duality in the context of the script?

    -Wave-particle duality is a key concept in quantum mechanics that is illustrated in the script by discussing how electromagnetic waves can be described by sinusoidal wave functions, which are also applicable to particles with mass when considering their wave functions.

  • What are the practical implications of the Schrödinger equation?

    -The Schrödinger equation is essential for predicting and understanding the behavior of quantum systems, which has applications in various fields including chemistry, materials science, and technology, such as in the development of quantum computers and understanding atomic and molecular structures.

Outlines
00:00
🌟 Intuitive Derivation of the Schrodinger Equation

This paragraph introduces the topic of the video, which is an intuitive derivation of the Schrodinger equation, a fundamental equation in quantum physics. The speaker, Spart, acknowledges that the derivation process is not commonly discussed and aims to fill this gap. The video also briefly touches on the historical context of the equation's discovery by Erwin Schrodinger and mentions an alternative derivation found in a paper by David W. Ward of Harvard and Saine Vulmer of MIT. The speaker sets the stage for a step-by-step explanation that aims to demystify the equation's origin.

05:01
📈 Understanding Electromagnetic Waves and Photons

The second paragraph delves into the specifics of the electromagnetic wave equation, focusing on a particular solution that describes how electromagnetic waves, such as light and X-rays, behave. The speaker explains the wave-particle duality and how the electric field fluctuates over space and time. The paragraph establishes the relationship between a photon's energy and momentum, which is a crucial step in understanding the mass-energy equivalence principle. This principle, encapsulated by Einstein's famous equation E=mc², is shown to be a special case of a more general relationship that applies to all objects, both with and without mass.

10:02
🚀 From Electromagnetic Waves to Massive Particles

In this paragraph, the speaker transitions from discussing electromagnetic waves to particles with mass. The Klein-Gordon equation is introduced as the wave equation that describes massive particles and accounts for relativistic effects. The speaker explains the process of reducing the Klein-Gordon equation to non-relativistic scenarios, which leads to the Schrodinger equation. This derivation provides a logical and mathematical pathway from classical physics to quantum mechanics, showing that the Schrodinger equation is not a product of mere conjecture but has a solid theoretical foundation.

Mindmap
Keywords
💡Schrodinger Equation
The Schrodinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. In the video, it is presented as not just a product of imagination but a result of logical mathematical steps, derived from classical physics equations and the mass-energy equivalence principle.
💡Quantum Physics
Quantum physics is the branch of physics that explores the behavior and interactions of particles at the smallest scales, where classical physics no longer adequately describes the phenomena. The Schrodinger Equation is a cornerstone of this field, as it helps describe the behavior of quantum systems.
💡Electromagnetic Wave Equation
The electromagnetic wave equation is a fundamental equation in classical physics that describes the propagation of electromagnetic waves, such as light, X-rays, and radio waves. It serves as a starting point in the video for deriving the Schrodinger Equation by analogy.
💡Wave-Particle Duality
Wave-particle duality is a key concept in quantum mechanics that states that particles such as photons can exhibit both wave-like and particle-like properties. This duality is illustrated in the video through the behavior of the electric field in a wave equation solution.
💡Mass-Energy Equivalence
The mass-energy equivalence principle, encapsulated by Einstein's famous equation E=mc², states that the energy of an object is directly proportional to its mass. This principle is crucial in understanding the relationship between energy and momentum for massless particles like photons.
💡Klein-Gordon Equation
The Klein-Gordon equation is a relativistic wave equation used to describe particles with mass that are free to move, meaning they can possess momentum. It is a step in the derivation of the Schrodinger Equation, showing how the equation changes when considering objects with mass.
💡Relativistic Effects
Relativistic effects refer to the phenomena that occur when particles move at speeds close to the speed of light, leading to counterintuitive behaviors as described by the theory of relativity. The Klein-Gordon equation accounts for these effects for massive particles.
💡Non-Relativistic Scenarios
Non-relativistic scenarios refer to situations where objects are moving at speeds much slower than the speed of light, and thus the effects of relativity are negligible. The Schrodinger Equation is derived for such scenarios, making it applicable to quantum systems at low speeds.
💡Wave Functions
Wave functions are mathematical descriptions in quantum mechanics that provide information about the probability amplitude of a particle's location or other physical properties. They are central to the interpretation of quantum mechanics and are solutions to the Schrodinger Equation.
💡Energy-Momentum Relationship
The energy-momentum relationship is a fundamental concept in physics that connects the energy and momentum of an object. In the context of the video, it is used to describe the relationship for massless particles like photons and is then generalized for objects with mass.
Highlights

The Schrodinger equation is considered the most important equation in quantum physics.

The derivation of the Schrodinger equation is not widely discussed.

This video aims to provide an intuitive derivation of the Schrodinger equation.

The derivation is based on a paper by David W.ard of Harvard and Saine Vulmer of MIT.

The starting point is the electromagnetic wave equation from classical physics.

A specific solution of the electromagnetic wave equation is analyzed.

The solution involves the electric field fluctuating between specific values over space and time.

The wave equation's solution is confirmed by substituting it back into the equation.

The relationship between a photon's energy and momentum is derived from the solution.

The mass-energy equivalence relation is introduced, applicable to massless objects like photons.

The Klein-Gordon equation is used to describe particles with mass and momentum.

The Klein-Gordon equation accounts for relativistic effects at high speeds.

The Schrodinger equation is derived by applying the mass-energy equivalence to non-relativistic scenarios.

The derivation shows the Schrodinger equation did not come out of thin air but has a theoretical foundation.

The video provides a logical step-by-step journey from classical wave equations to quantum mechanics.

The Schrodinger equation is well-tested and verified by experimental results.

Transcripts

Browse More Related Video

Schrodinger Equation - A simple derivation

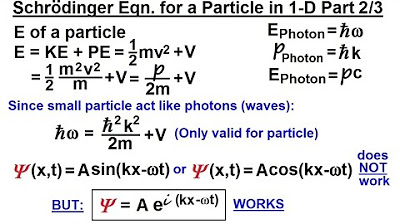
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (6 of 92) The Schrodinger Eqn. in 1-D (2/3)

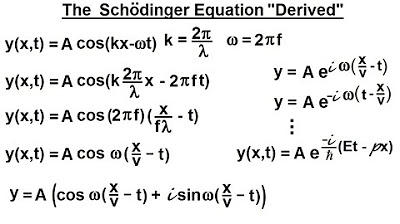
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (4 of 92) The Schrodinger Eqn. "Derived"

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (3 of 92) The Wave Equation

Visualization of Quantum Physics (Quantum Mechanics)

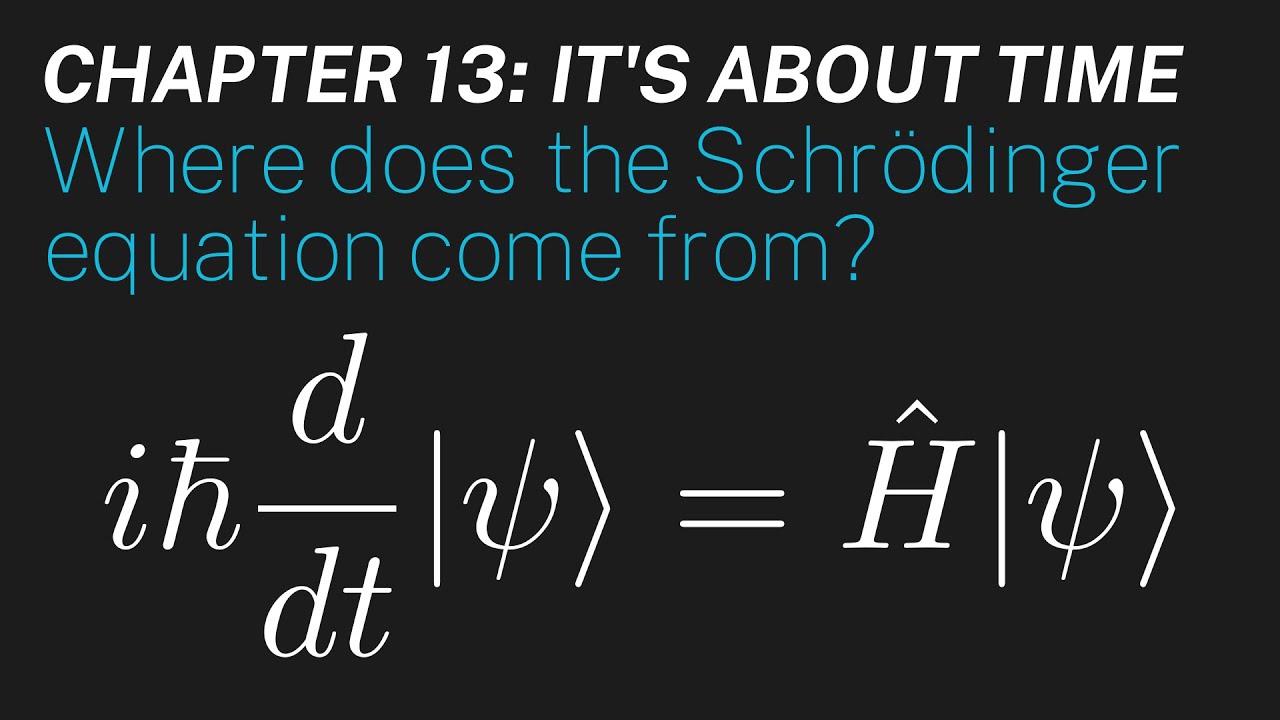
Ch 13: Where does the Schrödinger equation come from? | Maths of Quantum Mechanics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumPhysicsSchrodingerEquationWaveEquationClassicalPhysicsIntuitiveDerivationMassEnergyEquivalenceKleinGordonEquationRelativisticEffectsNonRelativisticScenariosPhysicsEducation