Ch 13: Where does the Schrödinger equation come from? | Maths of Quantum Mechanics

Quantum Sense
3 Feb 202314:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video script, the presenter delves into the concept of time evolution in quantum mechanics and guides the audience through the derivation of the Schrödinger equation. Starting with the basics of quantum states and their evolution, the script introduces the time evolution operator and its properties, such as unitarity and the conservation of probability. The presenter then employs physical intuition and mathematical rigor to derive the differential equation governing the time evolution of quantum states. The connection to classical physics and the role of energy as the generator of time evolution are highlighted, leading to the identification of the Hamiltonian operator. The script concludes with the formulation of the complete Schrödinger equation, emphasizing the importance of each term and the physical principles behind them.

Takeaways
  • 🌟 The concept of time evolution in quantum mechanics involves how a quantum state changes over time due to interactions with fields or other particles.
  • 🔄 The time evolution operator (denoted as U) is responsible for evolving the quantum state forward in time, with U(0) being the identity operator as it does not change the state.
  • ↔️ Time evolution is expected to be reversible, meaning that evolving a state forward and then backward in time should return the original state, implying the existence of U's inverse.
  • 💡 The total probability of a quantum state is conserved over time, which is a property satisfied by unitary operators.
  • 📈 The unitarity of the time evolution operator is derived from physical intuitions of reversibility and probability conservation.
  • 🎯 The Schrodinger equation is derived from the principles of unitary time evolution and the conservation of probability.
  • 🔢 The equation is a differential equation that describes the time evolution of a quantum state, with the time derivative of the state being equal to the derivative of the time evolution operator acting on the state.
  • 🌀 The derivative of the time evolution operator is anti-Hermitian, leading to the conclusion that it is related to a Hermitian operator when multiplied by the imaginary unit i.
  • 🔋 The Hamiltonian operator, representing energy, is introduced as the observable related to time evolution, based on the classical physics principle that energy generates time evolution.
  • 📏 The reduced Planck constant is introduced to ensure unit consistency between the time derivative of the quantum state and the Hamiltonian operator.
  • 🎓 Understanding the Schrodinger equation requires a combination of mathematical derivation and physical principles, highlighting the interplay between mathematics and physical intuition in quantum mechanics.
Q & A

  • What is the main focus of this episode?

    -The main focus of this episode is to connect the concept of generators in classical physics to quantum mechanics and derive the Schrödinger equation.

  • What does the time evolution operator do in quantum mechanics?

    -The time evolution operator, denoted as 'U', takes an initial quantum state and evolves it forward in time by a certain amount, 't'. For example, U(2s) would evolve the quantum state by two seconds.

  • What property does the time evolution operator have when 't' is zero?

    -When 't' is zero, the time evolution operator does not change the quantum state. Therefore, U(0) is the identity operator.

  • Why is time evolution in quantum mechanics expected to be invertible?

    -Time evolution is expected to be invertible because if a particle is evolved forward in time by two seconds, it should be possible to evolve it backward by the same amount and return to the initial state.

  • What is the significance of the unitary operator in the context of quantum state evolution?

    -Unitary operators are significant because they maintain the total probability of the quantum state, ensuring it remains equal to 1 even after the state has been evolved in time. This property is crucial for the conservation of probability in quantum mechanics.

  • How does the video derive that the time evolution operator is unitary?

    -The video derives that the time evolution operator is unitary by using physical intuition that time evolution is reversible and probability is conserved. It shows that the operator Udagger U equals the identity operator, which implies unitarity.

  • What is the role of the Hamiltonian operator in the Schrödinger equation?

    -The Hamiltonian operator represents the energy of the system and is the observable that generates time evolution in quantum mechanics. It is introduced into the Schrödinger equation based on the classical physics principle that energy generates changes in time.

  • Why is the imaginary unit 'i' used in the Schrödinger equation?

    -The imaginary unit 'i' is used in the Schrödinger equation to maintain the conservation of probability, which is a fundamental requirement in quantum mechanics. The unitary nature of time evolution, derived from probability conservation, necessitates the use of 'i'.

  • What is the significance of the reduced Planck constant in the Schrödinger equation?

    -The reduced Planck constant is introduced to match the units of the equation. It has units of joule seconds (energy times seconds) and is determined experimentally to ensure that the units on both sides of the equation are consistent.

  • How does the video connect classical physics to the derivation of the Schrödinger equation?

    -The video connects classical physics to the derivation of the Schrödinger equation by using the principle that energy generates changes in time, which is a concept from classical physics. This principle is used to identify the hermitian operator in the equation as the energy operator or Hamiltonian.

  • What is the general form of the Schrödinger equation derived in the video?

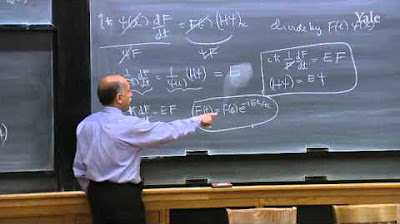
    -The general form of the Schrödinger equation derived in the video is i times the time derivative of the quantum state is equal to the Hamiltonian operator (energy operator) acting on the state, which is represented as iħ(∂/∂t)|ψ> = H|ψ>.

Outlines
00:00
🌟 Introduction to Quantum Time Evolution

This paragraph introduces the concept of time evolution in quantum mechanics, focusing on how a quantum state changes over time due to interactions with fields or other particles. The speaker aims to derive the Schrodinger equation and explains the need for a time evolution operator, denoted as 'U', which evolves the quantum state forward in time. The paragraph also discusses the properties of the time evolution operator, such as its identity at zero time and its invertibility, hinting at the unitary nature of time evolution and setting the stage for the derivation of the Schrodinger equation.

05:00
🔍 Deriving Unitarity of Time Evolution

The speaker delves into the physical intuition behind the unitarity of time evolution, explaining that it should be reversible and that the total probability should be conserved. The paragraph presents a mathematical proof to show that the time evolution operator 'U' is unitary, using the properties of hermitian and unitary operators. The derivation relies on the principles that time evolution is reversible and probability is conserved, which are essential for understanding the Schrodinger equation. The unitarity is confirmed by showing that the time evolution operator's derivative is antihermitian, leading to the conclusion that the time evolution operator is indeed unitary.

10:04
📈 The Schrodinger Equation: A Quantum Milestone

In this paragraph, the speaker completes the derivation of the Schrodinger equation by connecting the unitary time evolution to a physical observable, the Hamiltonian operator, which represents energy. The speaker explains that energy is the generator of time evolution, drawing from classical physics. The paragraph also addresses the need to introduce the reduced Planck constant to match units between the time derivative and the Hamiltonian operator. The speaker concludes by recapping the derivation and the significance of each term in the Schrodinger equation, emphasizing the importance of the equation in physics and the principles that underpin it.

Mindmap
Keywords
💡Generators
Generators, in the context of the video, refer to physical quantities that drive changes in a system over time. Specifically, energy is identified as the generator of changes in classical physics, meaning it is responsible for the evolution of the physical state of a system with time. This concept is crucial for understanding how the Schrodinger equation is derived, as it connects classical physics to quantum mechanics.
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior and interactions of particles at the atomic and subatomic level. It provides a framework for understanding phenomena that cannot be explained by classical physics. In the video, quantum mechanics is the domain where the Schrodinger equation operates, modeling the time evolution of quantum states and particles' interactions with fields or other particles.
💡Time Evolution
Time evolution in quantum mechanics refers to the process by which a quantum system changes over time due to interactions with other systems or fields. The video explains that the goal is to find out exactly how the quantum state changes as time progresses. This concept is central to the derivation of the Schrodinger equation, which describes the time evolution of quantum states.
💡Quantum State
A quantum state is a mathematical representation of the condition of a quantum system, which can be a particle or a collection of particles. It includes all the information needed to predict the probabilities of various measurements. In the video, the quantum state is represented as a 'ket' in a vector space, and the time evolution of this state is the focus of the Schrodinger equation.
💡Schrodinger Equation
The Schrodinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is a partial differential equation and is used to predict the behavior of quantum systems. In the video, the derivation of the Schrodinger equation is the main objective, with the explanation of how it connects classical physics principles to quantum mechanics.
💡Time Evolution Operator
The time evolution operator, denoted as 'U' in the video, is a mathematical operator that describes how a quantum state changes over time. It takes an initial quantum state and evolves it forward by a certain amount of time. The properties of this operator, such as being unitary and invertible, are essential in the derivation of the Schrodinger equation.
💡Unitary Operator
A unitary operator is a type of linear operator that preserves the inner product of any two vectors in the vector space it acts upon. This means that the total probability of a quantum state, calculated as the inner product with itself, remains constant after the operator is applied. In the video, the time evolution operator is shown to be unitary, which is crucial for conserving probability in quantum mechanics.
💡Hermitian Operator
A Hermitian operator, also known as a self-adjoint operator, is a linear operator that is equal to its own adjoint when operating on an appropriate domain of vectors. In quantum mechanics, Hermitian operators correspond to observable quantities because their eigenvalues are real numbers. The video explains that the time evolution operator's derivative is anti-Hermitian, which leads to the identification of the Hamiltonian operator.
💡Hamiltonian Operator
The Hamiltonian operator, often denoted by 'H', is a Hermitian operator in quantum mechanics that represents the total energy of a system. It is derived from the classical Lagrangian and is used to describe the dynamics of a system through the Schrodinger equation. In the video, the Hamiltonian is identified as the operator that drives the time evolution of quantum states.
💡Reduced Planck Constant
The reduced Planck constant, denoted by 'ħ', is a fundamental constant in quantum mechanics that relates the energy of a quantum state to its frequency. It is introduced in the Schrodinger equation to ensure that the units on both sides of the equation match, with 'ħ' having units of energy times seconds (joule-seconds).
💡Probability Conservation
Probability conservation is a principle in quantum mechanics that states that the total probability of all possible outcomes of a measurement on a quantum system must always equal one. This principle is essential for ensuring that the evolution of quantum states remains consistent with the probabilistic nature of quantum mechanics. The unitary nature of the time evolution operator ensures that probability is conserved over time.
Highlights

The episode aims to connect classical physics concepts to quantum mechanics, specifically focusing on the derivation of the Schrödinger equation.

In quantum mechanics, the time evolution of a quantum state is represented by a quantum state ket in a vector space.

The time evolution operator, denoted as U, is introduced to model the change in quantum states over time.

Physical intuition is used to determine properties of the time evolution operator, such as U(0) being the identity operator and the expectation of time evolution being reversible.

The conservation of total probability in a quantum state over time leads to the property of unitarity for the time evolution operator.

The unitarity of the time evolution operator is derived through mathematical proof, relying on the conservation of probability and reversibility of time evolution.

The Taylor expansion of the time evolution operator is used to simplify the problem of finding the operator's action over arbitrary time intervals.

By examining the Taylor expansion and unitary property, it is found that the derivative of the time evolution operator is anti-Hermitian.

The Schrödinger equation is derived from the general form of the time evolution of a quantum state, using principles of unitarity and physical observables.

The Hamiltonian operator, related to energy, is identified as the Hermitian operator in the Schrödinger equation through classical physics intuition.

The reduced Planck constant is introduced to match the units of the Schrödinger equation, determined through experimental means.

The episode emphasizes that the derivation of the Schrödinger equation relies on physical principles and cannot be derived purely from mathematics.

The imaginary unit 'i' in the Schrödinger equation is justified by the need to conserve probability in quantum mechanics.

The video concludes by recapping the derivation and significance of each term in the Schrödinger equation, providing a deeper understanding of its components.

The series will continue in the next episode by deriving the momentum operator and explaining the position basis Schrödinger equation.

The video is math-heavy and provides a rigorous derivation of one of the most important equations in physics, making it accessible to viewers.

Transcripts

Browse More Related Video

Lecture 9 | Quantum Entanglements, Part 1 (Stanford)

Lecture 9 | The Theoretical Minimum

24. Quantum Mechanics VI: Time-dependent Schrödinger Equation

Lecture 5 | The Theoretical Minimum

Lecture 4 | The Theoretical Minimum

Lecture 8 | Quantum Entanglements, Part 1 (Stanford)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsSchrodingerEquationTimeEvolutionUnitarityProbabilityConservationPhysicsDerivationEducationalContentMathematicalConceptsQuantumJourneyPhysicsSeries